Deck 25: Analysis of Variance

Question 1

A consumer interest group is interested in comparing the speed of four different desktop printers; four different documents are used for the experiment.Each test consists of randomly assigning one printer to one of the documents.The document is printed and the time to print in seconds is recorded.Each printer/document combination is tested.How many degrees of freedom does the treatment sum of squares have? How about the error sum of squares?

A)df-treatment = 3; df-error = 15
B)df-treatment = 4; df-error = 15
C)df-treatment = 3; df-error = 13
D)df-treatment = 3; df-error = 12
E)df-treatment = 4; df-error = 12

Answer 1

df-treatment = 3; df-error = 12

Question 2

An industrial engineer wants to test the effect of three different ways of assembling a part on the total assembly time.Five people are randomly assigned to each of the three assembly methods,and the total assembly time (in seconds)is recorded.How many degrees of freedom does the treatment sum of squares have? How about the error sum of squares?

A)df-treatment = 2; df-error = 14
B)df-treatment = 3; df-error = 12
C)df-treatment = 2; df-error = 12
D)df-treatment = 2; df-error = 13
E)df-treatment = 3; df-error = 14

Answer 2

df-treatment = 2; df-error = 12

Question 3

A mechanical engineer is studying bonding issues with the thermal protection tiles on the Space Shuttle.He wants to see whether there is any difference in the bonding strength between three different compounds.Fifteen tiles are randomly assigned to one of the three compounds so that each compound is tested on five tiles.Each tile is coated with its assigned compound and bonded to a surface.After a period of time,pull-tests are performed to determine the amount of force needed to break the bond.How many degrees of freedom does the treatment sum of squares have? How about the error sum of squares?

A)df-treatment = 2; df-error = 12
B)df-treatment = 3; df-error = 12
C)df-treatment = 2; df-error = 14
D)df-treatment = 3; df-error = 14
E)df-treatment = 2; df-error = 13

Answer 3

df-treatment = 2; df-error = 12

Question 4

A chemist is interested in comparing four different catalysts by measuring reaction times.Each catalyst is tested five times,with each experiment randomly assigned one of the four catalysts.How many degrees of freedom does the treatment sum of squares have? How about the error sum of squares?

A)df-treatment = 4; df-error = 16
B)df-treatment = 3; df-error = 16
C)df-treatment = 4; df-error = 19
D)df-treatment = 3; df-error = 19
E)df-treatment = 3; df-error = 17

Question 5

An independent research firm conducts a study to compare the taste of four new sports drinks.Five people are randomly assigned to each of the four drinks.Each person tastes the drink and judges it on a scale from one to five.

A)H0: μ1 = μ2 = μ3 = μ4 = μ5 vs.the alternative that not all the person mean scores are equal
B)H0: μdrink = μper vs.the alternative that the drink and person mean scores are not equal
C)H0: μ1 = μ2 = μ3 = μ4 vs.the alternative that not all the drink mean scores are equal
D)H0: μ1 ≠ μ2 ≠ μ3 ≠ μ4 vs.the alternative that all the drink mean scores are equal
E)H0: μ1 ≠ μ2 ≠ μ3 ≠ μ4 ≠ μ5 vs.the alternative that all the person mean scores are equal

Question 6

An independent research firm conducts a study to compare the taste of four new sports drinks.Five people are randomly assigned to each of the four drinks.Each person tastes the drink and judges it on a scale from one to five.How many degrees of freedom does the treatment sum of squares have? How about the error sum of squares?

A)df-treatment = 3; df-error = 19
B)df-treatment = 3; df-error = 17
C)df-treatment = 4; df-error = 17
D)df-treatment = 3; df-error = 16
E)df-treatment = 4; df-error = 16

Question 7

A hunting supply store is interested in knowing whether three different brands of goose decoys are equally effective in attracting geese.Each goose decoy is randomly assigned to five hunters,and the order of each experiment is also randomized.An experiment consists of a hunter using one goose decoy and waiting fifteen minutes.The number of geese attracted by the decoy is counted.

A)H0: μ1 ≠ μ2 ≠ μ3 vs.the alternative that all decoy brand mean number of geese are equal
B)H0: μ1 ≠ μ2 ≠ μ3 ≠ μ4 ≠ μ5 vs.the alternative that all hunter mean number of geese are equal
C)H0: μ1 = μ2 = μ3 vs.the alternative that not all decoy brand mean number of geese are equal
D)H0: μ1 = μ2 = μ3 = μ4 = μ5 vs.the alternative that not all hunter mean number of geese are equal
E)H0: μbrand ≠ μhunter vs.the alternative that decoy brand and hunter mean number of geese are equal

Question 8

The Professional Golfers' Association (PGA)wants to know which brand of golf ball goes the farthest when hit by a driver.Three brands of golf balls are compared by using "Iron Byron" to randomly hit each brand of ball five times.The total distance in yards is measured after each ball comes to rest.How many degrees of freedom does the treatment sum of squares have? How about the error sum of squares?

A)df-treatment = 2; df-error = 12
B)df-treatment = 2; df-error = 14
C)df-treatment = 3; df-error = 12
D)df-treatment = 3; df-error = 14
E)df-treatment = 2; df-error = 15

Question 9

An industrial engineer wants to test the effect of three different ways of assembling a part on the total assembly time.Five people are randomly assigned to each of the three assembly methods,and the total assembly time (in seconds)is recorded.

A)H0: μ1 = μ2 = μ3 = μ4 = μ5 vs.the alternative that not all the person mean times are equal
B)H0: μ1 = μ2 = μ3 vs.the alternative that not all assembly method mean times are equal
C)H0: μ1 ≠ μ2 ≠ μ3 vs.the alternative that all assembly method mean times are equal
D)H0: μmeth = μper vs.the alternative that the method and person mean times are not equal
E)H0: μ1 ≠ μ2 ≠ μ3 ≠ μ4 ≠ μ5 vs.the alternative that all the person mean times are equal

Question 10

An electrical engineer wants to compare the resistance of four types of wire made from different materials.Five wires of equal lengths and cross sectional areas are prepared for each of the four wire types.Each section of wire is randomly tested by sending a current through the wire and measuring the resistance.How many degrees of freedom does the treatment sum of squares have? How about the error sum of squares?

A)df-treatment = 4; df-error = 19
B)df-treatment = 3; df-error = 17
C)df-treatment = 3; df-error = 16
D)df-treatment = 4; df-error = 20
E)df-treatment = 3; df-error = 19

Question 11

A consumer interest group is interested in comparing the speed of four different desktop printers; four different documents are used for the experiment.Each test consists of randomly assigning one printer to one of the documents.The document is printed and the time to print in seconds is recorded.Each printer/document combination is tested.

A)H0: μ1 = μ2 = μ3 = μ4 vs.the alternative that not all the document mean times are equal
B)H0: μ1 = μ2 = μ3 = μ4 vs.the alternative that not all the printer mean times are equal
C)H0: μ1 ≠ μ2 ≠ μ3 ≠ μ4 vs.the alternative that all the printer mean times are equal
D)H0: μ1 ≠ μ2 ≠ μ3 ≠ μ4 vs.the alternative that all the document mean times are equal
E)H0: μprint ≠ μdoc vs.the alternative that printer and document mean times are equal

Question 12

A hunting supply store is interested in knowing whether three different brands of goose decoys are equally effective in attracting geese.Each goose decoy is randomly assigned to five hunters,and the order of each experiment is also randomized.An experiment consists of a hunter using one goose decoy and waiting fifteen minutes.How many degrees of freedom does the treatment sum of squares have? How about the error sum of squares?

A)df-treatment = 3; df-error = 14
B)df-treatment = 2; df-error = 13
C)df-treatment = 2; df-error = 14
D)df-treatment = 3; df-error = 12
E)df-treatment = 2; df-error = 12

Question 13

A horticulturist wants to test the effectiveness of four types of fertilizer on the growth rate of Bermuda grass.Six sections of grass are randomly assigned to each of the four fertilizer types,and the rate of growth for each section is measured.How many degrees of freedom does the treatment sum of squares have? How about the error sum of squares?

A)df-treatment = 3; df-error = 21
B)df-treatment = 3; df-error = 20
C)df-treatment = 4; df-error = 20
D)df-treatment = 5; df-error = 21
E)df-treatment = 5; df-error = 20

Question 14

A telemarketing company wants to compare the effectiveness of five of its employees by looking at the percentage of survey questions completed.Data from four past surveys are randomly chosen for each of the employees,and the percentage of completed questions is calculated.How many degrees of freedom does the treatment sum of squares have? How about the error sum of squares?

A)df-treatment = 5; df-error = 19
B)df-treatment = 4; df-error = 19
C)df-treatment = 4; df-error = 15
D)df-treatment = 4; df-error = 16
E)df-treatment = 5; df-error = 15

Question 15

The Professional Golfers' Association (PGA)wants to know which brand of golf ball goes the farthest when hit by a driver.Three brands of golf balls are compared by using "Iron Byron" to randomly hit each brand of ball five times.The total distance in yards is measured after each ball comes to rest.

A)H0: μ1 = μ2 = μ3 vs.the alternative that not all brand mean distances are equal
B)H0: μ1 = μ2 = μ3 = μ4 = μ5 vs.the alternative that not all hit mean distances are equal
C)H0: μ1 ≠ μ2 ≠ μ3 ≠ μ4 ≠ μ5 vs.the alternative that all hit mean distances are equal
D)H0: μ1 ≠ μ2 ≠ μ3 vs.the alternative that all brand mean distances are equal
E)H0: μbrand ≠ μhit vs.the alternative that brand and hit mean distances are equal

Question 16

A horticulturist wants to test the effectiveness of four types of fertilizer on the growth rate of Bermuda grass.Six sections of grass are randomly assigned to each of the four fertilizer types,and the rate of growth for each section is measured.

A)H0: μ1 = μ2 = μ3 = μ4 vs.the alternative that not all fertilizer mean growth rates are equal
B)H0: μ1 ≠ μ2 ≠ μ3 ≠ μ4 ≠ μ5 ≠ μ6 vs.the alternative that all six section means are equal
C)H0: μ1 = μ2 = μ3 = μ4 = μ5 = μ6 vs.the alternative that not all six section means are equal
D)H0: μ1 ≠ μ2 ≠ μ3 ≠ μ4 vs.the alternative that all fertilizer mean growth rates are equal
E)H0: μfert ≠ μsection vs.the alternative that the fertilizer and section means are equal

Question 17

An electrical engineer wants to compare the resistance of four types of wire made from different materials.Five wires of equal lengths and cross sectional areas are prepared for each of the four wire types.Each section of wire is randomly tested by sending a current through the wire and measuring the resistance.

A)H0: μ1 ≠ μ2 ≠ μ3 ≠ μ4 vs.the alternative that all the wire type mean resistances are equal
B)H0: μ1 = μ2 = μ3 = μ4 = μ5 vs.the alternative that not all the wire size mean resistances are equal
C)H0: μtype ≠ μsize vs.the alternative that wire type and wire size mean resistances are equal
D)H0: μ1 ≠ μ2 ≠ μ3 ≠ μ4 ≠ μ5 vs.the alternative that all the wire size mean resistances are equal
E)H0: μ1 = μ2 = μ3 = μ4 vs.the alternative that not all the wire type mean resistances are equal

Question 18

A mechanical engineer is studying bonding issues with the thermal protection tiles on the Space Shuttle.He wants to see whether there is any difference in the bonding strength between three different compounds.Fifteen tiles are randomly assigned to the three compounds so that each compound is tested on five tiles.Each tile is coated with its assigned compound and bonded to a surface.After a period of time,pull-tests are performed to determine the amount of force needed to break the bond.

A)H0: ?1 ? ?2 ? ?3 ? ?4 ? ?5 vs.the alternative that all five tile mean strengths are equal
B)H0: ?comp ? $\mu \text { tile }$ vs.the alternative that the compound and tile mean strengths are equal
C)H0: ?1 = ?2 = ?3 = ?4 = ?5 vs.the alternative that not all tile mean strengths are equal
D)H0: ?1 = ?2 = ?3 vs.the alternative that not all compound mean strengths are equal
E)H0: ?1 ? ?2 ? ?3 vs.the alternative that all compound mean strengths are equal

Question 19

A telemarketing company wants to compare the effectiveness of five of its employees by looking at the percentage of survey questions completed.Data from four past surveys are randomly chosen for each of the employees,and the percentage of completed questions is calculated.

A)H0: μ1 = μ2 = μ3 = μ4 vs.the alternative that not all survey mean percentages are equal
B)H0: μ1 ≠ μ2 ≠ μ3 ≠ μ4 vs.the alternative that all survey mean percentages are equal
C)H0: μ1 ≠ μ2 ≠ μ3 ≠ μ4 ≠ μ5 vs.the alternative that all employee mean percentages are equal
D)H0: μemp ≠ μsurv vs.the alternative that employee and survey mean percentages are equal
E)H0: μ1 = μ2 = μ3 = μ4 = μ5 vs.the alternative that not all employee mean percentages are equal

Question 20

A chemist is interested in comparing four different catalysts by measuring reaction times.Each catalyst is tested five times,with each experiment randomly assigned one of the four catalysts.

A)H0: μcat ≠ μtest vs.the alternative that catalyst and test mean times are equal
B)H0: μ1 ≠ μ2 ≠ μ3 ≠ μ4 ≠ μ5 vs.the alternative that all the test mean times are equal
C)H0: μ1 = μ2 = μ3 = μ4 vs.the alternative that not all the catalyst mean times are equal
D)H0: μ1 ≠ μ2 ≠ μ3 ≠ μ4 vs.the alternative that all the catalyst mean times are equal
E)H0: μ1 = μ2 = μ3 = μ4 = μ5 vs.the alternative that not all the test mean times are equal

Question 21

An industrial engineer wants to test the effect of three different ways of assembling a part on the total assembly time.Five people are randomly assigned to each of the three assembly methods,and the total assembly time (in seconds)is recorded.The box plots for the three methods are shown below.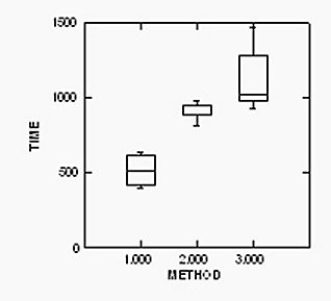

A)Yes,all the groups look like they are normally distributed.
B)Yes,the spreads look similar enough to proceed with the ANOVA.
C)No,the spreads of the three groups look different.The response may need to be re-expressed before proceeding with the ANOVA.
D)No,the centre line for method one is lower than the lines for methods two and three.
E)Yes,the widths of the boxes are all the same.

Question 22

A hunting supply store is interested in knowing whether three different brands of goose decoys are equally effective in attracting geese.Each goose decoy is randomly assigned to five hunters,and the order of each experiment is also randomized.An experiment consists of a hunter using one goose decoy with time as the dependent variable.Calculate MST,MSE and the F-ratio.Can you reject H0 using α = 0.05? The ANOVA table is listed below. Analysis of Variance
Source Sum-of-Squares df Mean-Square F-ratio P-value
CallBrand 8.933
Error 6.800
Total 15.733

A)MST = 4.467; MSE = 0.486; F-Ratio = 9.191; Yes,reject H0 using α = 0.05.
B)MST = 4.467; MSE = 0.523; F-Ratio = 8.541; Yes,reject H0 using α = 0.05.
C)MST = 2.978; MSE = 0.567; F-Ratio = 5.252; No,do not reject H0 using α = 0.05.
D)MST = 4.467; MSE = 0.567; F-Ratio = 7.878; Yes,reject H0 using α = 0.05.
E)MST = 2.978; MSE = 0.486; F-Ratio = 6.128; No,do not reject H0 using α = 0.05.

Question 23

A telemarketing company wants to compare the effectiveness of five of its employees by looking at the percentage of survey questions completed.Data from four past surveys are randomly chosen for each of the employees,and the percentage of completed questions is calculated.Calculate MST,MSE and the F-ratio.Can you reject H0 using α = 0.05? The ANOVA table is listed below. Analysis of Variance
Source Sum-of-Squares df Mean-Square F-ratio P-value
Employee 4359.540
Error 875.978
Total 5235.518

A)MST = 871.908; MSE = 58.399; F-Ratio = 14.930; No,do not reject H0 using α = 0.05.
B)MST = 1089.885; MSE = 54.749; F-Ratio = 19.907; Yes,reject H0 using α = 0.05.
C)MST = 1089.885; MSE = 46.104; F-Ratio = 23.640; Yes,reject H0 using α = 0.05.
D)MST = 1089.885; MSE = 58.399; F-Ratio = 18.663; Yes,reject H0 using α = 0.05.
E)MST = 871.908; MSE = 46.104; F-Ratio = 18.912; No,do not reject H0 using α = 0.05.

Question 24

A hunting supply store is interested in knowing whether three different brands of goose decoys are equally effective in attracting geese.Each goose decoy is randomly assigned to five hunters,and the order of each experiment is also randomized.An experiment consists of a hunter using one goose decoy and waiting fifteen minutes.The number of geese attracted by the decoy is counted.The data are analyzed and calculations show an F-ratio of 7.878.Assuming that the conditions required for ANOVA are satisfied,what is the P-value? What would you conclude?

A)p = 0.0065.Reject H0 using α = 0.05.
B)p = 0.7001.Do not reject H0 using α = 0.05.
C)p = 0.0703.Reject H0 using α = 0.10.
D)p = 0.0065.Reject H0 using α = 0.001.
E)p = 0.0703.Reject H0 using α = 0.05.

Question 25

A telemarketing company wants to compare the effectiveness of five of its employees by looking at the percentage of survey questions completed.Data from four past surveys are randomly chosen for each of the employees,and the percentage of completed questions is calculated.The box plots for the five employees are shown below.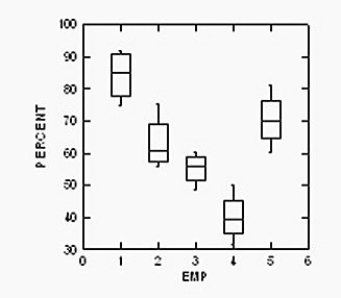

A)Yes,the widths of all the boxes are the same.
B)No,the data need to be log-transformed before conducting the ANOVA.
C)Yes,the spreads of the five box plots appear similar enough to satisfy the assumption.
D)No,the centre line for employee four is lower than all of the others.
E)No,the data probably need to be re-expressed before conducting the ANOVA.

Question 26

An industrial engineer wants to test the effect of three different ways of assembling a part on the total assembly time.Five people are randomly assigned to each of the three assembly methods,and the total assembly time (in seconds)is recorded.Calculate MST,MSE and the F-ratio.Can you reject H0 using α = 0.05? The ANOVA table is listed below. Analysis of Variance
Source Sum-of-Squares df Mean-Square F-ratio P-value
Method 985535.907
Error 276844.275
Total 1262380.182

A)MST = 492767.954; MSE = 19774.591; F-Ratio = 24.919; Yes,reject H0 using α = 0.05.
B)MST = 492767.954; MSE = 23070.356; F-Ratio = 21.359; Yes,reject H0 using α = 0.05.
C)MST = 328511.969; MSE = 23070.356; F-Ratio =14.240; Yes,reject H0 using α = 0.05.
D)MST = 328511.969; MSE = 19774.591; F-Ratio =16.613; Yes,reject H0 using α = 0.05.
E)MST = 492767.954; MSE = 21295.713; F-Ratio = 23.139; Yes,reject H0 using α = 0.05.

Question 27

A horticulturist wants to test the effectiveness of four types of fertilizer on the growth rate of Bermuda grass.Six sections of grass are randomly assigned to each of the four fertilizer types,and the rate of growth for each section is measured.The F-value from an ANOVA is 10.866.What is the P-value?

A)p = 0.0002
B)p = 0.010
C)p = 0.001
D)p = 0.0001
E)p = 0.0011

Question 28

A mechanical engineer is studying bonding issues with the thermal protection tiles on the Space Shuttle.He wants to see whether there is any difference in the bonding strength between three different compounds.Fifteen tiles are randomly assigned to the three compounds so that each compound is tested on five tiles.Each tile is coated with its assigned compound and bonded to a surface.After a period of time,pull-tests are performed to determine the amount of force needed to break the bond.Calculate MST,MSE,and the F-ratio.Can you reject H0 using α = 0.05? The ANOVA table is listed below. Analysis of Variance
Source Sum-of-Squares df Mean-Square F-ratio P-value
Compound 491.207
Error 2888.847
Total 4380.054

A)MST = 497.069; MSE = 206.346; F-Ratio =2.409; Yes,reject H0 using α = 0.05.
B)MST = 745.604; MSE = 206.346; F-Ratio = 3.613; Yes,reject H0 using α = 0.05.
C)MST = 497.069; MSE = 240.737; F-Ratio =2.065; No,do not reject H0 using α = 0.05.
D)MST = 745.604; MSE = 222.219; F-Ratio = 3.355; No,do not reject H0 using α = 0.05.
E)MST = 745.604; MSE = 240.737; F-Ratio = 3.097; No,do not reject H0 using α = 0.05.

Question 29

A consumer interest group is interested in comparing the speed of four different desktop printers; four different documents are used for the experiment.Each test consists of randomly assigning one printer to one of the documents.The document is printed and the time to print in seconds is recorded.Each printer/document combination is tested.The F-value from an ANOVA is 6.173.What is the P-value?

A)p = 0.0998
B)p = 0.0088
C)p = 0.0901
D)p = 0.9093
E)p = 0.0012

Question 30

An electrical engineer wants to compare the resistance of four types of wire made from different materials.Five wires of equal lengths and cross sectional areas are prepared for each of the four wire types.Each section of wire is randomly tested by sending a current through the wire and measuring the resistance.Calculate MST,MSE and the F-ratio.Can you reject H0 using α = 0.05? The ANOVA table is listed below. Analysis of Variance
Source Sum-of-Squares df Mean-Square F-ratio P-value
WireType 293.745
Error 1456.131
Total 1749.876

A)MST = 97.915; MSE = 91.008; F-Ratio = 1.076; No,do not reject H0 using α = 0.05.
B)MST = 97.915; MSE = 85.655; F-Ratio = 1.143; No,do not reject H0 using α = 0.05.
C)MST = 97.915; MSE = 76.638; F-Ratio = 1.278; Yes,reject H0 using α = 0.05.
D)MST =73.436; MSE = 76.638; F-Ratio = 0.958; No,do not reject H0 using α = 0.05.
E)MST =73.436; MSE = 72.807; F-Ratio = 1.009; No,do not reject H0 using α = 0.05.

Question 31

An electrical engineer wants to compare the resistance of four types of wire made from different materials.Five wires of equal lengths and cross sectional areas are prepared for each of the four wire types.Each section of wire is randomly tested by sending a current through the wire and measuring the resistance.After collecting the data and analyzing the results,she reports that the F-ratio is 1.076.Assuming that the conditions required for ANOVA are satisfied,what is the P-value? What would you conclude?

A)p = 0.0871.Do not reject H0 using α = 0.05.
B)p = 0.3872.Do not reject H0 using α = 0.05.
C)p = 0.0871.Reject H0 using α = 0.10.
D)p = 0.3872.Reject H0 using α = 0.05.
E)p = 0.3872.Reject H0 using α = 0.25.

Question 32

The Professional Golfers' Association (PGA)wants to know which brand of golf ball goes the farthest when hit by a driver.Three brands of golf balls are compared by using "Iron Byron" to randomly hit each brand of ball five times.The total distance in yards is measured after each ball comes to rest.Calculate MST,MSE and the F-ratio.Can you reject H0 using α = 0.05? The ANOVA table is listed below. Analysis of Variance
Source Sum-of-Squares df Mean-Square F-ratio P-value
Brand 1405.375
Error 1467.556
Total 2872.931

A)MST = 468.458; MSE = 122.296; F-Ratio =3.831; No,do not reject H0 using α = 0.05.
B)MST = 468.458; MSE = 104.825; F-Ratio =4.469; No,do not reject H0 using α = 0.05.
C)MST = 702.688; MSE = 97.837; F-Ratio = 7.182; Yes,reject H0 using α = 0.05.
D)MST = 702.688; MSE = 104.825; F-Ratio = 6.703; Yes,reject H0 using α = 0.05.
E)MST = 702.688; MSE = 122.296; F-Ratio = 5.746; Yes,reject H0 using α = 0.05.

Question 33

A chemist is interested in comparing four different catalysts by measuring reaction times.Each catalyst is tested five times,with each experiment randomly assigned one of the four catalysts.Calculate MST,MSE and the F-ratio.Can you reject H0 using α = 0.05? The ANOVA table is listed below. Analysis of Variance
Source Sum-of-Squares df Mean-Square F-ratio P-value
Catalyst 9.310
Error 79.320
Total 88.630

A)MST = 2.328; MSE = 4.958; F-Ratio = 0.470; No,do not reject H0 using α = 0.05.
B)MST = 3.103; MSE = 4.666; F-Ratio = 0.665; No,do not reject H0 using α = 0.05.
C)MST = 3.103; MSE = 4.175; F-Ratio = 0.743; No,do not reject H0 using α = 0.05.
D)MST = 2.328; MSE = 4.175; F-Ratio = 0.558; Yes,reject H0 using α = 0.05.
E)MST = 3.103; MSE = 4.958; F-Ratio = 0.626; No,do not reject H0 using α = 0.05.

Question 34

An electrical engineer wants to compare the resistance of four types of wire made from different materials.Five wires of equal lengths and cross sectional areas are prepared for each of the four wire types.Each section of wire is randomly tested by sending a current through the wire and measuring the resistance.The box plots for the four wire types are shown below.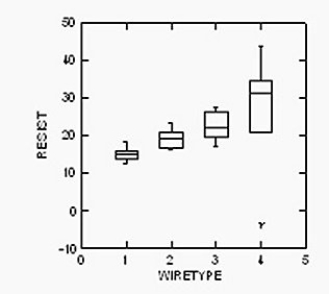

A)Yes,the widths of the boxes are all the same.
B)Yes,all the groups look like they are normally distributed.
C)No,the spreads of the four groups look different.The response may need to be re-expressed before proceeding with the ANOVA.
D)No,the centre lines are steadily increasing.
E)Yes,the spreads look similar enough to proceed with the ANOVA.

Question 35

A consumer interest group is interested in comparing the speed of four different desktop printers; four different documents are used for the experiment.Each test consists of randomly assigning one printer to one of the documents.The document is printed and the time to print in seconds is recorded.Each printer/document combination is tested.Calculate MST,MSE and the F-ratio.Can you reject H0 using α = 0.05? The ANOVA table is listed below. Analysis of Variance
Source Sum-of-Squares df Mean-Square F-ratio P-value
Printer 4.862
Error 3.150
Total 8.012

A)MST = 1.216; MSE = 0.210; F-Ratio = 5.790; No,do not reject H0 using α = 0.05.
B)MST = 1.621; MSE = 0.263; F-Ratio = 6.163; Yes,reject H0 using α = 0.05.
C)MST = 1.621; MSE = 0.210; F-Ratio = 7.719; Yes,reject H0 using α = 0.05.
D)MST = 1.216; MSE = 0.263; F-Ratio = 4.641; No,do not reject H0 using α = 0.05.
E)MST = 1.621; MSE = 0.242; F-Ratio = 6.698; Yes,reject H0 using α = 0.05.

Question 36

A mechanical engineer is studying bonding issues with the thermal protection tiles on the Space Shuttle.He wants to see whether there is any difference in the bonding strength between three different compounds.Fifteen tiles are randomly assigned to the three compounds so that each compound is tested on five tiles.Each tile is coated with its assigned compound and bonded to a surface.After a period of time,pull-tests are performed to determine the amount of force needed to break the bond.After collecting the data and analyzing the results,he reports that the F-ratio is 3.097.Assuming that the conditions required for ANOVA are satisfied,what is the P-value? What would you conclude?

A)p = 0.0827.Reject H0 using α = 0.05.
B)p = 0.0827.Do not reject H0 using α = 0.05.
C)p = 0.0827.Reject H0 using α = 0.01.
D)p = 0.208.Do not reject H0 using α = 0.05.
E)p = 0.0827.Do not reject H0 using α = 0.10.

Question 37

A horticulturist wants to test the effectiveness of four types of fertilizer on the growth rate of Bermuda grass.Six sections of grass are randomly assigned to each of the four fertilizer types,and the rate of growth for each section is measured.The box plots for the four types of fertilizer are shown below.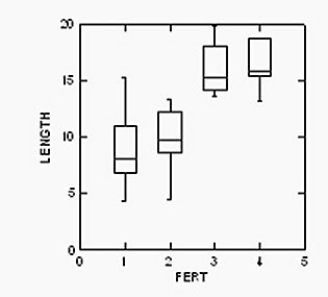

A)Yes,the widths of all the boxes are the same.
B)No,the data need to be log-transformed before conducting the ANOVA.
C)No,the centre lines for fertilizers three and four are higher than one and two.
D)Yes,the spreads of the four box plots appear similar enough to satisfy the assumption.
E)No,the data probably need to be re-expressed before conducting the ANOVA.

Question 38

An independent research firm conducts a study to compare the taste of four new sports drinks.Five people are randomly assigned to each of the four drinks.Each person tastes the drink and judges it on a scale from one to five.Calculate MST,MSE and the F-ratio.Can you reject H0 using α = 0.05? The ANOVA table is listed below. Analysis of Variance
Source Sum-of-Squares df Mean-Square F-ratio P-value
Drink 25.200
Error 10.000
Total 35.200

A)MST = 6.300; MSE = 0.588; F-Ratio = 10.714; No,do not reject H0 using α = 0.05.
B)MST = 8.400; MSE = 0.588; F-Ratio = 14.286; Yes,reject H0 using α = 0.05.
C)MST = 8.400; MSE = 0.625; F-Ratio = 13.44; Yes,reject H0 using α = 0.05.
D)MST = 8.400; MSE = 0.526; F-Ratio = 15.970; Yes,reject H0 using α = 0.05.
E)MST = 6.300; MSE = 0.625; F-Ratio = 10.080; No,do not reject H0 using α = 0.05.

Question 39

A horticulturist wants to test the effectiveness of four types of fertilizer on the growth rate of Bermuda grass.Six sections of grass are randomly assigned to each of the four fertilizer types,and the rate of growth for each section is measured.Calculate MST,MSE and the F-ratio.Can you reject H0 using α = 0.05? The ANOVA table is listed below. Analysis of Variance
Source Sum-of-Squares df Mean-Square F-ratio P-value
Fert 283.966
Error 174.218
Total 458.184

A)MST = 56.793; MSE = 8.711; F-Ratio = 6.520; No,do not reject H0 using α = 0.05.
B)MST = 70.992; MSE = 8.711; F-Ratio = 8.150; Yes,reject H0 using α = 0.05.
C)MST = 56.793; MSE = 8.296; F-Ratio = 6.846; No,do not reject H0 using α = 0.05.
D)MST = 94.655; MSE = 8.711; F-Ratio = 10.866; Yes,reject H0 using α = 0.05.
E)MST = 94.655; MSE = 8.296; F-Ratio = 11.410; Yes,reject H0 using α = 0.05.