Introduction to Management Science 12th Edition by Bernard Taylor

Exercise 14

In Problem, KanTech Corporation is just as concerned that its U.S. distributors receive shipments in the minimum amount of time as they are about minimizing their shipping costs. Suppose that each U.S. distributor receives one major container shipment each month. Following are summaries of the shipping times (in days) between each of the embarkation and destination points along KanTech's overseas supply chain. These times encompass not only travel time but also processing, loading, and unloading times at each port:


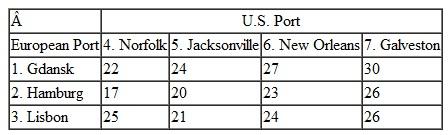
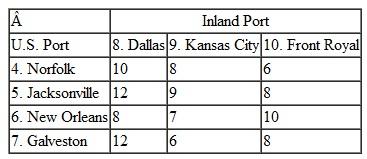
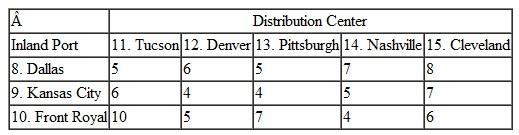a. Formulate and solve a linear programming model to determine the optimal shipping route for each distribution center along this supply chain that will result in the minimum total shipping time. Determine the shipping route and time for each U.S. distributor.
b. Suppose the European ports can accommodate only three shipments each. How will this affect the solution in part (a)
Problem
KanTech Corporation is a global distributor of electrical parts and components. Its customers are electronics companies in the United States, including computer manufacturers and audio/ visual product manufacturers. The company contracts to purchase components and parts from manufacturers in Russia, Eastern and Western Europe, and the Mediterranean, and it has them delivered to warehouses in three European ports, Gdansk, Hamburg, and Lisbon. The various components and parts are loaded into containers based on demand from U.S. customers. Each port has a limited fixed number of containers available each month. The containers are then shipped overseas by container ships to the ports of Norfolk, Jacksonville, New Orleans, and Galveston. From these seaports, the containers are typically coupled with trucks and hauled to inland ports in Front Royal (Virginia), Kansas City, and Dallas. There are a fixed number of freight haulers available at each port each month. These inland ports are sometimes called "freight villages," or intermodal junctions, where the containers are collected and transferred from one transport mode to another (i.e., from truck to rail or vice versa). From the inland ports, the containers are transported to KanTech's distribution centers in Tucson, Pittsburgh, Denver, Nashville, and Cleveland. Following are the handling and shipping costs ($/container) between each of the embarkation and destination points along this overseas supply chain and the available containers at each port:


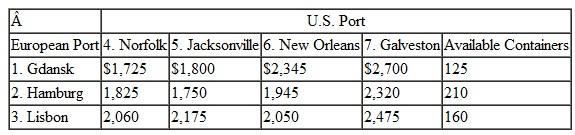
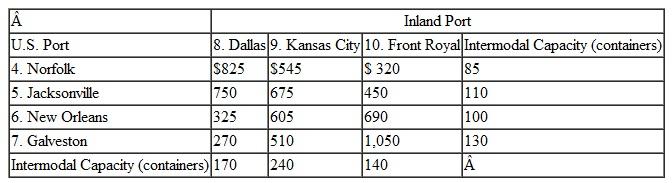
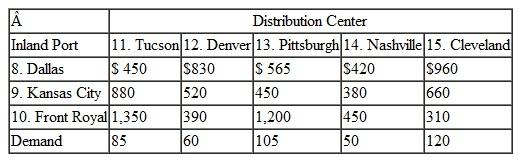Formulate and solve a linear programming model to determine the optimal shipments from each point of embarkation to each destination along this supply chain that will result in the minimum total shipping cost.