Deck 10: Introduction to the Derivative
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/139
Play
Full screen (f)
Deck 10: Introduction to the Derivative
1
Compute  algebraically for
algebraically for  .
.

 algebraically for
algebraically for  .
.

10
2
Compute .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)
3
Compute for .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)
4
Compute algebraically for .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
5
Compute .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
6
Compute the derivative function algebraically.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
7
Compute for .
A)8
B)
C) 0
D)
E) 10
A)8
B)
C) 0
D)
E) 10

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
8
Compute the derivative function algebraically.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
9
Compute for .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
10
Compute algebraically for .
A)129
B) 66
C) 126
D) 45
E) 43
A)129
B) 66
C) 126
D) 45
E) 43

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
11
If a stone is dropped from a height of Variable isn't defined feet, its height after t seconds is given by . Find the stone's velocity at time .
A) ft/s
B) ft/s
C) ft/s
D) ft/s
E) ft/s
A) ft/s
B) ft/s
C) ft/s
D) ft/s
E) ft/s

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
12
Compute the derivative function algebraically.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
13
Compute 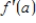 algebraically for
algebraically for 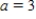 .
.
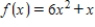
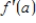 algebraically for
algebraically for 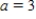 .
.

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
14
Compute .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
15
Compute .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
16
Compute the derivative function algebraically.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
17
Compute for .
A)-6
B) 6
C) -9
D) 3
E) 8
A)-6
B) 6
C) -9
D) 3
E) 8

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
18
Compute .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
19
Compute the derivative function algebraically.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
20
Compute the derivative function algebraically.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
21
The function represents the value of the U.S. dollar in Indian rupees as a function of time t in days.Find the average rates of change of over the time intervals , where t is as indicated and , , , and days. Hence, estimate (using ) the instantaneous rate of change of R at time . Please round the instantaneous rate to the nearest whole number.
A)29
B) 25
C) 27
D) 28
E) 30
A)29
B) 25
C) 27
D) 28
E) 30

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
22
Compute the derivative function 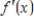 algebraically.
algebraically.
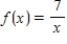
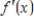 algebraically.
algebraically.
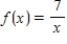

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
23
The function represents the value of the U.S. dollar in Indian rupees as a function of the time t in days. Find the average rates of change of over the time intervals , where t is as indicated and , , , and days. Hence, estimate (using ) the instantaneous rate of change of at time .
Select your answer rounded to the nearest whole number.
A)63
B) 69
C) 64
D) 67
E) 68
Select your answer rounded to the nearest whole number.
A)63
B) 69
C) 64
D) 67
E) 68

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
24
At which labeled point is the slope of the tangent line greatest ? 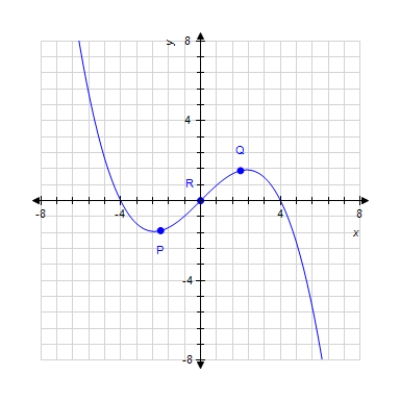 ?
?
A)Q
B) R
C) P
 ?
?A)Q
B) R
C) P

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
25
For the slope 2.2, determine at which of the labeled points on the graph the tangent line has that slope. 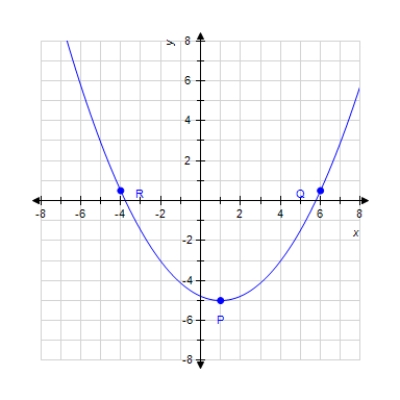
A)R
B) Q
C) P

A)R
B) Q
C) P

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
26
At which labeled point is the slope of the tangent line greatest ? 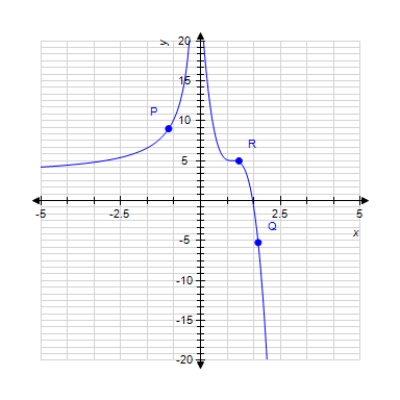 ?
?
A)R
B) P
C) Q
 ?
?A)R
B) P
C) Q

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
27
Estimate the derivative of the function at the point .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
28
Compute 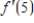 .
.
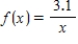
Enter your answer rounded to the nearest thousandth.
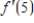 .
.
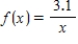
Enter your answer rounded to the nearest thousandth.

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
29
Compute the derivative function 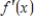 algebraically.
algebraically.
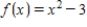
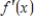 algebraically.
algebraically.
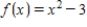

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
30
Compute the derivative function 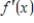 algebraically.
algebraically.
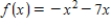
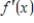 algebraically.
algebraically.
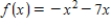

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
31
The function represents the value of the U.S. dollar in Indian rupees as a function of time t in days. Find the average rates of change of over the time intervals , where t is as indicated and , , , and days. Estimate (using ) the instantaneous rate of change of R at time . Please select the correct answer rounded to the nearest whole number.
A)443
B) 441
C) 437
D) 446
E) 444
A)443
B) 441
C) 437
D) 446
E) 444

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
32
At which labeled point is the slope of the tangent line greatest 
A)P
B) Q
C) R

A)P
B) Q
C) R

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
33
Compute 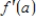 for
for 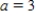 .
.
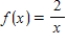
Enter your answer in fraction form.
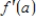 for
for 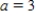 .
.
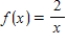
Enter your answer in fraction form.

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
34
The function given below gives the cost to manufacture items. Estimate (using ) the instantaneous rate of change of the cost at the production level .
Select your answer rounded to the nearest tenth.
A)3.8
B) 5.0
C) 4.0
D) 2.8
E) 4.8
Select your answer rounded to the nearest tenth.
A)3.8
B) 5.0
C) 4.0
D) 2.8
E) 4.8

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
35
Compute the derivative function  algebraically.
algebraically.
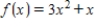
 algebraically.
algebraically.
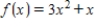

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
36
Compute  .
.
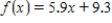
 .
.
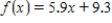

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
37
At which labeled point the slope of the tangent line is greatest 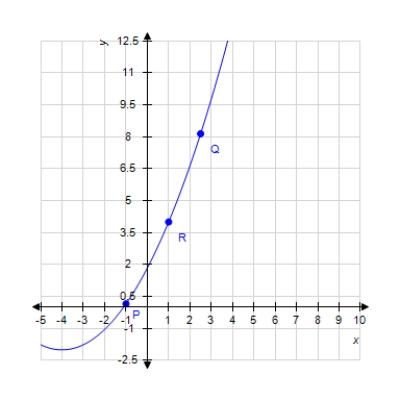
A)R
B) P
C) Q

A)R
B) P
C) Q

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
38
Estimate the derivative of the function at the point .
Select the answer rounded to the nearest whole number.
A)-9
B) -10
C) -8
D) -7
E) -13
Select the answer rounded to the nearest whole number.
A)-9
B) -10
C) -8
D) -7
E) -13

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
39
At which labeled point is the slope of the tangent line least (in the sense that -7 is less than 1) 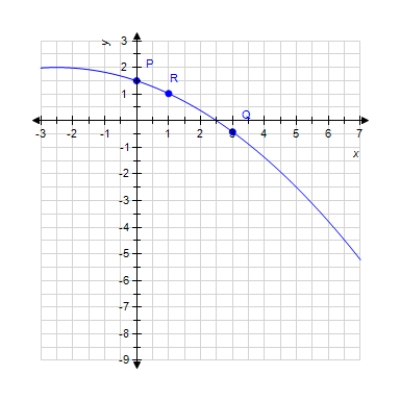
A)P
B) R
C) Q

A)P
B) R
C) Q

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
40
The function given below gives the cost to manufacture x items. Estimate (using ) the instantaneous rate of change of the total cost at the production level .
Select your answer rounded to the nearest tenth.
A)100.1
B) 99.9
C) 100.9
D) 98.9
E) 101.1
Select your answer rounded to the nearest tenth.
A)100.1
B) 99.9
C) 100.9
D) 98.9
E) 101.1

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
41
Find an equation of the tangent line to the graph of the function at the point that has x-coordinate . [Hint: use point-slope formula to find the equation of the tangent line.]
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
42
The function given below gives the cost to manufacture x items. Estimate (using  ) the instantaneous rate of change of the total cost at the production level
) the instantaneous rate of change of the total cost at the production level 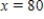 .
.
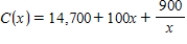
Enter your answer as a number without the units rounded to the nearest tenth.
 ) the instantaneous rate of change of the total cost at the production level
) the instantaneous rate of change of the total cost at the production level 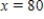 .
.
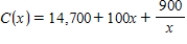
Enter your answer as a number without the units rounded to the nearest tenth.

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
43
Estimate of the function .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
44
Estimate the slope of the tangent line to the graph of the following function at the point .
A)-505
B) 505
C) 500
D) 2,500
E) -500
A)-505
B) 505
C) 500
D) 2,500
E) -500

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
45
Estimate of the function .
A)20
B) 17
C) 14
D) 16
E) 18
A)20
B) 17
C) 14
D) 16
E) 18

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
46
Estimate of the function .
A)0
B) 1
C) 2
D) 6
E) 3
A)0
B) 1
C) 2
D) 6
E) 3

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
47
Estimate 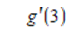
of the function
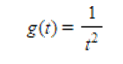
Please enter your answer as a fraction.
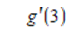
of the function
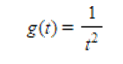
Please enter your answer as a fraction.

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
48
The function given below gives the cost to manufacture x items. Estimate (using  ) the instantaneous rate of change of the cost at the production level
) the instantaneous rate of change of the cost at the production level 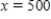 .
.
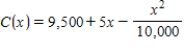
Enter your answer as a number without the units rounded to the nearest tenth.
 ) the instantaneous rate of change of the cost at the production level
) the instantaneous rate of change of the cost at the production level 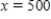 .
.
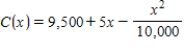
Enter your answer as a number without the units rounded to the nearest tenth.

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
49
Estimate the derivative of the function 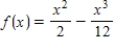 at the point
at the point 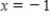 .
.
Please round your answer to the nearest hundredth.
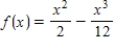 at the point
at the point 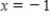 .
.
Please round your answer to the nearest hundredth.

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
50
Estimate of the function .
A)4
B) 3
C) 6
D) 9
E) 1
A)4
B) 3
C) 6
D) 9
E) 1

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
51
Estimate of the function .
A)
B)
C) 0
D) 5
E) 1
A)
B)
C) 0
D) 5
E) 1

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
52
Estimate the slope of the tangent line to the graph of the following function at the point 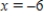 .
.

Please round the answer to the nearest whole number.
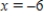 .
.

Please round the answer to the nearest whole number.

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
53
Estimate the derivative of the function 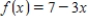 at the point
at the point 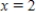 .
.
Please round the answer to the nearest whole number.
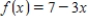 at the point
at the point 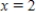 .
.
Please round the answer to the nearest whole number.

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
54
Estimate the derivative of the function at the point .
Please select the correct answer rounded to the nearest hundredth.
A)-2.5
B) -2.55
C) -3
D) -0.5
E) -2.45
Please select the correct answer rounded to the nearest hundredth.
A)-2.5
B) -2.55
C) -3
D) -0.5
E) -2.45

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
55
Find an equation of the tangent line to the graph of the function at the point that has x-coordinate .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
56
Use any method to find the slope of the tangent line to the graph of the function at the point that has x-coordinate .
A)-125
B) 25
C)
D)
E)
A)-125
B) 25
C)
D)
E)

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
57
Estimate of the function .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
58
If a stone is dropped from a height of 472 feet, its height after t seconds is given by . Find the stone's average velocity over the period .
A)-73
B) -69
C) -72
D) -70
E) -74
A)-73
B) -69
C) -72
D) -70
E) -74

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
59
Estimate of the function .
?
Please round the answer to the nearest whole number.
?
Please round the answer to the nearest whole number.

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
60
On January 1, 1996, America Online was the largest on-line service provider, with 5 million subscribers, and it was adding new subscribers at a rate of 42,000 per week. If is the number of America Online subscribers t weeks after January 1, 1996, what do the given data tell you about values of the function and its derivative
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
61
Calculate the average rate of change of the given function over the interval .
A)-400
B) 1,200
C) 800
D) -800
E) -200
A)-400
B) 1,200
C) 800
D) -800
E) -200

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
62
Calculate the average rate of change of the given function over the interval .
A)-$300,000
B) -$0
C) $150
D) -$150,000
E) $150,000
A)-$300,000
B) -$0
C) $150
D) -$150,000
E) $150,000

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
63
Calculate the average rate of change of the given function over the interval 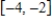 .
. 
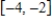 .
. 

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
64
Calculate the average rate of change of the given function over the interval .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
65
Calculate the limit algebraically.
A)4
B) 2
C) -2
D) 1
E) -4
A)4
B) 2
C) -2
D) 1
E) -4

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
66
Calculate the average rate of change of the given function f over the interval , where , and . (It will be easier to do this if you first simplify the difference quotient (dq) as much as possible.) Complete the table.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
67
Calculate the average rate of change of the given function over the interval .
A)64.90
B) 216.33
C) 717.00
D) 35.85
E) 119.50
A)64.90
B) 216.33
C) 717.00
D) 35.85
E) 119.50

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
68
Calculate the limit algebraically.
A)7.5
B) -2
C) 15
D) 13
E) 6.5
A)7.5
B) -2
C) 15
D) 13
E) 6.5

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
69
Calculate the average rate of change of the given function over the interval .
A)-24
B) 3
C) -3
D) 168
E) -21
A)-24
B) 3
C) -3
D) 168
E) -21

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
70
Calculate the average rate of change of the given function over the interval 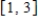 .
. 
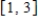 .
. 

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
71
Calculate the average rate of change of the given function (Inflation (%) of Budget deficit (% of GNP)) over the interval  .
.

Please enter your answer as a number without the units.
 .
.

Please enter your answer as a number without the units.

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
72
Calculate the average rate of change of the given function over the interval .
A)-42
B) 42
C) 4
D) 10.5
E) 16
A)-42
B) 42
C) 4
D) 10.5
E) 16

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
73
Calculate the average rate of change of the given function over the interval .
A)-22
B) 6
C) -6
D) -12
E) 12
A)-22
B) 6
C) -6
D) -12
E) 12

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
74
Calculate the average rate of change of the given function (Inflation(%)) over the interval 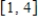 .
.
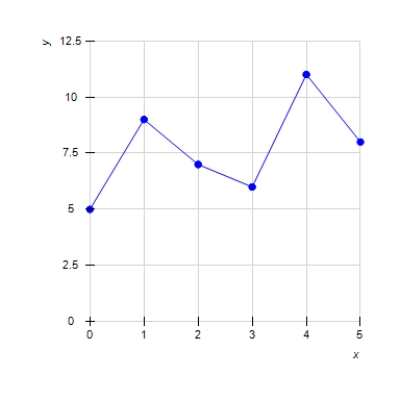
Please enter your answer as a fraction without the units.
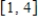 .
.

Please enter your answer as a fraction without the units.

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
75
The chart shows the total annual support for the arts in the U.S. by federal, state, and local government in 1995-2003 as a function of time in years ( represents 1995) together with the regression line. ![<strong>The chart shows the total annual support for the arts in the U.S. by federal, state, and local government in 1995-2003 as a function of time in years ( t = 0 represents 1995) together with the regression line. Over the period [ 0,4 ] the average rate of change of government funding for the arts was _____ the rate predicted by the regression line. </strong> A)greater than B) less than C) equal to](https://storage.examlex.com/TB6226/11eb0df5_e6c8_0a47_9431_998e43179bd3_TB6226_00.jpg)
Over the period the average rate of change of government funding for the arts was _____ the rate predicted by the regression line.
A)greater than
B) less than
C) equal to
![<strong>The chart shows the total annual support for the arts in the U.S. by federal, state, and local government in 1995-2003 as a function of time in years ( t = 0 represents 1995) together with the regression line. Over the period [ 0,4 ] the average rate of change of government funding for the arts was _____ the rate predicted by the regression line. </strong> A)greater than B) less than C) equal to](https://storage.examlex.com/TB6226/11eb0df5_e6c8_0a47_9431_998e43179bd3_TB6226_00.jpg)
Over the period the average rate of change of government funding for the arts was _____ the rate predicted by the regression line.
A)greater than
B) less than
C) equal to

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
76
Calculate the average rate of change of the given function over the interval .
A)6
B) -12
C) 3.85
D) -3.35
E) 4.9
A)6
B) -12
C) 3.85
D) -3.35
E) 4.9

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
77
Calculate the average rate of change of the given function over the interval .
A)6
B) 14
C) 8
D) 10
E) 18
A)6
B) 14
C) 8
D) 10
E) 18

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
78
Calculate the limit algebraically.
A)8
B) 3
C) -23.5
D) -47
E) 16
A)8
B) 3
C) -23.5
D) -47
E) 16

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
79
Calculate the average rate of change of the given function over the interval .
A)3
B) 0.5
C) -24
D) 12
E) -12
A)3
B) 0.5
C) -24
D) 12
E) -12

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck
80
Calculate the average rate of change of the given function (Inflation (%) of Budget deficit (% of GNP)) over the interval . ![<strong>Calculate the average rate of change of the given function (Inflation (%) of Budget deficit (% of GNP)) over the interval [ 0,2 ] . </strong> A)14 B) -1 C) -32 D) 2 E) 8](https://storage.examlex.com/TB6226/11eb0df5_e6c5_e74e_9431_f369ea53443d_TB6226_00.jpg)
A)14
B) -1
C) -32
D) 2
E) 8
![<strong>Calculate the average rate of change of the given function (Inflation (%) of Budget deficit (% of GNP)) over the interval [ 0,2 ] . </strong> A)14 B) -1 C) -32 D) 2 E) 8](https://storage.examlex.com/TB6226/11eb0df5_e6c5_e74e_9431_f369ea53443d_TB6226_00.jpg)
A)14
B) -1
C) -32
D) 2
E) 8

Unlock Deck
Unlock for access to all 139 flashcards in this deck.
Unlock Deck
k this deck


