Deck 6: Probability
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/112
Play
Full screen (f)
Deck 6: Probability
1
Which of the following is a requirement of the probabilities assigned to the outcomes 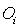 ?
? 
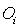 ?
? 
C
2
Two events A and B are said to be independent if: 

D
3
If the events A and B are independent, with P(A) = 0.30 and P(B) = 0.40, then the probability that both events will occur simultaneously is: 

B
4
If you roll a fair (unbiased) die 60 times, you should expect an odd number to appear: 


Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
5
The collection of all possible outcomes of an experiment is called: 


Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
6
Which of the following is not an approach to assigning probabilities? 


Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
7
An experiment consists of tossing three unbiased coins simultaneously. Drawing a probability tree for this experiment will show that the number of simple events in this experiment is: 


Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
8
Suppose P(A) = 0.25. The probability of complement of A is: 


Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
9
An experiment consists of three stages. There are two possible outcomes in the first stage, three possible outcomes in the second stage, and four possible outcomes in the third stage. Drawing a tree diagram for this experiment will show that the total number of outcomes is: 


Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
10
If P(A) = 0.65, P(B) =0.76 and P(A B) =0.80, then P(A B) is:

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
11
Which of the following statements is always correct? 


Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
12
A useful graphical method of constructing the sample space for an experiment is: 


Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
13
Two events A and B are said to mutually exclusive if: 


Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
14
If P(A) = 0.35, P(B) = 0.45 and P(A B) =0.20, then P(A | B) is:

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
15
If P(A) = 0.20, P(B) = 0.30 and P(A B) = 0.00, then A and B are:

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
16
An approach of assigning probabilities that assumes that all outcomes of the experiment are equally likely is referred to as the: 


Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
17
If P(A) = 0.60, P(B) = 0.58, and P(A B) = 0.70, then P(A B) is:

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
18
If A and B are mutually exclusive events with P(A) = 0.80, then P(B): 


Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
19
If A and B are independent events with P(A) = 0.60 and P(A/B) = 0.60, then P(B) is: 


Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
20
When a fair die is rolled once, the sample space consists of the following six outcomes: 1, 2, 3, 4, 5, 6. Given this sample space, which of the following is a simple event? 


Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
21
The classical approach to assigning probability can be applied for experiments that have equally likely outcomes.

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
22
If event A does not occur, then its complement must occur.

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
23
If a coin is tossed three times, and a statistician predicts that the probability of obtaining three heads in a row is 0.125, which of the following assumptions is irrelevant to his prediction? 


Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
24
Marginal probability is the probability that a given event will occur, with no other events taken into consideration.

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
25
The relative frequency approach to probability depends on the law of large numbers.

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
26
If A and B are mutually exclusive events, with P(A) = 0.20 and P(B) = 0.30, then P(A B) is:

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
27
Based on past exam results in principles of accounting you estimate that there is an 83% chance of passing the exam. This is an example of the subjective approach to probability.

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
28
If we wished to determine the probability that one or more of several events will occur in an experiment, we would use addition rules.

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
29
Two or more events are said to be independent when the occurrence of one event has an effect on the probability that another will occur.

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
30
Five students from a statistics class have formed a study group. Each may or may not attend a study session. Assuming that the members will be making independent decisions on whether or not to attend, there are 32 different possibilities for the composition of the study session.

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
31
If P(A) = 0.25 and P(B) = 0.65, then P(A B) is:

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
32
Probability refers to a number between 0 and 1 (inclusive), which expresses the chance that an event will occur.

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
33
If A and B are mutually exclusive events, with P(A) = 0.30 and P(B) = 0.40, then P(A B) is:

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
34
Of the last 400 customers entering a supermarket, 20 have purchased a mobile phone. If the classical approach for assigning probabilities is used, the probability that the next customer will purchase a mobile phone is: 


Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
35
The annual estimate of the number of deaths of infants is an example of the classical approach to probability.

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
36
Conditional probability is the probability that an event will occur, given that another event will also occur.

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
37
If A and B are independent events, with P(A) = 0.50 and P(B) = 0.70, then the probability that A occurs or B occurs or both occur is: 


Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
38
If A and B are independent events, with P(A) = 0.20 and P(B) =0.60, then P(A | B) is: 


Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
39
The probability of event A and event B occurring must be equal to 1.

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
40
If an experiment consists of five outcomes, with 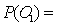 0.10,
0.10, 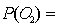 0.10,
0.10, 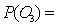 0.30,
0.30, 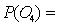 0.25, then
0.25, then  is:
is: 
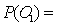 0.10,
0.10, 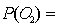 0.10,
0.10, 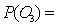 0.30,
0.30, 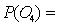 0.25, then
0.25, then  is:
is: 

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
41
If events A and B have nonzero probabilities, then they can be both independent and mutually exclusive.

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
42
Given that events A and B are independent, and that P(A) = 0.9 and P(B | A) = 0.5, then P(A B) = 0.45.

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
43
Two events A and B are said to mutually exclusive if P(A) = P(B).

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
44
A PhD graduate has applied for a job with two universities, A and
B. The graduate feels that she has a 60% chance of receiving an offer from university A, and a 30% chance of receiving an offer from university B. If she receives an offer from university B, she believes that she has an 70% chance of receiving an offer from universityA.
a. What is the probability that both universities will make her an offer?
b. What is the probability that at least one university will make her an offer?
c. If she receives an offer from university B, what is the probability that she will not receive an offer from university A?
B. The graduate feels that she has a 60% chance of receiving an offer from university A, and a 30% chance of receiving an offer from university B. If she receives an offer from university B, she believes that she has an 70% chance of receiving an offer from universityA.
a. What is the probability that both universities will make her an offer?
b. What is the probability that at least one university will make her an offer?
c. If she receives an offer from university B, what is the probability that she will not receive an offer from university A?

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
45
When events are mutually exclusive, they can happen at the same time.

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
46
Bayes' theorem allows us to compute conditional probabilities from other forms of probability.

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
47
Assume that A and B are independent events, with P(A) = 0.30 and P(B) = 0.50. The probability that both events will occur simultaneously is 0.80.

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
48
When it is not reasonable to use the classical approach to assigning probabilities to the outcomes of an experiment, and there is no history of the outcomes, we have no alternative but to employ the subjective approach.

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
49
An effective and simple method of applying the probability rules is the probability tree, wherein the events of an experiment are represented by lines.

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
50
Suppose A and B are two independent events, with P(A) = 0.20 and P(B) = 0.60.
a. Find P(B | A).
b. Find P(A | B).
c. Find P(A and B).
d. Find P(A or B).
a. Find P(B | A).
b. Find P(A | B).
c. Find P(A and B).
d. Find P(A or B).

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
51
According to an old song lyric, 'love and marriage go together like a horse and carriage'. Let love be event A and marriage be event
B. Events A and B cannot be mutually exclusive.
B. Events A and B cannot be mutually exclusive.

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
52
Jim and John go to a coffee shop during their lunch break and toss a coin to see who will pay. The probability that John will pay three days in a row is 0.125.

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
53
The relative frequency approach is not useful in interpreting probability statements such as those heard from weather forecasters or scientists.

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
54
An experiment consists of tossing three fair (unbiased) coins simultaneously. This experiment has eight possible outcomes.

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
55
Three candidates for the presidency of a university's student union, Alice, Brenda and Cameron, are to address a student forum. The forum's organiser is to select the order in which the candidates will give their speeches, and must do so in such a way that each possible order is equally likely to be selected.
a. What is the random experiment?
b. List the simple events in the sample space.
c. Assign probabilities to the simple events.
d. What is the probability that Cameron will speak first?
e. What is the probability that one of the women will speak first?
f. What is the probability that Alice will speak before Cameron does?
a. What is the random experiment?
b. List the simple events in the sample space.
c. Assign probabilities to the simple events.
d. What is the probability that Cameron will speak first?
e. What is the probability that one of the women will speak first?
f. What is the probability that Alice will speak before Cameron does?

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
56
If A and B are independent events, with P(A) = 0.30 and P(B) = 0.50, then P(B | A) is 0.10.

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
57
Baye' Law is a method of revising probabilities after another event has occurred.

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
58
Two events A and B are said to be independent if P(A B) = P(A) + P(B).

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
59
There are three approaches to determining the probability that an outcome will occur: the classical, relative frequency, and subjective approaches. Which is most appropriate in determining the probability of the following outcomes?
a. A flipped coin will land on tails.
b. The probability of your favourite team winning the finals.
c. Five of the next 20 new cars sold in Adelaide will be imported cars.
a. A flipped coin will land on tails.
b. The probability of your favourite team winning the finals.
c. Five of the next 20 new cars sold in Adelaide will be imported cars.

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
60
The probability of the union of two mutually exclusive events A and B is P(A B) = P(A) + P(B).

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
61
A woman is expecting her second child. Her doctor has told her that she has a 50-50 chance of having another girl. If she has another girl, there is a 90% chance that she will be taller than the first. If she has a boy, however, there is only a 25% chance that he will be taller than the first child. Find the probability that the woman's second child will be taller than the first.

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
62
The sample space of the toss of a fair die is S = {1, 2, 3, 4, 5, 6}. If the die is balanced, each simple event has the same probability. Find the probability of the following events.
a. Equal to 1.
b. A number greater than 3.
c. A number greater than 6.
d. A number between 2 and 4, inclusive.
a. Equal to 1.
b. A number greater than 3.
c. A number greater than 6.
d. A number between 2 and 4, inclusive.

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
63
Suppose P(A) = 0.30, P(B) = 0.40, and P(B /A) = 0.60.
a. Find P(A B).
b. Find P(A B).
c. Find P(A /B).
a. Find P(A B).
b. Find P(A B).
c. Find P(A /B).

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
64
The following table shows the numbers of cars sold by a car dealer during the last 30 weeks.  a. Define the random variable of interest to the dealer.
a. Define the random variable of interest to the dealer.
b. List the simple events in the sample space.
c. Assign probabilities to the simple events and show the probability distribution.
d. What approach have you used in determining the probabilities in part (c)?
e. What is the probability of selling no more than four cars in any given week?
 a. Define the random variable of interest to the dealer.
a. Define the random variable of interest to the dealer.b. List the simple events in the sample space.
c. Assign probabilities to the simple events and show the probability distribution.
d. What approach have you used in determining the probabilities in part (c)?
e. What is the probability of selling no more than four cars in any given week?

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
65
A statistics professor classifies his students according to their gender and the number of hours of paid work they do a week. The following table gives the proportions of students falling into the various categories. One student is selected at random.
Paid Work (hours/week) a. If the student selected is female, what is the probability that he works between 1 and 8 hours a week?
b. If the selected student works more than 16 hours a week, what is the probability that the student is male?
c. What is the probability that the student selected is female or does do any paid work or both?
d. Is gender independent of the number of hours of paid work done a week? Explain using probabilities.
Paid Work (hours/week) a. If the student selected is female, what is the probability that he works between 1 and 8 hours a week?
b. If the selected student works more than 16 hours a week, what is the probability that the student is male?
c. What is the probability that the student selected is female or does do any paid work or both?
d. Is gender independent of the number of hours of paid work done a week? Explain using probabilities.

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
66
Is it possible to have two events for which P(A) = 0.40, P(B) = 0.50, and P(A B) = 0.20? Explain.

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
67
Suppose P( ) = 0.10, P( | A) = 0.40, and P( | ) = 0.50.
a. Find P(A).
b. Find P( A).
c. Find P( ).
a. Find P(A).
b. Find P( A).
c. Find P( ).

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
68
Suppose P(A) = 0.10, P(B) = 0.70, and P(B/A) = 0.80.
a. Find P(A B).
b. Find P(A B).
c. Find P(A | B).
a. Find P(A B).
b. Find P(A B).
c. Find P(A | B).

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
69
Suppose P(A) = 0.50, P(B) = 0.30, and P(A or B) = 0.80.
a. Find P(A B).
b. Find P(B | A).
c. Are A and B mutually exclusive events? Explain using probabilities.
a. Find P(A B).
b. Find P(B | A).
c. Are A and B mutually exclusive events? Explain using probabilities.

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
70
A survey of a magazine's subscribers indicates that 40% own a home, 80% own a car, and 90% of the homeowners who subscribe also own a car. What proportion of subscribers:
a. own both a car and a house?
b. own a car or a house, or both?
c. own neither a car nor a house?
a. own both a car and a house?
b. own a car or a house, or both?
c. own neither a car nor a house?

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
71
An insurance company has collected the following data on the gender and marital status of 300 customers.
Marital Status Suppose that a customer is selected at random. Find the probability that the customer selected is:
a. a married female.
b. not single.
c. married, if the customer is male.
d. female or divorced.
e. Are gender and marital status mutually exclusive? Explain using probabilities.
f. Is marital status independent of gender? Explain using probabilities.
Marital Status Suppose that a customer is selected at random. Find the probability that the customer selected is:
a. a married female.
b. not single.
c. married, if the customer is male.
d. female or divorced.
e. Are gender and marital status mutually exclusive? Explain using probabilities.
f. Is marital status independent of gender? Explain using probabilities.

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
72
Suppose P(A) = 0.40, P(B) = 0.50, and P(A B) = 0.70.
a. Find P(A B).
b. Find P(B | A).
c. Are A and B independent events? Explain using probabilities.
a. Find P(A B).
b. Find P(B | A).
c. Are A and B independent events? Explain using probabilities.

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
73
A pharmaceutical firm has discovered a new diagnostic test for a certain disease that has infected 1% of the population. The firm has announced that 95% of those infected will show a positive test result, while 98% of those not infected will show a negative test result. What proportion of test results are correct?

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
74
At the beginning of each year, an investment newsletter predicts whether or not the stock market will rise over the coming year. Historical evidence reveals that there is a 75% chance that the stock market will rise in any given year. The newsletter has predicted a rise for 80% of the years when the market actually rose, and has predicted a rise for 40% of the years when the market fell. Find the probability that the newsletter's prediction for next year will be correct.

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
75
Suppose P(A) = 0.1, P(B) = 0.5, and P(A B) = 0.
a. Find P(A B).
b. Are A and B independent events? Explain.
c. Are A and B mutually exclusive events? Explain.
a. Find P(A B).
b. Are A and B independent events? Explain.
c. Are A and B mutually exclusive events? Explain.

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
76
An financial advisor tells you that in her estimation there is an 85% chance that a particular stock's price will increase over the next three weeks.
a. Which approach was used to produce this figure?
b. Interpret the 85% probability.
a. Which approach was used to produce this figure?
b. Interpret the 85% probability.

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
77
Suppose A and B are two mutually exclusive events for which P(A) = 0.25 and P(B) = 0.60.
a. Find P(A B).
b. Find P(A B).
c. Find P(A | B).
d. Are A and B independent events? Explain using probabilities.
a. Find P(A B).
b. Find P(A B).
c. Find P(A | B).
d. Are A and B independent events? Explain using probabilities.

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
78
Suppose P(A) = 0.10, P(B | A) = 0.20, and P(B | ) = 0.40.
a. Find P(A B).
b. Are A and B mutually exclusive events?
a. Find P(A B).
b. Are A and B mutually exclusive events?

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
79
A standard admissions test was given at three locations. One thousand students took the test at location A, 600 students at location B, and 400 students at location
C. The percentages of students from locations A, B and C who passed the test were 70%, 68% and 77%, respectively. One student is selected at random from among those who took the test.
a. What is the probability that the selected student passed the test?
b. If the selected student passed the test, what is the probability that the student took the test at location B?
c. What is the probability that the selected student took the test at location C and failed?
C. The percentages of students from locations A, B and C who passed the test were 70%, 68% and 77%, respectively. One student is selected at random from among those who took the test.
a. What is the probability that the selected student passed the test?
b. If the selected student passed the test, what is the probability that the student took the test at location B?
c. What is the probability that the selected student took the test at location C and failed?

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck
80
An insurance company has recently recruited ten graduates, four men and six women. Two of the graduates are to be selected at random to work in the firm's suburban office.
a. What is the probability that two men will be selected?
b. What is the probability that at least one man will be selected?
a. What is the probability that two men will be selected?
b. What is the probability that at least one man will be selected?

Unlock Deck
Unlock for access to all 112 flashcards in this deck.
Unlock Deck
k this deck


