Deck 12: Asking and Answering Questions About a Population Mean
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/51
Play
Full screen (f)
Deck 12: Asking and Answering Questions About a Population Mean
1
The spread of the sample mean decreases when
A)the population mean increases.
B)the population standard deviation increases.
C)the sample mean decreases.
D)the sample size decreases.
E)the sample size is increases.
A)the population mean increases.
B)the population standard deviation increases.
C)the sample mean decreases.
D)the sample size decreases.
E)the sample size is increases.
the sample size is increases.
2
Consider sampling from a skewed population. As the sample size, n, increases, some characteristics of the sampling distribution of  change. Does an increasing sample size cause changes in the characteristics of the sampling distribution shown below? If so, specifically how does the sampling distribution change?
change. Does an increasing sample size cause changes in the characteristics of the sampling distribution shown below? If so, specifically how does the sampling distribution change?
a)The mean of the sampling distribution of b)The standard deviation of the sampling distribution of
b)The standard deviation of the sampling distribution of  c)The shape of the sampling distribution of
c)The shape of the sampling distribution of 
 change. Does an increasing sample size cause changes in the characteristics of the sampling distribution shown below? If so, specifically how does the sampling distribution change?
change. Does an increasing sample size cause changes in the characteristics of the sampling distribution shown below? If so, specifically how does the sampling distribution change?a)The mean of the sampling distribution of
 b)The standard deviation of the sampling distribution of
b)The standard deviation of the sampling distribution of  c)The shape of the sampling distribution of
c)The shape of the sampling distribution of 
a)As the sample size increases,  stays the same.
stays the same.
b)As the sample size increases, will decrease.
will decrease.
c)As the sample size increases, the distribution of becomes less skewed and more normal.
becomes less skewed and more normal.
 stays the same.
stays the same.b)As the sample size increases,
 will decrease.
will decrease.c)As the sample size increases, the distribution of
 becomes less skewed and more normal.
becomes less skewed and more normal. 3
A report states that the mean yearly salary offer for students graduating with accounting degrees in 2012 was $49,007. Suppose that a random sample of 60 accounting graduates at a large university who received job offers resulted in a mean offer of $49,800 and a standard deviation of $3,100. What hypotheses should be used to test whether the accounting graduates at this school are securing starting salaries greater than $49,007?
A)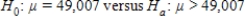
B)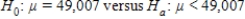
C)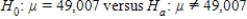
D)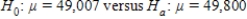
E)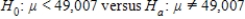
A)
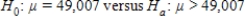
B)
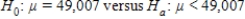
C)
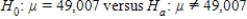
D)
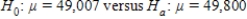
E)
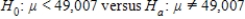
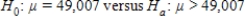
4
The distribution of  is normal if the population is normal.
is normal if the population is normal.
 is normal if the population is normal.
is normal if the population is normal.
Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
5
What is the standard deviation of  for random samples of size 80 from a population with a mean of 48 and a standard deviation of 11?
for random samples of size 80 from a population with a mean of 48 and a standard deviation of 11?
A)0.138
B)1.23
C)0.371
D)11
E)6.6
 for random samples of size 80 from a population with a mean of 48 and a standard deviation of 11?
for random samples of size 80 from a population with a mean of 48 and a standard deviation of 11? A)0.138
B)1.23
C)0.371
D)11
E)6.6

Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
6
The distribution of  will always have the same shape as the distribution of the population being sampled.
will always have the same shape as the distribution of the population being sampled.
 will always have the same shape as the distribution of the population being sampled.
will always have the same shape as the distribution of the population being sampled.
Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
7
A packaging machine is supposed to fill boxes of breakfast cereal to a weight of 16 ounces. Eight filled boxes are selected at random and the weight of cereal is determined. The results (in ounces) are summarized in the following table.  A technician wishes to test the claim that the machine is overfilling the boxes. At the 5% significance level, what is the P-value of the test statistic? (Assume the filling weights are normally distributed.)
A technician wishes to test the claim that the machine is overfilling the boxes. At the 5% significance level, what is the P-value of the test statistic? (Assume the filling weights are normally distributed.)
A)0.262
B)0.005
C)0.032
D)0.081
E)0.115
 A technician wishes to test the claim that the machine is overfilling the boxes. At the 5% significance level, what is the P-value of the test statistic? (Assume the filling weights are normally distributed.)
A technician wishes to test the claim that the machine is overfilling the boxes. At the 5% significance level, what is the P-value of the test statistic? (Assume the filling weights are normally distributed.)A)0.262
B)0.005
C)0.032
D)0.081
E)0.115

Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
8
The width of the one-sample confidence interval for μ decreases as the sample size grows larger.

Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
9
As n grows larger, the mean of the sampling distribution of  gets closer to μ.
gets closer to μ.
 gets closer to μ.
gets closer to μ.
Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
10
The t confidence interval formula for estimating μ should only be used when the population being sampled is at least approximately normally distributed.

Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
11
Let  represent the mean value of the
represent the mean value of the  sampling distribution and
sampling distribution and  represent the population mean. What can be done to reduce the difference between
represent the population mean. What can be done to reduce the difference between  and
and  ?
?
A)increase the sample size
B)decrease the sample size
C)decrease the population standard deviation
D)increase the population standard deviation
E)nothing, since for any population distribution.
for any population distribution.
 represent the mean value of the
represent the mean value of the  sampling distribution and
sampling distribution and  represent the population mean. What can be done to reduce the difference between
represent the population mean. What can be done to reduce the difference between  and
and  ?
? A)increase the sample size
B)decrease the sample size
C)decrease the population standard deviation
D)increase the population standard deviation
E)nothing, since
 for any population distribution.
for any population distribution.
Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
12
A recent survey sampled 1,086 parents of school-aged children. One question on the survey asked respondents how much time per month (in hours) they spent volunteering at their children's school during the previous school year. The following summary statistics for time volunteered per month were given: 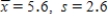
Which of the following represents the 98% confidence interval?
A)(5.445, 5.755)
B)(5.277, 5.923)
C)(5.290, 5.909)
D)(5.416, 5.784)
E)(5.193, 6.007)
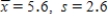
Which of the following represents the 98% confidence interval?
A)(5.445, 5.755)
B)(5.277, 5.923)
C)(5.290, 5.909)
D)(5.416, 5.784)
E)(5.193, 6.007)

Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
13
A t curve is bell-shaped like the z curve but is less spread out.

Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
14
The confidence interval formula for estimating μ when n is large is based on the Central Limit Theorem.

Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
15
The standard deviation of the distribution of  decreases as n increases.
decreases as n increases.
 decreases as n increases.
decreases as n increases.
Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
16
For n sufficiently large, the distribution of 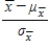 is approximately a standard normal distribution.
is approximately a standard normal distribution.
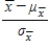 is approximately a standard normal distribution.
is approximately a standard normal distribution.
Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
17
Commute distances for employees of a large company have a distribution that is quite skewed. Suppose the mean commute distance is 20 miles and that the standard deviation is 7 miles. What can be said about the mean commute distance for a random sample of size 49 employees from this plant?
A)The distribution of will be approximately normal with mean 20 and standard deviation 7.
will be approximately normal with mean 20 and standard deviation 7.
B)The distribution of will be approximately normal with mean 20 and standard deviation 1.
will be approximately normal with mean 20 and standard deviation 1.
C)The mean and standard deviation of will be 20 and 7, but the sampling distribution of
will be 20 and 7, but the sampling distribution of  will not be approximately normal.
will not be approximately normal.
D)The mean and standard deviation of will be 20 and 1, but the sampling distribution of
will be 20 and 1, but the sampling distribution of  will not be approximately normal.
will not be approximately normal.
E) will have a standard normal distribution.
will have a standard normal distribution.
A)The distribution of
 will be approximately normal with mean 20 and standard deviation 7.
will be approximately normal with mean 20 and standard deviation 7.B)The distribution of
 will be approximately normal with mean 20 and standard deviation 1.
will be approximately normal with mean 20 and standard deviation 1.C)The mean and standard deviation of
 will be 20 and 7, but the sampling distribution of
will be 20 and 7, but the sampling distribution of  will not be approximately normal.
will not be approximately normal.D)The mean and standard deviation of
 will be 20 and 1, but the sampling distribution of
will be 20 and 1, but the sampling distribution of  will not be approximately normal.
will not be approximately normal.E)
 will have a standard normal distribution.
will have a standard normal distribution.
Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
18
The standard error of  is s.
is s.
 is s.
is s.
Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
19
Suppose you take a random sample from a population known to be normally distributed, but the value of σ is unknown. Your sample size is n =15. Which formula below should be used to find the 95% confidence interval for the mean?
A)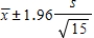
B)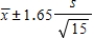
C)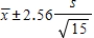
D)
E)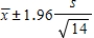
A)
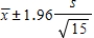
B)
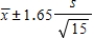
C)
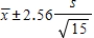
D)

E)
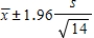

Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
20
For a random sample from a skewed population, for which of the following sample sizes would the sampling distribution of  be closest to normal in shape?
be closest to normal in shape?
A)n = 11
B)n = 18
C)n = 19
D)n = 22
E)n = 40
 be closest to normal in shape?
be closest to normal in shape? A)n = 11
B)n = 18
C)n = 19
D)n = 22
E)n = 40

Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
21
In cities and towns on the borders between states there is a significant amount of flight across state lines to avoid high state taxes on gasoline. Some states (such as Iowa) have large rivers for borders and tolls to cross the bridges. Do these tolls impede traffic to other states to get cheaper gasoline? To test this hypothesis, an experimental Toll-Free Week will be instituted at the Farmington Bridge, where currently 50 cars per day drive out of the state. Let μ denote the true average number of border crossings per day at Farmington if there were no toll.
a)What is the appropriate null hypothesis?
b)What is the appropriate alternative hypothesis?
c)In your own words, distinguish between a Type I and a Type II error in this context.
a)What is the appropriate null hypothesis?
b)What is the appropriate alternative hypothesis?
c)In your own words, distinguish between a Type I and a Type II error in this context.

Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
22
A boat manufacturer claims that a particular boat and motor combination will burn less than 4.0 gallons of fuel per hour. Fuel consumption for a random sample of 10 similar boats resulted in the data below:
4.06, 4.29, 4.26, 4.64, 4.23, 3.93, 3.64, 4.13, 3.93, 3.86
Is there sufficient evidence to conclude that the manufacturer's claim is correct? Use α = .05 and test the appropriate hypothesis.
4.06, 4.29, 4.26, 4.64, 4.23, 3.93, 3.64, 4.13, 3.93, 3.86
Is there sufficient evidence to conclude that the manufacturer's claim is correct? Use α = .05 and test the appropriate hypothesis.

Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
23
A random sample of the houses in a particular city is selected and the level of radon gas is measured for each house in the sample. The values collected are given below in parts per million (ppm). Experience has shown that radon gas level is approximately normally distributed for this population.
4, 8, 13, 6, 7, 3, 9, 8, 11, 8
a)Calculate an estimate of the population mean of radon gas levels.
b)Construct and interpret a 99% confidence interval for μ.
c)The city standards for radon call for no more than a level of 6 ppm. From these data, does it appear that the mean radon level for city houses is under 6 ppm? Provide statistical justification for your response.
4, 8, 13, 6, 7, 3, 9, 8, 11, 8
a)Calculate an estimate of the population mean of radon gas levels.
b)Construct and interpret a 99% confidence interval for μ.
c)The city standards for radon call for no more than a level of 6 ppm. From these data, does it appear that the mean radon level for city houses is under 6 ppm? Provide statistical justification for your response.

Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
24
Under what conditions is it reasonable to use the t confidence interval to estimate a population mean?

Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
25
The foraging capability of Downy Woodpeckers can be compromised if part of their habitat disappears due to significant human encroachment. These creatures eat insects in trees, and if they cannot find enough food due to a decreasing forest area their body mass may decrease. A 20-year-old study of Downy Woodpeckers indicates that for male Downy Woodpeckers the average mass is about 28g, with a standard deviation of approximately 1.5g. Suppose it is desired to estimate μ, the average mass of male Downy Woodpeckers in a local forest preserve. If the 20-year-old values are taken as reasonable estimates, determine the sample size necessary to estimate μ to within 0.2 grams with 95% confidence.

Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
26
At Abraham Lincoln High School a popular achievement test is used for college placement. Counselors selected a random sample of 100 students from Abraham Lincoln High School and scored their tests by hand before sending them in for computer processing. For this sample, 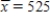 and s = 25. Construct a 99% confidence interval for μ, the population mean score for Abraham Lincoln High.
and s = 25. Construct a 99% confidence interval for μ, the population mean score for Abraham Lincoln High.
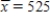 and s = 25. Construct a 99% confidence interval for μ, the population mean score for Abraham Lincoln High.
and s = 25. Construct a 99% confidence interval for μ, the population mean score for Abraham Lincoln High.
Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
27
How are the quantities,  and
and  , related?
, related?
 and
and  , related?
, related?
Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
28
The principal at John F. Kennedy High School has been asked to provide the average number of classes taken by the students at KHS. Since the computer system is down, she takes her alphabetized list of students, randomly selects 50 students, determines the number of classes each of the 50 selected students is taking, and calculates 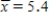 . She then reports to the PTA "Since I took a large random sample, the population mean number of classes taken by the students at KHS is 5.4." Write a short paragraph to send to her that explains why her statement is not correct.
. She then reports to the PTA "Since I took a large random sample, the population mean number of classes taken by the students at KHS is 5.4." Write a short paragraph to send to her that explains why her statement is not correct.
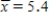 . She then reports to the PTA "Since I took a large random sample, the population mean number of classes taken by the students at KHS is 5.4." Write a short paragraph to send to her that explains why her statement is not correct.
. She then reports to the PTA "Since I took a large random sample, the population mean number of classes taken by the students at KHS is 5.4." Write a short paragraph to send to her that explains why her statement is not correct.
Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
29
Under what conditions is the z confidence interval an appropriate way to estimate a population mean?

Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
30
According to internal testing done by the HardCore Apple Company, the mean firmness of their apples, as measured on the Mohs hardness scale is 7.0, with a standard deviation of 0.3.
a)If the claim by Hardcore is true, what is the mean of the sampling distribution of for samples of size n = 16?
for samples of size n = 16?
b)If the claim by HardCore is true, what is the standard deviation of the sampling distribution of for samples of size n = 16?
for samples of size n = 16?
c)If the distribution of HardCore firmness is approximately normal, and their claim about the mean firmness is true, what is the probability that the mean firmness of a random sample of size n = 16 will be less than 6.9?
a)If the claim by Hardcore is true, what is the mean of the sampling distribution of
 for samples of size n = 16?
for samples of size n = 16?b)If the claim by HardCore is true, what is the standard deviation of the sampling distribution of
 for samples of size n = 16?
for samples of size n = 16?c)If the distribution of HardCore firmness is approximately normal, and their claim about the mean firmness is true, what is the probability that the mean firmness of a random sample of size n = 16 will be less than 6.9?

Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
31
Reading comprehension is arguably the single most important skill a student possesses. To assess the current levels of reading comprehension in 3rd grade, 25 students were randomly selected from school district class lists and given a reading comprehension test. The distribution of their scores was approximately normal with a mean of 180 and a standard deviation of 20.12. Construct a 99% confidence interval for μ, the population mean.

Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
32
The composition of the earth's atmosphere may have changed over time. One attempt to discover the nature of the atmosphere long ago involved measuring the gas trapped in bubbles inside ancient amber. Amber is tree resin that has hardened and been trapped in rocks. Measurements on nine amber specimens from the late Cretaceous era (95 to 65 million years ago) give the following percentages of nitrogen:
63.4, 65.0, 64.4, 63.3, 54.8, 68.5, 60.8, 59.1, 51.0
Assume that it is reasonable to regard these trapped gas bubbles as a random sample of bubbles from the Cretaceous atmosphere.
a)Construct and interpret a 95% confidence interval for the mean percentage of nitrogen in the Cretaceous atmosphere.
b)The mean percentage of nitrogen in the atmosphere today is about 79%. Based on the confidence interval from part (a), do you think that the percentage of the atmosphere that is nitrogen has changed from the late Cretaceous era? Explain.
63.4, 65.0, 64.4, 63.3, 54.8, 68.5, 60.8, 59.1, 51.0
Assume that it is reasonable to regard these trapped gas bubbles as a random sample of bubbles from the Cretaceous atmosphere.
a)Construct and interpret a 95% confidence interval for the mean percentage of nitrogen in the Cretaceous atmosphere.
b)The mean percentage of nitrogen in the atmosphere today is about 79%. Based on the confidence interval from part (a), do you think that the percentage of the atmosphere that is nitrogen has changed from the late Cretaceous era? Explain.

Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
33
When performing tests of hypotheses, there are assumptions that must be met in order for the test to be appropriate. For the test of a hypothesis about a population mean, and given the raw data, describe how you would check the assumptions.

Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
34
One indicator of the health of field mice is the length of their tails. The following data were gathered on a random sample of field mice. A University biologist would like to construct a 95% confidence interval for the population mean tail length of the field mice near Fairfield. (Measures are in mm). 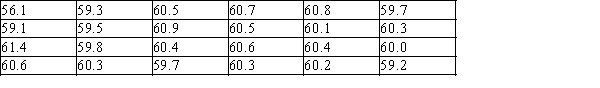 a)Using a graphical display of your choice, display the data in a way that will allow you to determine whether it would be appropriate to use a 95% t confidence interval to estimate the population mean.
a)Using a graphical display of your choice, display the data in a way that will allow you to determine whether it would be appropriate to use a 95% t confidence interval to estimate the population mean.
b)Using the graphical display from part (a), would you advise that constructing a 95% confidence interval is appropriate? Provide statistical justification for your answer.
 a)Using a graphical display of your choice, display the data in a way that will allow you to determine whether it would be appropriate to use a 95% t confidence interval to estimate the population mean.
a)Using a graphical display of your choice, display the data in a way that will allow you to determine whether it would be appropriate to use a 95% t confidence interval to estimate the population mean.b)Using the graphical display from part (a), would you advise that constructing a 95% confidence interval is appropriate? Provide statistical justification for your answer.

Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
35
Psychologists have noted that people tend make classifications based on their "typicality." For example, a dog is more readily classified as a mammal than is a whale, because dogs are "more typical" of mammals than are whales. Previous large-scale studies have established the following for songbirds such as robins: when young children are shown a picture of a songbird and asked if it is a bird they respond "yes" in an average of 750 milliseconds. Do children regard chickens as representative of birds? If so, are they more representative or less representative of birds than are songbirds? Let μ represent the mean time it takes children to respond to a question about whether a chicken is a bird. The investigators wish to determine whether the classification time differs from the time needed to classify songbirds.
a)What is the appropriate null hypothesis?
b)What is the appropriate alternative hypothesis?
c)In your own words, distinguish between a Type I and a Type II error in this context.
a)What is the appropriate null hypothesis?
b)What is the appropriate alternative hypothesis?
c)In your own words, distinguish between a Type I and a Type II error in this context.

Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
36
The department of natural resources defines a fish to be unsafe to eat if the polychlorinated biphenol (PCB) concentration exceeds 5 parts per billion (ppb). A random sample of 10 fish taken from a local lake resulted in the data listed below:
2.6, 6.6, 4.8, 5.4, 5.1, 4.5, 6.9, 4.9, 3.7, 3.8
Is there sufficient evidence to conclude that the mean PCB concentration for fish from this lake exceeds 5 ppb?
2.6, 6.6, 4.8, 5.4, 5.1, 4.5, 6.9, 4.9, 3.7, 3.8
Is there sufficient evidence to conclude that the mean PCB concentration for fish from this lake exceeds 5 ppb?

Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
37
Many national parks, wildlife refuges, and wilderness areas lie directly below designated military air space. This raises issues of whether wildlife and, for example, loud jet noises are compatible. In an effort to evaluate the effects of low-altitude jet-noise, researchers simulated these noises for captive desert mule deer. Three minutes after the loud noises were played, the heart rates (beats per minute) of these animals was measured, with the following results:
55.9, 51.1, 57.5, 65.5, 68.4, 64.9, 63.1, 55.3, 63.3
Assume that it is reasonable to think that these deer can be regarded as a random sample of desert mule deer.
a)Calculate and interpret a 95% confidence interval for the mean heart rate 3 minutes after loud jet noises.
b)The mean heart rate for desert mule deer is about 51.2 beats per minute. Based on the confidence interval in part (a), do you think that the loud jet noise has some lingering effect on the heart rate of desert mule deer? Explain.
55.9, 51.1, 57.5, 65.5, 68.4, 64.9, 63.1, 55.3, 63.3
Assume that it is reasonable to think that these deer can be regarded as a random sample of desert mule deer.
a)Calculate and interpret a 95% confidence interval for the mean heart rate 3 minutes after loud jet noises.
b)The mean heart rate for desert mule deer is about 51.2 beats per minute. Based on the confidence interval in part (a), do you think that the loud jet noise has some lingering effect on the heart rate of desert mule deer? Explain.

Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
38
Under what conditions is it reasonable to use the z confidence interval to estimate a population mean?

Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
39
The Environmental Protection Agency (EPA) is charged with monitoring the environment. One aspect of this is keeping track of "acid rain," a broad term describing the fall of water through an acidic atmosphere. Acidity is measured on the pH scale, where pure water has a pH of 7.0. Normal rain is slightly acidic because carbon dioxide dissolves into it, and thus has a pH of about 5.5. (A lower pH indicates greater acidity.) Suppose the EPA wishes to determine whether a particular area is subject to acid rain. Let μ denote the true average for pH in this area.
a)What is the appropriate null hypothesis?
b)What is the appropriate alternative hypothesis?
c)In your own words, distinguish between a Type I and a Type II error in this context.
a)What is the appropriate null hypothesis?
b)What is the appropriate alternative hypothesis?
c)In your own words, distinguish between a Type I and a Type II error in this context.

Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
40
A new species of sea crab has been discovered, and an experiment conducted to determine whether or not the animal can regulate its temperature. That the animal can maintain a body temperature different from the surroundings would be considered evidence of this regulating capability. Ten of these sea crabs were exposed to ambient temperatures of 24 degrees Celsius. Their body temperatures were measured with the results below:
24.33, 24.61, 24.67, 24.64, 24.42, 24.97, 25.23, 24.73, 24.90, 24.44
For purposes of this example, assume that it is reasonable to regard these 10 crabs as a random sample from the population of all crabs of this species.
a)Calculate an estimate of the population mean.
b)Construct and interpret a 99% confidence interval for μ.
c)Does it appear from these data that the crabs are able to regulate their body temperature? Provide statistical justification for your response.
24.33, 24.61, 24.67, 24.64, 24.42, 24.97, 25.23, 24.73, 24.90, 24.44
For purposes of this example, assume that it is reasonable to regard these 10 crabs as a random sample from the population of all crabs of this species.
a)Calculate an estimate of the population mean.
b)Construct and interpret a 99% confidence interval for μ.
c)Does it appear from these data that the crabs are able to regulate their body temperature? Provide statistical justification for your response.

Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
41
A company provides portable walkie-talkies to construction crews. Their batteries last, on average, 55 hours of continuous use. The purchasing manager receives a brochure advertising a new brand of batteries with a lower price, but suspects that the lifetime of the batteries may be shorter than the brand currently in use. To test this, the new brand is installed in 8 randomly selected radios. Here are the results for the lifetime of the batteries (in hours):
45 52 56 55 51 57 48 52
Is there sufficient evidence to conclude that the purchasing manager is correct in his conjecture that the new brand has a shorter average lifetime?
45 52 56 55 51 57 48 52
Is there sufficient evidence to conclude that the purchasing manager is correct in his conjecture that the new brand has a shorter average lifetime?

Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
42
Researchers studied the average height of 12 years old girls in the city. They determined that the mean growth for a representative sample of 10 girls was 59.4 inches. The original sample data values are:  Select the most appropriate 90% bootstrap confidence interval for a population mean height of 12 years old girls in the city and its correct interpretation.
Select the most appropriate 90% bootstrap confidence interval for a population mean height of 12 years old girls in the city and its correct interpretation.
A)The bootstrap confidence interval is .You can be 90% confident that the population mean height of 12 years old girls is between 55.9 and 62.6 inches.
.You can be 90% confident that the population mean height of 12 years old girls is between 55.9 and 62.6 inches.
B)The bootstrap confidence interval is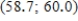 .You can be 90% confident that the population mean height of 12 years old girls is between 58.5 and 60.2 inches.
.You can be 90% confident that the population mean height of 12 years old girls is between 58.5 and 60.2 inches.
C)The bootstrap confidence interval is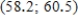 .You can be 90% confident that the population mean height of 12 years old girls is between 58.2 and 60.5 inches.
.You can be 90% confident that the population mean height of 12 years old girls is between 58.2 and 60.5 inches.
D)The bootstrap confidence interval is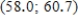 .You can be 90% confident that the population mean height of 12 years old girls is between 58.0 and 60.7 inches.
.You can be 90% confident that the population mean height of 12 years old girls is between 58.0 and 60.7 inches.
E)The bootstrap confidence interval is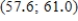 .You can be 90% confident that the population mean height of 12 years old girls is between 57.6 and 61.0 inches.
.You can be 90% confident that the population mean height of 12 years old girls is between 57.6 and 61.0 inches.
 Select the most appropriate 90% bootstrap confidence interval for a population mean height of 12 years old girls in the city and its correct interpretation.
Select the most appropriate 90% bootstrap confidence interval for a population mean height of 12 years old girls in the city and its correct interpretation.A)The bootstrap confidence interval is
 .You can be 90% confident that the population mean height of 12 years old girls is between 55.9 and 62.6 inches.
.You can be 90% confident that the population mean height of 12 years old girls is between 55.9 and 62.6 inches.B)The bootstrap confidence interval is
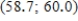 .You can be 90% confident that the population mean height of 12 years old girls is between 58.5 and 60.2 inches.
.You can be 90% confident that the population mean height of 12 years old girls is between 58.5 and 60.2 inches.C)The bootstrap confidence interval is
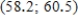 .You can be 90% confident that the population mean height of 12 years old girls is between 58.2 and 60.5 inches.
.You can be 90% confident that the population mean height of 12 years old girls is between 58.2 and 60.5 inches.D)The bootstrap confidence interval is
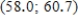 .You can be 90% confident that the population mean height of 12 years old girls is between 58.0 and 60.7 inches.
.You can be 90% confident that the population mean height of 12 years old girls is between 58.0 and 60.7 inches.E)The bootstrap confidence interval is
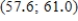 .You can be 90% confident that the population mean height of 12 years old girls is between 57.6 and 61.0 inches.
.You can be 90% confident that the population mean height of 12 years old girls is between 57.6 and 61.0 inches.
Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
43
In your own words, distinguish between "statistical" and "practical" significance.

Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
44
The lecturer decided to estimate the mean mental reading speed for the university students. Randomly, seventy nine students were selected and the speed of their reading was recorded. For this sample, the mean reading speed was 235 words per minute. Suppose that the sample standard deviation was 46 words per minute. Find the margin of error and construct a 95% confidence interval for the mean reading speed for the university students.
A)Margin of error is 10 words per minute, a 95% confidence interval is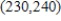 .
.
B)Margin of error is 5 words per minute, a 95% confidence interval is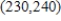 .
.
C)Margin of error is 5 words per minute, a 95% confidence interval is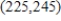 .
.
D)Margin of error is 10 words per minute, a 95% confidence interval is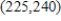 .
.
E)Margin of error is 10 words per minute, a 95% confidence interval is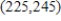 .
.
A)Margin of error is 10 words per minute, a 95% confidence interval is
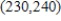 .
.B)Margin of error is 5 words per minute, a 95% confidence interval is
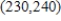 .
.C)Margin of error is 5 words per minute, a 95% confidence interval is
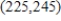 .
.D)Margin of error is 10 words per minute, a 95% confidence interval is
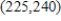 .
.E)Margin of error is 10 words per minute, a 95% confidence interval is
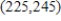 .
.
Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
45
Researchers studied how many emails a day receive the office workers of the large company. They determined that the mean number of incoming emails per day for a representative sample of 7 office workers was 108 emails. The original sample data values are:  Select the most appropriate 99% bootstrap confidence interval for a population mean number of incoming emails per day for office workers of the large company and its correct interpretation.
Select the most appropriate 99% bootstrap confidence interval for a population mean number of incoming emails per day for office workers of the large company and its correct interpretation.
A)The bootstrap confidence interval is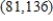 .You can be 99% confident that the population mean number of incoming emails per day is between 81 and 136 emails.
.You can be 99% confident that the population mean number of incoming emails per day is between 81 and 136 emails.
B)The bootstrap confidence interval is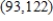 .You can be 99% confident that the population mean number of incoming emails per day is between 93 and 122 emails.
.You can be 99% confident that the population mean number of incoming emails per day is between 93 and 122 emails.
C)The bootstrap confidence interval is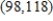 .You can be 99% confident that the population mean number of incoming emails per day is between 98 and 118 emails.
.You can be 99% confident that the population mean number of incoming emails per day is between 98 and 118 emails.
D)The bootstrap confidence interval is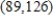 .You can be 99% confident that the population mean number of incoming emails per day is between 89 and 126 emails.
.You can be 99% confident that the population mean number of incoming emails per day is between 89 and 126 emails.
E)The bootstrap confidence interval is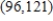 .You can be 99% confident that the population mean number of incoming emails per day is between 96 and 121 emails.
.You can be 99% confident that the population mean number of incoming emails per day is between 96 and 121 emails.
 Select the most appropriate 99% bootstrap confidence interval for a population mean number of incoming emails per day for office workers of the large company and its correct interpretation.
Select the most appropriate 99% bootstrap confidence interval for a population mean number of incoming emails per day for office workers of the large company and its correct interpretation.A)The bootstrap confidence interval is
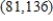 .You can be 99% confident that the population mean number of incoming emails per day is between 81 and 136 emails.
.You can be 99% confident that the population mean number of incoming emails per day is between 81 and 136 emails.B)The bootstrap confidence interval is
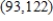 .You can be 99% confident that the population mean number of incoming emails per day is between 93 and 122 emails.
.You can be 99% confident that the population mean number of incoming emails per day is between 93 and 122 emails.C)The bootstrap confidence interval is
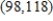 .You can be 99% confident that the population mean number of incoming emails per day is between 98 and 118 emails.
.You can be 99% confident that the population mean number of incoming emails per day is between 98 and 118 emails.D)The bootstrap confidence interval is
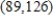 .You can be 99% confident that the population mean number of incoming emails per day is between 89 and 126 emails.
.You can be 99% confident that the population mean number of incoming emails per day is between 89 and 126 emails.E)The bootstrap confidence interval is
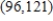 .You can be 99% confident that the population mean number of incoming emails per day is between 96 and 121 emails.
.You can be 99% confident that the population mean number of incoming emails per day is between 96 and 121 emails.
Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
46
In an analysis of hunting by African lions, biologists filmed prey captures from the safety of their vehicles. The capture of prey was divided into a sequence of events for study one of which is the stalk, defined as the reduction of predator-prey distance for prey that has been specifically located and the prey is unaware of or minimally alarmed by the predator. The investigators identified two types of stalk: (a) "crouching"--the lion is concealed and either the lion advances toward the prey or the prey advances (unaware) toward the lion, and (b) "running"--the lion is less concealed and advances toward the prey in a rapid manner.
Data on lions' stalks of wildebeests and zebras from a simple random sample of 159 kills appear in the table below.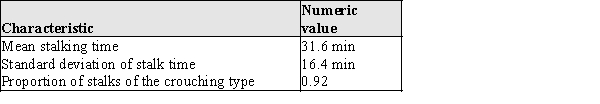
a)By monitoring radio-collared lions for many years, biologists believe that the average stalking time among all prey is approximately 25.6 minutes. Do the data above provide evidence that for this population of lions the average time to stalk wildebeests and zebras is different from what was originally thought?
b)The same monitoring of radio-collared lions over the years suggests that the overall proportion of stalks that are the crouching type is about 0.87. Do the data above provide evidence that for this population of lions the proportion of crouching stalks of wildebeests and zebras is greater than what was originally thought?
Data on lions' stalks of wildebeests and zebras from a simple random sample of 159 kills appear in the table below.

a)By monitoring radio-collared lions for many years, biologists believe that the average stalking time among all prey is approximately 25.6 minutes. Do the data above provide evidence that for this population of lions the average time to stalk wildebeests and zebras is different from what was originally thought?
b)The same monitoring of radio-collared lions over the years suggests that the overall proportion of stalks that are the crouching type is about 0.87. Do the data above provide evidence that for this population of lions the proportion of crouching stalks of wildebeests and zebras is greater than what was originally thought?

Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
47
Suppose that a study has been published linking arsenic, which is commonly found in tap water, to increased cancer rates. The study also stated that arsenic levels below 10 ppm could be considered harmless. Suppose that residents of Hacienda Heights, CA, are worried about the arsenic level in the local tap water.
a)What hypothesis should they test?
b)Describe a Type I and Type II error in this context.
a)What hypothesis should they test?
b)Describe a Type I and Type II error in this context.

Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
48
An important part of any dispensing process is statistical quality control. At the Billy Goat Gruff Inn, machines are set to dispense 600 ml of soda into every customer's glass. Over time, however, the machine can get "out of control" and dispense too much soda or too little. At a random point in time each clock hour, the owner dispenses and checks a glass of dispensed soda and determines the actual volume of soda dispensed. One day the volumes of the dispensed soda were:
600.15, 599.92, 599.85, 599.92, 599.81, 600.14, 600.04, 599.98
Is there sufficient evidence to conclude that the dispensing machine needs some adjustment?
600.15, 599.92, 599.85, 599.92, 599.81, 600.14, 600.04, 599.98
Is there sufficient evidence to conclude that the dispensing machine needs some adjustment?

Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
49
Researchers studied how many steps a day adult residents of the city make. They determined that the mean number of steps per day for a representative sample of 10 adult residents of the city was 5,139 steps. The original sample data values are:  Researchers are interested in deciding if there is evidence that adult residents of the city make more than 5,000 steps per day. Use a randomization test to select the appropriate output for one set of 1,000 simulated sample means and carry out a hypothesis test for a population mean.
Researchers are interested in deciding if there is evidence that adult residents of the city make more than 5,000 steps per day. Use a randomization test to select the appropriate output for one set of 1,000 simulated sample means and carry out a hypothesis test for a population mean.
A)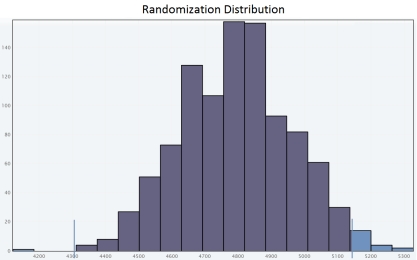
 Since the approximate P-value is less than
Since the approximate P-value is less than 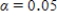 , we fail to reject H0 for a significance level of 0.05.So the sample does not provide convincing evidence that adult residents of the city make more than 5,000 steps per day.
, we fail to reject H0 for a significance level of 0.05.So the sample does not provide convincing evidence that adult residents of the city make more than 5,000 steps per day.
B)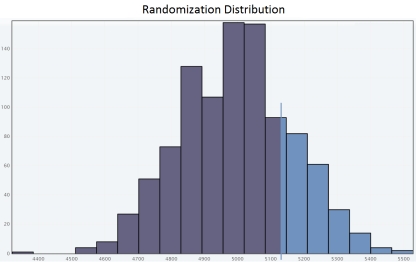
 Since the approximate P-value is greater than
Since the approximate P-value is greater than 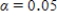 , we reject H0 for a significance level of 0.05.So the sample provide convincing evidence that adult residents of the city make more than 5,000 steps per day.
, we reject H0 for a significance level of 0.05.So the sample provide convincing evidence that adult residents of the city make more than 5,000 steps per day.
C)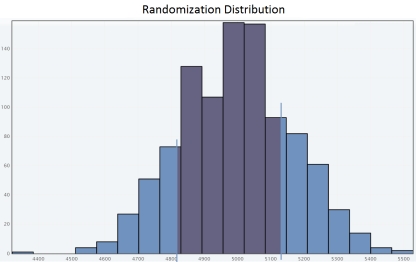

Since the approximate P-value is greater than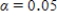 , we fail to reject H0 for a significance level of 0.05.So the sample does not provide convincing evidence that adult residents of the city make more than 5,000 steps per day.
, we fail to reject H0 for a significance level of 0.05.So the sample does not provide convincing evidence that adult residents of the city make more than 5,000 steps per day.
D)

Since the approximate P-value is less than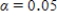 , we reject H0 for a significance level of 0.05.So the sample provide convincing evidence that adult residents of the city make more than 5,000 steps per day.
, we reject H0 for a significance level of 0.05.So the sample provide convincing evidence that adult residents of the city make more than 5,000 steps per day.
E)

Since the approximate P-value is greater than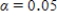 , we fail to reject H0 for a significance level of 0.05.So the sample does not provide convincing evidence that adult residents of the city make more than 5,000 steps per day.
, we fail to reject H0 for a significance level of 0.05.So the sample does not provide convincing evidence that adult residents of the city make more than 5,000 steps per day.
 Researchers are interested in deciding if there is evidence that adult residents of the city make more than 5,000 steps per day. Use a randomization test to select the appropriate output for one set of 1,000 simulated sample means and carry out a hypothesis test for a population mean.
Researchers are interested in deciding if there is evidence that adult residents of the city make more than 5,000 steps per day. Use a randomization test to select the appropriate output for one set of 1,000 simulated sample means and carry out a hypothesis test for a population mean.A)

 Since the approximate P-value is less than
Since the approximate P-value is less than 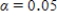 , we fail to reject H0 for a significance level of 0.05.So the sample does not provide convincing evidence that adult residents of the city make more than 5,000 steps per day.
, we fail to reject H0 for a significance level of 0.05.So the sample does not provide convincing evidence that adult residents of the city make more than 5,000 steps per day.B)

 Since the approximate P-value is greater than
Since the approximate P-value is greater than 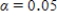 , we reject H0 for a significance level of 0.05.So the sample provide convincing evidence that adult residents of the city make more than 5,000 steps per day.
, we reject H0 for a significance level of 0.05.So the sample provide convincing evidence that adult residents of the city make more than 5,000 steps per day.C)


Since the approximate P-value is greater than
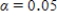 , we fail to reject H0 for a significance level of 0.05.So the sample does not provide convincing evidence that adult residents of the city make more than 5,000 steps per day.
, we fail to reject H0 for a significance level of 0.05.So the sample does not provide convincing evidence that adult residents of the city make more than 5,000 steps per day.D)


Since the approximate P-value is less than
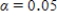 , we reject H0 for a significance level of 0.05.So the sample provide convincing evidence that adult residents of the city make more than 5,000 steps per day.
, we reject H0 for a significance level of 0.05.So the sample provide convincing evidence that adult residents of the city make more than 5,000 steps per day.E)


Since the approximate P-value is greater than
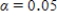 , we fail to reject H0 for a significance level of 0.05.So the sample does not provide convincing evidence that adult residents of the city make more than 5,000 steps per day.
, we fail to reject H0 for a significance level of 0.05.So the sample does not provide convincing evidence that adult residents of the city make more than 5,000 steps per day.
Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
50
Researchers conducted a survey among 1793 families of a certain city who reported that they participated in charity one or more times in 2016. The sample was selected in a way to be representative for families of a certain city who participated in charity in 2016. For this sample, the mean amount of money spent on charity was $876. Suppose that the sample standard deviation was $514. Find the margin of error and construct a 95% confidence interval for the mean amount of money spent in the certain city on charity in 2016.
A)Margin of error is $23.79, a 95% confidence interval is (852.21,899.79).
B)Margin of error is $12.14, a 95% confidence interval is (863.86,888.14).
C)Margin of error is $23.79, a 95% confidence interval is (863.86,888.14).
D)Margin of error is $12.14, a 95% confidence interval is (852.21,899.79).
E)Margin of error is $23.79, a 95% confidence interval is (852.21,888.14).
A)Margin of error is $23.79, a 95% confidence interval is (852.21,899.79).
B)Margin of error is $12.14, a 95% confidence interval is (863.86,888.14).
C)Margin of error is $23.79, a 95% confidence interval is (863.86,888.14).
D)Margin of error is $12.14, a 95% confidence interval is (852.21,899.79).
E)Margin of error is $23.79, a 95% confidence interval is (852.21,888.14).

Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck
51
In an analysis of hunting by African lions, biologists filmed prey captures from the safety of their vehicles. The capture of prey was divided into a sequence of events for study, one of which is the stalk, defined as the reduction of predator-prey distance for prey that has been specifically located and the prey is unaware of or minimally alarmed by the predator. The investigators identified two types of stalk: (a) "crouching,"--the lion is concealed and either the lion advances toward the prey or the prey advances (unaware) toward the lion, and (b) "running,"--the lion is less concealed and advances toward the prey in a rapid manner.
Data on lions' stalks of Thomson's and Grant's gazelles from a sample of 151 kills appear in the table below.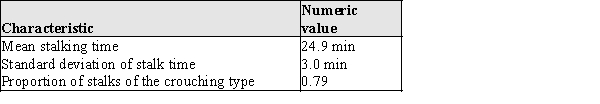
a)On the basis of monitoring radio-collared lions for many years, biologists believe that the average stalking time for all prey is approximately 25.6 minutes. Do the data above provide evidence that for this population of lions the average time to stalk Thomson's and Grant's gazelles is different from what was originally thought?
b)The same monitoring of radio-collared lions over the years has suggested that the overall proportion of stalks that are the crouching type is about 0.87. Do the data above provide evidence that for this population of lions the proportion of crouching stalks of Thomson's and Grant's gazelles is less than what was originally thought?
Data on lions' stalks of Thomson's and Grant's gazelles from a sample of 151 kills appear in the table below.

a)On the basis of monitoring radio-collared lions for many years, biologists believe that the average stalking time for all prey is approximately 25.6 minutes. Do the data above provide evidence that for this population of lions the average time to stalk Thomson's and Grant's gazelles is different from what was originally thought?
b)The same monitoring of radio-collared lions over the years has suggested that the overall proportion of stalks that are the crouching type is about 0.87. Do the data above provide evidence that for this population of lions the proportion of crouching stalks of Thomson's and Grant's gazelles is less than what was originally thought?

Unlock Deck
Unlock for access to all 51 flashcards in this deck.
Unlock Deck
k this deck


