Deck 14: Chi-Square Applications for Nominal Data

Question 1

i. Chi-square goodness-of-fit test is the appropriate statistical test to use when you wish to determine how well an observed set of data fits an expected set of data
Ii) The null hypothesis in the goodness-of-fit test is that there is no difference.
Iii) The alternative hypothesis states that there is a difference between the observed frequencies and the expected frequencies in a goodness-of-fit test.

A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Answer 1

A

Question 2

i. The null hypothesis in the goodness-of-fit test is that there is no difference ii. The alternative hypothesis states that there is a difference between the observed frequencies and the expected frequencies in a goodness-of-fit test.
Iii) The number of degrees of freedom appropriate for the chi-square goodness-of-fit test is the number of categories minus 1.

A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Answer 2

A

Question 3

i. If the computed value of chi-square is less than the critical value, reject the null hypothesis at a predetermined level of significance.
Ii) If there are only two cells in a goodness-of-fit test, we should expect 5 or more frequencies.
Iii) Chi-square goodness-of-fit test is the appropriate statistical test to use when you wish to determine how well an observed set of data fits an expected set of data.

A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Answer 3

D

Question 4

i. If there are only two cells in a goodness-of-fit test, we should expect 5 or more frequencies.
ii. Chi-square goodness-of-fit test is the appropriate statistical test to use when you wish to determine how well an observed set of data fits an expected set of data
Iii) The null hypothesis in the goodness-of-fit test is that there is no difference.

A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Question 5

i. The alternative hypothesis states that there is a difference between the observed frequencies and the expected frequencies in a goodness-of-fit test.
Ii) The number of degrees of freedom appropriate for the chi-square goodness-of-fit test is the number of categories minus 1.
Iii) If there are extremely large differences between observed and expected frequencies the correct decision is to reject H0.

A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Question 6

i. For a goodness-of-fit test, the number of degrees of freedom is determined by k - 2, where k is the number of categories.
Ii) The sum of the expected frequencies in a goodness-of-fit test need not equal the sum of the observed frequencies.
Iii) A goodness-of-fit test is a nonparametric test involving a set of observed frequencies and a corresponding set of expected frequencies.

A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) (iii) is a correct statement, but (i) and (ii) are false.

Question 7

i. The chi-square goodness-of-fit test can be applied if there are equal or unequal expected frequencies.
Ii) For a goodness-of-fit test, the number of degrees of freedom is determined by k - 2, where k is the number of categories
Iii) The sum of the expected frequencies in a goodness-of-fit test need not equal the sum of the observed frequencies.

A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) (i) is a correct statement, but (ii) and (iii) are false.

Question 8

i. The sum of the expected frequencies and the sum of the observed frequencies must be equal.
ii. If the computed value of chi-square is less than the critical value, reject the null hypothesis at a predetermined level of significance.
Iii) If there are only two cells in a goodness-of-fit test, we should expect 5 or more frequencies.

A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Question 9

i. The chi-square goodness-of-fit test can be applied if there are equal or unequal expected frequencies.
Ii) For a goodness-of-fit test, the following are possible null and alternate hypotheses. Null: Sales are uniformly distributed among the five locations. Alternate: Sales are not uniformly distributed among the five locations.
Iii) The number of degrees of freedom appropriate for the chi-square goodness-of-fit test is the number of categories minus 1.

A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Question 10

i. For a goodness-of-fit test, the following are possible null and alternate hypotheses. Null: Sales are uniformly distributed among the five locations. Alternate: Sales are not uniformly distributed among the five locations.
Ii) The number of degrees of freedom appropriate for the chi-square goodness-of-fit test is the number of categories minus 1.
Iii) If there are extremely large differences between observed and expected frequencies the correct decision is to accept H0.

A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Question 11

i. For a goodness-of-fit test, the following are possible null and alternate hypotheses. Null: Sales are uniformly distributed among the five locations. Alternate: Sales are not uniformly distributed among the five locations.
Ii) In the goodness-of-fit test, the chi-square distribution is used to determine how well an observed set of observations "fits" an "expected" set of observations.
Iii) The sum of the expected frequencies and the sum of the observed frequencies must be equal.

A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Question 12

i. For a goodness-of-fit test, the number of degrees of freedom is determined by k - 2, where k is the number of categories.
ii. The sum of the expected frequencies in a goodness-of-fit test need not equal the sum of the observed frequencies.
Iii) If the computed value of chi-square is less than the critical value, reject the null hypothesis at a predetermined level of significance.

A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Question 13

i. The number of degrees of freedom appropriate for the chi-square goodness-of-fit test is the number of categories minus 2.
Ii) If there are extremely large differences between observed and expected frequencies the correct decision is to reject H0.
Iii) There are 2 degrees of freedom for a contingency table classifying three levels of income with each gender.

A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Question 14

i. The sum of the expected frequencies in a goodness-of-fit test need not equal the sum of the observed frequencies.
ii. A goodness-of-fit test is a nonparametric test involving a set of observed frequencies and a corresponding set of expected frequencies.
Iii) For a goodness-of-fit test, the following are possible null and alternate hypotheses; Null: Sales are uniformly distributed among the five locations.; Alternate: Sales are not uniformly distributed among the five locations.

A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Question 15

i. The chi-square goodness-of-fit test is appropriate for nominal and ordinal levels of data.
ii. chi-square test statistic used in a goodness-of-fit test has k - 1 degrees of freedom.
iii. The chi-square goodness-of-fit test can be applied if there are equal or unequal expected frequencies.

A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Question 16

i. chi-square test statistic used in a goodness-of-fit test has k - 2 degrees of freedom.

Question 17

i. If the computed value of chi-square is less than the critical value, reject the null hypothesis at a predetermined level of significance.
Ii) The alternative hypothesis states that there is a difference between the observed frequencies and the expected frequencies in a goodness-of-fit test.
Iii) There are 2 degrees of freedom for a contingency table classifying three levels of income with each gender.

A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Question 18

i. A goodness-of-fit test is a nonparametric test involving a set of observed frequencies and a corresponding set of expected frequencies.
Ii) For a goodness-of-fit test, the following are possible null and alternate hypotheses: Null: Sales are uniformly distributed among the five locations; Alternate: Sales are not uniformly distributed among the five locations.
Iii) In the goodness-of-fit test, the chi-square distribution is used to determine how well an observed set of observations "fits" an "expected" set of observations.

A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Question 19

i. In the goodness-of-fit test, the chi-square distribution is used to determine how well an observed set of observations "fits" an "expected" set of observations.
Ii) The sum of the expected frequencies and the sum of the observed frequencies must be equal.
Iii) If the computed value of chi-square is less than the critical value, reject the null hypothesis at a predetermined level of significance.

A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Question 20

i. chi-square test statistic used in a goodness-of-fit test has k - 1 degrees of freedom.T2 ii. The chi-square goodness-of-fit test can be applied if there are equal or unequal expected frequencies.T2
Iii) For a goodness-of-fit test, the number of degrees of freedom is determined by k - 2, where k is the number of categories.

A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Question 21

When determining how well an observed set of frequencies fit an expected set of frequencies the test is the:

A) F test.
B) t test.
C) goodness-of-fit test
D) test for association.
E) None of these statements are correct.

Question 22

A student asked a statistics professor if grades were marked "on the curve." The professor decided to give the student a project to determine if last year's statistics grades were normally distributed. The professor told the student that last year's mean mark was 70 with a standard deviation of 10 and to use the following results. 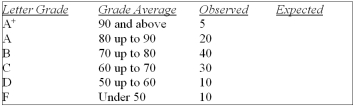What is the expected number of A's?

A) 18.25
B) 20.00
C) 13.59
D) 15.87
E) 15.63

Question 23

A student asked a statistics professor if grades were marked "on the curve." The professor decided to give the student a project to determine if last year's statistics grades were normally distributed. The professor told the student that last year's mean mark was 70 with a standard deviation of 10 and to use the following results. 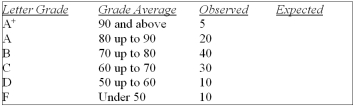What is the calculated value of chi-square?

A) 29.76
B) 14.20
C) 14.88
D) 28.39
E) 12.59

Question 24

Canadian Accounting classifies accounts receivable as "current", "late", and "not collectible". Industry figures show that 60% of A/R are current, 30% are late, and 10% are uncollectible. A law firm in Markham Ontario has 500 accounts receivable: 320 are current, 120 are late and 60 are not collectible. Are these numbers in agreement with the industry distribution?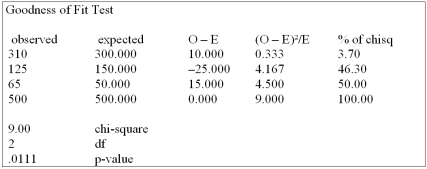

A) The Markham firm's data reflect the national average, when tested at the 0.10 level of significance
B) The Markham firm's data reflect the national average, when tested at the 0.05 level of significance
C) The Markham firm's data reflect the national average, when tested at the 0.01 level of significance
D) The Markham firm's data do not reflect the national average, when tested above a 0.01 level of significance
E) A, B and C are true

Question 25

A student asked a statistics professor if grades were marked "on the curve." The professor decided to give the student a project to determine if last year's statistics grades were normally distributed. The professor told the student that last year's mean mark was 70 with a standard deviation of 10 and to use the following results. 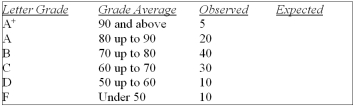What is your decision if α = 0.05?

A) The letter grades are evenly distributed.
B) The letter grades are not evenly distributed.
C) The letter grades are normally distributed.
D) The letter grades are not normally distributed.
E) The letter grades are unfairly distributed.

Question 26

In the chi-square test, the null hypothesis (no difference between sets of observed and expected frequencies) is rejected when the:

A) computed chi-square is less than the critical value.
B) difference between the observed and expected frequencies is significant.
C) difference between the observed and expected frequencies is small.
D) difference between the observed and expected frequencies occurs by chance.
E) None of these statements are correct.

Question 27

Canadian Accounting classifies accounts receivable as "current", "late", and "not collectible". Industry figures show that 60% of A/R are current, 30% are late, and 10% are uncollectible. A law firm in Markham Ontario has 500 accounts receivable: 320 are current, 120 are late and 60 are not collectible. Are these numbers in agreement with the industry distribution?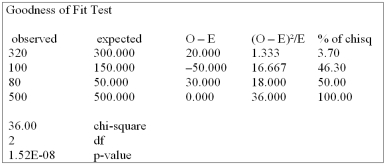

A) The Markham firm's data reflect the national average, when tested at the 0.10 level of significance
B) The Markham firm's data reflect the national average, when tested at the 0.05 level of significance
C) The Markham firm's data reflect the national average, when tested at the 0.01 level of significance
D) The Markham firm's data do not reflect the national average, when tested above a 0.01 level of significance
E) A, B and C are true

Question 28

A student asked a statistics professor if grades were marked "on the curve." The professor decided to give the student a project to determine if last year's statistics grades were normally distributed. The professor told the student that last year's mean mark was 70 with a standard deviation of 10 and to use the following results. 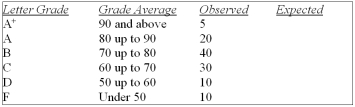What is the alternative hypothesis?

A) The letter grades are evenly distributed.
B) The letter grades are not evenly distributed.
C) The letter grades are normally distributed.
D) The letter grades are not normally distributed.
E) The letter grades are unfairly distributed.

Question 29

Three new colors have been proposed for the Jeep Grand Cherokee vehicle. They are silvered-blue, almond, and willow green. The null hypothesis for a goodness-of-fit test would be

A) willow green preferred over the other colors.
B) no preference between the colors.
C) any one color preferred over the other colors.
D) impossible to determine.
E) None of these statements are correct.

Question 30

What is our decision regarding the differences between the observed and expected frequencies if the critical value of chi-square is 9.488 and the computed value is 6.079?

A) Due to chance; do not reject the null hypothesis
B) Not due to chance; reject the null hypothesis
C) Not due to chance; do not reject the alternate hypothesis
D) Too close; reserve judgment
E) None of these statements are correct

Question 31

What is the critical value at the 0.05 level of significance for a goodness-of-fit test if there are six categories?

A) 3.841
B) 5.991
C) 7.815
D) 11.070
E) None of these statements are correct

Question 32

A student asked a statistics professor if grades were marked "on the curve." The professor decided to give the student a project to determine if last year's statistics grades were normally distributed. The professor told the student that last year's mean mark was 70 with a standard deviation of 10 and to use the following results.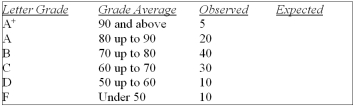
What is your decision if ? = 0.05?

A) The letter grades are evenly distributed.
B) The letter grades are not evenly distributed.
C) The letter grades are normally distributed.
D) The letter grades are not normally distributed.
E) The letter grades are unfairly distributed.

Question 33

A student asked a statistics professor if grades were marked "on the curve." The professor decided to give the student a project to determine if last year's statistics grades were normally distributed. The professor told the student that last year's mean mark was 70 with a standard deviation of 10 and to use the following results. What is the critical value of chi-square at the .05 level?

A) 12.833
B) 11.071
C) 12.592
D) 14.449
E) 19.675

Question 34

A question has these possible choices-excellent, very good, good, fair and unsatisfactory. How many degrees of freedom are there, using the goodness-of-fit test to the sample results?

A) 0
B) 2
C) 4
D) 5
E) None of these statements are correct

Question 35

A student asked a statistics professor if grades were marked "on the curve." The professor decided to give the student a project to determine if last year's statistics grades were normally distributed. The professor told the student that last year's mean mark was 70 with a standard deviation of 10 and to use the following results.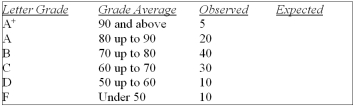
Using the data from this Megastat printout, you determine:

A) The letter grades are evenly distributed when tested at the 0.05 level of significance
B) The letter grades are not normally distributed when tested at the 0.01 level of significance
C) The letter grades are normally distributed when tested at the 0.05 level of significance
D) The letter grades are not normally distributed when tested at the 0.05 level of significance
E) B & D are true

Question 36

The computed chi-square value is positive because the difference between the observed and expected frequencies is:

A) squared.
B) linear.
C) uniform.
D) always positive.
E) None of these statements are correct.

Question 37

A distributor of personal computers has five locations in the city. The sales in units for the first quarter of the year were as follows:
What is the critical value at the 0.01 level of risk?

A) 7.779
B) 15.033
C) 13.277
D) 5.412
E) None of these statements are correct

Question 38

Canadian Accounting classifies accounts receivable as "current", "late", and "not collectible". Industry figures show that 60% of A/R are current, 30% are late, and 10% are uncollectible. A law firm in Markham Ontario has 500 accounts receivable: 320 are current, 120 are late and 60 are not collectible. Are these numbers in agreement with the industry distribution?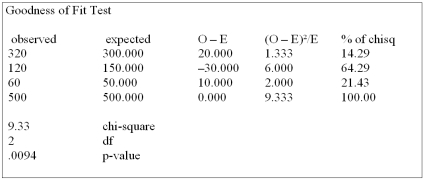
Using the data from this Megastat printout, you determine:

A) The Markham firm's data reflect the national average, when tested at the 0.10 level of significance
B) The Markham firm's data reflect the national average, when tested at the 0.05 level of significance
C) The Markham firm's data reflect the national average, when tested at the 0.01 level of significance
D) The Markham firm's data do not reflect the national average, when tested above a 0.01 level of significance
E) A, B and C are true

Question 39

A student asked a statistics professor if grades were marked "on the curve." The professor decided to give the student a project to determine if last year's statistics grades were normally distributed. The professor told the student that last year's mean mark was 70 with a standard deviation of 10 and to use the following results. 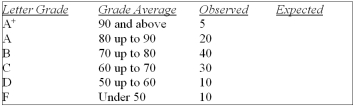What is the expected number of F's?

A) 10.00
B) 2.28
C) 2.62
D) 13.59
E) 4.56

Question 40

A student asked a statistics professor if grades were marked "on the curve." The professor decided to give the student a project to determine if last year's statistics grades were normally distributed. The professor told the student that last year's mean mark was 70 with a standard deviation of 10 and to use the following results. 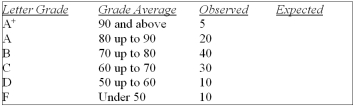What is the expected number of C's?

A) 39.25
B) 34.13
C) 13.59
D) 30.00
E) 15.87

Question 41

Which of the following are correct statements regarding the goodness-of-fit test?

A) Data may be of nominal scale
B) Population must be normal
C) All the expected frequencies must be equal
D) All of the above are true
E) None of these statements are correct are true

Question 42

i. There is not one, but a family of chi-square distributions. There is a chi-square distribution for 1 degree of freedom, another for 2 degrees of freedom, another for 3 degrees of freedom, and so on.
ii. The shape of the chi-square distribution depends on the size of the sample.
Iii) Small differences between observed and expected frequencies are due to chance.

A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Question 43

The chi-square distribution is:

A) positively skewed.
B) negatively skewed.
C) normally distributed.
D) negatively or positively skewed.
E) None of these statements are correct.

Question 44

i. Nonparametric tests of hypotheses, which are also called distribution free tests, require the population to be normally distributed.
Ii) The shape of the chi-square distribution changes for each number of degrees of freedom.
iii. The chi-square distribution is a positively skewed distribution.

A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Question 45

i. Nonparametric tests of hypotheses, which are also called distribution free tests, require the population to be normally distributed.
Ii) The computed value of chi-square is always positive because the difference between the observed frequencies and the expected frequencies are squared.
Iii) The shape of the chi-square distribution changes for each number of degrees of freedom.

A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Question 46

i. Nonparametric tests require no assumptions about the shape of the population distribution.
ii. There is not one, but a family of chi-square distributions. There is a chi-square distribution for 1 degree of freedom, another for 2 degrees of freedom, another for 3 degrees of freedom, and so on.
iii. The chi-square distribution with large degrees of freedom approaches a normal distribution.

A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Question 47

To find the expected frequency in a contingency table:

A) Take the square root of the degrees of freedom.
B) Multiply the row and column totals and divide by the grand total.
C) Use the total number of observations minus one.
D) None of the above.

Question 48

i. The computed value of chi-square is always positive because the difference between the observed frequencies and the expected frequencies are squared.
ii. The shape of the chi-square distribution changes for each number of degrees of freedom.
iii. The minimum computed value of chi-square is zero.

A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Question 49

i. Nonparametric tests require no assumptions about the shape of the population distribution.
ii. Tests of hypotheses for nominal or ordinal levels of measurement are called nonparametric or distribution-free tests.
Iii) There is not one, but a family of chi-square distributions. There is a chi-square distribution for 1 degree of freedom, another for 2 degrees of freedom, another for 3 degrees of freedom, and so on.

A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Question 50

i. Small differences between observed and expected frequencies are due to chance.
ii. The chi-square distribution with large degrees of freedom approaches a normal distribution.
iii. The chi-square distribution is positively skewed.

A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Question 51

i. The shape of the chi-square distribution changes for each number of degrees of freedom.
ii. The minimum computed value of chi-square is zero.
Iii) The chi-square distribution is a positively skewed distribution.

A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Question 52

Suppose that we wish to test the null hypothesis that for 3 cells, A, B, and C, the cell categories are equal. We observed 8 data in cell A, 13 in cell B, and 9 in cell C. What is the decision rule using the
0.05 significance level?

A) 7.815
B) 5.991
C) 43.773
D) 42.557
E) None of these choices are correct.

Question 53

The chi-square distribution can assume:

A) only positive values.
B) only negative values.
C) negative and positive values or zero.
D) only zero.
E) None of these statements are correct.

Question 54

Two chi-square distributions were plotted on the same chart. One distribution was for 3 degrees of freedom and the other was for 12 degrees of freedom. Which distribution would tend to approach a normal distribution?

A) 3 degrees
B) 12 degrees
C) 15 degrees
D) All would
E) None of these statements are correct

Question 55

Which of the following assumptions is necessary to apply a nonparametric test of hypothesis using chi-square?

A) Normal population is required
B) Interval scale of measurement is required
C) Population variance must be known
D) Both "a" and "c"
E) None of these statements are correct

Question 56

i. Tests of hypotheses for nominal or ordinal levels of measurement are called nonparametric or distribution-free tests.
Ii) There is not one, but a family of chi-square distributions. There is a chi-square distribution for 1 degree of freedom, another for 2 degrees of freedom, another for 3 degrees of freedom, and so on.
iii. The shape of the chi-square distribution depends on the size of the sample.

A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Question 57

i. The chi-square distribution with large degrees of freedom approaches a normal distribution.
ii. The chi-square distribution is positively skewed.
Iii) Nonparametric tests of hypotheses, which are also called distribution free tests, require the population to be normally distributed.

A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Question 58

The chi-square distribution becomes more symmetrical as:

A) number of variables increase.
B) the chi-square value increases.
C) degrees of freedom decrease.
D) degrees of freedom increase.
E) None of these statements are correct.

Question 59

What is our decision for a goodness-of-fit test with a computed value of chi-square of 1.273 and a critical value of 13.388?

A) Do not reject the null hypothesis
B) Reject the null hypothesis
C) Unable to reject or not reject the null hypothesis based on data
D) Should take a larger sample
E) None of these statements are correct

Question 60

Suppose that we wish to test the null hypothesis that for 3 cells, A, B, and C, the cell categories are equal. We observed 8 data in cell A, 13 in cell B, and 9 in cell

A) Do not reject the null hypothesis
B) Reject the null hypothesis.
C) There is not enough information to reach a decision.
C) What is the decision regarding the null hypothesis?
D) None of the above.

Question 61

The chi-square has:

A) one distribution.
B) two distributions.
C) a family of distribution.
D) a uniform distribution.
E) None of these statements are correct.

Question 62

Six people have declared their intentions to run for a trustee seat in the next local election. A political poll is conducted during the campaign among 1,020 voters to determine if there is any clear preference among the voters. The responses are shown below. If the computed chi-square is 30, what is your decision at the 1% level of significance? What is your decision at the 5% level of significance?

A) Reject H0; preferences among the candidates exist at the 1% level of significance; also reject at the 5% level of significance.
B) Reject H0 at the 1% level of significance but not at the 5% level of significance.
C) Reject H0 at the 5% level of significance but not at the 1% level of significance.
D) Accept Ho at both the 5% and 1% levels of significance; no preferences among the candidates exist.

Question 63

Six people have declared their intentions to run for a trustee seat in the next local election. A political poll is conducted during the campaign among 1,020 voters to determine if there is any clear preference among the voters. The responses are shown below. What is the critical value at the 5% level of significance?

A) 11.070
B) 3.841
C) 5.991
D) 9.236
E) 9.438

Question 64

The personnel manager is concerned about absenteeism. She decides to sample the records to determine if absenteeism is distributed evenly throughout the six-day workweek. The null hypothesis to be tested is: Absenteeism is distributed evenly throughout the week. The 0.01 level is to be used. The sample results are: What is the calculated value of chi-square?

A) 1.0
B) 0.5
C) 0.8
D) 8.0
E) None of these statements are correct

Question 65

Six people have declared their intentions to run for a trustee seat in the next local election. A political poll is conducted during the campaign among 1,020 voters to determine if there is any clear preference among the voters. The responses are shown below. Determine the null and alternate hypotheses.

A) Ho: No preference among candidates exists H1: Preference among candidates
B) Ho: Preference among candidates exists H1: No preference among candidates exists
C) We are unable to determine because there are too many candidates.
D) We need more information to determine the null and alternate hypotheses.

Question 66

A school is trying to determine if there is a relationship between gender and which of its 3 programs that students enroll in. It was observed that 20 females enrolled in accounting, 15 in finance and 25 in marketing. For males, 10 enrolled in accounting, 20 enrolled in finance and 15 enrolled in marketing. What is the chi square value?

A) 4.497
B) 5
C) 3.25
D) 4.25
E) None of these choices is correct.

Question 67

i. The claim that "male and female University of Toledo students prefer different parking lots on campus" is an example of a chi-square null hypothesis.
Ii) For contingency table analysis using the chi-square test, multiplying the number of rows minus 1 by the number of columns minus 1 will give you the degrees of freedom.
Iii) For a contingency table, the expected frequency for a cell is found by dividing the row total by the grand total.

A) (i), (ii) and (iii) are all correct statements
B) (ii) is a correct statement, but not (i) or (iii).
C) (iii) is a correct statement but not (i) or (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Question 68

In a chi-square goodness-of-fit-test, the larger the difference between the set of expected frequencies and the set of observed frequencies:

A) The more likely we are to conclude that the observed distribution is similar to the expected distribution
B) The more likely it will be that we will not reject the null hypothesis
C) The more likely we will reject the null hypothesis.
D) The more likely we will be to not accept the alternative hypothesis.

Question 69

Six people have declared their intentions to run for a trustee seat in the next local election. A political poll is conducted during the campaign among 1,020 voters to determine if there is any clear preference among the voters. The responses are shown below. What is the critical value at the 1% level of significance?

A) 6.635
B) 9.210
C) 11.345
D) 13.277
E) 15.086

Question 70

The personnel manager is concerned about absenteeism. She decides to sample the records to determine if absenteeism is distributed evenly throughout the six-day workweek. The null hypothesis to be tested is: Absenteeism is distributed evenly throughout the week. The 0.01 level is to be used. The sample results are: How many degrees of freedom are there?

A) 0
B) 3
C) 4
D) 5
E) None of these statements are correct

Question 71

i. For a contingency table, the expected frequency for a cell is found by dividing the row total by the grand total.
Ii) The claim that "male and female University of Toledo students prefer different parking lots on campus" is an example of a chi-square null hypothesis.
Iii) For contingency table analysis using the chi-square test, multiplying the number of rows minus 1 by the number of columns minus 1 will give you the degrees of freedom.

A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (iii) is a correct statement but not (i) or (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Question 72

How many degrees of freedom do we use for the chi square goodness of fit test?

A) k, where k represents the number of classes/categories.
B) k - 1, where k represents the number of classes/categories
C) k - 1, where k represents the number of data values
D) k, where k represents the number of data values.

Question 73

The personnel manager is concerned about absenteeism. She decides to sample the records to determine if absenteeism is distributed evenly throughout the six-day workweek. The null hypothesis to be tested is: Absenteeism is distributed evenly throughout the week. The 0.01 level is to be used. The sample results are: What is the expected frequency?

A) 9
B) 10
C) 11
D) 12
E) None of these statements are correct

Question 74

The personnel manager is concerned about absenteeism. She decides to sample the records to determine if absenteeism is distributed evenly throughout the six-day workweek. The null hypothesis to be tested is: Absenteeism is distributed evenly throughout the week. The 0.01 level is to be used. The sample results are: What kind of frequencies are the numbers 12, 9, 11, 10, and 9 called?

A) Acceptance
B) Critical value
C) Expected
D) Observed
E) None of these statements are correct

Question 75

Which of the following are correct statements regarding the chi-square distribution?

A) Distribution is negatively skewed
B) Chi-square is based on two sets of degrees of freedom, one for the numerator and one for the denominator
C) Its shape is based on the degrees of freedom
D) All of the above are true
E) None of these statements are correct are true

Question 76

i. The claim that "male and female University of Toledo students prefer different parking lots on campus" is an example of a chi-square null hypothesis.
Ii) For a contingency table, the expected frequency for a cell is found by dividing the row total by the grand total.
Iii) For contingency table analysis using the chi-square test, multiplying the number of rows minus 1 by the number of columns minus 1 will give you the degrees of freedom.

A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are false statements, only (iii) is correct.
C) (iii) is a correct statement but not (i) or (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Question 77

Six people have declared their intentions to run for a trustee seat in the next local election. A political poll is conducted during the campaign among 1,020 voters to determine if there is any clear preference among the voters. The responses are shown below. How many degrees of freedom are there?

A) 1
B) 2
C) 3
D) 4
E) 5

Question 78

A school is trying to determine if there is a relationship between gender and which of its 3 programs that students enrol. It was observed that 20 females enrolled in accounting, 15 in finance and 25 in marketing. For males, 10 enrolled in accounting, 20 enrolled in finance and 15 enrolled in marketing. Can we conclude that there is a relationship between gender and program? Use the 0.05 significance level.

A) There is no evidence of a relationship between gender and program of study.
B) There is evidence of a relationship between gender and program of study.
C) Not enough information is given to reach a conclusion.
D) None of the above.

Question 79

Which of the following is not a characteristic of the chi square distribution?

A) Its shape is based on the sample size.
B) It is not negative.
C) It is positively skewed.
D) It approaches a normal distribution as the degrees of freedom increase.

Question 80

Six people have declared their intentions to run for a trustee seat in the next local election. A political poll is conducted during the campaign among 1,020 voters to determine if there is any clear preference among the voters. The responses are shown below. What is the expected frequency for each candidate?

A) 204
B) 170
C) 510
D) 180
E) None of these statements are correct