Deck 12: Linear Regression and Correlation
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/150
Play
Full screen (f)
Deck 12: Linear Regression and Correlation
1
A sales manager for an advertising agency believes there is a relationship between the number of contacts and the amount of the sales. To verify this believe, the following data was collected: 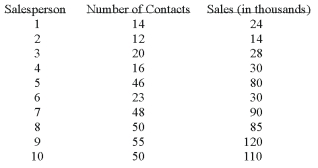
What is the dependent variable?
A) Salesperson
B) Number of contacts
C) Amount of sales
D) All the choices are correct
E) None of the choices are correct


What is the dependent variable?
A) Salesperson
B) Number of contacts
C) Amount of sales
D) All the choices are correct
E) None of the choices are correct
Amount of sales
2
In the regression equation, Y' = a + bX, what does the letter "a" represent?
A) Y intercept
B) Slope of the line
C) Any value of the independent variable that is selected
D) None of these statements are correct
A) Y intercept
B) Slope of the line
C) Any value of the independent variable that is selected
D) None of these statements are correct
A
3
i. If we are studying the relationship between high school performance and college performance, and want to predict college performance, high school performance is the independent variable.
ii. A financial advisor is interested in predicting bond yield based on bond term, i.e., one year, two years, etc. The dependent variable is bond yield.
Iii) The variable used to predict the value of another is called the independent variable.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false
ii. A financial advisor is interested in predicting bond yield based on bond term, i.e., one year, two years, etc. The dependent variable is bond yield.
Iii) The variable used to predict the value of another is called the independent variable.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false
(i), (ii) and (iii) are all correct statements
4
A scatter diagram is a chart
A) In which the dependent variable is scaled along the vertical axis.
B) In which the independent variable is scaled along the horizontal axis.
C) That portrays the relationship between two variables.
D) All of the above.
A) In which the dependent variable is scaled along the vertical axis.
B) In which the independent variable is scaled along the horizontal axis.
C) That portrays the relationship between two variables.
D) All of the above.

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
5
i. If we are studying the relationship between high school performance and college performance, and want to predict college performance, high school performance is the independent variable.
Ii) An economist is interested in predicting the unemployment rate based on gross domestic product. Since the economist is interested in predicting unemployment, the independent variable is gross domestic product.
Iii) The variable used to predict the value of another is called the dependent variable.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false
Ii) An economist is interested in predicting the unemployment rate based on gross domestic product. Since the economist is interested in predicting unemployment, the independent variable is gross domestic product.
Iii) The variable used to predict the value of another is called the dependent variable.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
6
Data is collected from 20 sales people in order to verify that the more contacts made with potential clients, the greater the sales volume. A scatter diagram of the collected information is shown below. 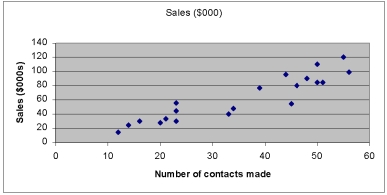 Looking at this scatter diagram you determine:
Looking at this scatter diagram you determine:
A) There is clearly no relationship between the number of sales contacts made and the sales earned.
B) There is a moderate but inverse relationship between the two variables
C) There is a moderate and direct relationship between the two variables
D) The Sales ($000s) is the independent variable
E) C & D are true
 Looking at this scatter diagram you determine:
Looking at this scatter diagram you determine:A) There is clearly no relationship between the number of sales contacts made and the sales earned.
B) There is a moderate but inverse relationship between the two variables
C) There is a moderate and direct relationship between the two variables
D) The Sales ($000s) is the independent variable
E) C & D are true

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
7
i. The least squares technique minimizes the sum of the squares of the vertical distances between the actual Y values and the predicted values of Y.
ii. When a regression line has a zero slope, indicating a lack of a relationship, the line is vertical to the x-axis.
Iii) In regression analysis, the predicted value of Y' rarely agrees exactly with the actual Y value, i.e., we expect some prediction error.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false
ii. When a regression line has a zero slope, indicating a lack of a relationship, the line is vertical to the x-axis.
Iii) In regression analysis, the predicted value of Y' rarely agrees exactly with the actual Y value, i.e., we expect some prediction error.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
8
i. The technique used to measure the strength of the relationship between two sets of variables using the coefficient of correlation and the coefficient of determination is called regression analysis.
ii. In order to visualize the form of the regression equation, we can draw a scatter diagram.
Iii) When a regression line has a zero slope, indicating a lack of a relationship, the line is horizontal to the x-axis.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false
ii. In order to visualize the form of the regression equation, we can draw a scatter diagram.
Iii) When a regression line has a zero slope, indicating a lack of a relationship, the line is horizontal to the x-axis.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
9
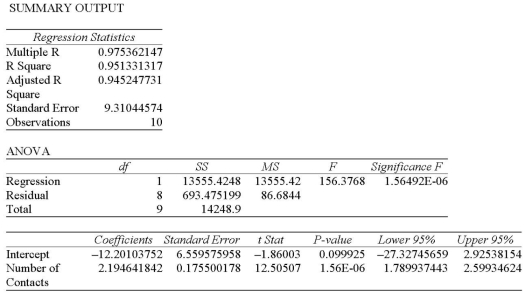
What is the independent variable?
A) Salesperson
B) Number of contacts
C) Amount of sales
D) All the choices are correct
E) None of the choices are correct

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
10
Given the scatter diagram below, that shows the number of workdays absent per year based on the age of the employees, which of the following statements are true? 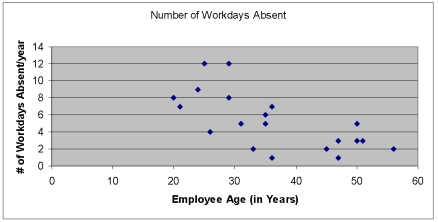
A) There is clearly no relationship whatsoever between an employee's age and the number of workday absences that they take.
B) There is a single but strong outlier in this data set.
C) There appears to be an inverse relationship between the two variables
D) A & B are true
E) B & C are true

A) There is clearly no relationship whatsoever between an employee's age and the number of workday absences that they take.
B) There is a single but strong outlier in this data set.
C) There appears to be an inverse relationship between the two variables
D) A & B are true
E) B & C are true

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
11
i. In order to visualize the form of the regression equation, we can draw a scatter diagram.
ii. The least squares technique minimizes the sum of the squares of the vertical distances between the actual Y values and the predicted values of Y.
Iii) In regression analysis, the predicted value of Y' rarely agrees exactly with the actual Y value, i.e., we expect some prediction error.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false
ii. The least squares technique minimizes the sum of the squares of the vertical distances between the actual Y values and the predicted values of Y.
Iii) In regression analysis, the predicted value of Y' rarely agrees exactly with the actual Y value, i.e., we expect some prediction error.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
12
Given the scatter diagram below, that shows the number of workdays absent per year based on the age of the employees, which of the following statements are true? 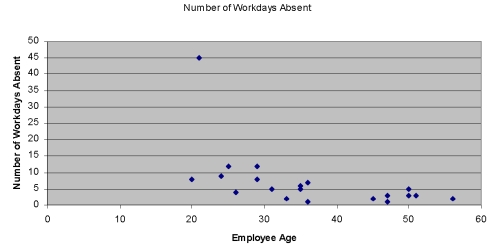
A) There is clearly no relationship whatsoever between an employee's age and the number of workday absences that they take.
B) There is a single but strong outlier in this data set.
C) In analyzing this data, you may wish to remove the one point that doesn't "fit" with all the others before continuing your analysis.
D) A & B are true
E) B & C are true

A) There is clearly no relationship whatsoever between an employee's age and the number of workday absences that they take.
B) There is a single but strong outlier in this data set.
C) In analyzing this data, you may wish to remove the one point that doesn't "fit" with all the others before continuing your analysis.
D) A & B are true
E) B & C are true

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
13
i. If we are studying the relationship between high school performance and college performance, and want to predict college performance, high school performance is the dependent variable.
Ii) A financial advisor is interested in predicting bond yield based on bond term, i.e., one year, two years, etc. The dependent variable is bond term.
Iii) The variable used to predict the value of another is called the dependent variable.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false
Ii) A financial advisor is interested in predicting bond yield based on bond term, i.e., one year, two years, etc. The dependent variable is bond term.
Iii) The variable used to predict the value of another is called the dependent variable.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
14
In the equation Y' = a + bX, what is Y'?
A) Slope of the line
B) Y intercept
C) Predicted value of Y, given a specific X value
D) Value of Y when X = 0
E) None of these statements are correct
A) Slope of the line
B) Y intercept
C) Predicted value of Y, given a specific X value
D) Value of Y when X = 0
E) None of these statements are correct

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
15
Suppose the least squares regression equation is Y' = 1202 + 1,133X. When X = 3, what does Y' equal?
A) 5,734
B) 8,000
C) 4,601
D) 4,050
E) None of these statements are correct
A) 5,734
B) 8,000
C) 4,601
D) 4,050
E) None of these statements are correct

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
16
i. In order to visualize the form of the regression equation, we can draw a scatter diagram.
ii. When a regression line has a zero slope, indicating a lack of a relationship, the line is vertical to the x-axis.
Iii) In regression analysis, the predicted value of Y' rarely agrees exactly with the actual Y value, i.e., we expect some prediction error.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false
ii. When a regression line has a zero slope, indicating a lack of a relationship, the line is vertical to the x-axis.
Iii) In regression analysis, the predicted value of Y' rarely agrees exactly with the actual Y value, i.e., we expect some prediction error.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
17
i. A scatter diagram is a chart that portrays the relationship between two variables.
ii. If a scatter diagram shows very little scatter about a straight line drawn through the plots, it indicates a rather weak relationship.
Iii) A scatter diagram may be put together using excel or megastat.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false
ii. If a scatter diagram shows very little scatter about a straight line drawn through the plots, it indicates a rather weak relationship.
Iii) A scatter diagram may be put together using excel or megastat.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
18
What is the variable used to predict the value of another called?
A) Independent
B) Dependent
C) Correlation
D) Determination
E) None of these statements are correct
A) Independent
B) Dependent
C) Correlation
D) Determination
E) None of these statements are correct

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
19
In the regression equation, Y' = a + bX, what does the letter "b" represent?
A) Y intercept
B) Slope of the line
C) Any value of the independent variable that is selected
D) Value of Y when X = 0
E) None of these statements are correct
A) Y intercept
B) Slope of the line
C) Any value of the independent variable that is selected
D) Value of Y when X = 0
E) None of these statements are correct

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
20
What is the chart called when the paired data (the dependent and independent variables) are plotted?
A) Scatter diagram
B) Bar
C) Pie
D) Linear regression
E) None of these statements are correct
A) Scatter diagram
B) Bar
C) Pie
D) Linear regression
E) None of these statements are correct

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
21
Data is collected from 20 sales people in order to verify that the more contacts made with potential clients, the greater the sales volume. The Excel printout is shown below. 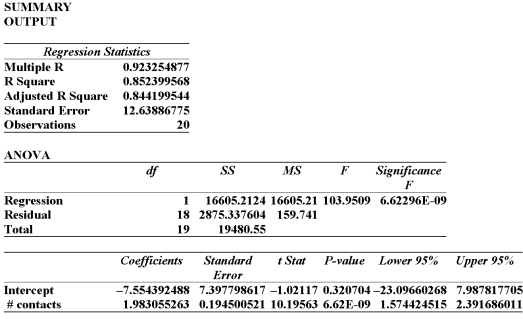
This model predicts that with 25 sales contacts, sales will be:
A) $49 576
B) $42 022
C) $190 843
D) $19 429
E) $16 605

This model predicts that with 25 sales contacts, sales will be:
A) $49 576
B) $42 022
C) $190 843
D) $19 429
E) $16 605

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
22
Information was collected from employee records to determine whether there is an association between an employee's age and the number or workdays they miss. Excel results are summarized below: 
From this printout you determine:
A) The employee age is the dependent variable
B) The employee age is the independent variable
C) The older the employee the more days they are absent from work
D) The intercept of 23 indicates the most days absent
E) B & C are true

From this printout you determine:
A) The employee age is the dependent variable
B) The employee age is the independent variable
C) The older the employee the more days they are absent from work
D) The intercept of 23 indicates the most days absent
E) B & C are true

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
23
The partial megastat output below is regression analysis of the relationship between annual payroll and number of wins in a season for 28 teams in professional sports. The purpose of the analysis
Is to predict the number of wins when given an annual payroll in $millions. Although technically not a sample, the baseball data below will be treated as a convenience sample of all major league professional sports.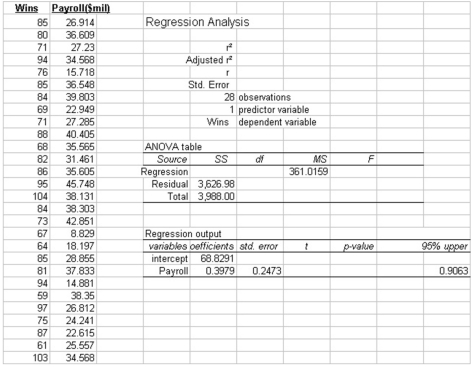
Refer to the printout above. Predict the annual attendance (000) for a team with 100 wins
A) 2,820.49
B) 3,222.61
C) 2,903.01
D) 3,695.06
E) 8,279.78
Is to predict the number of wins when given an annual payroll in $millions. Although technically not a sample, the baseball data below will be treated as a convenience sample of all major league professional sports.

Refer to the printout above. Predict the annual attendance (000) for a team with 100 wins
A) 2,820.49
B) 3,222.61
C) 2,903.01
D) 3,695.06
E) 8,279.78

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
24
Given the following five points: (-2,0), (-1,0), (0,1), (1,1), and (2,3). What is the Y intercept?
A) 0.0
B) 0.7
C) 1.0
D) 1.5
E) None of the choices are correct
A) 0.0
B) 0.7
C) 1.0
D) 1.5
E) None of the choices are correct

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
25
Information was collected from employee records to determine whether there is an association between an employee's age and the number or workdays they miss. Excel results are summarized below: 
From this printout you determine:
A) When tested at the 2% level of significance, there is no relationship between an employee's age and the number of days of work absences
B) For each additional year of age, we can expect the number of days of absence to increase by 0.45 days
C) Almost 53% of the variation in the number of absent days can be explained by the variation in the employees ages
D) A & B are true
E) A & C are true

From this printout you determine:
A) When tested at the 2% level of significance, there is no relationship between an employee's age and the number of days of work absences
B) For each additional year of age, we can expect the number of days of absence to increase by 0.45 days
C) Almost 53% of the variation in the number of absent days can be explained by the variation in the employees ages
D) A & B are true
E) A & C are true

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
26
Information was collected from employee records to determine whether there is an association between an employee's age and the number or workdays they miss. Excel results are summarized below: 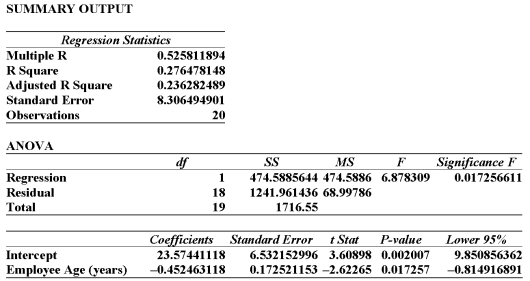
From this printout you determine:
A) The employee age is the dependent variable
B) The employee age is the independent variable
C) The regression equation is Y = 23.57 - 0.45x
D) The regression equation is Y = 23.57 x -0.45
E) B & C are true

From this printout you determine:
A) The employee age is the dependent variable
B) The employee age is the independent variable
C) The regression equation is Y = 23.57 - 0.45x
D) The regression equation is Y = 23.57 x -0.45
E) B & C are true

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
27
Assume the least squares equation is Y' = 10 + 20X. What does the value of 10 in the equation indicate?
A) Y intercept
B) For each unit increased in Y, X increases by 10
C) For each unit increased in X, Y increases by 10
D) None of these statements are correct
A) Y intercept
B) For each unit increased in Y, X increases by 10
C) For each unit increased in X, Y increases by 10
D) None of these statements are correct

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
28
In the least squares equation, Y' = 10 + 20X the value of 20 indicates
A) the Y intercept.
B) for each unit increased in X, Y increases by 20.
C) for each unit increased in Y, X increases by 20.
D) None of these statements are correct.
A) the Y intercept.
B) for each unit increased in X, Y increases by 20.
C) for each unit increased in Y, X increases by 20.
D) None of these statements are correct.

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
29
i. The technique used to measure the strength of the relationship between two sets of variables using the coefficient of correlation and the coefficient of determination is called regression analysis.
ii. In order to visualize the form of the regression equation, we can draw a scatter diagram.
Iii) The least squares technique minimizes the sum of the squares of the vertical distances between the actual Y values and the predicted values of Y.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false
ii. In order to visualize the form of the regression equation, we can draw a scatter diagram.
Iii) The least squares technique minimizes the sum of the squares of the vertical distances between the actual Y values and the predicted values of Y.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
30
Information was collected from employee records to determine whether there is an association between an employee's age and the number or workdays they miss. Excel results are summarized
Below:
From this printout you determine:
A) The y-intercept of 23 makes no sense
B) For each additional year of age, we can expect the number of days of absence to increase by 0.45 days
C) For each additional year of age, we can expect the number of days of absence to decrease by 0.45 days
D) A & B are true
E) A & C are true
Below:

From this printout you determine:
A) The y-intercept of 23 makes no sense
B) For each additional year of age, we can expect the number of days of absence to increase by 0.45 days
C) For each additional year of age, we can expect the number of days of absence to decrease by 0.45 days
D) A & B are true
E) A & C are true

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
31
i. The technique used to measure the strength of the relationship between two sets of variables using the coefficient of correlation and the coefficient of determination is called regression analysis.
ii. In order to visualize the form of the regression equation, we can draw a scatter diagram.
Iii) A regression equation may be determined using a mathematical method called the least squares principle.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false
ii. In order to visualize the form of the regression equation, we can draw a scatter diagram.
Iii) A regression equation may be determined using a mathematical method called the least squares principle.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
32
The partial megastat output below is regression analysis of the relationship between annual payroll and number of wins in a season for 28 teams in professional sports. The purpose of the analysis is to predict the number of wins when given an annual payroll in $millions. Although technically not a sample, the baseball data below will be treated as a convenience sample of all major league professional sports 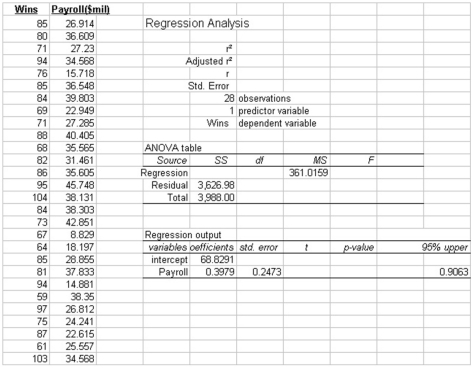
Refer to the printout above. The regression equation is:
A) = 2,049 + 68.8291x
B) = 82.5157 + 28.2049x
C) = 28.2049 + 7.5888x
D) = 82.5157 + 7.5888x
E) = 7.5888 + 28.2049x

Refer to the printout above. The regression equation is:
A) = 2,049 + 68.8291x
B) = 82.5157 + 28.2049x
C) = 28.2049 + 7.5888x
D) = 82.5157 + 7.5888x
E) = 7.5888 + 28.2049x

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
33
Information was collected from employee records to determine whether there is an association between an employee's age and the number or workdays they miss. Excel results are summarized below: 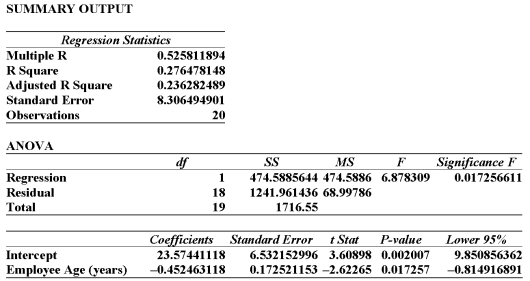
From this printout you determine:
A) The y-intercept of 23 makes no sense
B) The employee age is the independent variable
C) The regression equation is Y = 23.57 - 0.45x
D) B & C are true
E) All of the choices are true

From this printout you determine:
A) The y-intercept of 23 makes no sense
B) The employee age is the independent variable
C) The regression equation is Y = 23.57 - 0.45x
D) B & C are true
E) All of the choices are true

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
34
i. The technique used to measure the strength of the relationship between two sets of variables using the coefficient of correlation and the coefficient of determination is called regression analysis.
ii. In order to visualize the form of the regression equation, we can draw a scatter diagram.
Iii) The equation for a straight line going through the plots on a scatter diagram is called a regression
Equation. It is alternately called an estimating equation and a predicting equation.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false
ii. In order to visualize the form of the regression equation, we can draw a scatter diagram.
Iii) The equation for a straight line going through the plots on a scatter diagram is called a regression
Equation. It is alternately called an estimating equation and a predicting equation.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
35
Data is collected from 20 sales people in order to verify that the more contacts made with potential clients, the greater the sales volume. The Excel printout is shown below. 
From this printout you determine:
A) There is a very weak relationship between the # of contacts and the sales $
B) There is a very strong relationship between the # of contacts and the sales $
C) The regression equation is y = 1.98 x +7.55
D) The regression equation is y = -7.55 x +1.98
E) B & C are true

From this printout you determine:
A) There is a very weak relationship between the # of contacts and the sales $
B) There is a very strong relationship between the # of contacts and the sales $
C) The regression equation is y = 1.98 x +7.55
D) The regression equation is y = -7.55 x +1.98
E) B & C are true

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
36
i. In order to visualize the form of the regression equation, we can draw a scatter diagram.
ii. In regression analysis, the predicted value of Y' rarely agrees exactly with the actual Y value, i.e., we expect some prediction error.
Iii) The technique used to measure the strength of the relationship between two sets of variables using the coefficient of correlation and the coefficient of determination is called regression analysis.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false
ii. In regression analysis, the predicted value of Y' rarely agrees exactly with the actual Y value, i.e., we expect some prediction error.
Iii) The technique used to measure the strength of the relationship between two sets of variables using the coefficient of correlation and the coefficient of determination is called regression analysis.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
37
Information was collected from employee records to determine whether there is an association between an employee's age and the number or workdays they miss. Excel results are summarized below: 
From this printout you determine:
A) The employee age is the dependent variable
B) The employee age is the independent variable
C) The regression equation is Y = 23.57 - 0.45x
D) The regression equation is Y = 23.57 x -0.45
E) B & C are true

From this printout you determine:
A) The employee age is the dependent variable
B) The employee age is the independent variable
C) The regression equation is Y = 23.57 - 0.45x
D) The regression equation is Y = 23.57 x -0.45
E) B & C are true

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
38
i. The technique used to measure the strength of the relationship between two sets of variables using the coefficient of correlation and the coefficient of determination is called regression analysis.
ii. In order to visualize the form of the regression equation, we can draw a scatter diagram.
Iii) A line found using the least squares principle is the best-fitting line because the sum of the squares of the vertical deviations between the actual and estimated values is minimized.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false
ii. In order to visualize the form of the regression equation, we can draw a scatter diagram.
Iii) A line found using the least squares principle is the best-fitting line because the sum of the squares of the vertical deviations between the actual and estimated values is minimized.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
39
Given the following five points: (-2,0), (-1,0), (0,1), (1,1), and (2,3). What is the slope of the line?
A) 0.0
B) 0.5
C) 0.6
D) 0.7
E) None of the choices are correct
A) 0.0
B) 0.5
C) 0.6
D) 0.7
E) None of the choices are correct

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
40
i. The technique used to measure the strength of the relationship between two sets of variables using the coefficient of correlation and the coefficient of determination is called regression analysis.
ii. In order to visualize the form of the regression equation, we can draw a scatter diagram.
Iii) When a regression line has a zero slope, indicating a lack of a relationship, the line is horizontal to the x-axis.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false
ii. In order to visualize the form of the regression equation, we can draw a scatter diagram.
Iii) When a regression line has a zero slope, indicating a lack of a relationship, the line is horizontal to the x-axis.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
41
The partial megastat output below is regression analysis of the relationship between annual payroll and number of wins in a season for 28 teams in professional sports. The purpose of the analysis is to predict the number of wins when given an annual payroll in $millions. Although technically not a sample, the baseball data below will be treated as a convenience sample of all major league professional sports. 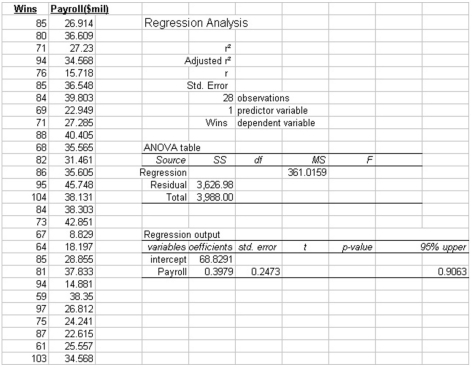
Refer to the printout above. Predict the number of wins for a team with PAYROLL = 25(million) (nearest whole number)
A) 10
B) 69
C) 79
D) 74
E) 64

Refer to the printout above. Predict the number of wins for a team with PAYROLL = 25(million) (nearest whole number)
A) 10
B) 69
C) 79
D) 74
E) 64

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
42
i. A coefficient of correlation r close to 0 (say, 0.08) shows that the relationship between two variables is quite weak.
ii. Coefficients of -0.91 and +0.91 have equal strength.
Iii) If the coefficient of correlation is 0.68, the coefficient of determination is 0.4624.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false
ii. Coefficients of -0.91 and +0.91 have equal strength.
Iii) If the coefficient of correlation is 0.68, the coefficient of determination is 0.4624.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
43
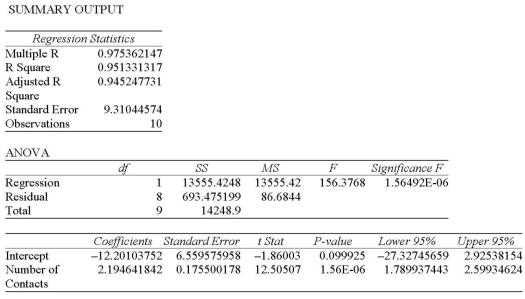
A sales manager for an advertising agency believes there is a relationship between the number of contacts and the amount of the sales. To verify this believe, the following data was collected: 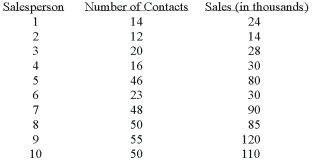
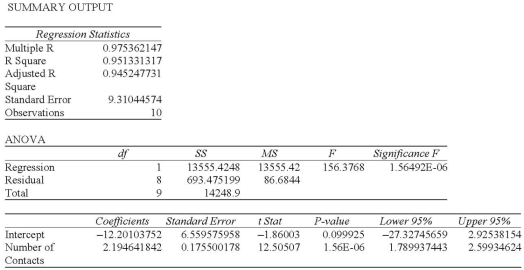
The slope in this instance indicates:
A) For each additional contact made, the salesperson can anticipate an additional $2195 in sales
B) For each additional contact made, the salesperson can anticipate an additional $2.19 in sales
C) For each additional contact made, the salesperson can anticipate an additional $12,201 in sales
D) For each additional contact made, the salesperson can anticipate a drop of $12,201 in sales
E) For each additional sale made, the salesperson can anticipate an additional 2 contacts are needed


The slope in this instance indicates:
A) For each additional contact made, the salesperson can anticipate an additional $2195 in sales
B) For each additional contact made, the salesperson can anticipate an additional $2.19 in sales
C) For each additional contact made, the salesperson can anticipate an additional $12,201 in sales
D) For each additional contact made, the salesperson can anticipate a drop of $12,201 in sales
E) For each additional sale made, the salesperson can anticipate an additional 2 contacts are needed

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
44
i. The strength of the correlation between two variables depends on the sign of the coefficient of correlation.
Ii) A coefficient of correlation r close to 0 (say, 0.08) shows that the relationship between two variables is quite weak.
Iii) Coefficients of -0.91 and +0.91 have equal strength.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false
Ii) A coefficient of correlation r close to 0 (say, 0.08) shows that the relationship between two variables is quite weak.
Iii) Coefficients of -0.91 and +0.91 have equal strength.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
45
Information was collected from employee records to determine whether there is an association between an employee's age and the number or workdays they miss. Partial excel results are
Summarized below from two different samples: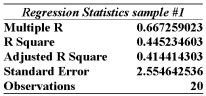
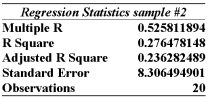
Given this information alone, would you decide to continue with the regression analysis for sample #1 or #2 or both?
A) Continue with both samples, because the sample sizes are over 15
B) Continue with sample #1 because the multiple r value is larger than that of sample #2
C) Continue with sample #2 because the multiple r value is larger than that of sample #1
D) Don't continue with either sample, because the standard error values are more than 2
E) Don't continue with either sample, because the sample sizes are too small to be of use
Summarized below from two different samples:


Given this information alone, would you decide to continue with the regression analysis for sample #1 or #2 or both?
A) Continue with both samples, because the sample sizes are over 15
B) Continue with sample #1 because the multiple r value is larger than that of sample #2
C) Continue with sample #2 because the multiple r value is larger than that of sample #1
D) Don't continue with either sample, because the standard error values are more than 2
E) Don't continue with either sample, because the sample sizes are too small to be of use

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
46
We have collected price per share and dividend information from a sample of 30 companies. 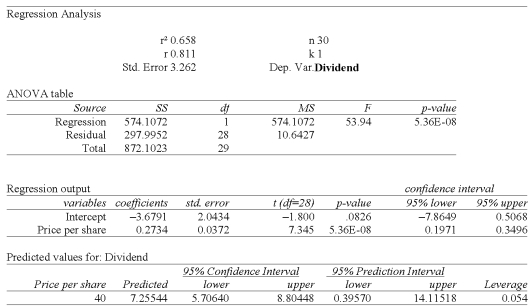
The slope in this instance indicates:
A) For each additional dollar in stock price, we can anticipate an additional $2.73 in dividend
B) For each additional dollar in stock price, we can anticipate an additional $3.68 in dividend
C) For each additional dollar in stock price, we can anticipate an additional $0.27 in dividend
D) For each additional dollar in dividend, we can anticipate an additional $2.71 in stock price
E) For each additional dollar in dividend, we can anticipate a drop of $3.68 in stock price

The slope in this instance indicates:
A) For each additional dollar in stock price, we can anticipate an additional $2.73 in dividend
B) For each additional dollar in stock price, we can anticipate an additional $3.68 in dividend
C) For each additional dollar in stock price, we can anticipate an additional $0.27 in dividend
D) For each additional dollar in dividend, we can anticipate an additional $2.71 in stock price
E) For each additional dollar in dividend, we can anticipate a drop of $3.68 in stock price

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
47
A sales manager for an advertising agency believes there is a relationship between the number of contacts and the amount of the sales. To verify this believe, the following data was collected: 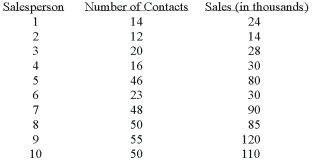
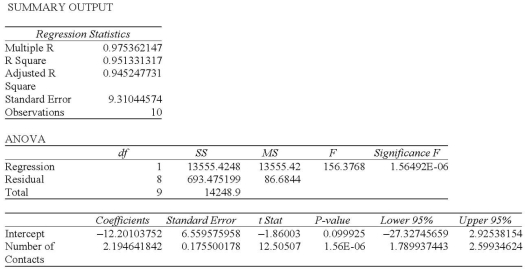
What is the Y-intercept of the linear equation?
A) -12.201
B) 2.1946
C) -2.1946
D) 12.201
E) None of the choices are correct


What is the Y-intercept of the linear equation?
A) -12.201
B) 2.1946
C) -2.1946
D) 12.201
E) None of the choices are correct

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
48
A sales manager for an advertising agency believes there is a relationship between the number of contacts and the amount of the sales. To verify this believe, the following data was collected: 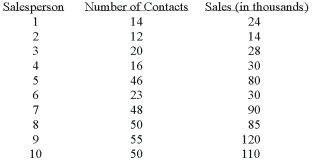
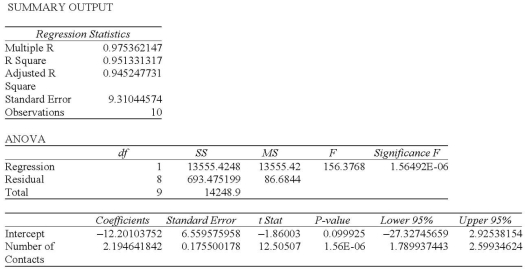
What is the slope of the linear equation?
A) -12.201
B) 12.201
C) 2.1946
D) -2.1946
E) None of the choices are correct


What is the slope of the linear equation?
A) -12.201
B) 12.201
C) 2.1946
D) -2.1946
E) None of the choices are correct

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
49
A sales manager for an advertising agency believes there is a relationship between the number of contacts and the amount of the sales. To verify this belief, the following data was collected: 
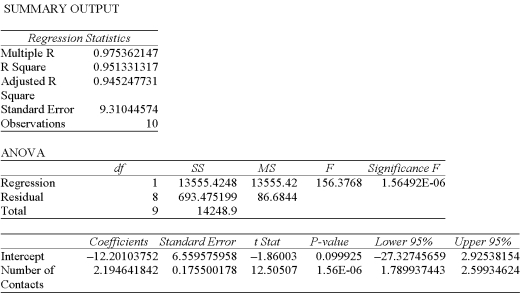
What is the regression equation?
A) Y' = 2.1946 - 12.201X
B) Y' = -12.201X + 2.1946X
C) Y' = 12.201 + 2.1946X
D) Y' = 2.1946 + 12.201X
E) None of the choices are correct


What is the regression equation?
A) Y' = 2.1946 - 12.201X
B) Y' = -12.201X + 2.1946X
C) Y' = 12.201 + 2.1946X
D) Y' = 2.1946 + 12.201X
E) None of the choices are correct

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
50
Data is collected from 20 sales people in order to verify that the more contacts made with potential clients, the greater the sales volume. The Excel printout is shown below. 
The slope in this instance indicates:
A) For each additional contact made, the salesperson can anticipate an additional $1983 in sales
B) For each additional contact made, the salesperson can anticipate an additional $1.98 in sales
C) For each additional contact made, the salesperson can anticipate an additional $7,554 in sales
D) For each additional contact made, the salesperson can anticipate a drop of $7,554 in sales
E) For each additional sale made, the salesperson can anticipate an additional 2 contacts are needed

The slope in this instance indicates:
A) For each additional contact made, the salesperson can anticipate an additional $1983 in sales
B) For each additional contact made, the salesperson can anticipate an additional $1.98 in sales
C) For each additional contact made, the salesperson can anticipate an additional $7,554 in sales
D) For each additional contact made, the salesperson can anticipate a drop of $7,554 in sales
E) For each additional sale made, the salesperson can anticipate an additional 2 contacts are needed

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
51
i. The purpose of correlation analysis is to find how strong the relationship is between two variables.
ii. A correlation coefficient of -1 or +1 indicates perfect correlation.
Iii) The standard error of estimate measures the accuracy of our prediction.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false
ii. A correlation coefficient of -1 or +1 indicates perfect correlation.
Iii) The standard error of estimate measures the accuracy of our prediction.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
52
i. A coefficient of correlation r close to 0 (say, 0.08) shows that the relationship between two variables is quite weak.
Ii) The coefficient of determination is the proportion of the total variation in the dependent variable Y
That is explained or accounted for by its relationship with the independent variable X.
iii. If the coefficient of correlation is -0.90, the coefficient of determination is -0.81.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false
Ii) The coefficient of determination is the proportion of the total variation in the dependent variable Y
That is explained or accounted for by its relationship with the independent variable X.
iii. If the coefficient of correlation is -0.90, the coefficient of determination is -0.81.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
53
i. A coefficient of correlation r close to 0 (say, 0.08) shows that the relationship between two variables is quite weak.
Ii) A coefficient of correlation of -0.96 indicates a very weak negative correlation.
iii. The coefficient of determination can only be positive.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false
Ii) A coefficient of correlation of -0.96 indicates a very weak negative correlation.
iii. The coefficient of determination can only be positive.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
54
i. The strength of the correlation between two variables depends on the sign of the coefficient of correlation.
Ii) A coefficient of correlation r close to 0 (say, 0.08) shows that the relationship between two variables is quite weak.
Iii) The coefficient of determination is found by taking the square root of the coefficient of correlation.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) is a correct statement but not (i) or (iii).
E) All statements are false
Ii) A coefficient of correlation r close to 0 (say, 0.08) shows that the relationship between two variables is quite weak.
Iii) The coefficient of determination is found by taking the square root of the coefficient of correlation.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) is a correct statement but not (i) or (iii).
E) All statements are false

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
55
i. The purpose of correlation analysis is to find how strong the relationship is between two variables.
ii. A coefficient of correlation r close to 0 (say, 0.08) shows that the relationship between two variables is quite weak.
Iii) The strength of the correlation between two variables depends on the sign of the coefficient of correlation.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false
ii. A coefficient of correlation r close to 0 (say, 0.08) shows that the relationship between two variables is quite weak.
Iii) The strength of the correlation between two variables depends on the sign of the coefficient of correlation.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
56
i. The purpose of correlation analysis is to find how strong the relationship is between two variables.
ii. A coefficient of correlation of -0.96 indicates a very weak negative correlation.
iii. The standard error of estimate measures the accuracy of our prediction.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false
ii. A coefficient of correlation of -0.96 indicates a very weak negative correlation.
iii. The standard error of estimate measures the accuracy of our prediction.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
57
We have collected price per share and dividend information from a sample of 30 companies. Using the Megastat printout, determine the regression equation that predicts the dividend from the stock's selling price. 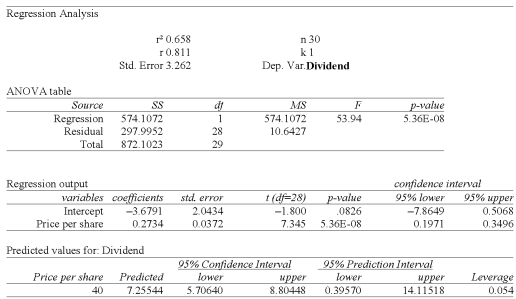
A) Y = 0.27 +3.68x
B) Y = 0.27x + 3.68
C) Y = -3.68 + 0.27x
D) Y = -0.27x - 3.68
E) None of the choices are correct.

A) Y = 0.27 +3.68x
B) Y = 0.27x + 3.68
C) Y = -3.68 + 0.27x
D) Y = -0.27x - 3.68
E) None of the choices are correct.

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
58
i. A coefficient of correlation r close to 0 (say, 0.08) shows that the relationship between two variables is quite weak.
ii. If the coefficient of correlation is 0.68, the coefficient of determination is 0.4624.
iii. The standard error of estimate measures the accuracy of our prediction.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false
ii. If the coefficient of correlation is 0.68, the coefficient of determination is 0.4624.
iii. The standard error of estimate measures the accuracy of our prediction.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
59
Data is collected from 20 sales people in order to verify that the more contacts made with potential clients, the greater the sales volume. The Excel printout is shown below. 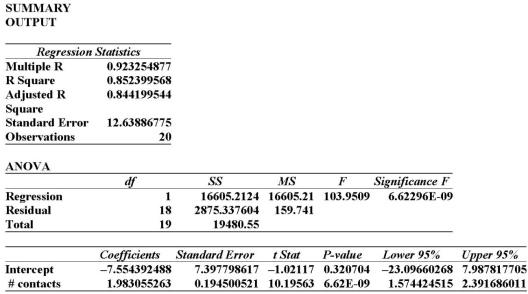
The y-intercept in this instance suggests:
A) For each additional contact made, the salesperson can anticipate an additional $193 in sales
B) For each additional contact made, the salesperson can anticipate a drop of $1983 in sales
C) When no contacts are made, the salesperson can anticipate sales of $7554
D) When no contacts are made, the salesperson can anticipate sales of $1983
E)When no contacts are made, the salesperson can anticipate negative sales - therefore the regression model doesn't make sense for no contacts

The y-intercept in this instance suggests:
A) For each additional contact made, the salesperson can anticipate an additional $193 in sales
B) For each additional contact made, the salesperson can anticipate a drop of $1983 in sales
C) When no contacts are made, the salesperson can anticipate sales of $7554
D) When no contacts are made, the salesperson can anticipate sales of $1983
E)When no contacts are made, the salesperson can anticipate negative sales - therefore the regression model doesn't make sense for no contacts

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
60
We have collected price per share and dividend information from a sample of 30 companies. 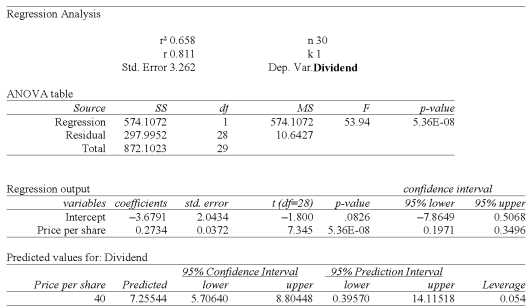 The y-intercept in this instance suggests:
The y-intercept in this instance suggests:
A) For each additional dollar in stock price, we can anticipate an additional $2.73 in dividend
B) For each additional dollar in stock price, we can anticipate a drop of $2.41 in dividend
C) When the stock price is zero, we can anticipate a dividend of $0.27. This value, however, makes no sense
D) When the stock price is zero, we can anticipate a dividend of $-3.68. This value, however, makes no sense
E) When the dividends are zero, we can anticipate a negative share price
 The y-intercept in this instance suggests:
The y-intercept in this instance suggests:A) For each additional dollar in stock price, we can anticipate an additional $2.73 in dividend
B) For each additional dollar in stock price, we can anticipate a drop of $2.41 in dividend
C) When the stock price is zero, we can anticipate a dividend of $0.27. This value, however, makes no sense
D) When the stock price is zero, we can anticipate a dividend of $-3.68. This value, however, makes no sense
E) When the dividends are zero, we can anticipate a negative share price

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
61
i. The coefficient of determination is the proportion of the total variation in the dependent variable Y that is explained or accounted for by its relationship with the independent variable X.
Ii) The coefficient of determination is found by taking the square root of the coefficient of correlation.
iii. The standard error of estimate measures the accuracy of our prediction.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false
Ii) The coefficient of determination is found by taking the square root of the coefficient of correlation.
iii. The standard error of estimate measures the accuracy of our prediction.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
62
i. If the value of r is -0.96, what does this indicate about the dependent variable as the independent variable increases?
Ii) What is the value of the correlation coefficient if there is perfect correlation?
Iii) If the dependent variable is measured in dollars, in what units is the standard error of estimate measured?
A) it increases; zero, dollars squared
B) it decreases; +/- 1.0, dollars squared
C) it increases; 1.0, dollars ($)
D) it decreases; +/- 1.0; dollars ($)
E) it decreases; zero; dollars
Ii) What is the value of the correlation coefficient if there is perfect correlation?
Iii) If the dependent variable is measured in dollars, in what units is the standard error of estimate measured?
A) it increases; zero, dollars squared
B) it decreases; +/- 1.0, dollars squared
C) it increases; 1.0, dollars ($)
D) it decreases; +/- 1.0; dollars ($)
E) it decreases; zero; dollars

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
63
The partial megastat output below is regression analysis of the relationship between annual payroll and number of wins in a season for 28 teams in professional sports. The purpose of the analysis
Is to predict the number of wins when given an annual payroll in $millions. Although technically not a sample, the baseball data below will be treated as a convenience sample of all major league professional sports.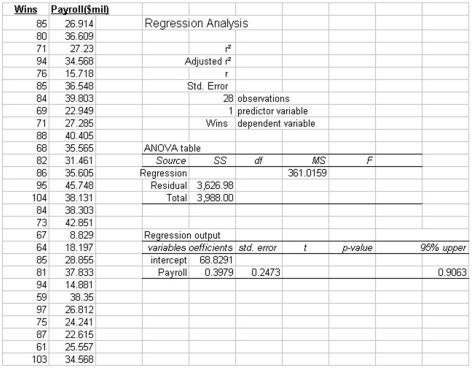
Refer to the printout above. How many independent variables?
A) 1
B) 2
C) 9
D) 10
E) 11
Is to predict the number of wins when given an annual payroll in $millions. Although technically not a sample, the baseball data below will be treated as a convenience sample of all major league professional sports.

Refer to the printout above. How many independent variables?
A) 1
B) 2
C) 9
D) 10
E) 11

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
64
What does the coefficient of determination equal if r = 0.89?
A) 0.94
B) 0.89
C) 0.79
D) 0.06
E) None of these statements are correct
A) 0.94
B) 0.89
C) 0.79
D) 0.06
E) None of these statements are correct

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
65
What is the range of values for a coefficient of correlation?
A) 0 to +1.0
B) -3 to +3 inclusive
C) -1.0 to +1.0 inclusive
D) Unlimited range
E) None of these statements are correct
A) 0 to +1.0
B) -3 to +3 inclusive
C) -1.0 to +1.0 inclusive
D) Unlimited range
E) None of these statements are correct

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
66
i. If there is absolutely no relationship between two variables, what will Pearson's r equal? ii. If the coefficient of correlation is 0.80, what is the coefficient of determination?
Iii) If the coefficient of determination is 0.81, what is the coefficient of correlation?
A) zero (0), 0.64, 0.9 or -0.9
B) zero (0), 0.64, 0.09
C) one (1), 0.64, 0.6561
D) one (1), 0.64, 0.9 or -0.9
E) zero (0), 0.8944, 0.6561
Iii) If the coefficient of determination is 0.81, what is the coefficient of correlation?
A) zero (0), 0.64, 0.9 or -0.9
B) zero (0), 0.64, 0.09
C) one (1), 0.64, 0.6561
D) one (1), 0.64, 0.9 or -0.9
E) zero (0), 0.8944, 0.6561

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
67
i. The coefficient of determination can only be positive.
ii. If the coefficient of correlation is 0.68, the coefficient of determination is 0.4624.
iii. The standard error of estimate measures the accuracy of our prediction.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false
ii. If the coefficient of correlation is 0.68, the coefficient of determination is 0.4624.
iii. The standard error of estimate measures the accuracy of our prediction.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
68
i. If there is absolutely no relationship between two variables, what will Pearson's r equal? ii. If the value of r is -0.96, what does this indicate about the dependent variable as the independent variable increases?
Iii) What is the value of the correlation coefficient if there is perfect correlation?
A) one (1); decreases; zero (0)
B) one (1); increases; zero (0)
C) zero (0); decreases; +/- 1.0
D) zero (0); increases; +/- 1.0
E) +/- 1.0; nothing; +/- 1.0
Iii) What is the value of the correlation coefficient if there is perfect correlation?
A) one (1); decreases; zero (0)
B) one (1); increases; zero (0)
C) zero (0); decreases; +/- 1.0
D) zero (0); increases; +/- 1.0
E) +/- 1.0; nothing; +/- 1.0

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
69
i. Correlation analysis is a group of statistical techniques used to measure the strength of the relationship (correlation) between two variables.
Ii) A correlation coefficient of -1 or +1 indicates perfect correlation.
Iii) The strength of the correlation between two variables depends on the sign of the coefficient of correlation.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false
Ii) A correlation coefficient of -1 or +1 indicates perfect correlation.
Iii) The strength of the correlation between two variables depends on the sign of the coefficient of correlation.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
70
If the correlation between two variables is close to one, the association is
A) strong.
B) moderate.
C) weak.
D) none.
A) strong.
B) moderate.
C) weak.
D) none.

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
71
i. A coefficient of correlation r close to 0 (say, 0.08) shows that the relationship between two variables is quite weak.
Ii) A coefficient of correlation of -0.96 indicates a very weak negative correlation.
Iii) If the coefficient of correlation is 0.68, the coefficient of determination is 0.4624.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false
Ii) A coefficient of correlation of -0.96 indicates a very weak negative correlation.
Iii) If the coefficient of correlation is 0.68, the coefficient of determination is 0.4624.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
72
i. Perfect correlation means that the scatter diagram will appear as a straight line ii. If the coefficient of correlation is 0.80, the coefficient of determination is 0.64.
iii. The coefficient of determination can assume values between 0% and 100%
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false
iii. The coefficient of determination can assume values between 0% and 100%
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
73
Use the scatter diagrams to verify which statements are correct:
Chart A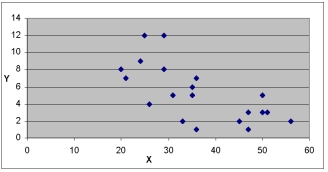
Chart B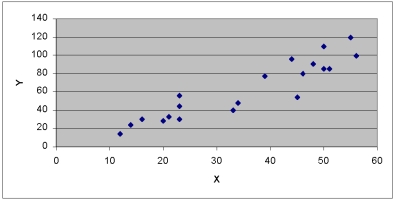
Chart C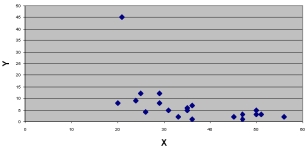
A) The variables in Chart A have a strong positive correlation
B) The variables in Chart C have a strong positive correlation
C) The variables in Chart B have a negative correlation
D) Charts A & B have no obvious outliers
E) None of the choices are correct
Chart A

Chart B

Chart C

A) The variables in Chart A have a strong positive correlation
B) The variables in Chart C have a strong positive correlation
C) The variables in Chart B have a negative correlation
D) Charts A & B have no obvious outliers
E) None of the choices are correct

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
74
i. The coefficient of correlation is a measure of the strength of relationship between two variables.
ii. The coefficient of determination can only be positive.
Iii) The standard error of estimate measures the accuracy of our prediction.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false
ii. The coefficient of determination can only be positive.
Iii) The standard error of estimate measures the accuracy of our prediction.
A) (i), (ii) and (iii) are all correct statements
B) (i) and (ii) are correct statements, but not (iii).
C) (i) and (iii) are correct statements but not (ii).
D) (ii) and (iii) are correct statements but not (i).
E) All statements are false

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
75
i. If the coefficient of correlation is 0.80, what is the coefficient of determination? ii. What is a measure of the scatter of observed values around the regression line called?
Iii) If the correlation between sales and advertising is +0.6, what percent of the variation in sales can be attributed to advertising?
A) 0.4; standard deviation; 0.3
B) 0.64; standard error of the estimate; 36%
C) 0.64; standard error of the estimate; 60%
D) 0.08; variation; 36%
E) 0.4; standard error of the estimate, 60%
Iii) If the correlation between sales and advertising is +0.6, what percent of the variation in sales can be attributed to advertising?
A) 0.4; standard deviation; 0.3
B) 0.64; standard error of the estimate; 36%
C) 0.64; standard error of the estimate; 60%
D) 0.08; variation; 36%
E) 0.4; standard error of the estimate, 60%

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
76
i. If the coefficient of correlation is 0.70, what is the coefficient of determination? ii. If the value of r is -0.88, what does this indicate about the dependent variable as the independent variable increases?
Iii) If the dependent variable is measured in hours, in what units is the standard error of estimate measured?
A) 0.49; it decreases; hours
B) 0.49; it decreases; hours squared
C) 0.49; it increases; hours
D) 0.8367; it decreases; hours
E) 0.8367; it increases; hours squared
Iii) If the dependent variable is measured in hours, in what units is the standard error of estimate measured?
A) 0.49; it decreases; hours
B) 0.49; it decreases; hours squared
C) 0.49; it increases; hours
D) 0.8367; it decreases; hours
E) 0.8367; it increases; hours squared

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
77
Which of the following statements regarding the coefficient of correlation is true?
A) It ranges from -1.0 to +1.0 inclusive
B) It measures the strength of the relationship between two variables
C) A value of 0.00 indicates two variables are not related
D) All of these statements are correct
E) None of these statements are correct
A) It ranges from -1.0 to +1.0 inclusive
B) It measures the strength of the relationship between two variables
C) A value of 0.00 indicates two variables are not related
D) All of these statements are correct
E) None of these statements are correct

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
78
If r = 0.65, what does the coefficient of determination equal?
A) 0.194
B) 0.423
C) 0.577
D) 0.806
E) None of these statements are correct
A) 0.194
B) 0.423
C) 0.577
D) 0.806
E) None of these statements are correct

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
79
If the correlation coefficient between two variables equals zero, what can be said of the variables X and Y?
A) Not related
B) Dependent on each other
C) Highly related
D) All of these statements are correct
E) None of these statements are correct
A) Not related
B) Dependent on each other
C) Highly related
D) All of these statements are correct
E) None of these statements are correct

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck
80
What does a coefficient of correlation of 0.70 infer?
A) Almost no correlation because 0.70 is close to 1.0
B) 70% of the variation in one variable is explained by the other
C) Coefficient of determination is 0.49
D) Coefficient of nondetermination is 0.30
E) None of these statements are correct
A) Almost no correlation because 0.70 is close to 1.0
B) 70% of the variation in one variable is explained by the other
C) Coefficient of determination is 0.49
D) Coefficient of nondetermination is 0.30
E) None of these statements are correct

Unlock Deck
Unlock for access to all 150 flashcards in this deck.
Unlock Deck
k this deck


