Deck 13: Vectors and the Geometry of Space
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/240
Play
Full screen (f)
Deck 13: Vectors and the Geometry of Space
1
Describe the given set of points with a single equation or with a pair of equations.
The circle of radius 6 centered at the point (6, -4, 36) and lying in a plane parallel to the xy-plane
The circle of radius 6 centered at the point (6, -4, 36) and lying in a plane parallel to the xy-plane

B
2
Give a geometric description of the set of points whose coordinates satisfy the given conditions.
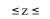
A) All points between z = 10 and z = 11 in the x-y plane
B) The slab between the planes z = 10 and z = 11 (including planes)
C) No set of points satisfies the given relation
D) The line from z = 10 to z = 11
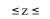
A) All points between z = 10 and z = 11 in the x-y plane
B) The slab between the planes z = 10 and z = 11 (including planes)
C) No set of points satisfies the given relation
D) The line from z = 10 to z = 11
B
2
Give a geometric description of the set of points whose coordinates satisfy the given conditions.
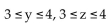
A) The square with corners at (0, 3, 3), (0, 3, 4), (0, 4, 3), and (0, 4, 4)
B) The infinitely long square prism parallel to the x-axis
C) The line between the points (0, 3, 3) and (0, 4, 4)
D) The cube located in the first quadrant and with sides 3 units in length
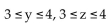
A) The square with corners at (0, 3, 3), (0, 3, 4), (0, 4, 3), and (0, 4, 4)
B) The infinitely long square prism parallel to the x-axis
C) The line between the points (0, 3, 3) and (0, 4, 4)
D) The cube located in the first quadrant and with sides 3 units in length
B
3
Describe the given set of points with a single equation or with a pair of equations.
The circle in which the plane through the point (-8, 12, ) perpendicular to the y-axis meets the sphere of radius 15 centered at the origin.
The circle in which the plane through the point (-8, 12, ) perpendicular to the y-axis meets the sphere of radius 15 centered at the origin.

A
4
Describe the given set of points with a single equation or with a pair of equations.
The circle of radius 2 centered at the point (10, -2, 2) and lying in a plane perpendicular to the x-axis
The circle of radius 2 centered at the point (10, -2, 2) and lying in a plane perpendicular to the x-axis


Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
5
Write one or more inequalities that describe the set of points.
The slab bounded by the planes x = and x = 5 (planes included)
The slab bounded by the planes x = and x = 5 (planes included)


Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
6
Give a geometric description of the set of points whose coordinates satisfy the given conditions.



Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
7
Give a geometric description of the set of points whose coordinates satisfy the given conditions.
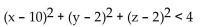
A) No set of points satisfy the given relations.
B) All points on the lower hemisphere centered at (10, 2, 2)
C) All points within the lower hemisphere centered at (10, 2, 2)
D) All points outside the lower hemisphere centered at (10, 2, 2)
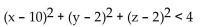
A) No set of points satisfy the given relations.
B) All points on the lower hemisphere centered at (10, 2, 2)
C) All points within the lower hemisphere centered at (10, 2, 2)
D) All points outside the lower hemisphere centered at (10, 2, 2)

Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
8
Describe the given set of points with a single equation or with a pair of equations.
The circle in which the plane through the point (5, -8, -2) perpendicular to the x-axis meets the sphere of radius 13 centered at the origin.
The circle in which the plane through the point (5, -8, -2) perpendicular to the x-axis meets the sphere of radius 13 centered at the origin.


Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
9
Describe the given set of points with a single equation or with a pair of equations.
The plane through the point (-7, -3, 2) and parallel to the xy-plane
A) x + y + 2 = 0
B) x + y = -10
C) z = 2
D) x = -7 and y = -3
The plane through the point (-7, -3, 2) and parallel to the xy-plane
A) x + y + 2 = 0
B) x + y = -10
C) z = 2
D) x = -7 and y = -3

Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
10
Describe the given set of points with a single equation or with a pair of equations.
The plane perpendicular to the y-axis and passing through the point (2, -1, 7)
A) x - 1 + z = 0
B) y = -1
C) 2x + 7z = 0 and y = -1
D) x = 2 and z = 7
The plane perpendicular to the y-axis and passing through the point (2, -1, 7)
A) x - 1 + z = 0
B) y = -1
C) 2x + 7z = 0 and y = -1
D) x = 2 and z = 7

Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
11
Describe the given set of points with a single equation or with a pair of equations.
The set of points equidistant from the points (0, 0, -6) and (0, 0, 4)
A) x + y = -1
B) z = -1
C) z > -6 and z < 4
D) x + y = 0 and -6 < z < 4
The set of points equidistant from the points (0, 0, -6) and (0, 0, 4)
A) x + y = -1
B) z = -1
C) z > -6 and z < 4
D) x + y = 0 and -6 < z < 4

Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
12
Give a geometric description of the set of points whose coordinates satisfy the given conditions.
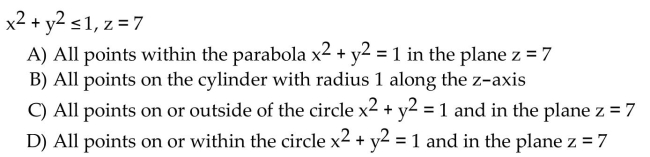
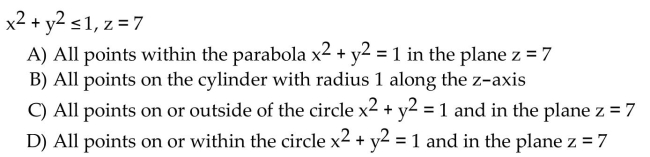

Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
13
Describe the given set of points with a single equation or with a pair of equations.
The plane through the point (1, -4, -5) and parallel to the yz-plane
A) x = 1
B) 1 + y + z = 0
C) -4y - 5z = 0 and x = 1
D) y = -4 and z = -5
The plane through the point (1, -4, -5) and parallel to the yz-plane
A) x = 1
B) 1 + y + z = 0
C) -4y - 5z = 0 and x = 1
D) y = -4 and z = -5

Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
14
Give a geometric description of the set of points whose coordinates satisfy the given conditions.
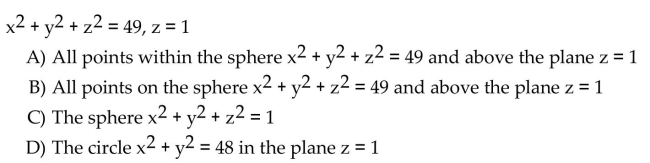
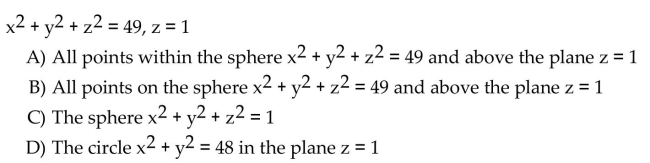

Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
15
Describe the given set of points with a single equation or with a pair of equations.
The set of points equidistant from the points (-1, 0, 0) and (2, 0, 0)
A) y + z = 0.5
B) y + z = 0 and -1 < x < 2
C) x = 0.5
D) x > -1 and x < 2
The set of points equidistant from the points (-1, 0, 0) and (2, 0, 0)
A) y + z = 0.5
B) y + z = 0 and -1 < x < 2
C) x = 0.5
D) x > -1 and x < 2

Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
16
Give a geometric description of the set of points whose coordinates satisfy the given conditions.
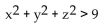
A) All points outside the cylinder with radius 3
B) All points outside the sphere of radius 3
C) All points in space
D) All points on the surface of the cylinder with radius 3
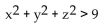
A) All points outside the cylinder with radius 3
B) All points outside the sphere of radius 3
C) All points in space
D) All points on the surface of the cylinder with radius 3

Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
17
Describe the given set of points with a single equation or with a pair of equations.
The plane through the point (-1, -2, -5) and perpendicular to the x-axis
A) y = -2 and z = -5
B) -1 + y + z = 0
C) x = -1
D) y + z = -7
The plane through the point (-1, -2, -5) and perpendicular to the x-axis
A) y = -2 and z = -5
B) -1 + y + z = 0
C) x = -1
D) y + z = -7

Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
18
Write one or more inequalities that describe the set of points.
The rectangular solid in the first octant bounded by the planes x = 5, x = 8, y = 7, y = 9, z = 6 and z = 7 (planes excluded)
A) The given planes do not form a rectangular solid
B) x < y < z
C) 5 < x < 8; 7 < y < 9; 6 < z < 7
D) x < 5, x > 8; y < 7, y > 9; z < 6, z > 7
The rectangular solid in the first octant bounded by the planes x = 5, x = 8, y = 7, y = 9, z = 6 and z = 7 (planes excluded)
A) The given planes do not form a rectangular solid
B) x < y < z
C) 5 < x < 8; 7 < y < 9; 6 < z < 7
D) x < 5, x > 8; y < 7, y > 9; z < 6, z > 7

Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
18
Give a geometric description of the set of points whose coordinates satisfy the given conditions.
x = , z =
A) All points in the x-z plane
B) The point (9, 2)
C) The line through the point (9, 2, 0) and parallel to the z-axis
D) The line through the point (9, 0, 2) and parallel to the y-axis
x = , z =
A) All points in the x-z plane
B) The point (9, 2)
C) The line through the point (9, 2, 0) and parallel to the z-axis
D) The line through the point (9, 0, 2) and parallel to the y-axis

Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
19
Write one or more inequalities that describe the set of points.
The exterior of the sphere of radius 5 centered at the point (-2, -3, -5)
The exterior of the sphere of radius 5 centered at the point (-2, -3, -5)


Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
20
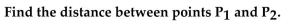


Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
21
Write one or more inequalities that describe the set of points.



Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
22
Find an equation for the sphere with the given center and radius.
Center (-5, 7, 0), radius = 8
Center (-5, 7, 0), radius = 8


Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
23
Find an equation for the sphere with the given center and radius.
Center (0, 0, 10), radius = 8
Center (0, 0, 10), radius = 8


Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
24
Find the center and radius of the sphere.



Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
25
Find an equation for the sphere with the given center and radius.
Center (-2, 0, 0), radius = 9
Center (-2, 0, 0), radius = 9


Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
26
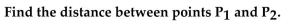


Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
27
Find the center and radius of the sphere.

A) C(0, 10, -1), a = 64
B) C(0, -10, 1), a = 64
C) C(0, 10, -1), a = 8
D) C(0, -10, 1), a = 8

A) C(0, 10, -1), a = 64
B) C(0, -10, 1), a = 64
C) C(0, 10, -1), a = 8
D) C(0, -10, 1), a = 8

Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
28
Find the center and radius of the sphere.
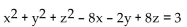
A) C(4, 1, 4), a = 6
B) C(4, 1, -4), a = 6
C) C(4, 1, -4), a = 36
D) C(-4, -1, 4), a = 6
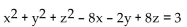
A) C(4, 1, 4), a = 6
B) C(4, 1, -4), a = 6
C) C(4, 1, -4), a = 36
D) C(-4, -1, 4), a = 6

Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
29
Write one or more inequalities that describe the set of points.
The closed region bounded by the spheres of radius 2 and 7, both centered at the origin, and the planes x = 3 and x = 5
The closed region bounded by the spheres of radius 2 and 7, both centered at the origin, and the planes x = 3 and x = 5


Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
30
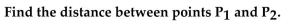


Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
31
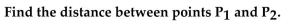

A) 15
B) 18
C) 14
D) 10

Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
32
Find an equation for the sphere with the given center and radius.
Center (0, -8, -1), radius = 10
Center (0, -8, -1), radius = 10


Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
33
Find the center and radius of the sphere.

A) C(9, 5, 3), a = 100
B) C(-9, -5, -3), a = 10
C) C(-9, -5, -3), a = 100
D) C(9, 5, 3), a = 10

A) C(9, 5, 3), a = 100
B) C(-9, -5, -3), a = 10
C) C(-9, -5, -3), a = 100
D) C(9, 5, 3), a = 10

Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
34
Solve the problem.
Find a formula for the distance from the point P(x, y, z) to the z-axis.
Find a formula for the distance from the point P(x, y, z) to the z-axis.


Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
35
Solve the problem.
Find a formula for the distance from the point P(x, y, z) to the xz plane.
Find a formula for the distance from the point P(x, y, z) to the xz plane.


Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
36
Write one or more inequalities that describe the set of points.
The half-space consisting of the points on and behind the yz-plane
The half-space consisting of the points on and behind the yz-plane


Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
37
Find the center and radius of the sphere.



Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
38
Find the center and radius of the sphere.



Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
39
Find the component form of the specified vector.
The vector from the point A(8, 1) to the origin
The vector from the point A(8, 1) to the origin


Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
40
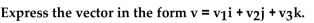

A) v = 5i - 7j - 3k
B) v = 5i - 7j + 3k
C) v = 5i + 7j - 3k
D) v = 5i + 7j + 3k

Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
41
Find the magnitude.
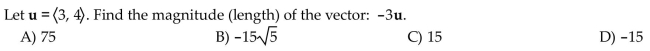
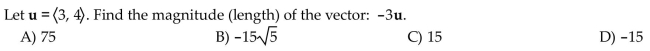

Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
42
Find the indicated vector.



Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
43
Find the magnitude.
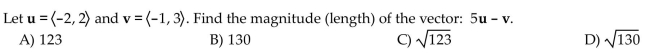
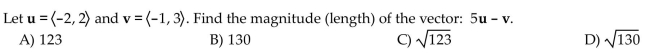

Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
44
Find the indicated vector.



Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
45
Find the indicated vector.



Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
46
Find the indicated vector.



Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
47
Find the component form of the specified vector.
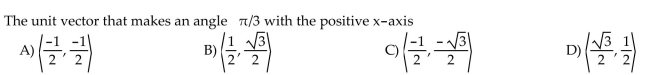
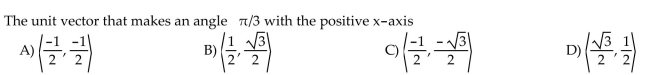

Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
48
Find the component form of the specified vector.
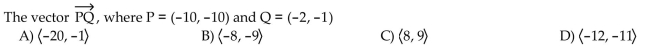
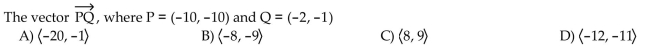

Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
49
Find the indicated vector.



Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
50
Solve the problem.
Find the perimeter of the triangle with vertices A(2, 2, 6), B(2, -2, 6), and C(3, 5, 7).
Find the perimeter of the triangle with vertices A(2, 2, 6), B(2, -2, 6), and C(3, 5, 7).


Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
51
Solve the problem.
Show that the point P( , -5, ) is equidistant from the points A( , -7, ) and B( , -3, ).
Show that the point P( , -5, ) is equidistant from the points A( , -7, ) and B( , -3, ).


Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
52
Find the magnitude.



Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
53
Find the indicated vector.



Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
54
Find the indicated vector.



Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
55
Find the indicated vector.



Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
56
Find the indicated vector.



Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
57
Find the indicated vector.



Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
58
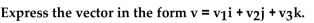
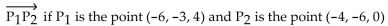
A) v = -2i + 3j - 4k
B) v = -2i - 3j + 4k
C) v = -2i + 3j + 4k
D) v = 2i - 3j - 4k

Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
59
Express the vector as a product of its length and direction.
-5i + 5j + 5k
-5i + 5j + 5k


Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
60
Express the vector as a product of its length and direction.
7j
7j


Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
61
Express the vector as a product of its length and direction.



Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
62
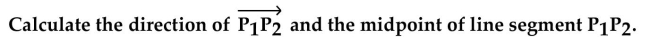


Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
63
Express the vector as a product of its length and direction.
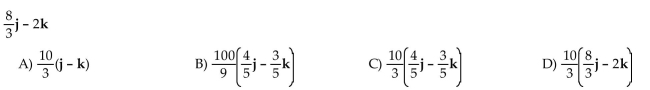
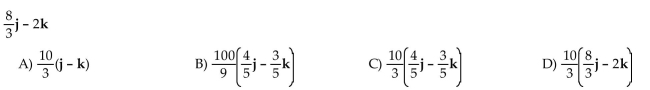

Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
64
Express the vector as a product of its length and direction.
5i + 10j + 10k
5i + 10j + 10k


Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
65
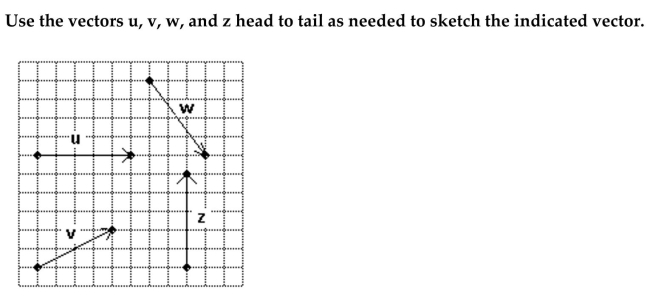
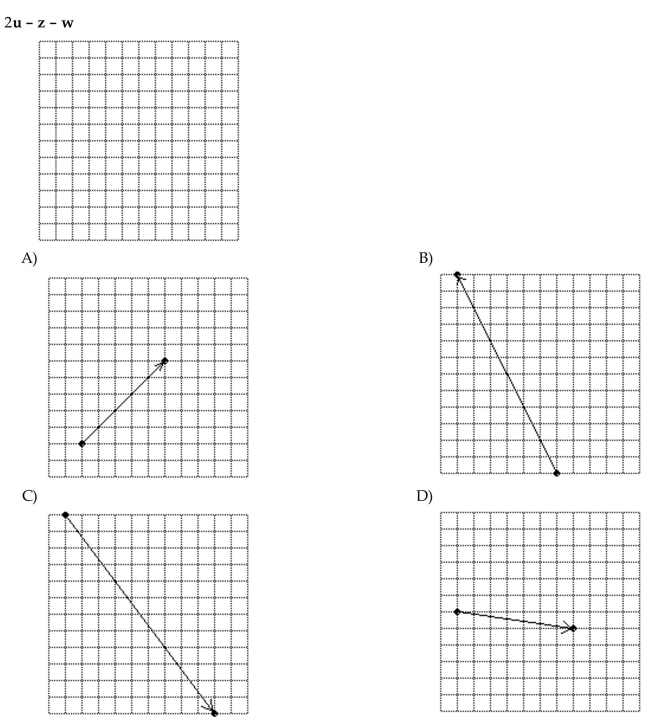

Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
66
Solve the problem.
Find a vector of magnitude 11 in the direction of v = 5i - 12k.
Find a vector of magnitude 11 in the direction of v = 5i - 12k.


Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
67
Express the vector as a product of its length and direction.
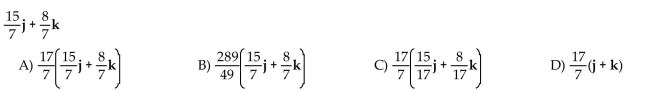
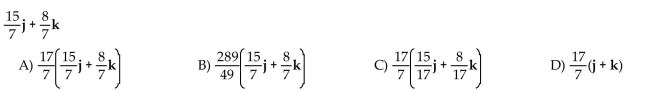

Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
68

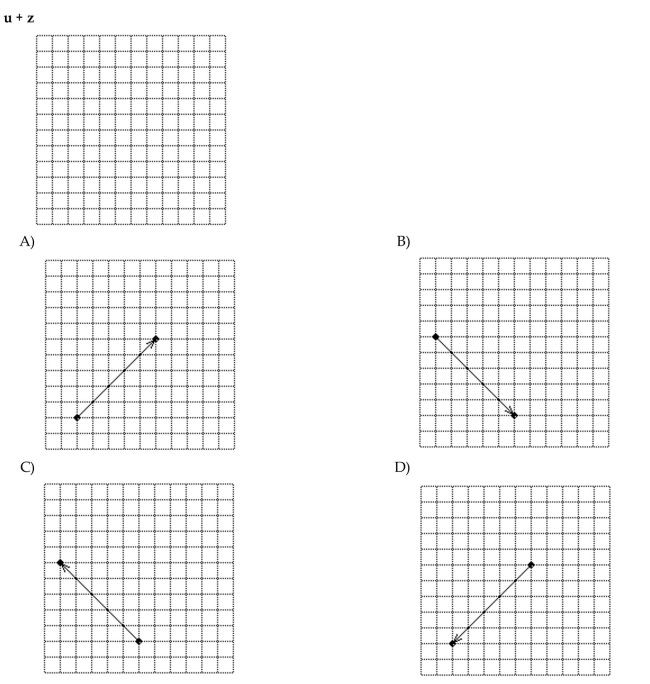

Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
69
Express the vector as a product of its length and direction.



Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
70

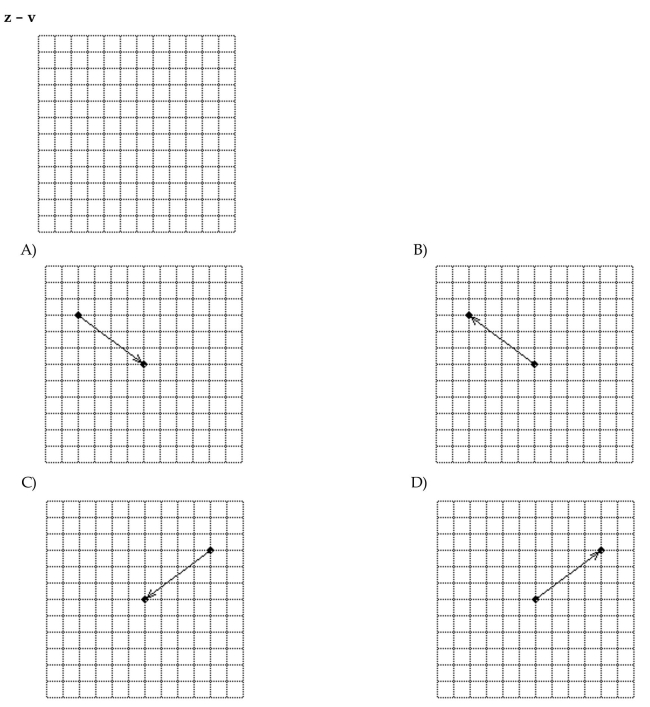

Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
71
Express the vector as a product of its length and direction.



Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
72
Express the vector as a product of its length and direction.



Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
73

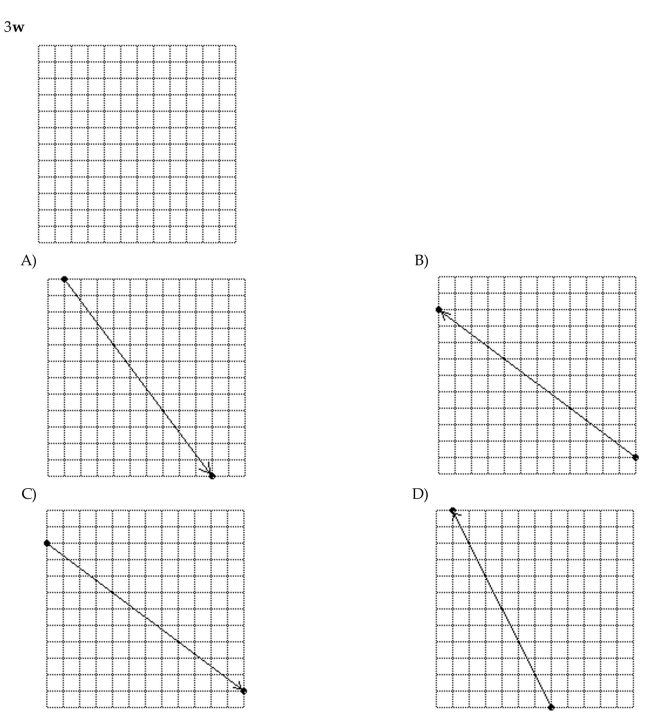

Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
74
Solve the problem.



Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
75
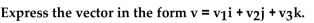

A) v = -8i + 12j - 5k
B) v = 22i + 7j - 5k
C) v = 7i + 7j - 5k
D) v = -8i + 7j - 5k

Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
76

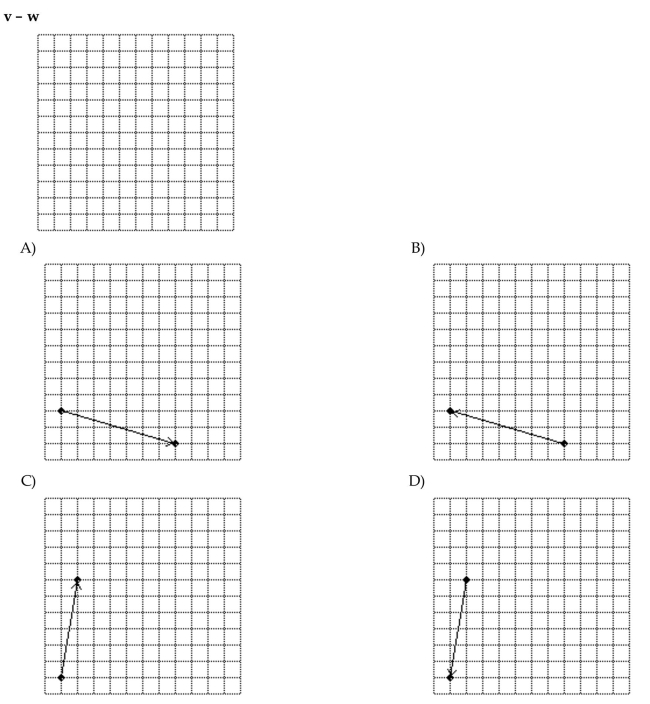

Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
77
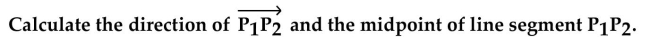


Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck
78
Express the vector as a product of its length and direction.



Unlock Deck
Unlock for access to all 240 flashcards in this deck.
Unlock Deck
k this deck


