Deck 4: Quadrilaterals
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/14
Play
Full screen (f)
Deck 4: Quadrilaterals
1
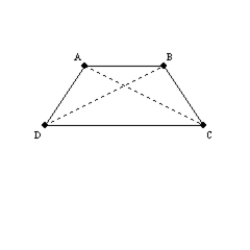
Suppose that you have already proved that "The diagonals of an isosceles trapezoid are congruent." Use this theorem to prove that "A pair of base angles of an isosceles trapezoid are congruent." Supply missing statements and missing reasons for the proof.
Given: Trapezoid ABCD;
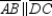 and
and 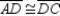 Prove:
Prove: 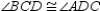 S1. R1.
S1. R1.S2. Draw
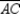 and
and 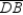 R2.
R2.S3.
 R3.
R3.S4. R4. Identity
S5.
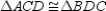 R5.
R5.S6.
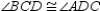 R6.
R6.S1. Trapezoid ABCD;  and
and 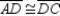 R1. Given
R1. Given
R2. Through 2 points, there is exactly one line.
R3. The diagonals of an isosceles trapezoid are congruent.
S4.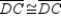 R5. SSS
R5. SSS
R6. CPCTC
 and
and 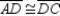 R1. Given
R1. GivenR2. Through 2 points, there is exactly one line.
R3. The diagonals of an isosceles trapezoid are congruent.
S4.
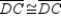 R5. SSS
R5. SSSR6. CPCTC
2
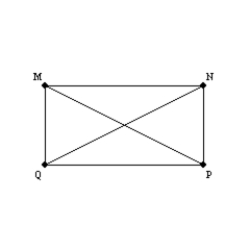
Supply missing statements and missing reasons for the following proof.
Given: Rectangle MNPQ with diagonals
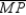 and
and 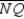 Prove:
Prove: 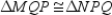 S1. R1.
S1. R1.S2.
 and
and 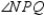 are rt.
are rt.  R2.
R2.S3.
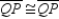 R3.
R3.S4. R4. The diagonals of a rectangle are congruent.
S5. R5.
S1. Rectangle MNPQ with diagonals 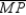 and
and 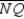 R1. Given
R1. Given
R2. All angles of a rectangle are right angles.
R3. Identity
S4.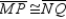 S5.
S5. 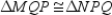 R5. HL
R5. HL
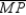 and
and 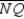 R1. Given
R1. GivenR2. All angles of a rectangle are right angles.
R3. Identity
S4.
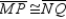 S5.
S5. 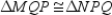 R5. HL
R5. HL 3
Provide missing reasons for the proof of the theorem, "A diagonal of a parallelogram separates it into two congruent triangles."
Given:
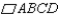 with diagonal
with diagonal 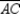 Prove:
Prove:  S1.
S1.  with diagonal
with diagonal  R1.
R1.S2.
 R2.
R2.S3.
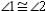 R3.
R3.S4.
 R4.
R4.S5.
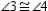 R5.
R5.S6.
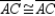 R6.
R6.S7.
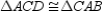 R7.
R7.R1. Given
R2. The opposite sides of a parallelogram are parallel (definition).
R3. If 2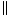 lines are cut by a trans, the alternate interior angles are congruent.
lines are cut by a trans, the alternate interior angles are congruent.
R4. Same as reason 2
R5. Same as reason 3.
R6. Identity
R7. ASA
R2. The opposite sides of a parallelogram are parallel (definition).
R3. If 2
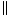 lines are cut by a trans, the alternate interior angles are congruent.
lines are cut by a trans, the alternate interior angles are congruent.R4. Same as reason 2
R5. Same as reason 3.
R6. Identity
R7. ASA
4
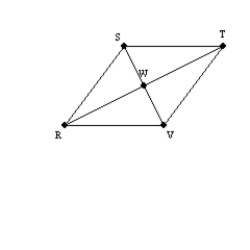
Given the rhombus RSTV, diagonals
 and
and  intersect at point W. Explain why it follows that
intersect at point W. Explain why it follows that 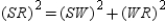 .
.
Unlock Deck
Unlock for access to all 14 flashcards in this deck.
Unlock Deck
k this deck
5
![Use the drawing shown to explain the following theorem. The length of the median of a trapezoid equals one-half the sum of the lengths of the two bases. Given: Trapezoid ABCD with median Prove: [Hint: X is the midpoint of auxiliary diagonal .]](https://storage.examlex.com/TB7237/11eb4b36_770b_04b5_a05a_59dc4a48d610_TB7237_11.jpg)
Use the drawing shown to explain the following theorem.
"The length of the median of a trapezoid equals one-half the sum of the lengths of the two bases."
Given: Trapezoid ABCD with median
![Use the drawing shown to explain the following theorem. The length of the median of a trapezoid equals one-half the sum of the lengths of the two bases. Given: Trapezoid ABCD with median Prove: [Hint: X is the midpoint of auxiliary diagonal .]](https://storage.examlex.com/TB7237/11eb4b36_770b_04b6_a05a_8b919b6290ac_TB7237_11.jpg) Prove:
Prove: ![Use the drawing shown to explain the following theorem. The length of the median of a trapezoid equals one-half the sum of the lengths of the two bases. Given: Trapezoid ABCD with median Prove: [Hint: X is the midpoint of auxiliary diagonal .]](https://storage.examlex.com/TB7237/11eb4b36_770b_04b7_a05a_d9b26ae03aaf_TB7237_11.jpg) [Hint: X is the midpoint of auxiliary diagonal
[Hint: X is the midpoint of auxiliary diagonal ![Use the drawing shown to explain the following theorem. The length of the median of a trapezoid equals one-half the sum of the lengths of the two bases. Given: Trapezoid ABCD with median Prove: [Hint: X is the midpoint of auxiliary diagonal .]](https://storage.examlex.com/TB7237/11eb4b36_770b_04b8_a05a_3bcb21128df7_TB7237_11.jpg) .]
.]
Unlock Deck
Unlock for access to all 14 flashcards in this deck.
Unlock Deck
k this deck
6
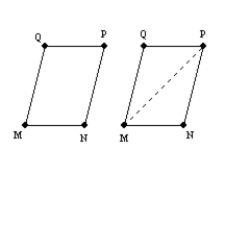
Provide the missing statements abd nissing reasons for the proof of this theorem.
"If both pairs of opposite sides of a quadrilateral are congruent, the quadrilateral is a parallelogram."
Given: Quad. MNPQ;
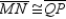 and
and  Prove: MNPQ is a parallelogram
Prove: MNPQ is a parallelogramS1. R1. Given
S2. Draw diagonal
 R2.
R2.S3. R3. Identity
S4. R4. SSS
S5.
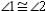 R5.
R5.S6. R6. If 2 lines are cut by a trans. so that alternate interior angles
are congruent, these lines are parallel.
S7.
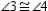 R7.
R7.S8.
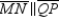 R8.
R8.S9. R9.

Unlock Deck
Unlock for access to all 14 flashcards in this deck.
Unlock Deck
k this deck
7
Supply missing statements and missing reasons for this proof.
Given: Kite MNPQ with diagonal
 ;
;  and
and  Prove:
Prove: 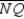 bisects
bisects 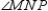 S1. R1.
S1. R1.S2.
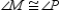 R2. In a kite, one pair of opposite angles are congruent.
R2. In a kite, one pair of opposite angles are congruent.S3.
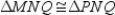 R3.
R3.S4.
 R4.
R4.S5. R5.

Unlock Deck
Unlock for access to all 14 flashcards in this deck.
Unlock Deck
k this deck
8
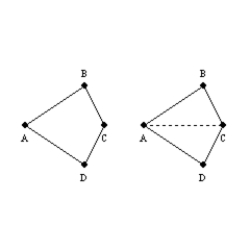
Provide missing statements and missing reasons for the proof of this theorem.
"In a kite, one pair of opposite angles are congruent."
Given: Kite ABCD with
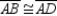 and
and 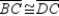 Prove:
Prove: 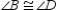 S1. R1.
S1. R1.S2. Draw
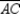 R2.
R2.S3.
 R3.
R3.S4. R4. SSS
S5. R5.

Unlock Deck
Unlock for access to all 14 flashcards in this deck.
Unlock Deck
k this deck
9
In quadrilateral ABCD, the midpoints of the sides are joined in order.
Use the auxiliary diagonals to explain why the resulting quadrilateral MNPQ must be a parallelogram.

Unlock Deck
Unlock for access to all 14 flashcards in this deck.
Unlock Deck
k this deck
10
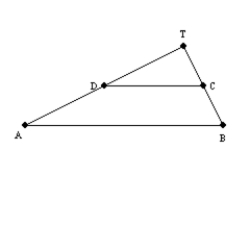
Supply all statements and all reasons for the following proof.
Given:
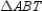 ; M is the midpoint of
; M is the midpoint of 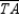 and N is the midpoint of
and N is the midpoint of 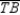 Prove: MNAB is a trapezoid
Prove: MNAB is a trapezoid
Unlock Deck
Unlock for access to all 14 flashcards in this deck.
Unlock Deck
k this deck
11
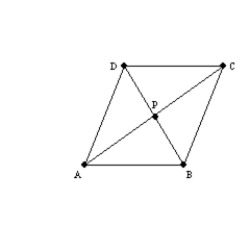
Provide missing statements and missing reasons for the following proof.
Given:
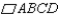 ; diagonals
; diagonals 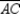 and
and 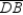 intersect at point P
intersect at point PProve:
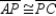 and
and 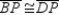 S1. R1. Given
S1. R1. GivenS2.
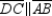 R2.
R2.S3.
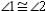 and
and 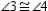 R3.
R3.S4.
 R4.
R4.S5. R5. ASA
S6. R6.

Unlock Deck
Unlock for access to all 14 flashcards in this deck.
Unlock Deck
k this deck
12
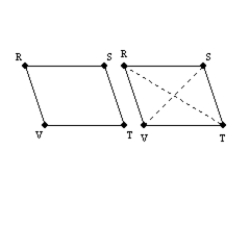
Consider the drawing provided. Using the auxiliary diagonals (shown dashed), explain why the "opposite angles and opposite sides of a parallelogram must be congruent."

Unlock Deck
Unlock for access to all 14 flashcards in this deck.
Unlock Deck
k this deck
13
Provide missing statements and missing reasons for the proof of the following theorem.
"The diagonals of a rhombus are perpendicular."
Given: Rhombus ABCD; diagonals
 and
and 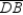 intersect at point X
intersect at point XProve:
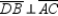 S1. R1.
S1. R1.S2.
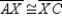 R2. The diagonals of a rhombus (
R2. The diagonals of a rhombus (  ) bisect each other.
) bisect each other.S3.
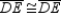 R3.
R3.S4. R4. All sides of a rhombus are congruent.
S5. R5. SSS
S6.
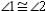 R6.
R6.S7. R7.

Unlock Deck
Unlock for access to all 14 flashcards in this deck.
Unlock Deck
k this deck
14
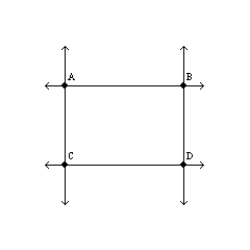
Provide all statements and all reasons for the following proof.
Given:
 ,
, 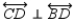 ,
, 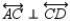 , and
, and 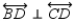 Prove: Quad. ABCD is a parallelogram
Prove: Quad. ABCD is a parallelogram
Unlock Deck
Unlock for access to all 14 flashcards in this deck.
Unlock Deck
k this deck


