Deck 8: The Arbitrage-Free Valuation Framework
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/17
Play
Full screen (f)
Deck 8: The Arbitrage-Free Valuation Framework
1
The following information relates to Questions 1-6
katrina black, portfolio manager at Coral bond Management, ltd., is conducting a training
session with alex Sun, a junior analyst in the fixed income department. black wants to ex-
plain to Sun the arbitrage-free valuation framework used by the firm. black presents Sun with
exhibit 1, showing a fictitious bond being traded on three exchanges, and asks Sun to identify
the arbitrage opportunity of the bond. Sun agrees to ignore transaction costs in his analysis.
exhibit 1 Three-Year, €100 par, 3.00% Coupon, annual-Pay option-Free bond
 black shows Sun some exhibits that were part of a recent presentation. exhibit 3 presents
black shows Sun some exhibits that were part of a recent presentation. exhibit 3 presents
most of the data of a binomial lognormal interest rate tree fit to the yield curve shown in ex-
hibit 2. exhibit 4 presents most of the data of the implied values for a four-year, option-free,
annual-pay bond with a 2.5% coupon based on the information in exhibit 3.
exhibit 2 Yield to Maturity Par rates for one-, two-, and Three-Year annual-Pay
option-Free bonds
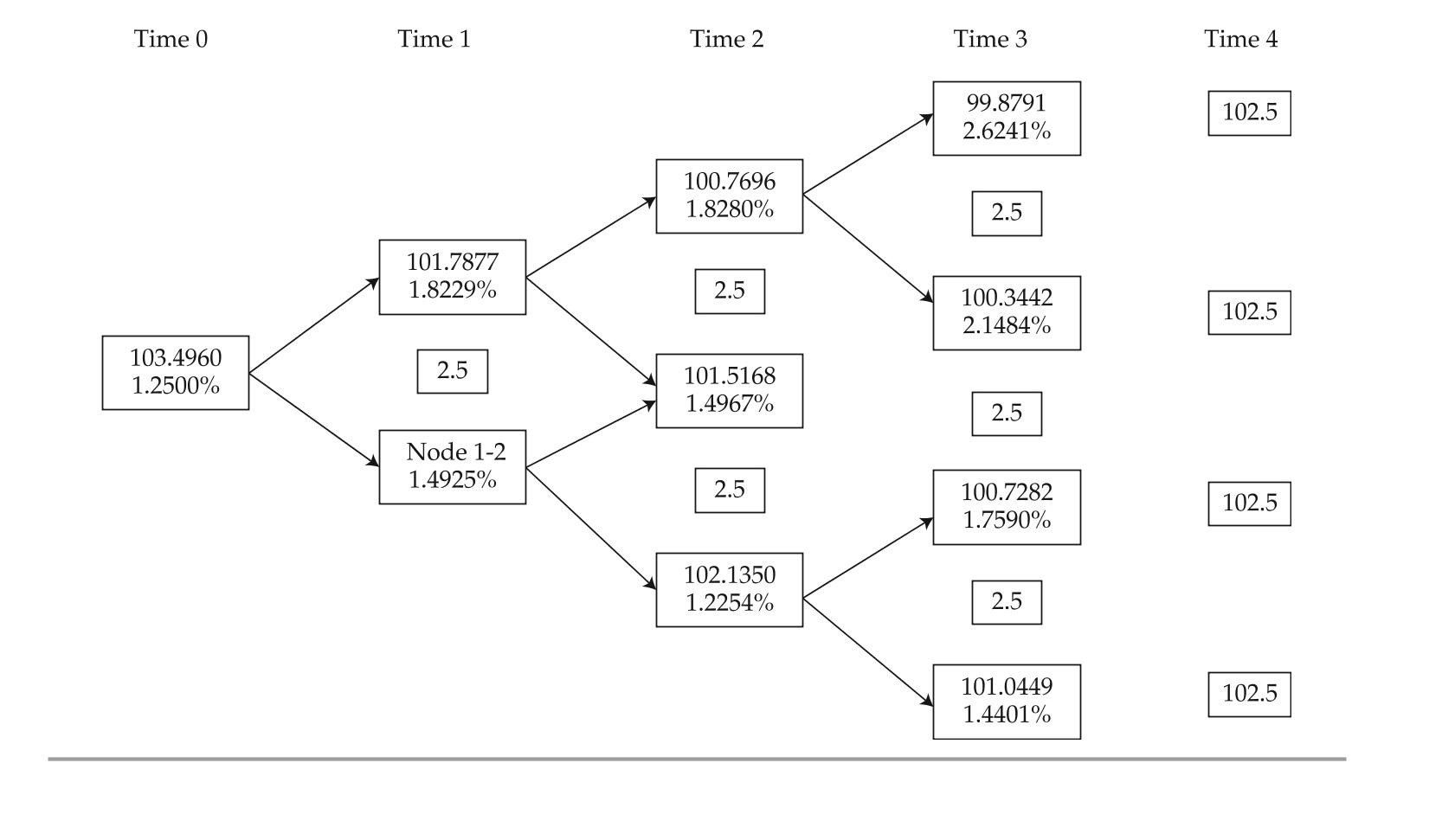
 exhibit 3 binomial interest rate tree Fit to the Yield Curve (Volatility = 10%)
exhibit 3 binomial interest rate tree Fit to the Yield Curve (Volatility = 10%)
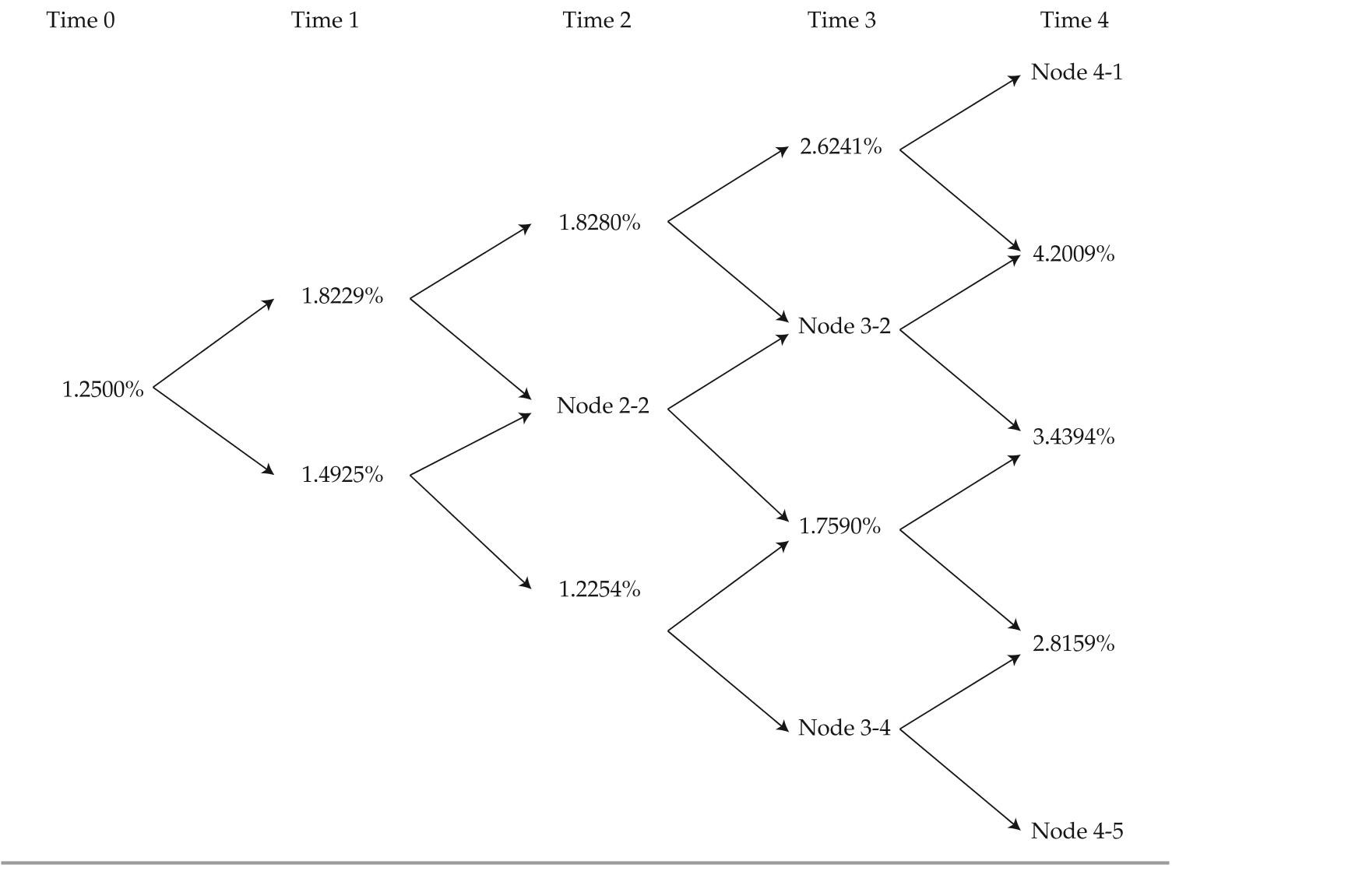 exhibit 4 implied Values (in euros) for a 2.5%, Four-Year, option-Free, annual-Pay bond
exhibit 4 implied Values (in euros) for a 2.5%, Four-Year, option-Free, annual-Pay bond
based on exhibit 3
 black asks about the missing data in exhibits 3 and 4 and directs Sun to complete the
black asks about the missing data in exhibits 3 and 4 and directs Sun to complete the
following tasks related to those exhibits:
task 1 test that the binomial interest tree has been properly calibrated to be
arbitrage-free.
task 2 Develop a spreadsheet model to calculate pathwise valuations. to test the ac-
curacy of the spreadsheet, use the data in exhibit 3 and calculate the value of
the bond if it takes a path of lowest rates in Year 1 and Year 2 and the second
lowest rate in Year 3.
task 3 identify a type of bond where the Monte Carlo calibration method should
be used in place of the binomial interest rate method.
task 4 update exhibit 3 to reflect the current volatility, which is now 15%.
based on exhibit 1, the best action that an investor should take to profit from the arbitrage opportunity is to:
A) buy on Frankfurt, sell on eurex.
B) buy on nYSe euronext, sell on eurex.
C) buy on Frankfurt, sell on nYSe euronext.
katrina black, portfolio manager at Coral bond Management, ltd., is conducting a training
session with alex Sun, a junior analyst in the fixed income department. black wants to ex-
plain to Sun the arbitrage-free valuation framework used by the firm. black presents Sun with
exhibit 1, showing a fictitious bond being traded on three exchanges, and asks Sun to identify
the arbitrage opportunity of the bond. Sun agrees to ignore transaction costs in his analysis.
exhibit 1 Three-Year, €100 par, 3.00% Coupon, annual-Pay option-Free bond
 black shows Sun some exhibits that were part of a recent presentation. exhibit 3 presents
black shows Sun some exhibits that were part of a recent presentation. exhibit 3 presents most of the data of a binomial lognormal interest rate tree fit to the yield curve shown in ex-
hibit 2. exhibit 4 presents most of the data of the implied values for a four-year, option-free,
annual-pay bond with a 2.5% coupon based on the information in exhibit 3.
exhibit 2 Yield to Maturity Par rates for one-, two-, and Three-Year annual-Pay
option-Free bonds
 exhibit 3 binomial interest rate tree Fit to the Yield Curve (Volatility = 10%)
exhibit 3 binomial interest rate tree Fit to the Yield Curve (Volatility = 10%) exhibit 4 implied Values (in euros) for a 2.5%, Four-Year, option-Free, annual-Pay bond
exhibit 4 implied Values (in euros) for a 2.5%, Four-Year, option-Free, annual-Pay bond based on exhibit 3
 black asks about the missing data in exhibits 3 and 4 and directs Sun to complete the
black asks about the missing data in exhibits 3 and 4 and directs Sun to complete the following tasks related to those exhibits:
task 1 test that the binomial interest tree has been properly calibrated to be
arbitrage-free.
task 2 Develop a spreadsheet model to calculate pathwise valuations. to test the ac-
curacy of the spreadsheet, use the data in exhibit 3 and calculate the value of
the bond if it takes a path of lowest rates in Year 1 and Year 2 and the second
lowest rate in Year 3.
task 3 identify a type of bond where the Monte Carlo calibration method should
be used in place of the binomial interest rate method.
task 4 update exhibit 3 to reflect the current volatility, which is now 15%.
based on exhibit 1, the best action that an investor should take to profit from the arbitrage opportunity is to:
A) buy on Frankfurt, sell on eurex.
B) buy on nYSe euronext, sell on eurex.
C) buy on Frankfurt, sell on nYSe euronext.
A
2
The following information relates to Questions 1-6
katrina black, portfolio manager at Coral bond Management, ltd., is conducting a training
session with alex Sun, a junior analyst in the fixed income department. black wants to ex-
plain to Sun the arbitrage-free valuation framework used by the firm. black presents Sun with
exhibit 1, showing a fictitious bond being traded on three exchanges, and asks Sun to identify
the arbitrage opportunity of the bond. Sun agrees to ignore transaction costs in his analysis.
exhibit 1 Three-Year, €100 par, 3.00% Coupon, annual-Pay option-Free bond
 black shows Sun some exhibits that were part of a recent presentation. exhibit 3 presents
black shows Sun some exhibits that were part of a recent presentation. exhibit 3 presents
most of the data of a binomial lognormal interest rate tree fit to the yield curve shown in ex-
hibit 2. exhibit 4 presents most of the data of the implied values for a four-year, option-free,
annual-pay bond with a 2.5% coupon based on the information in exhibit 3.
exhibit 2 Yield to Maturity Par rates for one-, two-, and Three-Year annual-Pay
option-Free bonds
 exhibit 3 binomial interest rate tree Fit to the Yield Curve (Volatility = 10%)
exhibit 3 binomial interest rate tree Fit to the Yield Curve (Volatility = 10%)
 exhibit 4 implied Values (in euros) for a 2.5%, Four-Year, option-Free, annual-Pay bond
exhibit 4 implied Values (in euros) for a 2.5%, Four-Year, option-Free, annual-Pay bond
based on exhibit 3
 black asks about the missing data in exhibits 3 and 4 and directs Sun to complete the
black asks about the missing data in exhibits 3 and 4 and directs Sun to complete the
following tasks related to those exhibits:
task 1 test that the binomial interest tree has been properly calibrated to be
arbitrage-free.
task 2 Develop a spreadsheet model to calculate pathwise valuations. to test the ac-
curacy of the spreadsheet, use the data in exhibit 3 and calculate the value of
the bond if it takes a path of lowest rates in Year 1 and Year 2 and the second
lowest rate in Year 3.
task 3 identify a type of bond where the Monte Carlo calibration method should
be used in place of the binomial interest rate method.
task 4 update exhibit 3 to reflect the current volatility, which is now 15%.
if the assumed volatility is changed as black requested in task 4, the forward rates shown in exhibit 3 will most likely:
A) spread out.
B) remain unchanged.
C) converge to the spot rates.
katrina black, portfolio manager at Coral bond Management, ltd., is conducting a training
session with alex Sun, a junior analyst in the fixed income department. black wants to ex-
plain to Sun the arbitrage-free valuation framework used by the firm. black presents Sun with
exhibit 1, showing a fictitious bond being traded on three exchanges, and asks Sun to identify
the arbitrage opportunity of the bond. Sun agrees to ignore transaction costs in his analysis.
exhibit 1 Three-Year, €100 par, 3.00% Coupon, annual-Pay option-Free bond
 black shows Sun some exhibits that were part of a recent presentation. exhibit 3 presents
black shows Sun some exhibits that were part of a recent presentation. exhibit 3 presents most of the data of a binomial lognormal interest rate tree fit to the yield curve shown in ex-
hibit 2. exhibit 4 presents most of the data of the implied values for a four-year, option-free,
annual-pay bond with a 2.5% coupon based on the information in exhibit 3.
exhibit 2 Yield to Maturity Par rates for one-, two-, and Three-Year annual-Pay
option-Free bonds
 exhibit 3 binomial interest rate tree Fit to the Yield Curve (Volatility = 10%)
exhibit 3 binomial interest rate tree Fit to the Yield Curve (Volatility = 10%) exhibit 4 implied Values (in euros) for a 2.5%, Four-Year, option-Free, annual-Pay bond
exhibit 4 implied Values (in euros) for a 2.5%, Four-Year, option-Free, annual-Pay bond based on exhibit 3
 black asks about the missing data in exhibits 3 and 4 and directs Sun to complete the
black asks about the missing data in exhibits 3 and 4 and directs Sun to complete the following tasks related to those exhibits:
task 1 test that the binomial interest tree has been properly calibrated to be
arbitrage-free.
task 2 Develop a spreadsheet model to calculate pathwise valuations. to test the ac-
curacy of the spreadsheet, use the data in exhibit 3 and calculate the value of
the bond if it takes a path of lowest rates in Year 1 and Year 2 and the second
lowest rate in Year 3.
task 3 identify a type of bond where the Monte Carlo calibration method should
be used in place of the binomial interest rate method.
task 4 update exhibit 3 to reflect the current volatility, which is now 15%.
if the assumed volatility is changed as black requested in task 4, the forward rates shown in exhibit 3 will most likely:
A) spread out.
B) remain unchanged.
C) converge to the spot rates.
A
3
The following information relates to Questions
Meredith alvarez is a junior fixed-income analyst with Canzim asset Management. her su-
pervisor, Stephanie hartson, asks alvarez to review the asset price and payoff data shown in
exhibit 1 to determine whether an arbitrage opportunity exists.
exhibit 1 Price and Payoffs for two risk-Free assets

hartson also shows alvarez data for a bond that trades in three different markets in the
same currency. These data appear in exhibit 2.
exhibit 2 2% Coupon, Five-Year Maturity, annual-Pay bond

hartson asks alvarez to value two bonds (bond C and bond D) using the binomial tree
in exhibit 3. exhibit 4 presents selected data for both bonds.
exhibit 3 binomial interest rate tree with Volatility = 25%

exhibit 4 Selected Data on annual-Pay bonds

hartson tells alvarez that she and her peers have been debating various viewpoints regard-
ing the conditions underlying binomial interest rate trees. The following statements were made
in the course of the debate.
Statement 1: The only requirements needed to create a binomial interest rate tree are
current benchmark interest rates and an assumption about interest rate
volatility.
Statement 2: Potential interest rate volatility in a binomial interest rate tree can be es-
timated using historical interest rate volatility or observed market prices
from interest rate derivatives.
Statement 3: a bond value derived from a binomial interest rate tree with a relatively
high volatility assumption will be different from the value calculated by
discounting the bond's cash flows using current spot rates.
based on data in exhibit 5, hartson asks alvarez to calibrate a binomial interest rate tree
starting with the calculation of implied forward rates shown in exhibit 6.
exhibit 5 Selected Data for a binomial interest rate tree
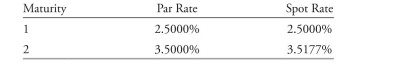
exhibit 6 Calibration of binomial interest rate
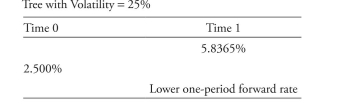
hartson mentions pathwise valuations as another method to value bonds using a binomial
interest rate tree. using the binomial interest rate tree in exhibit 3, alvarez calculates the pos-
sible interest rate paths for bond D shown in exhibit 7.
exhibit 7 interest rate Paths for bond D
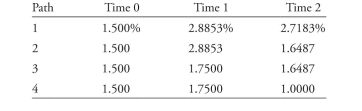
before leaving for the day, hartson asks alvarez about the value of using the Monte Carlo
method to simulate a large number of potential interest rate paths to value a bond. alvarez
makes the following statements.
Statement 4: increasing the number of paths increases the estimate's statistical accuracy.
Statement 5: The bond value derived from a Monte Carlo simulation will be closer to
the bond's true fundamental value.
based on exhibits 4 and 7, the present value of bond D's cash flows following Path 2 is closest to:
A) 97.0322.
B) 102.8607.
C) 105.8607.
Meredith alvarez is a junior fixed-income analyst with Canzim asset Management. her su-
pervisor, Stephanie hartson, asks alvarez to review the asset price and payoff data shown in
exhibit 1 to determine whether an arbitrage opportunity exists.
exhibit 1 Price and Payoffs for two risk-Free assets

hartson also shows alvarez data for a bond that trades in three different markets in the
same currency. These data appear in exhibit 2.
exhibit 2 2% Coupon, Five-Year Maturity, annual-Pay bond

hartson asks alvarez to value two bonds (bond C and bond D) using the binomial tree
in exhibit 3. exhibit 4 presents selected data for both bonds.
exhibit 3 binomial interest rate tree with Volatility = 25%

exhibit 4 Selected Data on annual-Pay bonds

hartson tells alvarez that she and her peers have been debating various viewpoints regard-
ing the conditions underlying binomial interest rate trees. The following statements were made
in the course of the debate.
Statement 1: The only requirements needed to create a binomial interest rate tree are
current benchmark interest rates and an assumption about interest rate
volatility.
Statement 2: Potential interest rate volatility in a binomial interest rate tree can be es-
timated using historical interest rate volatility or observed market prices
from interest rate derivatives.
Statement 3: a bond value derived from a binomial interest rate tree with a relatively
high volatility assumption will be different from the value calculated by
discounting the bond's cash flows using current spot rates.
based on data in exhibit 5, hartson asks alvarez to calibrate a binomial interest rate tree
starting with the calculation of implied forward rates shown in exhibit 6.
exhibit 5 Selected Data for a binomial interest rate tree

exhibit 6 Calibration of binomial interest rate

hartson mentions pathwise valuations as another method to value bonds using a binomial
interest rate tree. using the binomial interest rate tree in exhibit 3, alvarez calculates the pos-
sible interest rate paths for bond D shown in exhibit 7.
exhibit 7 interest rate Paths for bond D

before leaving for the day, hartson asks alvarez about the value of using the Monte Carlo
method to simulate a large number of potential interest rate paths to value a bond. alvarez
makes the following statements.
Statement 4: increasing the number of paths increases the estimate's statistical accuracy.
Statement 5: The bond value derived from a Monte Carlo simulation will be closer to
the bond's true fundamental value.
based on exhibits 4 and 7, the present value of bond D's cash flows following Path 2 is closest to:
A) 97.0322.
B) 102.8607.
C) 105.8607.
B
4
The following information relates to Questions
Meredith alvarez is a junior fixed-income analyst with Canzim asset Management. her su-
pervisor, Stephanie hartson, asks alvarez to review the asset price and payoff data shown in
exhibit 1 to determine whether an arbitrage opportunity exists.
exhibit 1 Price and Payoffs for two risk-Free assets

hartson also shows alvarez data for a bond that trades in three different markets in the
same currency. These data appear in exhibit 2.
exhibit 2 2% Coupon, Five-Year Maturity, annual-Pay bond

hartson asks alvarez to value two bonds (bond C and bond D) using the binomial tree
in exhibit 3. exhibit 4 presents selected data for both bonds.
exhibit 3 binomial interest rate tree with Volatility = 25%

exhibit 4 Selected Data on annual-Pay bonds

hartson tells alvarez that she and her peers have been debating various viewpoints regard-
ing the conditions underlying binomial interest rate trees. The following statements were made
in the course of the debate.
Statement 1: The only requirements needed to create a binomial interest rate tree are
current benchmark interest rates and an assumption about interest rate
volatility.
Statement 2: Potential interest rate volatility in a binomial interest rate tree can be es-
timated using historical interest rate volatility or observed market prices
from interest rate derivatives.
Statement 3: a bond value derived from a binomial interest rate tree with a relatively
high volatility assumption will be different from the value calculated by
discounting the bond's cash flows using current spot rates.
based on data in exhibit 5, hartson asks alvarez to calibrate a binomial interest rate tree
starting with the calculation of implied forward rates shown in exhibit 6.
exhibit 5 Selected Data for a binomial interest rate tree

exhibit 6 Calibration of binomial interest rate

hartson mentions pathwise valuations as another method to value bonds using a binomial
interest rate tree. using the binomial interest rate tree in exhibit 3, alvarez calculates the pos-
sible interest rate paths for bond D shown in exhibit 7.
exhibit 7 interest rate Paths for bond D

before leaving for the day, hartson asks alvarez about the value of using the Monte Carlo
method to simulate a large number of potential interest rate paths to value a bond. alvarez
makes the following statements.
Statement 4: increasing the number of paths increases the estimate's statistical accuracy.
Statement 5: The bond value derived from a Monte Carlo simulation will be closer to
the bond's true fundamental value.
based on exhibits 3 and 4, the price for bond D is closest to:
A) 97.4785.
B) 103.3230.
C) 106.3230.
Meredith alvarez is a junior fixed-income analyst with Canzim asset Management. her su-
pervisor, Stephanie hartson, asks alvarez to review the asset price and payoff data shown in
exhibit 1 to determine whether an arbitrage opportunity exists.
exhibit 1 Price and Payoffs for two risk-Free assets

hartson also shows alvarez data for a bond that trades in three different markets in the
same currency. These data appear in exhibit 2.
exhibit 2 2% Coupon, Five-Year Maturity, annual-Pay bond

hartson asks alvarez to value two bonds (bond C and bond D) using the binomial tree
in exhibit 3. exhibit 4 presents selected data for both bonds.
exhibit 3 binomial interest rate tree with Volatility = 25%

exhibit 4 Selected Data on annual-Pay bonds

hartson tells alvarez that she and her peers have been debating various viewpoints regard-
ing the conditions underlying binomial interest rate trees. The following statements were made
in the course of the debate.
Statement 1: The only requirements needed to create a binomial interest rate tree are
current benchmark interest rates and an assumption about interest rate
volatility.
Statement 2: Potential interest rate volatility in a binomial interest rate tree can be es-
timated using historical interest rate volatility or observed market prices
from interest rate derivatives.
Statement 3: a bond value derived from a binomial interest rate tree with a relatively
high volatility assumption will be different from the value calculated by
discounting the bond's cash flows using current spot rates.
based on data in exhibit 5, hartson asks alvarez to calibrate a binomial interest rate tree
starting with the calculation of implied forward rates shown in exhibit 6.
exhibit 5 Selected Data for a binomial interest rate tree

exhibit 6 Calibration of binomial interest rate

hartson mentions pathwise valuations as another method to value bonds using a binomial
interest rate tree. using the binomial interest rate tree in exhibit 3, alvarez calculates the pos-
sible interest rate paths for bond D shown in exhibit 7.
exhibit 7 interest rate Paths for bond D

before leaving for the day, hartson asks alvarez about the value of using the Monte Carlo
method to simulate a large number of potential interest rate paths to value a bond. alvarez
makes the following statements.
Statement 4: increasing the number of paths increases the estimate's statistical accuracy.
Statement 5: The bond value derived from a Monte Carlo simulation will be closer to
the bond's true fundamental value.
based on exhibits 3 and 4, the price for bond D is closest to:
A) 97.4785.
B) 103.3230.
C) 106.3230.

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
5
The following information relates to Questions
Meredith alvarez is a junior fixed-income analyst with Canzim asset Management. her su-
pervisor, Stephanie hartson, asks alvarez to review the asset price and payoff data shown in
exhibit 1 to determine whether an arbitrage opportunity exists.
exhibit 1 Price and Payoffs for two risk-Free assets

hartson also shows alvarez data for a bond that trades in three different markets in the
same currency. These data appear in exhibit 2.
exhibit 2 2% Coupon, Five-Year Maturity, annual-Pay bond

hartson asks alvarez to value two bonds (bond C and bond D) using the binomial tree
in exhibit 3. exhibit 4 presents selected data for both bonds.
exhibit 3 binomial interest rate tree with Volatility = 25%

exhibit 4 Selected Data on annual-Pay bonds

hartson tells alvarez that she and her peers have been debating various viewpoints regard-
ing the conditions underlying binomial interest rate trees. The following statements were made
in the course of the debate.
Statement 1: The only requirements needed to create a binomial interest rate tree are
current benchmark interest rates and an assumption about interest rate
volatility.
Statement 2: Potential interest rate volatility in a binomial interest rate tree can be es-
timated using historical interest rate volatility or observed market prices
from interest rate derivatives.
Statement 3: a bond value derived from a binomial interest rate tree with a relatively
high volatility assumption will be different from the value calculated by
discounting the bond's cash flows using current spot rates.
based on data in exhibit 5, hartson asks alvarez to calibrate a binomial interest rate tree
starting with the calculation of implied forward rates shown in exhibit 6.
exhibit 5 Selected Data for a binomial interest rate tree

exhibit 6 Calibration of binomial interest rate

hartson mentions pathwise valuations as another method to value bonds using a binomial
interest rate tree. using the binomial interest rate tree in exhibit 3, alvarez calculates the pos-
sible interest rate paths for bond D shown in exhibit 7.
exhibit 7 interest rate Paths for bond D

before leaving for the day, hartson asks alvarez about the value of using the Monte Carlo
method to simulate a large number of potential interest rate paths to value a bond. alvarez
makes the following statements.
Statement 4: increasing the number of paths increases the estimate's statistical accuracy.
Statement 5: The bond value derived from a Monte Carlo simulation will be closer to
the bond's true fundamental value.
which of the various statements regarding binomial interest rate trees is correct?
A) Statement 1
B) Statement 2
C) Statement 3
Meredith alvarez is a junior fixed-income analyst with Canzim asset Management. her su-
pervisor, Stephanie hartson, asks alvarez to review the asset price and payoff data shown in
exhibit 1 to determine whether an arbitrage opportunity exists.
exhibit 1 Price and Payoffs for two risk-Free assets

hartson also shows alvarez data for a bond that trades in three different markets in the
same currency. These data appear in exhibit 2.
exhibit 2 2% Coupon, Five-Year Maturity, annual-Pay bond

hartson asks alvarez to value two bonds (bond C and bond D) using the binomial tree
in exhibit 3. exhibit 4 presents selected data for both bonds.
exhibit 3 binomial interest rate tree with Volatility = 25%

exhibit 4 Selected Data on annual-Pay bonds

hartson tells alvarez that she and her peers have been debating various viewpoints regard-
ing the conditions underlying binomial interest rate trees. The following statements were made
in the course of the debate.
Statement 1: The only requirements needed to create a binomial interest rate tree are
current benchmark interest rates and an assumption about interest rate
volatility.
Statement 2: Potential interest rate volatility in a binomial interest rate tree can be es-
timated using historical interest rate volatility or observed market prices
from interest rate derivatives.
Statement 3: a bond value derived from a binomial interest rate tree with a relatively
high volatility assumption will be different from the value calculated by
discounting the bond's cash flows using current spot rates.
based on data in exhibit 5, hartson asks alvarez to calibrate a binomial interest rate tree
starting with the calculation of implied forward rates shown in exhibit 6.
exhibit 5 Selected Data for a binomial interest rate tree

exhibit 6 Calibration of binomial interest rate

hartson mentions pathwise valuations as another method to value bonds using a binomial
interest rate tree. using the binomial interest rate tree in exhibit 3, alvarez calculates the pos-
sible interest rate paths for bond D shown in exhibit 7.
exhibit 7 interest rate Paths for bond D

before leaving for the day, hartson asks alvarez about the value of using the Monte Carlo
method to simulate a large number of potential interest rate paths to value a bond. alvarez
makes the following statements.
Statement 4: increasing the number of paths increases the estimate's statistical accuracy.
Statement 5: The bond value derived from a Monte Carlo simulation will be closer to
the bond's true fundamental value.
which of the various statements regarding binomial interest rate trees is correct?
A) Statement 1
B) Statement 2
C) Statement 3

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
6
The following information relates to Questions 1-6
katrina black, portfolio manager at Coral bond Management, ltd., is conducting a training
session with alex Sun, a junior analyst in the fixed income department. black wants to ex-
plain to Sun the arbitrage-free valuation framework used by the firm. black presents Sun with
exhibit 1, showing a fictitious bond being traded on three exchanges, and asks Sun to identify
the arbitrage opportunity of the bond. Sun agrees to ignore transaction costs in his analysis.
exhibit 1 Three-Year, €100 par, 3.00% Coupon, annual-Pay option-Free bond
 black shows Sun some exhibits that were part of a recent presentation. exhibit 3 presents
black shows Sun some exhibits that were part of a recent presentation. exhibit 3 presents
most of the data of a binomial lognormal interest rate tree fit to the yield curve shown in ex-
hibit 2. exhibit 4 presents most of the data of the implied values for a four-year, option-free,
annual-pay bond with a 2.5% coupon based on the information in exhibit 3.
exhibit 2 Yield to Maturity Par rates for one-, two-, and Three-Year annual-Pay
option-Free bonds
 exhibit 3 binomial interest rate tree Fit to the Yield Curve (Volatility = 10%)
exhibit 3 binomial interest rate tree Fit to the Yield Curve (Volatility = 10%)
 exhibit 4 implied Values (in euros) for a 2.5%, Four-Year, option-Free, annual-Pay bond
exhibit 4 implied Values (in euros) for a 2.5%, Four-Year, option-Free, annual-Pay bond
based on exhibit 3
 black asks about the missing data in exhibits 3 and 4 and directs Sun to complete the
black asks about the missing data in exhibits 3 and 4 and directs Sun to complete the
following tasks related to those exhibits:
task 1 test that the binomial interest tree has been properly calibrated to be
arbitrage-free.
task 2 Develop a spreadsheet model to calculate pathwise valuations. to test the ac-
curacy of the spreadsheet, use the data in exhibit 3 and calculate the value of
the bond if it takes a path of lowest rates in Year 1 and Year 2 and the second
lowest rate in Year 3.
task 3 identify a type of bond where the Monte Carlo calibration method should
be used in place of the binomial interest rate method.
task 4 update exhibit 3 to reflect the current volatility, which is now 15%.
based on exhibits 1 and 2, the exchange that reflects the arbitrage-free price of the bond is:
A) eurex.
B) Frankfurt.
C) nYSe euronext.
katrina black, portfolio manager at Coral bond Management, ltd., is conducting a training
session with alex Sun, a junior analyst in the fixed income department. black wants to ex-
plain to Sun the arbitrage-free valuation framework used by the firm. black presents Sun with
exhibit 1, showing a fictitious bond being traded on three exchanges, and asks Sun to identify
the arbitrage opportunity of the bond. Sun agrees to ignore transaction costs in his analysis.
exhibit 1 Three-Year, €100 par, 3.00% Coupon, annual-Pay option-Free bond
 black shows Sun some exhibits that were part of a recent presentation. exhibit 3 presents
black shows Sun some exhibits that were part of a recent presentation. exhibit 3 presents most of the data of a binomial lognormal interest rate tree fit to the yield curve shown in ex-
hibit 2. exhibit 4 presents most of the data of the implied values for a four-year, option-free,
annual-pay bond with a 2.5% coupon based on the information in exhibit 3.
exhibit 2 Yield to Maturity Par rates for one-, two-, and Three-Year annual-Pay
option-Free bonds
 exhibit 3 binomial interest rate tree Fit to the Yield Curve (Volatility = 10%)
exhibit 3 binomial interest rate tree Fit to the Yield Curve (Volatility = 10%) exhibit 4 implied Values (in euros) for a 2.5%, Four-Year, option-Free, annual-Pay bond
exhibit 4 implied Values (in euros) for a 2.5%, Four-Year, option-Free, annual-Pay bond based on exhibit 3
 black asks about the missing data in exhibits 3 and 4 and directs Sun to complete the
black asks about the missing data in exhibits 3 and 4 and directs Sun to complete the following tasks related to those exhibits:
task 1 test that the binomial interest tree has been properly calibrated to be
arbitrage-free.
task 2 Develop a spreadsheet model to calculate pathwise valuations. to test the ac-
curacy of the spreadsheet, use the data in exhibit 3 and calculate the value of
the bond if it takes a path of lowest rates in Year 1 and Year 2 and the second
lowest rate in Year 3.
task 3 identify a type of bond where the Monte Carlo calibration method should
be used in place of the binomial interest rate method.
task 4 update exhibit 3 to reflect the current volatility, which is now 15%.
based on exhibits 1 and 2, the exchange that reflects the arbitrage-free price of the bond is:
A) eurex.
B) Frankfurt.
C) nYSe euronext.

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
7
The following information relates to Questions
Meredith alvarez is a junior fixed-income analyst with Canzim asset Management. her su-
pervisor, Stephanie hartson, asks alvarez to review the asset price and payoff data shown in
exhibit 1 to determine whether an arbitrage opportunity exists.
exhibit 1 Price and Payoffs for two risk-Free assets

hartson also shows alvarez data for a bond that trades in three different markets in the
same currency. These data appear in exhibit 2.
exhibit 2 2% Coupon, Five-Year Maturity, annual-Pay bond

hartson asks alvarez to value two bonds (bond C and bond D) using the binomial tree
in exhibit 3. exhibit 4 presents selected data for both bonds.
exhibit 3 binomial interest rate tree with Volatility = 25%

exhibit 4 Selected Data on annual-Pay bonds

hartson tells alvarez that she and her peers have been debating various viewpoints regard-
ing the conditions underlying binomial interest rate trees. The following statements were made
in the course of the debate.
Statement 1: The only requirements needed to create a binomial interest rate tree are
current benchmark interest rates and an assumption about interest rate
volatility.
Statement 2: Potential interest rate volatility in a binomial interest rate tree can be es-
timated using historical interest rate volatility or observed market prices
from interest rate derivatives.
Statement 3: a bond value derived from a binomial interest rate tree with a relatively
high volatility assumption will be different from the value calculated by
discounting the bond's cash flows using current spot rates.
based on data in exhibit 5, hartson asks alvarez to calibrate a binomial interest rate tree
starting with the calculation of implied forward rates shown in exhibit 6.
exhibit 5 Selected Data for a binomial interest rate tree

exhibit 6 Calibration of binomial interest rate

hartson mentions pathwise valuations as another method to value bonds using a binomial
interest rate tree. using the binomial interest rate tree in exhibit 3, alvarez calculates the pos-
sible interest rate paths for bond D shown in exhibit 7.
exhibit 7 interest rate Paths for bond D

before leaving for the day, hartson asks alvarez about the value of using the Monte Carlo
method to simulate a large number of potential interest rate paths to value a bond. alvarez
makes the following statements.
Statement 4: increasing the number of paths increases the estimate's statistical accuracy.
Statement 5: The bond value derived from a Monte Carlo simulation will be closer to
the bond's true fundamental value.
based on exhibits 3 and 4, the value of bond C at the upper node at time 1 is closest to:
A) 97.1957.
B) 99.6255.
C) 102.1255.
Meredith alvarez is a junior fixed-income analyst with Canzim asset Management. her su-
pervisor, Stephanie hartson, asks alvarez to review the asset price and payoff data shown in
exhibit 1 to determine whether an arbitrage opportunity exists.
exhibit 1 Price and Payoffs for two risk-Free assets

hartson also shows alvarez data for a bond that trades in three different markets in the
same currency. These data appear in exhibit 2.
exhibit 2 2% Coupon, Five-Year Maturity, annual-Pay bond

hartson asks alvarez to value two bonds (bond C and bond D) using the binomial tree
in exhibit 3. exhibit 4 presents selected data for both bonds.
exhibit 3 binomial interest rate tree with Volatility = 25%

exhibit 4 Selected Data on annual-Pay bonds

hartson tells alvarez that she and her peers have been debating various viewpoints regard-
ing the conditions underlying binomial interest rate trees. The following statements were made
in the course of the debate.
Statement 1: The only requirements needed to create a binomial interest rate tree are
current benchmark interest rates and an assumption about interest rate
volatility.
Statement 2: Potential interest rate volatility in a binomial interest rate tree can be es-
timated using historical interest rate volatility or observed market prices
from interest rate derivatives.
Statement 3: a bond value derived from a binomial interest rate tree with a relatively
high volatility assumption will be different from the value calculated by
discounting the bond's cash flows using current spot rates.
based on data in exhibit 5, hartson asks alvarez to calibrate a binomial interest rate tree
starting with the calculation of implied forward rates shown in exhibit 6.
exhibit 5 Selected Data for a binomial interest rate tree

exhibit 6 Calibration of binomial interest rate

hartson mentions pathwise valuations as another method to value bonds using a binomial
interest rate tree. using the binomial interest rate tree in exhibit 3, alvarez calculates the pos-
sible interest rate paths for bond D shown in exhibit 7.
exhibit 7 interest rate Paths for bond D

before leaving for the day, hartson asks alvarez about the value of using the Monte Carlo
method to simulate a large number of potential interest rate paths to value a bond. alvarez
makes the following statements.
Statement 4: increasing the number of paths increases the estimate's statistical accuracy.
Statement 5: The bond value derived from a Monte Carlo simulation will be closer to
the bond's true fundamental value.
based on exhibits 3 and 4, the value of bond C at the upper node at time 1 is closest to:
A) 97.1957.
B) 99.6255.
C) 102.1255.

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
8
The following information relates to Questions 1-6
katrina black, portfolio manager at Coral bond Management, ltd., is conducting a training
session with alex Sun, a junior analyst in the fixed income department. black wants to ex-
plain to Sun the arbitrage-free valuation framework used by the firm. black presents Sun with
exhibit 1, showing a fictitious bond being traded on three exchanges, and asks Sun to identify
the arbitrage opportunity of the bond. Sun agrees to ignore transaction costs in his analysis.
exhibit 1 Three-Year, €100 par, 3.00% Coupon, annual-Pay option-Free bond
 black shows Sun some exhibits that were part of a recent presentation. exhibit 3 presents
black shows Sun some exhibits that were part of a recent presentation. exhibit 3 presents
most of the data of a binomial lognormal interest rate tree fit to the yield curve shown in ex-
hibit 2. exhibit 4 presents most of the data of the implied values for a four-year, option-free,
annual-pay bond with a 2.5% coupon based on the information in exhibit 3.
exhibit 2 Yield to Maturity Par rates for one-, two-, and Three-Year annual-Pay
option-Free bonds
 exhibit 3 binomial interest rate tree Fit to the Yield Curve (Volatility = 10%)
exhibit 3 binomial interest rate tree Fit to the Yield Curve (Volatility = 10%)
 exhibit 4 implied Values (in euros) for a 2.5%, Four-Year, option-Free, annual-Pay bond
exhibit 4 implied Values (in euros) for a 2.5%, Four-Year, option-Free, annual-Pay bond
based on exhibit 3
 black asks about the missing data in exhibits 3 and 4 and directs Sun to complete the
black asks about the missing data in exhibits 3 and 4 and directs Sun to complete the
following tasks related to those exhibits:
task 1 test that the binomial interest tree has been properly calibrated to be
arbitrage-free.
task 2 Develop a spreadsheet model to calculate pathwise valuations. to test the ac-
curacy of the spreadsheet, use the data in exhibit 3 and calculate the value of
the bond if it takes a path of lowest rates in Year 1 and Year 2 and the second
lowest rate in Year 3.
task 3 identify a type of bond where the Monte Carlo calibration method should
be used in place of the binomial interest rate method.
task 4 update exhibit 3 to reflect the current volatility, which is now 15%.
a benefit of performing task 1 is that it:
A) enables the model to price bonds with embedded options.
B) identifies benchmark bonds that have been mispriced by the market.
C) allows investors to realize arbitrage profits through stripping and reconstitution.
katrina black, portfolio manager at Coral bond Management, ltd., is conducting a training
session with alex Sun, a junior analyst in the fixed income department. black wants to ex-
plain to Sun the arbitrage-free valuation framework used by the firm. black presents Sun with
exhibit 1, showing a fictitious bond being traded on three exchanges, and asks Sun to identify
the arbitrage opportunity of the bond. Sun agrees to ignore transaction costs in his analysis.
exhibit 1 Three-Year, €100 par, 3.00% Coupon, annual-Pay option-Free bond
 black shows Sun some exhibits that were part of a recent presentation. exhibit 3 presents
black shows Sun some exhibits that were part of a recent presentation. exhibit 3 presents most of the data of a binomial lognormal interest rate tree fit to the yield curve shown in ex-
hibit 2. exhibit 4 presents most of the data of the implied values for a four-year, option-free,
annual-pay bond with a 2.5% coupon based on the information in exhibit 3.
exhibit 2 Yield to Maturity Par rates for one-, two-, and Three-Year annual-Pay
option-Free bonds
 exhibit 3 binomial interest rate tree Fit to the Yield Curve (Volatility = 10%)
exhibit 3 binomial interest rate tree Fit to the Yield Curve (Volatility = 10%) exhibit 4 implied Values (in euros) for a 2.5%, Four-Year, option-Free, annual-Pay bond
exhibit 4 implied Values (in euros) for a 2.5%, Four-Year, option-Free, annual-Pay bond based on exhibit 3
 black asks about the missing data in exhibits 3 and 4 and directs Sun to complete the
black asks about the missing data in exhibits 3 and 4 and directs Sun to complete the following tasks related to those exhibits:
task 1 test that the binomial interest tree has been properly calibrated to be
arbitrage-free.
task 2 Develop a spreadsheet model to calculate pathwise valuations. to test the ac-
curacy of the spreadsheet, use the data in exhibit 3 and calculate the value of
the bond if it takes a path of lowest rates in Year 1 and Year 2 and the second
lowest rate in Year 3.
task 3 identify a type of bond where the Monte Carlo calibration method should
be used in place of the binomial interest rate method.
task 4 update exhibit 3 to reflect the current volatility, which is now 15%.
a benefit of performing task 1 is that it:
A) enables the model to price bonds with embedded options.
B) identifies benchmark bonds that have been mispriced by the market.
C) allows investors to realize arbitrage profits through stripping and reconstitution.

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
9
The following information relates to Questions
Meredith alvarez is a junior fixed-income analyst with Canzim asset Management. her su-
pervisor, Stephanie hartson, asks alvarez to review the asset price and payoff data shown in
exhibit 1 to determine whether an arbitrage opportunity exists.
exhibit 1 Price and Payoffs for two risk-Free assets

hartson also shows alvarez data for a bond that trades in three different markets in the
same currency. These data appear in exhibit 2.
exhibit 2 2% Coupon, Five-Year Maturity, annual-Pay bond

hartson asks alvarez to value two bonds (bond C and bond D) using the binomial tree
in exhibit 3. exhibit 4 presents selected data for both bonds.
exhibit 3 binomial interest rate tree with Volatility = 25%

exhibit 4 Selected Data on annual-Pay bonds

hartson tells alvarez that she and her peers have been debating various viewpoints regard-
ing the conditions underlying binomial interest rate trees. The following statements were made
in the course of the debate.
Statement 1: The only requirements needed to create a binomial interest rate tree are
current benchmark interest rates and an assumption about interest rate
volatility.
Statement 2: Potential interest rate volatility in a binomial interest rate tree can be es-
timated using historical interest rate volatility or observed market prices
from interest rate derivatives.
Statement 3: a bond value derived from a binomial interest rate tree with a relatively
high volatility assumption will be different from the value calculated by
discounting the bond's cash flows using current spot rates.
based on data in exhibit 5, hartson asks alvarez to calibrate a binomial interest rate tree
starting with the calculation of implied forward rates shown in exhibit 6.
exhibit 5 Selected Data for a binomial interest rate tree

exhibit 6 Calibration of binomial interest rate

hartson mentions pathwise valuations as another method to value bonds using a binomial
interest rate tree. using the binomial interest rate tree in exhibit 3, alvarez calculates the pos-
sible interest rate paths for bond D shown in exhibit 7.
exhibit 7 interest rate Paths for bond D

before leaving for the day, hartson asks alvarez about the value of using the Monte Carlo
method to simulate a large number of potential interest rate paths to value a bond. alvarez
makes the following statements.
Statement 4: increasing the number of paths increases the estimate's statistical accuracy.
Statement 5: The bond value derived from a Monte Carlo simulation will be closer to
the bond's true fundamental value.
based on the data in exhibit 2, the most profitable arbitrage opportunity would be to buy the bond in:
A) Mumbai and sell it in hong kong.
B) hong kong and sell it in new York.
C) new York and sell it in hong kong.
Meredith alvarez is a junior fixed-income analyst with Canzim asset Management. her su-
pervisor, Stephanie hartson, asks alvarez to review the asset price and payoff data shown in
exhibit 1 to determine whether an arbitrage opportunity exists.
exhibit 1 Price and Payoffs for two risk-Free assets

hartson also shows alvarez data for a bond that trades in three different markets in the
same currency. These data appear in exhibit 2.
exhibit 2 2% Coupon, Five-Year Maturity, annual-Pay bond

hartson asks alvarez to value two bonds (bond C and bond D) using the binomial tree
in exhibit 3. exhibit 4 presents selected data for both bonds.
exhibit 3 binomial interest rate tree with Volatility = 25%

exhibit 4 Selected Data on annual-Pay bonds

hartson tells alvarez that she and her peers have been debating various viewpoints regard-
ing the conditions underlying binomial interest rate trees. The following statements were made
in the course of the debate.
Statement 1: The only requirements needed to create a binomial interest rate tree are
current benchmark interest rates and an assumption about interest rate
volatility.
Statement 2: Potential interest rate volatility in a binomial interest rate tree can be es-
timated using historical interest rate volatility or observed market prices
from interest rate derivatives.
Statement 3: a bond value derived from a binomial interest rate tree with a relatively
high volatility assumption will be different from the value calculated by
discounting the bond's cash flows using current spot rates.
based on data in exhibit 5, hartson asks alvarez to calibrate a binomial interest rate tree
starting with the calculation of implied forward rates shown in exhibit 6.
exhibit 5 Selected Data for a binomial interest rate tree

exhibit 6 Calibration of binomial interest rate

hartson mentions pathwise valuations as another method to value bonds using a binomial
interest rate tree. using the binomial interest rate tree in exhibit 3, alvarez calculates the pos-
sible interest rate paths for bond D shown in exhibit 7.
exhibit 7 interest rate Paths for bond D

before leaving for the day, hartson asks alvarez about the value of using the Monte Carlo
method to simulate a large number of potential interest rate paths to value a bond. alvarez
makes the following statements.
Statement 4: increasing the number of paths increases the estimate's statistical accuracy.
Statement 5: The bond value derived from a Monte Carlo simulation will be closer to
the bond's true fundamental value.
based on the data in exhibit 2, the most profitable arbitrage opportunity would be to buy the bond in:
A) Mumbai and sell it in hong kong.
B) hong kong and sell it in new York.
C) new York and sell it in hong kong.

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
10
The following information relates to Questions
betty tatton is a fixed income analyst with the hedge fund Sailboat asset Management (SaM).
SaM invests in a variety of global fixed-income strategies, including fixed-income arbitrage.
tatton is responsible for pricing individual investments and analyzing market data to assess the
opportunity for arbitrage. She uses two methods to value bonds:
Method 1: Discount each year's cash flow separately using the appropriate interest rate
curve.
Method 2: build and use a binomial interest rate tree.
tatton compiles pricing data for a list of annual pay bonds (exhibit 1). each of the bonds
will mature in two years, and tatton considers the bonds as being risk-free; both the one-year
and two-year benchmark spot rates are 2%. tatton calculates the arbitrage-free prices and
identifies an arbitrage opportunity to recommend to her team.
exhibit 1 Market Data for Selected bonds

next, tatton uses the benchmark yield curve provided in exhibit 2 to consider arbitrage
opportunities of both option-free corporate bonds and corporate bonds with embedded op-
tions. The benchmark bonds in exhibit 2 pay coupons annually, and the bonds are priced
at par.
exhibit 2 benchmark Par Curve

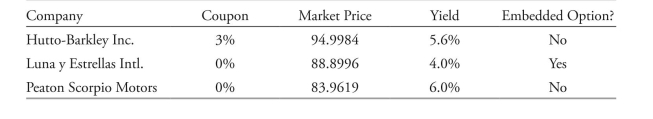
tatton then identifies three mispriced three-year annual-pay bonds and compiles data on
the bonds (see exhibit 3).
exhibit 3 Market Data of annual-Pay Corporate bonds
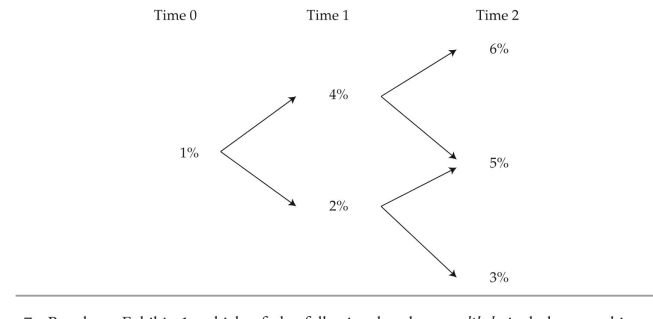
last, tatton identifies two mispriced Swiss bonds, bond x, a three-year bond, and bond
Y, a five-year bond. both are annual-pay bonds with a coupon rate of 6%. to calculate the
bonds' values, tatton devises the first three years of the interest rate lognormal tree presented
in exhibit 4 using historical interest rate volatility data. tatton considers how these data would
change if implied volatility, which is higher than historical volatility, were used instead.
exhibit 4 interest rate tree; Forward rates based on Swiss Market
Method 1 would most likely not be an appropriate valuation technique for the bond issued by:
A) hutto-barkley inc.
B) luna y estrellas intl.
C) Peaton Scorpio Motors.
betty tatton is a fixed income analyst with the hedge fund Sailboat asset Management (SaM).
SaM invests in a variety of global fixed-income strategies, including fixed-income arbitrage.
tatton is responsible for pricing individual investments and analyzing market data to assess the
opportunity for arbitrage. She uses two methods to value bonds:
Method 1: Discount each year's cash flow separately using the appropriate interest rate
curve.
Method 2: build and use a binomial interest rate tree.
tatton compiles pricing data for a list of annual pay bonds (exhibit 1). each of the bonds
will mature in two years, and tatton considers the bonds as being risk-free; both the one-year
and two-year benchmark spot rates are 2%. tatton calculates the arbitrage-free prices and
identifies an arbitrage opportunity to recommend to her team.
exhibit 1 Market Data for Selected bonds

next, tatton uses the benchmark yield curve provided in exhibit 2 to consider arbitrage
opportunities of both option-free corporate bonds and corporate bonds with embedded op-
tions. The benchmark bonds in exhibit 2 pay coupons annually, and the bonds are priced
at par.
exhibit 2 benchmark Par Curve

tatton then identifies three mispriced three-year annual-pay bonds and compiles data on
the bonds (see exhibit 3).
exhibit 3 Market Data of annual-Pay Corporate bonds

last, tatton identifies two mispriced Swiss bonds, bond x, a three-year bond, and bond
Y, a five-year bond. both are annual-pay bonds with a coupon rate of 6%. to calculate the
bonds' values, tatton devises the first three years of the interest rate lognormal tree presented
in exhibit 4 using historical interest rate volatility data. tatton considers how these data would
change if implied volatility, which is higher than historical volatility, were used instead.
exhibit 4 interest rate tree; Forward rates based on Swiss Market

Method 1 would most likely not be an appropriate valuation technique for the bond issued by:
A) hutto-barkley inc.
B) luna y estrellas intl.
C) Peaton Scorpio Motors.

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
11
The following information relates to Questions
Meredith alvarez is a junior fixed-income analyst with Canzim asset Management. her su-
pervisor, Stephanie hartson, asks alvarez to review the asset price and payoff data shown in
exhibit 1 to determine whether an arbitrage opportunity exists.
exhibit 1 Price and Payoffs for two risk-Free assets

hartson also shows alvarez data for a bond that trades in three different markets in the
same currency. These data appear in exhibit 2.
exhibit 2 2% Coupon, Five-Year Maturity, annual-Pay bond

hartson asks alvarez to value two bonds (bond C and bond D) using the binomial tree
in exhibit 3. exhibit 4 presents selected data for both bonds.
exhibit 3 binomial interest rate tree with Volatility = 25%

exhibit 4 Selected Data on annual-Pay bonds

hartson tells alvarez that she and her peers have been debating various viewpoints regard-
ing the conditions underlying binomial interest rate trees. The following statements were made
in the course of the debate.
Statement 1: The only requirements needed to create a binomial interest rate tree are
current benchmark interest rates and an assumption about interest rate
volatility.
Statement 2: Potential interest rate volatility in a binomial interest rate tree can be es-
timated using historical interest rate volatility or observed market prices
from interest rate derivatives.
Statement 3: a bond value derived from a binomial interest rate tree with a relatively
high volatility assumption will be different from the value calculated by
discounting the bond's cash flows using current spot rates.
based on data in exhibit 5, hartson asks alvarez to calibrate a binomial interest rate tree
starting with the calculation of implied forward rates shown in exhibit 6.
exhibit 5 Selected Data for a binomial interest rate tree

exhibit 6 Calibration of binomial interest rate

hartson mentions pathwise valuations as another method to value bonds using a binomial
interest rate tree. using the binomial interest rate tree in exhibit 3, alvarez calculates the pos-
sible interest rate paths for bond D shown in exhibit 7.
exhibit 7 interest rate Paths for bond D

before leaving for the day, hartson asks alvarez about the value of using the Monte Carlo
method to simulate a large number of potential interest rate paths to value a bond. alvarez
makes the following statements.
Statement 4: increasing the number of paths increases the estimate's statistical accuracy.
Statement 5: The bond value derived from a Monte Carlo simulation will be closer to
the bond's true fundamental value.
based on exhibits 5 and 6, the value of the lower one-period forward rate is closest to:
A) 3.5122%.
B) 3.5400%.
C) 4.8037%.
Meredith alvarez is a junior fixed-income analyst with Canzim asset Management. her su-
pervisor, Stephanie hartson, asks alvarez to review the asset price and payoff data shown in
exhibit 1 to determine whether an arbitrage opportunity exists.
exhibit 1 Price and Payoffs for two risk-Free assets

hartson also shows alvarez data for a bond that trades in three different markets in the
same currency. These data appear in exhibit 2.
exhibit 2 2% Coupon, Five-Year Maturity, annual-Pay bond

hartson asks alvarez to value two bonds (bond C and bond D) using the binomial tree
in exhibit 3. exhibit 4 presents selected data for both bonds.
exhibit 3 binomial interest rate tree with Volatility = 25%

exhibit 4 Selected Data on annual-Pay bonds

hartson tells alvarez that she and her peers have been debating various viewpoints regard-
ing the conditions underlying binomial interest rate trees. The following statements were made
in the course of the debate.
Statement 1: The only requirements needed to create a binomial interest rate tree are
current benchmark interest rates and an assumption about interest rate
volatility.
Statement 2: Potential interest rate volatility in a binomial interest rate tree can be es-
timated using historical interest rate volatility or observed market prices
from interest rate derivatives.
Statement 3: a bond value derived from a binomial interest rate tree with a relatively
high volatility assumption will be different from the value calculated by
discounting the bond's cash flows using current spot rates.
based on data in exhibit 5, hartson asks alvarez to calibrate a binomial interest rate tree
starting with the calculation of implied forward rates shown in exhibit 6.
exhibit 5 Selected Data for a binomial interest rate tree

exhibit 6 Calibration of binomial interest rate

hartson mentions pathwise valuations as another method to value bonds using a binomial
interest rate tree. using the binomial interest rate tree in exhibit 3, alvarez calculates the pos-
sible interest rate paths for bond D shown in exhibit 7.
exhibit 7 interest rate Paths for bond D

before leaving for the day, hartson asks alvarez about the value of using the Monte Carlo
method to simulate a large number of potential interest rate paths to value a bond. alvarez
makes the following statements.
Statement 4: increasing the number of paths increases the estimate's statistical accuracy.
Statement 5: The bond value derived from a Monte Carlo simulation will be closer to
the bond's true fundamental value.
based on exhibits 5 and 6, the value of the lower one-period forward rate is closest to:
A) 3.5122%.
B) 3.5400%.
C) 4.8037%.

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
12
The following information relates to Questions
betty tatton is a fixed income analyst with the hedge fund Sailboat asset Management (SaM).
SaM invests in a variety of global fixed-income strategies, including fixed-income arbitrage.
tatton is responsible for pricing individual investments and analyzing market data to assess the
opportunity for arbitrage. She uses two methods to value bonds:
Method 1: Discount each year's cash flow separately using the appropriate interest rate
curve.
Method 2: build and use a binomial interest rate tree.
tatton compiles pricing data for a list of annual pay bonds (exhibit 1). each of the bonds
will mature in two years, and tatton considers the bonds as being risk-free; both the one-year
and two-year benchmark spot rates are 2%. tatton calculates the arbitrage-free prices and
identifies an arbitrage opportunity to recommend to her team.
exhibit 1 Market Data for Selected bonds

next, tatton uses the benchmark yield curve provided in exhibit 2 to consider arbitrage
opportunities of both option-free corporate bonds and corporate bonds with embedded op-
tions. The benchmark bonds in exhibit 2 pay coupons annually, and the bonds are priced
at par.
exhibit 2 benchmark Par Curve

tatton then identifies three mispriced three-year annual-pay bonds and compiles data on
the bonds (see exhibit 3).
exhibit 3 Market Data of annual-Pay Corporate bonds

last, tatton identifies two mispriced Swiss bonds, bond x, a three-year bond, and bond
Y, a five-year bond. both are annual-pay bonds with a coupon rate of 6%. to calculate the
bonds' values, tatton devises the first three years of the interest rate lognormal tree presented
in exhibit 4 using historical interest rate volatility data. tatton considers how these data would
change if implied volatility, which is higher than historical volatility, were used instead.
exhibit 4 interest rate tree; Forward rates based on Swiss Market

based on exhibit 1, which of the following bonds most likely includes an arbitrage opportunity?
A) bond a
B) bond b
C) bond C
betty tatton is a fixed income analyst with the hedge fund Sailboat asset Management (SaM).
SaM invests in a variety of global fixed-income strategies, including fixed-income arbitrage.
tatton is responsible for pricing individual investments and analyzing market data to assess the
opportunity for arbitrage. She uses two methods to value bonds:
Method 1: Discount each year's cash flow separately using the appropriate interest rate
curve.
Method 2: build and use a binomial interest rate tree.
tatton compiles pricing data for a list of annual pay bonds (exhibit 1). each of the bonds
will mature in two years, and tatton considers the bonds as being risk-free; both the one-year
and two-year benchmark spot rates are 2%. tatton calculates the arbitrage-free prices and
identifies an arbitrage opportunity to recommend to her team.
exhibit 1 Market Data for Selected bonds

next, tatton uses the benchmark yield curve provided in exhibit 2 to consider arbitrage
opportunities of both option-free corporate bonds and corporate bonds with embedded op-
tions. The benchmark bonds in exhibit 2 pay coupons annually, and the bonds are priced
at par.
exhibit 2 benchmark Par Curve

tatton then identifies three mispriced three-year annual-pay bonds and compiles data on
the bonds (see exhibit 3).
exhibit 3 Market Data of annual-Pay Corporate bonds

last, tatton identifies two mispriced Swiss bonds, bond x, a three-year bond, and bond
Y, a five-year bond. both are annual-pay bonds with a coupon rate of 6%. to calculate the
bonds' values, tatton devises the first three years of the interest rate lognormal tree presented
in exhibit 4 using historical interest rate volatility data. tatton considers how these data would
change if implied volatility, which is higher than historical volatility, were used instead.
exhibit 4 interest rate tree; Forward rates based on Swiss Market

based on exhibit 1, which of the following bonds most likely includes an arbitrage opportunity?
A) bond a
B) bond b
C) bond C

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
13
The following information relates to Questions
betty tatton is a fixed income analyst with the hedge fund Sailboat asset Management (SaM).
SaM invests in a variety of global fixed-income strategies, including fixed-income arbitrage.
tatton is responsible for pricing individual investments and analyzing market data to assess the
opportunity for arbitrage. She uses two methods to value bonds:
Method 1: Discount each year's cash flow separately using the appropriate interest rate
curve.
Method 2: build and use a binomial interest rate tree.
tatton compiles pricing data for a list of annual pay bonds (exhibit 1). each of the bonds
will mature in two years, and tatton considers the bonds as being risk-free; both the one-year
and two-year benchmark spot rates are 2%. tatton calculates the arbitrage-free prices and
identifies an arbitrage opportunity to recommend to her team.
exhibit 1 Market Data for Selected bonds

next, tatton uses the benchmark yield curve provided in exhibit 2 to consider arbitrage
opportunities of both option-free corporate bonds and corporate bonds with embedded op-
tions. The benchmark bonds in exhibit 2 pay coupons annually, and the bonds are priced
at par.
exhibit 2 benchmark Par Curve

tatton then identifies three mispriced three-year annual-pay bonds and compiles data on
the bonds (see exhibit 3).
exhibit 3 Market Data of annual-Pay Corporate bonds

last, tatton identifies two mispriced Swiss bonds, bond x, a three-year bond, and bond
Y, a five-year bond. both are annual-pay bonds with a coupon rate of 6%. to calculate the
bonds' values, tatton devises the first three years of the interest rate lognormal tree presented
in exhibit 4 using historical interest rate volatility data. tatton considers how these data would
change if implied volatility, which is higher than historical volatility, were used instead.
exhibit 4 interest rate tree; Forward rates based on Swiss Market

based on exhibit 4 and using Method 2, the correct price for bond x is closest to:
A) 97.2998.
B) 109.0085.
C) 115.0085.
betty tatton is a fixed income analyst with the hedge fund Sailboat asset Management (SaM).
SaM invests in a variety of global fixed-income strategies, including fixed-income arbitrage.
tatton is responsible for pricing individual investments and analyzing market data to assess the
opportunity for arbitrage. She uses two methods to value bonds:
Method 1: Discount each year's cash flow separately using the appropriate interest rate
curve.
Method 2: build and use a binomial interest rate tree.
tatton compiles pricing data for a list of annual pay bonds (exhibit 1). each of the bonds
will mature in two years, and tatton considers the bonds as being risk-free; both the one-year
and two-year benchmark spot rates are 2%. tatton calculates the arbitrage-free prices and
identifies an arbitrage opportunity to recommend to her team.
exhibit 1 Market Data for Selected bonds

next, tatton uses the benchmark yield curve provided in exhibit 2 to consider arbitrage
opportunities of both option-free corporate bonds and corporate bonds with embedded op-
tions. The benchmark bonds in exhibit 2 pay coupons annually, and the bonds are priced
at par.
exhibit 2 benchmark Par Curve

tatton then identifies three mispriced three-year annual-pay bonds and compiles data on
the bonds (see exhibit 3).
exhibit 3 Market Data of annual-Pay Corporate bonds

last, tatton identifies two mispriced Swiss bonds, bond x, a three-year bond, and bond
Y, a five-year bond. both are annual-pay bonds with a coupon rate of 6%. to calculate the
bonds' values, tatton devises the first three years of the interest rate lognormal tree presented
in exhibit 4 using historical interest rate volatility data. tatton considers how these data would
change if implied volatility, which is higher than historical volatility, were used instead.
exhibit 4 interest rate tree; Forward rates based on Swiss Market

based on exhibit 4 and using Method 2, the correct price for bond x is closest to:
A) 97.2998.
B) 109.0085.
C) 115.0085.

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
14
The following information relates to Questions
betty tatton is a fixed income analyst with the hedge fund Sailboat asset Management (SaM).
SaM invests in a variety of global fixed-income strategies, including fixed-income arbitrage.
tatton is responsible for pricing individual investments and analyzing market data to assess the
opportunity for arbitrage. She uses two methods to value bonds:
Method 1: Discount each year's cash flow separately using the appropriate interest rate
curve.
Method 2: build and use a binomial interest rate tree.
tatton compiles pricing data for a list of annual pay bonds (exhibit 1). each of the bonds
will mature in two years, and tatton considers the bonds as being risk-free; both the one-year
and two-year benchmark spot rates are 2%. tatton calculates the arbitrage-free prices and
identifies an arbitrage opportunity to recommend to her team.
exhibit 1 Market Data for Selected bonds

next, tatton uses the benchmark yield curve provided in exhibit 2 to consider arbitrage
opportunities of both option-free corporate bonds and corporate bonds with embedded op-
tions. The benchmark bonds in exhibit 2 pay coupons annually, and the bonds are priced
at par.
exhibit 2 benchmark Par Curve

tatton then identifies three mispriced three-year annual-pay bonds and compiles data on
the bonds (see exhibit 3).
exhibit 3 Market Data of annual-Pay Corporate bonds

last, tatton identifies two mispriced Swiss bonds, bond x, a three-year bond, and bond
Y, a five-year bond. both are annual-pay bonds with a coupon rate of 6%. to calculate the
bonds' values, tatton devises the first three years of the interest rate lognormal tree presented
in exhibit 4 using historical interest rate volatility data. tatton considers how these data would
change if implied volatility, which is higher than historical volatility, were used instead.
exhibit 4 interest rate tree; Forward rates based on Swiss Market

based on exhibits 2 and 3 and using Method 1, the amount (in absolute terms) by which the hutto-barkley corporate bond is mispriced is closest to:
A) 0.3368 per 100 of par value.
B) 0.4682 per 100 of par value.
C) 0.5156 per 100 of par value.
betty tatton is a fixed income analyst with the hedge fund Sailboat asset Management (SaM).
SaM invests in a variety of global fixed-income strategies, including fixed-income arbitrage.
tatton is responsible for pricing individual investments and analyzing market data to assess the
opportunity for arbitrage. She uses two methods to value bonds:
Method 1: Discount each year's cash flow separately using the appropriate interest rate
curve.
Method 2: build and use a binomial interest rate tree.
tatton compiles pricing data for a list of annual pay bonds (exhibit 1). each of the bonds
will mature in two years, and tatton considers the bonds as being risk-free; both the one-year
and two-year benchmark spot rates are 2%. tatton calculates the arbitrage-free prices and
identifies an arbitrage opportunity to recommend to her team.
exhibit 1 Market Data for Selected bonds

next, tatton uses the benchmark yield curve provided in exhibit 2 to consider arbitrage
opportunities of both option-free corporate bonds and corporate bonds with embedded op-
tions. The benchmark bonds in exhibit 2 pay coupons annually, and the bonds are priced
at par.
exhibit 2 benchmark Par Curve

tatton then identifies three mispriced three-year annual-pay bonds and compiles data on
the bonds (see exhibit 3).
exhibit 3 Market Data of annual-Pay Corporate bonds

last, tatton identifies two mispriced Swiss bonds, bond x, a three-year bond, and bond
Y, a five-year bond. both are annual-pay bonds with a coupon rate of 6%. to calculate the
bonds' values, tatton devises the first three years of the interest rate lognormal tree presented
in exhibit 4 using historical interest rate volatility data. tatton considers how these data would
change if implied volatility, which is higher than historical volatility, were used instead.
exhibit 4 interest rate tree; Forward rates based on Swiss Market

based on exhibits 2 and 3 and using Method 1, the amount (in absolute terms) by which the hutto-barkley corporate bond is mispriced is closest to:
A) 0.3368 per 100 of par value.
B) 0.4682 per 100 of par value.
C) 0.5156 per 100 of par value.

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
15
The following information relates to Questions 1-6
katrina black, portfolio manager at Coral bond Management, ltd., is conducting a training
session with alex Sun, a junior analyst in the fixed income department. black wants to ex-
plain to Sun the arbitrage-free valuation framework used by the firm. black presents Sun with
exhibit 1, showing a fictitious bond being traded on three exchanges, and asks Sun to identify
the arbitrage opportunity of the bond. Sun agrees to ignore transaction costs in his analysis.
exhibit 1 Three-Year, €100 par, 3.00% Coupon, annual-Pay option-Free bond
 black shows Sun some exhibits that were part of a recent presentation. exhibit 3 presents
black shows Sun some exhibits that were part of a recent presentation. exhibit 3 presents
most of the data of a binomial lognormal interest rate tree fit to the yield curve shown in ex-
hibit 2. exhibit 4 presents most of the data of the implied values for a four-year, option-free,
annual-pay bond with a 2.5% coupon based on the information in exhibit 3.
exhibit 2 Yield to Maturity Par rates for one-, two-, and Three-Year annual-Pay
option-Free bonds
 exhibit 3 binomial interest rate tree Fit to the Yield Curve (Volatility = 10%)
exhibit 3 binomial interest rate tree Fit to the Yield Curve (Volatility = 10%)
 exhibit 4 implied Values (in euros) for a 2.5%, Four-Year, option-Free, annual-Pay bond
exhibit 4 implied Values (in euros) for a 2.5%, Four-Year, option-Free, annual-Pay bond
based on exhibit 3
 black asks about the missing data in exhibits 3 and 4 and directs Sun to complete the
black asks about the missing data in exhibits 3 and 4 and directs Sun to complete the
following tasks related to those exhibits:
task 1 test that the binomial interest tree has been properly calibrated to be
arbitrage-free.
task 2 Develop a spreadsheet model to calculate pathwise valuations. to test the ac-
curacy of the spreadsheet, use the data in exhibit 3 and calculate the value of
the bond if it takes a path of lowest rates in Year 1 and Year 2 and the second
lowest rate in Year 3.
task 3 identify a type of bond where the Monte Carlo calibration method should
be used in place of the binomial interest rate method.
task 4 update exhibit 3 to reflect the current volatility, which is now 15%.
which of the following statements about the missing data in exhibit 3 is correct?
A) node 3-2 can be derived from node 2-2.
B) node 4-1 should be equal to node 4-5 multiplied by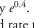
C) node 2-2 approximates the implied one-year forward rate two years from now.
katrina black, portfolio manager at Coral bond Management, ltd., is conducting a training
session with alex Sun, a junior analyst in the fixed income department. black wants to ex-
plain to Sun the arbitrage-free valuation framework used by the firm. black presents Sun with
exhibit 1, showing a fictitious bond being traded on three exchanges, and asks Sun to identify
the arbitrage opportunity of the bond. Sun agrees to ignore transaction costs in his analysis.
exhibit 1 Three-Year, €100 par, 3.00% Coupon, annual-Pay option-Free bond
 black shows Sun some exhibits that were part of a recent presentation. exhibit 3 presents
black shows Sun some exhibits that were part of a recent presentation. exhibit 3 presents most of the data of a binomial lognormal interest rate tree fit to the yield curve shown in ex-
hibit 2. exhibit 4 presents most of the data of the implied values for a four-year, option-free,
annual-pay bond with a 2.5% coupon based on the information in exhibit 3.
exhibit 2 Yield to Maturity Par rates for one-, two-, and Three-Year annual-Pay
option-Free bonds
 exhibit 3 binomial interest rate tree Fit to the Yield Curve (Volatility = 10%)
exhibit 3 binomial interest rate tree Fit to the Yield Curve (Volatility = 10%) exhibit 4 implied Values (in euros) for a 2.5%, Four-Year, option-Free, annual-Pay bond
exhibit 4 implied Values (in euros) for a 2.5%, Four-Year, option-Free, annual-Pay bond based on exhibit 3
 black asks about the missing data in exhibits 3 and 4 and directs Sun to complete the
black asks about the missing data in exhibits 3 and 4 and directs Sun to complete the following tasks related to those exhibits:
task 1 test that the binomial interest tree has been properly calibrated to be
arbitrage-free.
task 2 Develop a spreadsheet model to calculate pathwise valuations. to test the ac-
curacy of the spreadsheet, use the data in exhibit 3 and calculate the value of
the bond if it takes a path of lowest rates in Year 1 and Year 2 and the second
lowest rate in Year 3.
task 3 identify a type of bond where the Monte Carlo calibration method should
be used in place of the binomial interest rate method.
task 4 update exhibit 3 to reflect the current volatility, which is now 15%.
which of the following statements about the missing data in exhibit 3 is correct?
A) node 3-2 can be derived from node 2-2.
B) node 4-1 should be equal to node 4-5 multiplied by
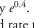
C) node 2-2 approximates the implied one-year forward rate two years from now.

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
16
The following information relates to Questions
Meredith alvarez is a junior fixed-income analyst with Canzim asset Management. her su-
pervisor, Stephanie hartson, asks alvarez to review the asset price and payoff data shown in
exhibit 1 to determine whether an arbitrage opportunity exists.
exhibit 1 Price and Payoffs for two risk-Free assets

hartson also shows alvarez data for a bond that trades in three different markets in the
same currency. These data appear in exhibit 2.
exhibit 2 2% Coupon, Five-Year Maturity, annual-Pay bond

hartson asks alvarez to value two bonds (bond C and bond D) using the binomial tree
in exhibit 3. exhibit 4 presents selected data for both bonds.
exhibit 3 binomial interest rate tree with Volatility = 25%

exhibit 4 Selected Data on annual-Pay bonds

hartson tells alvarez that she and her peers have been debating various viewpoints regard-
ing the conditions underlying binomial interest rate trees. The following statements were made
in the course of the debate.
Statement 1: The only requirements needed to create a binomial interest rate tree are
current benchmark interest rates and an assumption about interest rate
volatility.
Statement 2: Potential interest rate volatility in a binomial interest rate tree can be es-
timated using historical interest rate volatility or observed market prices
from interest rate derivatives.
Statement 3: a bond value derived from a binomial interest rate tree with a relatively
high volatility assumption will be different from the value calculated by
discounting the bond's cash flows using current spot rates.
based on data in exhibit 5, hartson asks alvarez to calibrate a binomial interest rate tree
starting with the calculation of implied forward rates shown in exhibit 6.
exhibit 5 Selected Data for a binomial interest rate tree

exhibit 6 Calibration of binomial interest rate

hartson mentions pathwise valuations as another method to value bonds using a binomial
interest rate tree. using the binomial interest rate tree in exhibit 3, alvarez calculates the pos-
sible interest rate paths for bond D shown in exhibit 7.
exhibit 7 interest rate Paths for bond D

before leaving for the day, hartson asks alvarez about the value of using the Monte Carlo
method to simulate a large number of potential interest rate paths to value a bond. alvarez
makes the following statements.
Statement 4: increasing the number of paths increases the estimate's statistical accuracy.
Statement 5: The bond value derived from a Monte Carlo simulation will be closer to
the bond's true fundamental value.
based on exhibit 1, alvarez finds that an arbitrage opportunity is:
A) not available.
B) available based on the dominance principle.
C) available based on the value additivity principle.
Meredith alvarez is a junior fixed-income analyst with Canzim asset Management. her su-
pervisor, Stephanie hartson, asks alvarez to review the asset price and payoff data shown in
exhibit 1 to determine whether an arbitrage opportunity exists.
exhibit 1 Price and Payoffs for two risk-Free assets

hartson also shows alvarez data for a bond that trades in three different markets in the
same currency. These data appear in exhibit 2.
exhibit 2 2% Coupon, Five-Year Maturity, annual-Pay bond

hartson asks alvarez to value two bonds (bond C and bond D) using the binomial tree
in exhibit 3. exhibit 4 presents selected data for both bonds.
exhibit 3 binomial interest rate tree with Volatility = 25%

exhibit 4 Selected Data on annual-Pay bonds

hartson tells alvarez that she and her peers have been debating various viewpoints regard-
ing the conditions underlying binomial interest rate trees. The following statements were made
in the course of the debate.
Statement 1: The only requirements needed to create a binomial interest rate tree are
current benchmark interest rates and an assumption about interest rate
volatility.
Statement 2: Potential interest rate volatility in a binomial interest rate tree can be es-
timated using historical interest rate volatility or observed market prices
from interest rate derivatives.
Statement 3: a bond value derived from a binomial interest rate tree with a relatively
high volatility assumption will be different from the value calculated by
discounting the bond's cash flows using current spot rates.
based on data in exhibit 5, hartson asks alvarez to calibrate a binomial interest rate tree
starting with the calculation of implied forward rates shown in exhibit 6.
exhibit 5 Selected Data for a binomial interest rate tree

exhibit 6 Calibration of binomial interest rate

hartson mentions pathwise valuations as another method to value bonds using a binomial
interest rate tree. using the binomial interest rate tree in exhibit 3, alvarez calculates the pos-
sible interest rate paths for bond D shown in exhibit 7.
exhibit 7 interest rate Paths for bond D

before leaving for the day, hartson asks alvarez about the value of using the Monte Carlo
method to simulate a large number of potential interest rate paths to value a bond. alvarez
makes the following statements.
Statement 4: increasing the number of paths increases the estimate's statistical accuracy.
Statement 5: The bond value derived from a Monte Carlo simulation will be closer to
the bond's true fundamental value.
based on exhibit 1, alvarez finds that an arbitrage opportunity is:
A) not available.
B) available based on the dominance principle.
C) available based on the value additivity principle.

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
17
The following information relates to Questions
Meredith alvarez is a junior fixed-income analyst with Canzim asset Management. her su-
pervisor, Stephanie hartson, asks alvarez to review the asset price and payoff data shown in
exhibit 1 to determine whether an arbitrage opportunity exists.
exhibit 1 Price and Payoffs for two risk-Free assets

hartson also shows alvarez data for a bond that trades in three different markets in the
same currency. These data appear in exhibit 2.
exhibit 2 2% Coupon, Five-Year Maturity, annual-Pay bond

hartson asks alvarez to value two bonds (bond C and bond D) using the binomial tree
in exhibit 3. exhibit 4 presents selected data for both bonds.
exhibit 3 binomial interest rate tree with Volatility = 25%

exhibit 4 Selected Data on annual-Pay bonds

hartson tells alvarez that she and her peers have been debating various viewpoints regard-
ing the conditions underlying binomial interest rate trees. The following statements were made
in the course of the debate.
Statement 1: The only requirements needed to create a binomial interest rate tree are
current benchmark interest rates and an assumption about interest rate
volatility.
Statement 2: Potential interest rate volatility in a binomial interest rate tree can be es-
timated using historical interest rate volatility or observed market prices
from interest rate derivatives.
Statement 3: a bond value derived from a binomial interest rate tree with a relatively
high volatility assumption will be different from the value calculated by
discounting the bond's cash flows using current spot rates.
based on data in exhibit 5, hartson asks alvarez to calibrate a binomial interest rate tree
starting with the calculation of implied forward rates shown in exhibit 6.
exhibit 5 Selected Data for a binomial interest rate tree

exhibit 6 Calibration of binomial interest rate

hartson mentions pathwise valuations as another method to value bonds using a binomial
interest rate tree. using the binomial interest rate tree in exhibit 3, alvarez calculates the pos-
sible interest rate paths for bond D shown in exhibit 7.
exhibit 7 interest rate Paths for bond D

before leaving for the day, hartson asks alvarez about the value of using the Monte Carlo
method to simulate a large number of potential interest rate paths to value a bond. alvarez
makes the following statements.
Statement 4: increasing the number of paths increases the estimate's statistical accuracy.
Statement 5: The bond value derived from a Monte Carlo simulation will be closer to
the bond's true fundamental value.
which of the statements regarding Monte Carlo simulation is correct?
A) only Statement 4 is correct.
B) only Statement 5 is correct.
C) both Statement 4 and Statement 5 are correct.
Meredith alvarez is a junior fixed-income analyst with Canzim asset Management. her su-
pervisor, Stephanie hartson, asks alvarez to review the asset price and payoff data shown in
exhibit 1 to determine whether an arbitrage opportunity exists.
exhibit 1 Price and Payoffs for two risk-Free assets

hartson also shows alvarez data for a bond that trades in three different markets in the
same currency. These data appear in exhibit 2.
exhibit 2 2% Coupon, Five-Year Maturity, annual-Pay bond

hartson asks alvarez to value two bonds (bond C and bond D) using the binomial tree
in exhibit 3. exhibit 4 presents selected data for both bonds.
exhibit 3 binomial interest rate tree with Volatility = 25%

exhibit 4 Selected Data on annual-Pay bonds

hartson tells alvarez that she and her peers have been debating various viewpoints regard-
ing the conditions underlying binomial interest rate trees. The following statements were made
in the course of the debate.
Statement 1: The only requirements needed to create a binomial interest rate tree are
current benchmark interest rates and an assumption about interest rate
volatility.
Statement 2: Potential interest rate volatility in a binomial interest rate tree can be es-
timated using historical interest rate volatility or observed market prices
from interest rate derivatives.
Statement 3: a bond value derived from a binomial interest rate tree with a relatively
high volatility assumption will be different from the value calculated by
discounting the bond's cash flows using current spot rates.
based on data in exhibit 5, hartson asks alvarez to calibrate a binomial interest rate tree
starting with the calculation of implied forward rates shown in exhibit 6.
exhibit 5 Selected Data for a binomial interest rate tree

exhibit 6 Calibration of binomial interest rate

hartson mentions pathwise valuations as another method to value bonds using a binomial
interest rate tree. using the binomial interest rate tree in exhibit 3, alvarez calculates the pos-
sible interest rate paths for bond D shown in exhibit 7.
exhibit 7 interest rate Paths for bond D

before leaving for the day, hartson asks alvarez about the value of using the Monte Carlo
method to simulate a large number of potential interest rate paths to value a bond. alvarez
makes the following statements.
Statement 4: increasing the number of paths increases the estimate's statistical accuracy.
Statement 5: The bond value derived from a Monte Carlo simulation will be closer to
the bond's true fundamental value.
which of the statements regarding Monte Carlo simulation is correct?
A) only Statement 4 is correct.
B) only Statement 5 is correct.
C) both Statement 4 and Statement 5 are correct.

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck


