Deck 17: Probability Web
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/89
Play
Full screen (f)
Deck 17: Probability Web
1
A computer manufacturer offers a computer system with three different disk drives, two different monitors, and five different keyboards. How many different computer systems could a consumer purchase from this manufacturer?
A)16
B)24
C)30
D)20
E)60
A)16
B)24
C)30
D)20
E)60
30
2
Find the probability for the experiment of drawing two marbles (without replacement) from a bag containing three green, five yellow, and four red marbles such that both marbles are yellow.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)
3
Find the number of distinguishable permutations of the group of letters.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)
4
In how many ways can a 9-question true-false exam be answered? (Assume that no questions are omitted.)
A)512
B)131,072
C)8192
D)524,288
E)4096
A)512
B)131,072
C)8192
D)524,288
E)4096

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
5
Determine the number of ways a computer can randomly generate an integer divisible by 4 from 1 through 15.
A)6
B)3
C)11
D)7
E)14
A)6
B)3
C)11
D)7
E)14

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
6
A computer randomly generates an integer from 1 through 50. Find the probability of the event that a multiple of 3 is generated.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
7
A state lottery game requires a person to select ten different numbers from thirty-three numbers. The order of the selection is not important. In how many ways can this be done?
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
8
Eighteen students are selected as semifinalists for a literary award. Of the eighteen students, eight finalists will be selected. In how many ways can eight finalists be selected from the eighteen students?
A)6435
B)203,490
C)24,310
D)43,758
E)125,970
A)6435
B)203,490
C)24,310
D)43,758
E)125,970

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
9
In a certain state, each automobile license plate number consists of two letters followed by a four-digit number. To avoid confusion between "O" and "zero" and "I" and "one", the letters "O" and "I" are not used. How many distinct license plate numbers can be formed?
A)57,600,000
B)5,760,000
C)13,824,000
D)138,240,000
E)331,776,000
A)57,600,000
B)5,760,000
C)13,824,000
D)138,240,000
E)331,776,000

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
10
Evaluate: 7P3
A)35
B)840
C)210
D)21
E)undefined
A)35
B)840
C)210
D)21
E)undefined

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
11
A combination lock will open when the right choice of three numbers (from 1 to 32) is selected. How many different lock combinations are possible?
A)98,304
B)1024
C)32
D)96
E)32,768
A)98,304
B)1024
C)32
D)96
E)32,768

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
12
Evaluate the expression.
A)5
B)20
C)625
D)1024
E)9
A)5
B)20
C)625
D)1024
E)9

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
13
There are 11 patients in Dr. Ziglar's waiting room. Dr. Ziglar can see 5 patients before lunch. In how many different orders can Dr. Ziglar see 5 of the patients before lunch?
A)462
B)332,640
C)55
D)5
E)55,440
A)462
B)332,640
C)55
D)5
E)55,440

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
14
Thirteen students, of whom three are seniors, are selected as semifinalists for a literary award. Of the thirteen students, nine finalists will be selected. In how many ways can the nine finalists contain two seniors?
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
15
At a high school cafeteria, diners can choose one vegetable from a choice of 2 vegetables, one meat from a choice of 3 meats, one serving of bread from among 4 breads, and a dessert from among 4 desserts. How many meal configurations are possible?
A)13
B)96
C)4
D)24
E)48
A)13
B)96
C)4
D)24
E)48

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
16
Decide which of the scenarios below should be counted using permutations or combinations. Scenario I:
Number of ways 12 movies can be ordered to play on television.
Scenario II:
Number of ways three different roles can be filled by 11 people auditioning for a play.
Scenario III:
Number of different two-topping pizzas that can be made from an assortment of 10 different toppings.
A)Scenario III is a combination and scenarios I and II are permutations.
B)All scenarios are combinations.
C)Scenario II is a combination and scenarios I and III are permutations.
D)Scenarios I and II are combinations and scenario III is a permutation.
E)All scenarios are permutations.
Number of ways 12 movies can be ordered to play on television.
Scenario II:
Number of ways three different roles can be filled by 11 people auditioning for a play.
Scenario III:
Number of different two-topping pizzas that can be made from an assortment of 10 different toppings.
A)Scenario III is a combination and scenarios I and II are permutations.
B)All scenarios are combinations.
C)Scenario II is a combination and scenarios I and III are permutations.
D)Scenarios I and II are combinations and scenario III is a permutation.
E)All scenarios are permutations.

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
17
Seven cards are chosen at random from a standard deck of playing cards. In how many ways can the cards be chosen if all seven cards are spades.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
18
A card is selected from a standard deck of 52 cards. Find the probability of getting a card that is less than 4 (aces are low).
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
19
A coin is tossed three times. Find the probability of getting at least two heads.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
20
Two six-sided dice are tossed. Find the probability that the sum is odd.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
21
Find given the probability distribution.
A)0.328
B)0.474
C)0.526
D)0.802
E)0.198
A)0.328
B)0.474
C)0.526
D)0.802
E)0.198

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
22
Estimate the variance for the following probability distribution to two decimal places.
A)
B)
C)
D)
E)1.00
A)
B)
C)
D)
E)1.00

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
23
Determine whether the table represents a probability distribution.
A)yes
B)no
A)yes
B)no

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
24
Assume that the probability of the birth of a child of a particular gender is 50%. In a family with seven children, what is the probability that there is at least one girl?
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
25
A sales representative makes a sale at approximately one-third of the businesses he calls on. On a given day, he goes to four businesses. What is the probability that he will make a sale at all four businesses?
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
26
A coin is tossed three times. Describe the event A that at least two heads occur.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
27
You are given the probability that an event will not happen. Find the probability that the event will happen.
A)
B)0
C)1
D)
E)
A)
B)0
C)1
D)
E)

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
28
Sketch a graph of the probability distribution.
A)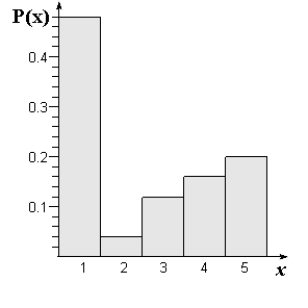
B)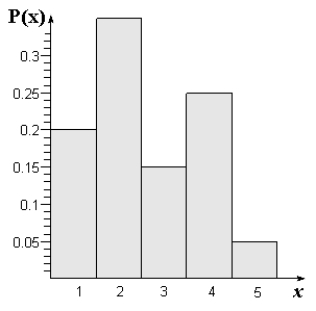
C)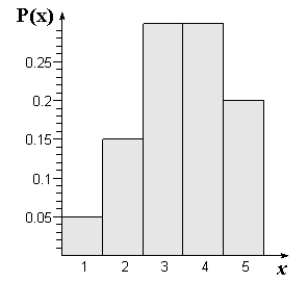
D)
E)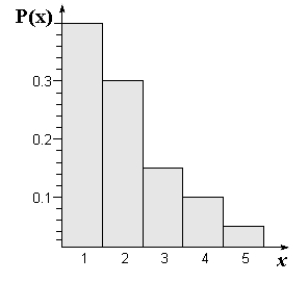
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
29
A card is chosen at random from two 52-card decks of playing cards" . What is the probability that the card will be black and a face card? A face card is a king, a queen, or a jack.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
30
You are given the probability that an event will happen. Find the probability that the event will not happen. P(E) = 0.29
A)0.29
B)0.71
C)0.355
D)0
E)1
A)0.29
B)0.71
C)0.355
D)0
E)1

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
31
Find the standard deviation for the following probability distribution. Round your answer to three decimal places.
A)2.800
B)1.720
C)8.762
D)2.960
E)1.673
A)2.800
B)1.720
C)8.762
D)2.960
E)1.673

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
32
A quality control inspector receives a shipment of 30 computer monitors. From the 30 monitors, the inspector randomly chooses 8 for inspection. If the probability of a monitor being defective is 0.07, what is the probability that at least one of the monitors chosen by the inspector is defective? Round to the nearest hundredth.
A)0.44
B)0.52
C)0.51
D)0.47
E)0.45
A)0.44
B)0.52
C)0.51
D)0.47
E)0.45

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
33
In a survey, Americans were asked how well informed they are about new scientific discoveries. The results are shown in the pie graph below. 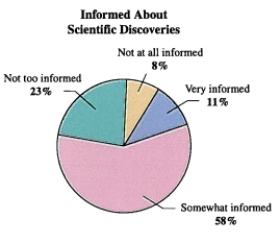 If two people from the survey are chosen at random, what is the probability that neither person feels very informed about scientific discoveries? Round to the nearest ten-thousandth.
If two people from the survey are chosen at random, what is the probability that neither person feels very informed about scientific discoveries? Round to the nearest ten-thousandth.
A)0.3864
B)0.7921
C)0.8464
D)0.6853
E)0.7084
 If two people from the survey are chosen at random, what is the probability that neither person feels very informed about scientific discoveries? Round to the nearest ten-thousandth.
If two people from the survey are chosen at random, what is the probability that neither person feels very informed about scientific discoveries? Round to the nearest ten-thousandth.A)0.3864
B)0.7921
C)0.8464
D)0.6853
E)0.7084

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
34
Find given the probability distribution.
A)0.344
B)0.656
C)0.794
D)1.000
E)0.250
A)0.344
B)0.656
C)0.794
D)1.000
E)0.250

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
35
One card is randomly drawn from a standard deck of playing cards where aces are not considered numbered cards and are the highest card in the suit. The card is replaced and another card is drawn. What is the probability that both cards drawn are numbered cards greater than two?
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
36
Find the probability for the experiment of drawing two marbles (without replacement) from a bag containing three green, six yellow, and four red marbles such that the marbles are different colors.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
37
A shipment of nine calculators contains five defective calculators. Three calculators are chosen from the shipment. Find the probability that exactly three are defective.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
38
Find the expected value for the following probability distribution. Round your answer to three decimal places. 5,000 2,500 300 0.012
0.052
0.936
A)9.529
B)837.255
C)8,244.640
D)700,995.360
E)90.800
0.052
0.936
A)9.529
B)837.255
C)8,244.640
D)700,995.360
E)90.800

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
39
Two people are asked their opinions on a political issue. They can answer "Opposed" (O) or "Undecided" (U). Find the sample space S.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
40
A biology instructor gives her class a list of eight study problems, from which she will select five to be answered on an exam. A student knows how to solve six of the problems. Find the probability that the student will be able to answer all five questions on the exam.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
41
A baseball fan examined the record of a favorite baseball player's performance during his last 50 games. The numbers of games in which the player had zero, one, two, three, and four hits are recorded in the table shown below. Find the standard deviation . Round your answer to two decimal places.
A)1.10
B)1.00
C)1.02
D)1.01
E)1.05
A)1.10
B)1.00
C)1.02
D)1.01
E)1.05

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
42
Find the mean, variance, and standard deviation of the uniform distribution over the interval [0, 18] without using integration.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
43
Find the value of the constant a that makes the given function a probability density function on the stated interval.
A)
B)
C)
D)
E)1
A)
B)
C)
D)
E)1

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
44
For the probability density function on the interval , find the probability that . Round your answer to the nearest hundredth.
A)0.1
B)0.63
C)0.86
D)0.08
E)0.06
A)0.1
B)0.63
C)0.86
D)0.08
E)0.06

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
45
The daily demand for gasoline (in millions of gallons) in a city is described by the probability density function over the interval . Find the probability that the daily demand for gasoline will be at least 3 million gallons.
A)0.640
B)0.360
C)0.423
D)0.562
E)0.490
A)0.640
B)0.360
C)0.423
D)0.562
E)0.490

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
46
Sketch the graph of the probability density function over the interval .
A)![<strong>Sketch the graph of the probability density function f ( x ) = \frac { 1 } { 3 } over the interval [ 0,3 ] .</strong> A) B) C) D) E)](https://storage.examlex.com/TB8692/11eb99ec_11ba_fa02_bdc5_c790d870c4cf_TB8692_11.jpg)
B)![<strong>Sketch the graph of the probability density function f ( x ) = \frac { 1 } { 3 } over the interval [ 0,3 ] .</strong> A) B) C) D) E)](https://storage.examlex.com/TB8692/11eb99ec_11ba_fa03_bdc5_2d2b1871ae1e_TB8692_11.jpg)
C)![<strong>Sketch the graph of the probability density function f ( x ) = \frac { 1 } { 3 } over the interval [ 0,3 ] .</strong> A) B) C) D) E)](https://storage.examlex.com/TB8692/11eb99ec_11ba_fa04_bdc5_311535a33b3e_TB8692_11.jpg)
D)![<strong>Sketch the graph of the probability density function f ( x ) = \frac { 1 } { 3 } over the interval [ 0,3 ] .</strong> A) B) C) D) E)](https://storage.examlex.com/TB8692/11eb99ec_11ba_fa05_bdc5_e38404d3474f_TB8692_11.jpg)
E)![<strong>Sketch the graph of the probability density function f ( x ) = \frac { 1 } { 3 } over the interval [ 0,3 ] .</strong> A) B) C) D) E)](https://storage.examlex.com/TB8692/11eb99ec_11ba_fa06_bdc5_73a115bf04b1_TB8692_11.jpg)
A)
![<strong>Sketch the graph of the probability density function f ( x ) = \frac { 1 } { 3 } over the interval [ 0,3 ] .</strong> A) B) C) D) E)](https://storage.examlex.com/TB8692/11eb99ec_11ba_fa02_bdc5_c790d870c4cf_TB8692_11.jpg)
B)
![<strong>Sketch the graph of the probability density function f ( x ) = \frac { 1 } { 3 } over the interval [ 0,3 ] .</strong> A) B) C) D) E)](https://storage.examlex.com/TB8692/11eb99ec_11ba_fa03_bdc5_2d2b1871ae1e_TB8692_11.jpg)
C)
![<strong>Sketch the graph of the probability density function f ( x ) = \frac { 1 } { 3 } over the interval [ 0,3 ] .</strong> A) B) C) D) E)](https://storage.examlex.com/TB8692/11eb99ec_11ba_fa04_bdc5_311535a33b3e_TB8692_11.jpg)
D)
![<strong>Sketch the graph of the probability density function f ( x ) = \frac { 1 } { 3 } over the interval [ 0,3 ] .</strong> A) B) C) D) E)](https://storage.examlex.com/TB8692/11eb99ec_11ba_fa05_bdc5_e38404d3474f_TB8692_11.jpg)
E)
![<strong>Sketch the graph of the probability density function f ( x ) = \frac { 1 } { 3 } over the interval [ 0,3 ] .</strong> A) B) C) D) E)](https://storage.examlex.com/TB8692/11eb99ec_11ba_fa06_bdc5_73a115bf04b1_TB8692_11.jpg)

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
47
For the probability density function on the interval find the probability that . Round your answer to the nearest hundredth.
A)0.05
B)0.33
C)0.93
D)0.67
E)0.02
A)0.05
B)0.33
C)0.93
D)0.67
E)0.02

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
48
For the given probability density function, find
A)2.000
B)1.150
C)1.500
D)0.150
E)0.800
A)2.000
B)1.150
C)1.500
D)0.150
E)0.800

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
49
The time t (in hours) required for a new employee to successfully learn to operate a machine in a manufacturing process is described by the probability density function over the interval . Find the probability that a new employee will learn to operate the machine in more than 3 hours but less than 7 hours.
A)0.6634
B)0.3052
C)0.5895
D)0.2733
E)0.4632
A)0.6634
B)0.3052
C)0.5895
D)0.2733
E)0.4632

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
50
A meteorologist predicts that the amount of rainfall (in inches) expected for a certain coastal community during a hurricane has the probability density function . Find and interpret the probability .
A)17% probability of receiving up to 5 inches of rain
B)25% probability of receiving up to 5 inches of rain
C)30% probability of receiving up to 5 inches of rain
D)75% probability of receiving up to 5 inches of rain
E)83% probability of receiving up to 5 inches of rain
A)17% probability of receiving up to 5 inches of rain
B)25% probability of receiving up to 5 inches of rain
C)30% probability of receiving up to 5 inches of rain
D)75% probability of receiving up to 5 inches of rain
E)83% probability of receiving up to 5 inches of rain

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
51
For the probability density function on the interval , find the probability that . Round your answer to the nearest thousandth.
A)0.112
B)0.388
C)0.183
D)0.333
E)0.223
A)0.112
B)0.388
C)0.183
D)0.333
E)0.223

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
52
Find the median of the exponential probability density function over the interval .
A)0.555
B)0.693
C)0.866
D)0.485
E)0.400
A)0.555
B)0.693
C)0.866
D)0.485
E)0.400

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
53
Sketch the graph of the following probability density function and locate the mean on the graph. ![<strong>Sketch the graph of the following probability density function and locate the mean on the graph. f ( t ) = \frac { t } { 18 } , [ 0,6 ] </strong> A) mean :1.5 B) mean :2.0 C) mean :4.0 D) mean :3.0 E) mean :4.0](https://storage.examlex.com/TB8692/11eb99ec_11bb_bd6e_bdc5_11ebf90f849a_TB8692_11.jpg)
![<strong>Sketch the graph of the following probability density function and locate the mean on the graph. f ( t ) = \frac { t } { 18 } , [ 0,6 ] </strong> A) mean :1.5 B) mean :2.0 C) mean :4.0 D) mean :3.0 E) mean :4.0](https://storage.examlex.com/TB8692/11eb99ec_11bb_bd70_bdc5_930aacff34cb_TB8692_11.jpg)
A)![<strong>Sketch the graph of the following probability density function and locate the mean on the graph. f ( t ) = \frac { t } { 18 } , [ 0,6 ] </strong> A) mean :1.5 B) mean :2.0 C) mean :4.0 D) mean :3.0 E) mean :4.0](https://storage.examlex.com/TB8692/11eb99ec_11bb_bd71_bdc5_9d981926687e_TB8692_11.jpg) mean :1.5
mean :1.5
B)![<strong>Sketch the graph of the following probability density function and locate the mean on the graph. f ( t ) = \frac { t } { 18 } , [ 0,6 ] </strong> A) mean :1.5 B) mean :2.0 C) mean :4.0 D) mean :3.0 E) mean :4.0](https://storage.examlex.com/TB8692/11eb99ec_11bb_bd72_bdc5_7bfbbabc1a78_TB8692_11.jpg) mean :2.0
mean :2.0
C)![<strong>Sketch the graph of the following probability density function and locate the mean on the graph. f ( t ) = \frac { t } { 18 } , [ 0,6 ] </strong> A) mean :1.5 B) mean :2.0 C) mean :4.0 D) mean :3.0 E) mean :4.0](https://storage.examlex.com/TB8692/11eb99ec_11bb_bd73_bdc5_d9b6bec356c9_TB8692_11.jpg) mean :4.0
mean :4.0
D)![<strong>Sketch the graph of the following probability density function and locate the mean on the graph. f ( t ) = \frac { t } { 18 } , [ 0,6 ] </strong> A) mean :1.5 B) mean :2.0 C) mean :4.0 D) mean :3.0 E) mean :4.0](https://storage.examlex.com/TB8692/11eb99ec_11bb_bd74_bdc5_e950cdbc2479_TB8692_11.jpg) mean :3.0
mean :3.0
E)![<strong>Sketch the graph of the following probability density function and locate the mean on the graph. f ( t ) = \frac { t } { 18 } , [ 0,6 ] </strong> A) mean :1.5 B) mean :2.0 C) mean :4.0 D) mean :3.0 E) mean :4.0](https://storage.examlex.com/TB8692/11eb99ec_11bb_e485_bdc5_c912e32675fe_TB8692_11.jpg) mean :4.0
mean :4.0
![<strong>Sketch the graph of the following probability density function and locate the mean on the graph. f ( t ) = \frac { t } { 18 } , [ 0,6 ] </strong> A) mean :1.5 B) mean :2.0 C) mean :4.0 D) mean :3.0 E) mean :4.0](https://storage.examlex.com/TB8692/11eb99ec_11bb_bd6e_bdc5_11ebf90f849a_TB8692_11.jpg)
![<strong>Sketch the graph of the following probability density function and locate the mean on the graph. f ( t ) = \frac { t } { 18 } , [ 0,6 ] </strong> A) mean :1.5 B) mean :2.0 C) mean :4.0 D) mean :3.0 E) mean :4.0](https://storage.examlex.com/TB8692/11eb99ec_11bb_bd70_bdc5_930aacff34cb_TB8692_11.jpg)
A)
![<strong>Sketch the graph of the following probability density function and locate the mean on the graph. f ( t ) = \frac { t } { 18 } , [ 0,6 ] </strong> A) mean :1.5 B) mean :2.0 C) mean :4.0 D) mean :3.0 E) mean :4.0](https://storage.examlex.com/TB8692/11eb99ec_11bb_bd71_bdc5_9d981926687e_TB8692_11.jpg) mean :1.5
mean :1.5B)
![<strong>Sketch the graph of the following probability density function and locate the mean on the graph. f ( t ) = \frac { t } { 18 } , [ 0,6 ] </strong> A) mean :1.5 B) mean :2.0 C) mean :4.0 D) mean :3.0 E) mean :4.0](https://storage.examlex.com/TB8692/11eb99ec_11bb_bd72_bdc5_7bfbbabc1a78_TB8692_11.jpg) mean :2.0
mean :2.0C)
![<strong>Sketch the graph of the following probability density function and locate the mean on the graph. f ( t ) = \frac { t } { 18 } , [ 0,6 ] </strong> A) mean :1.5 B) mean :2.0 C) mean :4.0 D) mean :3.0 E) mean :4.0](https://storage.examlex.com/TB8692/11eb99ec_11bb_bd73_bdc5_d9b6bec356c9_TB8692_11.jpg) mean :4.0
mean :4.0D)
![<strong>Sketch the graph of the following probability density function and locate the mean on the graph. f ( t ) = \frac { t } { 18 } , [ 0,6 ] </strong> A) mean :1.5 B) mean :2.0 C) mean :4.0 D) mean :3.0 E) mean :4.0](https://storage.examlex.com/TB8692/11eb99ec_11bb_bd74_bdc5_e950cdbc2479_TB8692_11.jpg) mean :3.0
mean :3.0E)
![<strong>Sketch the graph of the following probability density function and locate the mean on the graph. f ( t ) = \frac { t } { 18 } , [ 0,6 ] </strong> A) mean :1.5 B) mean :2.0 C) mean :4.0 D) mean :3.0 E) mean :4.0](https://storage.examlex.com/TB8692/11eb99ec_11bb_e485_bdc5_c912e32675fe_TB8692_11.jpg) mean :4.0
mean :4.0
Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
54
A publishing company introduces a new weekly magazine that sells for $4.95 on the newsstand. The marketing group of the company estimates that sales x (in thousands) will be approximated by the following probability function. Find the expected revenue. Round your answer to the nearest dollar.
A) 99,495
B) 99
C) 455
D) 20,100
E) 455,351
A) 99,495
B) 99
C) 455
D) 20,100
E) 455,351

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
55
Find the constant k such that the function is a probability density function over the interval .
A)5
B)
C)
D)
E)
A)5
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
56
Find the constant such that the function is a probability density function over the interval .
A)1
B)
C)
D)
E)
A)1
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
57
Buses arrive and depart from a college every 25 minutes. The probability density function for the waiting time t (in minutes) for a person arriving at the bus stop is on the interval . Find the probability that the person will wait no longer than 10 minutes.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
58
Sketch the graph of the following probability density function and locate the mean on the graph.
A)![<strong>Sketch the graph of the following probability density function and locate the mean on the graph. f ( x ) = \frac { 5 } { 2 } x ^ { 3 / 2 } , [ 0,1 ] </strong> A) mean :0.714 B) mean :1.600 C) mean :2.012 D) mean :0.555 E) mean :1.848](https://storage.examlex.com/TB8692/11eb99ec_11bb_e487_bdc5_db8218992145_TB8692_11.jpg) mean :0.714
mean :0.714
B)![<strong>Sketch the graph of the following probability density function and locate the mean on the graph. f ( x ) = \frac { 5 } { 2 } x ^ { 3 / 2 } , [ 0,1 ] </strong> A) mean :0.714 B) mean :1.600 C) mean :2.012 D) mean :0.555 E) mean :1.848](https://storage.examlex.com/TB8692/11eb99ec_11bb_e488_bdc5_3f8e76d3b7a1_TB8692_11.jpg) mean :1.600
mean :1.600
C)![<strong>Sketch the graph of the following probability density function and locate the mean on the graph. f ( x ) = \frac { 5 } { 2 } x ^ { 3 / 2 } , [ 0,1 ] </strong> A) mean :0.714 B) mean :1.600 C) mean :2.012 D) mean :0.555 E) mean :1.848](https://storage.examlex.com/TB8692/11eb99ec_11bb_e489_bdc5_edea98faa8f9_TB8692_11.jpg) mean :2.012
mean :2.012
D)![<strong>Sketch the graph of the following probability density function and locate the mean on the graph. f ( x ) = \frac { 5 } { 2 } x ^ { 3 / 2 } , [ 0,1 ] </strong> A) mean :0.714 B) mean :1.600 C) mean :2.012 D) mean :0.555 E) mean :1.848](https://storage.examlex.com/TB8692/11eb99ec_11bb_e48a_bdc5_4d9030eb2099_TB8692_11.jpg) mean :0.555
mean :0.555
E)![<strong>Sketch the graph of the following probability density function and locate the mean on the graph. f ( x ) = \frac { 5 } { 2 } x ^ { 3 / 2 } , [ 0,1 ] </strong> A) mean :0.714 B) mean :1.600 C) mean :2.012 D) mean :0.555 E) mean :1.848](https://storage.examlex.com/TB8692/11eb99ec_11bc_0b9b_bdc5_951bc56722ed_TB8692_11.jpg) mean :1.848
mean :1.848
A)
![<strong>Sketch the graph of the following probability density function and locate the mean on the graph. f ( x ) = \frac { 5 } { 2 } x ^ { 3 / 2 } , [ 0,1 ] </strong> A) mean :0.714 B) mean :1.600 C) mean :2.012 D) mean :0.555 E) mean :1.848](https://storage.examlex.com/TB8692/11eb99ec_11bb_e487_bdc5_db8218992145_TB8692_11.jpg) mean :0.714
mean :0.714B)
![<strong>Sketch the graph of the following probability density function and locate the mean on the graph. f ( x ) = \frac { 5 } { 2 } x ^ { 3 / 2 } , [ 0,1 ] </strong> A) mean :0.714 B) mean :1.600 C) mean :2.012 D) mean :0.555 E) mean :1.848](https://storage.examlex.com/TB8692/11eb99ec_11bb_e488_bdc5_3f8e76d3b7a1_TB8692_11.jpg) mean :1.600
mean :1.600C)
![<strong>Sketch the graph of the following probability density function and locate the mean on the graph. f ( x ) = \frac { 5 } { 2 } x ^ { 3 / 2 } , [ 0,1 ] </strong> A) mean :0.714 B) mean :1.600 C) mean :2.012 D) mean :0.555 E) mean :1.848](https://storage.examlex.com/TB8692/11eb99ec_11bb_e489_bdc5_edea98faa8f9_TB8692_11.jpg) mean :2.012
mean :2.012D)
![<strong>Sketch the graph of the following probability density function and locate the mean on the graph. f ( x ) = \frac { 5 } { 2 } x ^ { 3 / 2 } , [ 0,1 ] </strong> A) mean :0.714 B) mean :1.600 C) mean :2.012 D) mean :0.555 E) mean :1.848](https://storage.examlex.com/TB8692/11eb99ec_11bb_e48a_bdc5_4d9030eb2099_TB8692_11.jpg) mean :0.555
mean :0.555E)
![<strong>Sketch the graph of the following probability density function and locate the mean on the graph. f ( x ) = \frac { 5 } { 2 } x ^ { 3 / 2 } , [ 0,1 ] </strong> A) mean :0.714 B) mean :1.600 C) mean :2.012 D) mean :0.555 E) mean :1.848](https://storage.examlex.com/TB8692/11eb99ec_11bc_0b9b_bdc5_951bc56722ed_TB8692_11.jpg) mean :1.848
mean :1.848
Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
59
If x is the net gain to a player in a game of chance, then E(x) is usually negative. This value gives the average amount per game the player can expect to lose over the long run. A service organization is selling $2 raffle tickets as part of a fundraising program. The first prize is a boat valued at $2950, and the second prize is a camping tent valued at $400. In addition to the first and second prizes, there are twenty-four $23 gift certificates to be awarded. The number of tickets sold is 3000. Find the expected net gain to the player for one play of the game. Round your answer to the nearest cent.
A) 1122.33
B) 1.40
C) 0.70
D)-$0.7
E)-$1122.33
A) 1122.33
B) 1.40
C) 0.70
D)-$0.7
E)-$1122.33

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
60
An insurance company needs to determine the annual premium required to break even on fire protection policies with a face value of 80,000. If x is the claim size on these policies and the analysis is restricted to the losses 30,000, 60,000, and 80,000, then the probability distribution of x is as shown in the table. What premium should customers be charged for the company to break even? Round your answer to the nearest dollar.
A) 298
B) 17
C) 42,500
D) 300
E) 50,000
A) 298
B) 17
C) 42,500
D) 300
E) 50,000

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
61
Let be a random variable that is normally distributed with the given mean and standard deviation . Find the probability using a symbolic integration utility. Round your answer to four decimal places.
A)0.2525
B)0.1908
C)0.3085
D)0.0763
E)0.1223
A)0.2525
B)0.1908
C)0.3085
D)0.0763
E)0.1223

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
62
Find the sum using the formulas for the sums of powers of integers.
A)-1100
B)-4488
C)-396
D)264
E)-1496
A)-1100
B)-4488
C)-396
D)264
E)-1496

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
63
Let be a random variable that is normally distributed with the given mean and standard deviation . Find the probability using a symbolic integration utility.
A)0.7970
B)0.5889
C)0.6687
D)0.5359
E)0.6302
A)0.7970
B)0.5889
C)0.6687
D)0.5359
E)0.6302

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
64
Find a quadratic model for the sequence with the indicated terms.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
65
Evaluate the binomial coefficient.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
66
The arrival time t of a bus at a bus stop is uniformly distributed between A.M. and A.M. What is the probability that you will miss the bus if you arrive at the bus stop at A.M.? Round your answer to two decimal places.
A)0.25
B)0.67
C)0.36
D)0.33
E)0.57
A)0.25
B)0.67
C)0.36
D)0.33
E)0.57

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
67
Use mathematical induction to prove the formula for every positive integer n. Show all your work. 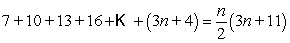
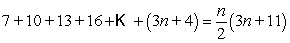

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
68
Find Pk+1 for the given Pk.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
69
The daily demand for a certain product (in hundreds of pounds) is a random variable with the probability density function over the interval . Find the probability that is within one standard deviation of the mean. Express your answer as a percent.
A)96.44
B)49.28
C)62.61
D)13.41
E)72.77
A)96.44
B)49.28
C)62.61
D)13.41
E)72.77

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
70
Find Pk+1 for the given Pk.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
71
If is an exponential random variable with the probability density function find
A)
B)
C)
D)
E)1
A)
B)
C)
D)
E)1

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
72
Find the mean, variance, and standard deviation of the normal density function over . Do not use integration.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
73
Evaluate using Pascal's triangle. Show your work. 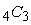
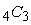

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
74
Find a formula for the sum of the n terms of the sequence.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
75
Prove the inequality for the indicated integer values of n. 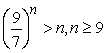
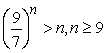

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
76
Use mathematical induction to prove the property for all positive integers n. 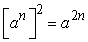
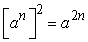

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
77
Find the sum using the formulas for the sums of powers of integers.
A)1296
B)4050
C)729
D)285
E)2025
A)1296
B)4050
C)729
D)285
E)2025

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
78
Find the mean and median of .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
79
Use mathematical induction to prove the formula for every positive integer n. Show all your work. 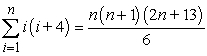
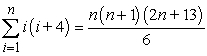

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck
80
Calculate the binomial coefficient:
A)2520
B)35
C)21
D)1
E)0
A)2520
B)35
C)21
D)1
E)0

Unlock Deck
Unlock for access to all 89 flashcards in this deck.
Unlock Deck
k this deck


