Deck 12: What Is Algebra
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/28
Play
Full screen (f)
Deck 12: What Is Algebra
1
Express symbolically, using variables, the general properties in algebra that are illustrated.
A)
B)
C)
D)
A)
B)
C)
D)
A) , or , or just ac + bc
B)
C)
D) . Compare this answer with (a + b) ÷ c = (a ÷ c) + (b ÷ c).
B)
C)
D) . Compare this answer with (a + b) ÷ c = (a ÷ c) + (b ÷ c).
2
Name the property (or properties) that justifies each of the following.
A) (700 + 60 + 3) + (200 + 30 + 5) = (3 + 5) + (60 + 30) + (700 + 200)
B) 5 × (17 + 3) = (5 × 17) + (5 × 3)
C) 984 + (717 + 563) = 984 + (563 + 717)
D) (56 × 89) ×

= 56 × 89
E)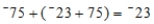
(Hint: More than one property!)
F)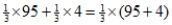
G)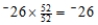
H) 5 × (17 × 3) = 5 × (3 × 17)
A) (700 + 60 + 3) + (200 + 30 + 5) = (3 + 5) + (60 + 30) + (700 + 200)
B) 5 × (17 + 3) = (5 × 17) + (5 × 3)
C) 984 + (717 + 563) = 984 + (563 + 717)
D) (56 × 89) ×

= 56 × 89
E)
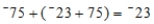
(Hint: More than one property!)
F)
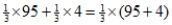
G)
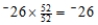
H) 5 × (17 × 3) = 5 × (3 × 17)
A) commutativity and associativity of addition
B) distributivity (of multiplication over addition)
C) commutativity of addition (not associativity)
D) One is the multiplicative identity.
E) commutativity and associativity of addition; additive inverse property; zero is the additive identity
F) distributivity (of multiplication over addition)
G) One is the multiplicative identity.
H) commutativity of multiplication (not associativity)
B) distributivity (of multiplication over addition)
C) commutativity of addition (not associativity)
D) One is the multiplicative identity.
E) commutativity and associativity of addition; additive inverse property; zero is the additive identity
F) distributivity (of multiplication over addition)
G) One is the multiplicative identity.
H) commutativity of multiplication (not associativity)
3
Test each algebra statement to see whether they appear to be true in general. If a statement appears always to be true, draw a diagram to justify the statement. Add an explanation if the diagram is not self-explanatory. If a statement is not true in general, give a counterexample.
A)
B)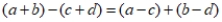
C)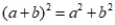
D)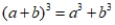
E)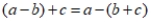
A)

B)
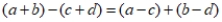
C)
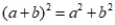
D)
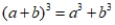
E)
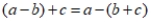
A) Not true in general; student should have shown a counterexample.
B) True. Sample diagram:
C) Not true in general; student should have shown a counterexample.
D) Not true in general; student should have shown a counterexample.
E) Not true in general; student should have shown a counterexample.
B) True. Sample diagram:

C) Not true in general; student should have shown a counterexample.
D) Not true in general; student should have shown a counterexample.
E) Not true in general; student should have shown a counterexample.
4
Make a drawing to justify 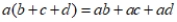
.
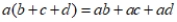
.

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
5
The "balance" diagram below shows x + 2 = x3. (If it is not true, give a correct equation.) 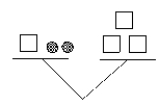


Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
6
Make a drawing of a balance for the equation 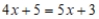
. Solve the equation by showing actions with the balance, one step at a time.
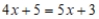
. Solve the equation by showing actions with the balance, one step at a time.

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
7
Show your mastery of the conventional order of operations by evaluating each.
A) 6 + 3 × 7 - (2 + -1) 5
B) 10 - 4 ÷ 3 × 2 + 1
C) 10 - 4 ÷ 3 × (2 + 1)
D) , when
E) , when
A) 6 + 3 × 7 - (2 + -1) 5
B) 10 - 4 ÷ 3 × 2 + 1
C) 10 - 4 ÷ 3 × (2 + 1)
D) , when
E) , when

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
8
In each part, use the given, correct algebraic statements to answer the calculations.
A) Part I:
implies that 14seventeen × 21seventeen = _____seventeen.
B) Part II:
implies that 23eleven × 21eleven = _____eleven.
C) Bonus: What other bases could be used in parts I and II?
A) Part I:
implies that 14seventeen × 21seventeen = _____seventeen.
B) Part II:
implies that 23eleven × 21eleven = _____eleven.
C) Bonus: What other bases could be used in parts I and II?

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
9
Complete the following and tell how they are alike.
A)
B)
A)
B)

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
10
A) Part I: It is correct that 12nine × 32nine = 384nine. How might that inform  ?
?
B) Part II: Explain the "might" in part A by considering 4nine × 13nine = 53nine. Give another calculation that would misinform an algebraic expression. Bonus: Why does 4nine × 13nine = 53nine, or your calculation, give an incorrect idea for algebra?
 ?
? B) Part II: Explain the "might" in part A by considering 4nine × 13nine = 53nine. Give another calculation that would misinform an algebraic expression. Bonus: Why does 4nine × 13nine = 53nine, or your calculation, give an incorrect idea for algebra?

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
11
Calculate the sum and product of the pairs of polynomials.
A)
B)
A)
B)

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
12
Use specific values for m and n in
to give a basis for justifying that
.
to give a basis for justifying that
.

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
13
Use to justify defining =

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
14
Use as the basis for defining a0 = 1 and a for nonzero values for a.

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
15
Give the 100th and the nth entries for these lists, assuming the patterns continue. A) 100th nth
B) 100th nth
C) 100th nth
B) 100th nth
C) 100th nth

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
16
Give the first four numbers of each of the following sequences.
A) An arithmetic sequence with first term 19 and difference 20
B) A geometric sequence with first term 1.2 and ratio 3
A) An arithmetic sequence with first term 19 and difference 20
B) A geometric sequence with first term 1.2 and ratio 3

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
17
What is the difference between an arithmetic sequence and a geometric sequence?

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
18
The repeating decimal for is 3.2715427154…. What digit is in the 99th decimal place in the repeating decimal? Explain how you know.

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
19
Does each of the following give a function? Include correct reasons for credit.
A) Associate with each whole number n its third power n 3.
B) Assign to each person in the town his/her current last name.
C) Assign to the last names of people in town the first names.
D) The ordered pairs (5, 2), (7, 2), and (4, 9)
E) This dot diagram:
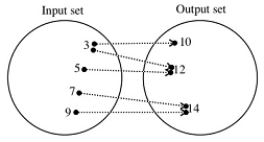
A) Associate with each whole number n its third power n 3.
B) Assign to each person in the town his/her current last name.
C) Assign to the last names of people in town the first names.
D) The ordered pairs (5, 2), (7, 2), and (4, 9)
E) This dot diagram:


Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
20
Find a likely function rule for each of the following. Show your work.
A)
f(x)=
B)
y=
C)
g(x)=
D)
y=
A)
f(x)=
B)
y=
C)
g(x)=
D)
y=

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
21
You find a function rule for a given table of data. Explain why your answer might not be the correct one.

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
22
Two students have been looking for a function rule for the data below.
n | f(n)
1 3
2 5
3 8
4 12
Akeena: "I got f(n) =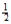
n(n +1) + 2."
Bea: "Yes, but my mom worked a long time on it and said f(n) =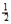
n(n + 1) + 2 + (n - 1)(n - 2)(n - 3)(n - 4). Let's ask the teacher."
How would you respond to the two students?
n | f(n)
1 3
2 5
3 8
4 12
Akeena: "I got f(n) =
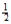
n(n +1) + 2."
Bea: "Yes, but my mom worked a long time on it and said f(n) =
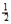
n(n + 1) + 2 + (n - 1)(n - 2)(n - 3)(n - 4). Let's ask the teacher."
How would you respond to the two students?

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
23
A child is making "space modules with antennas" from toothpicks. 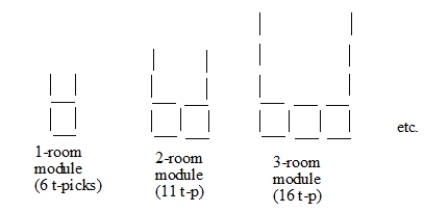
The child wonders, "How many toothpicks would it take to make a 100-room module with antennas?!"

The child wonders, "How many toothpicks would it take to make a 100-room module with antennas?!"

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
24
Take-home question: Here are examples of a shortcut for mentally squaring a number.
Example 1) 762: Go to the closest multiple of 10-here, for 76, plus 4 to 80. Then go the opposite way from 76 by the same amount, minus 4 to 72. Multiply 80 and 72 mentally for 5760. Add the square of the up-down number 4. As a result, 5760 + 16 = 5776 and 762 = 5776.
Example 2) 622: Go to 60 (down 2). Then go up from 62 by 2 to 64. Therefore, 60 × 64 = 3840. Add the square of 2 for 622 = 3844.
Example 3) 572: Go to 60 then 54. Therefore, 60 × 54 = 3240. Add 32 for 572 = 3249.
Example 4) 1982: 200 × 196 = 39,200. Add 22 for 39,204 (= 1982).
A) Use the shortcut to calculate 372 mentally, and then write the mental steps you did.
B) Give a justification that the method works for squaring any n. Label the up-down number x.
Example 1) 762: Go to the closest multiple of 10-here, for 76, plus 4 to 80. Then go the opposite way from 76 by the same amount, minus 4 to 72. Multiply 80 and 72 mentally for 5760. Add the square of the up-down number 4. As a result, 5760 + 16 = 5776 and 762 = 5776.
Example 2) 622: Go to 60 (down 2). Then go up from 62 by 2 to 64. Therefore, 60 × 64 = 3840. Add the square of 2 for 622 = 3844.
Example 3) 572: Go to 60 then 54. Therefore, 60 × 54 = 3240. Add 32 for 572 = 3249.
Example 4) 1982: 200 × 196 = 39,200. Add 22 for 39,204 (= 1982).
A) Use the shortcut to calculate 372 mentally, and then write the mental steps you did.
B) Give a justification that the method works for squaring any n. Label the up-down number x.

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
25
Finish each story problem so that it can be described by the given equation.
A) 50 - n = 16: "Jamal had 50 pieces of paper …"
B) 3n + 16.99 = 37: "Jose went shopping and bought a CD for $16.99 …"
A) 50 - n = 16: "Jamal had 50 pieces of paper …"
B) 3n + 16.99 = 37: "Jose went shopping and bought a CD for $16.99 …"

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
26
For each situation, write an equation using a variable. Then solve the equation to answer the question.
A) Krista has 4 more T-shirts than Leeann has, and Marika has 2 fewer T-shirts than Leeann has. The three students have 20 T-shirts in all. How many T-shirts does each have?
B) The entry fee at the fair is $5, and ride tickets cost 75¢ each. Pat spent $19.25 in all, for entry and ride tickets. How many ride tickets did Pat buy?
A) Krista has 4 more T-shirts than Leeann has, and Marika has 2 fewer T-shirts than Leeann has. The three students have 20 T-shirts in all. How many T-shirts does each have?
B) The entry fee at the fair is $5, and ride tickets cost 75¢ each. Pat spent $19.25 in all, for entry and ride tickets. How many ride tickets did Pat buy?

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
27
Over a four-day period, one Girl Scout troop sold 178 boxes of cookies on the first day, 39 more boxes on the third day than on the second day, and 10 boxes fewer on the fourth day than on the second day. The troop sold 489 boxes in the four days. How many boxes did they sell each day?

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck
28
Suppose g(x) = 3x - 2, and h(x) is defined by machine X below. What number is each of the following? Show your work. 
A) g(10) + g(5)
B) h(20)

A) g(10) + g(5)
B) h(20)

Unlock Deck
Unlock for access to all 28 flashcards in this deck.
Unlock Deck
k this deck


