Deck 10: Series
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/26
Play
Full screen (f)
Deck 10: Series
1
Use the nth term test to investigate the series .
A) The series converges.
B) , so the series diverges.
C) , so the test fails to tell us anything about the series.
D) , so the test fails to tell us anything about the series.
A) The series converges.
B) , so the series diverges.
C) , so the test fails to tell us anything about the series.
D) , so the test fails to tell us anything about the series.
, so the series diverges.
2
Use the integral test to investigate the series .
A) The integral , so the series .
B) The integral , so the series converges.
C) The integral , so the series diverges.
D) The integral , so the test fails to tell us anything about the series.
A) The integral , so the series .
B) The integral , so the series converges.
C) The integral , so the series diverges.
D) The integral , so the test fails to tell us anything about the series.
The integral , so the series converges.
3
Use the ratio test to investigate the series .
A) , so the series diverges.
B) , so the series converges.
C) , so the ratio test fails to tell us anything about the series.
D) , so the series converges.
A) , so the series diverges.
B) , so the series converges.
C) , so the ratio test fails to tell us anything about the series.
D) , so the series converges.
, so the series converges.
4
Investigate the alternating series .
A) The p- series converges, so the series converges absolutely.
B) The p-series diverges, so the series converges conditionally.
C) The ratio test gives , so the series diverges.
D) The p-series diverges, so the series diverges.
A) The p- series converges, so the series converges absolutely.
B) The p-series diverges, so the series converges conditionally.
C) The ratio test gives , so the series diverges.
D) The p-series diverges, so the series diverges.

Unlock Deck
Unlock for access to all 26 flashcards in this deck.
Unlock Deck
k this deck
5
Find the interval of convergence of the power series .
A)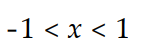
B)
C)
D)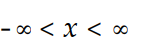
A)
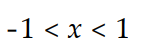
B)
C)
D)

Unlock Deck
Unlock for access to all 26 flashcards in this deck.
Unlock Deck
k this deck
6
Find a Maclaurin series expansion forf(x) .
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 26 flashcards in this deck.
Unlock Deck
k this deck
7
Find a Taylor series expansion for with .
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 26 flashcards in this deck.
Unlock Deck
k this deck
8
Use the first four non- zero terms of the Maclaurin series for to estimate .
A) .7405
B).7399
C) .7407
D).7402
A) .7405
B).7399
C) .7407
D).7402

Unlock Deck
Unlock for access to all 26 flashcards in this deck.
Unlock Deck
k this deck
9
Find the Fourier series for the square wave (of period ) given by
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 26 flashcards in this deck.
Unlock Deck
k this deck
10
For the problems below, determine whether each series converges or diverges.
-
-

Unlock Deck
Unlock for access to all 26 flashcards in this deck.
Unlock Deck
k this deck
11
For the problems below, determine whether each series converges or diverges.
-
-

Unlock Deck
Unlock for access to all 26 flashcards in this deck.
Unlock Deck
k this deck
12
For the problems below, use either the ratio test or the integral test to determine whether each series converges or diverges.
-
-

Unlock Deck
Unlock for access to all 26 flashcards in this deck.
Unlock Deck
k this deck
13
For the problems below, use either the ratio test or the integral test to determine whether each series converges or diverges.
-
-

Unlock Deck
Unlock for access to all 26 flashcards in this deck.
Unlock Deck
k this deck
14
For the problems below, determine whether each alternating series converges or diverges. If it converges, find whether it converges absolutely or converges conditionally.
-
-

Unlock Deck
Unlock for access to all 26 flashcards in this deck.
Unlock Deck
k this deck
15
For the problems below, determine whether each alternating series converges or diverges. If it converges, find whether it converges absolutely or converges conditionally.
-
-

Unlock Deck
Unlock for access to all 26 flashcards in this deck.
Unlock Deck
k this deck
16
For the problems below, determine whether each alternating series converges or diverges. If it converges, find whether it converges absolutely or converges conditionally.
-
-

Unlock Deck
Unlock for access to all 26 flashcards in this deck.
Unlock Deck
k this deck
17
For the problems below, find the interval of convergences of each series.
-
-

Unlock Deck
Unlock for access to all 26 flashcards in this deck.
Unlock Deck
k this deck
18
For the problems below, find the interval of convergences of each series.
-18
-18

Unlock Deck
Unlock for access to all 26 flashcards in this deck.
Unlock Deck
k this deck
19
For the problems below, find a Maclaurin series expansion for each function. Include at least thereetarms.
-
-

Unlock Deck
Unlock for access to all 26 flashcards in this deck.
Unlock Deck
k this deck
20
For the problems below, find a Maclaurin series expansion for each function. Include at least thereetarms.
-
-

Unlock Deck
Unlock for access to all 26 flashcards in this deck.
Unlock Deck
k this deck
21
Find a Maclaurin series expansion for .

Unlock Deck
Unlock for access to all 26 flashcards in this deck.
Unlock Deck
k this deck
22
Evaluate . (Use three non-zero terms.) Round to four significant digits.

Unlock Deck
Unlock for access to all 26 flashcards in this deck.
Unlock Deck
k this deck
23
For the problems below, find the Taylor series expansion for each function for the given value of a. Give at least three terms.
-
-

Unlock Deck
Unlock for access to all 26 flashcards in this deck.
Unlock Deck
k this deck
24
For the problems below, find the Taylor series expansion for each function for the given value of a. Give at least three terms.
-
-

Unlock Deck
Unlock for access to all 26 flashcards in this deck.
Unlock Deck
k this deck
25
Calculate the value of using the first four non- zero terms of a Taylor series. Round to five significant digits.

Unlock Deck
Unlock for access to all 26 flashcards in this deck.
Unlock Deck
k this deck
26
Find the Fourier series expansion of . Write at least four terms.

Unlock Deck
Unlock for access to all 26 flashcards in this deck.
Unlock Deck
k this deck


