Deck 3: Functions and Graphs
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/323
Play
Full screen (f)
Deck 3: Functions and Graphs
1
Determine whether the following rule defines as a function of .
-The , y pairs:
-The , y pairs:
True
2
Determine whether the following rule defines as a function of .
-The , y pairs:
-The , y pairs:
False
3
Determine whether the following rule defines as a function of .
-The , y pairs:
-The , y pairs:
False
4
Determine whether the following rule defines as a function of .
-
-

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
5
Determine whether the following rule defines as a function of .
-
-

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
6
Determine whether the following rule defines as a function of .
-
-

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
7
Determine whether the following rule defines as a function of .
-
-

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
8
Determine whether the following rule defines as a function of .
-
-

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
9
State the domain of the given function.
-
A) all real numbers except 0
B)
C)
D)
-
A) all real numbers except 0
B)
C)
D)

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
10
State the domain of the given function.
-
A)
B)
C)
D)
-
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
11
State the domain of the given function.
-
A) all real numbers except 7
B) 7
C)
D)
-
A) all real numbers except 7
B) 7
C)
D)

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
12
State the domain of the given function.
-
A) all real numbers except 6
B) all real numbers except -6
C)
D)
-
A) all real numbers except 6
B) all real numbers except -6
C)
D)

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
13
State the domain of the given function.
-
A) all real number except
B)
C)
D)
-
A) all real number except
B)
C)
D)

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
14
State the domain of the given function.
-
A) all real numbers except -2
B)
C)
D)
-
A) all real numbers except -2
B)
C)
D)

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
15
State the domain of the given function.
-
A)
B)
C)
D)
-
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
16
State the domain of the given function.
-
A)
B) all real numbers except 0
C) all whole numbers
D)
-
A)
B) all real numbers except 0
C) all whole numbers
D)

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
17
State the domain of the given function.
-
A)
B) all real numbers except
C)
D) all real numbers except
-
A)
B) all real numbers except
C)
D) all real numbers except

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
18
State the domain of the given function.
-
A)
B) or
C)
D)
-
A)
B) or
C)
D)

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
19
Evaluate the function.
-Given that , find
A) -24.8
B) -8
C) 3.1
D) -4.9
-Given that , find
A) -24.8
B) -8
C) 3.1
D) -4.9

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
20
Evaluate the function.
-Find when
A) -1
B) 3
C) 5
D) -3
-Find when
A) -1
B) 3
C) 5
D) -3

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
21
Evaluate the function.
-Find when
A) 6
B) 10
C) 18
D) 14
-Find when
A) 6
B) 10
C) 18
D) 14

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
22
Evaluate the function.
-Given that , find
A)
B)
C)
D)
-Given that , find
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
23
Evaluate the function.
-Given that , find .
A)
B)
C)
D)
-Given that , find .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
24
Evaluate the function.
-Given that , find .
A) 49.4
B) 64.1
C) 76.6
D) 28.6
-Given that , find .
A) 49.4
B) 64.1
C) 76.6
D) 28.6

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
25
Evaluate the function.
-Given that , find .
A) 21.4875
B) 13.8375
C) -9.0
D) -11.3625
-Given that , find .
A) 21.4875
B) 13.8375
C) -9.0
D) -11.3625

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
26
Evaluate the function.
-Given that , find
A) -0.728
B) 0.728
C) 3.968
D) 20.872
-Given that , find
A) -0.728
B) 0.728
C) 3.968
D) 20.872

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
27
Evaluate the function.
-Given that , find .
A)
B)
C)
D)
-Given that , find .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
28
Evaluate the function.
-Given that , find .
A)
B)
C)
D)
-Given that , find .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
29
Use a graphing calculator to construct a table of values for the given function.
-Use a graphing calculator to display a table showing the (approximate) values of the function at .
A)
B) C)
C) 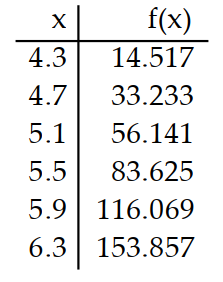 D)
D) 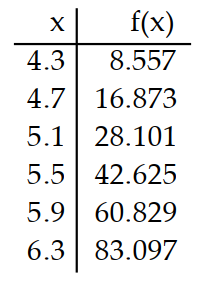
-Use a graphing calculator to display a table showing the (approximate) values of the function at .
A)

B)
 C)
C)  D)
D) 

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
30
Use a graphing calculator to construct a table of values for the given function.
-Use a graphing calculator to display a table showing the (approximate) values of the function at 2.1, 2.5, 2.9, 3.3, 3.7, 4.1.
A)
B)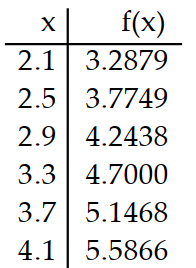 C)
C)  D)
D) 
-Use a graphing calculator to display a table showing the (approximate) values of the function at 2.1, 2.5, 2.9, 3.3, 3.7, 4.1.
A)

B)
 C)
C)  D)
D) 

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
31
Evaluate the function.
-Given that , and .
A)
B)
C)
D)
-Given that , and .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
32
Evaluate the function.
-Given that , find .
A)
B)
C)
D)
-Given that , find .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
33
Evaluate the function.
-Find when:
A)
B)
C)
D)
-Find when:
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
34
Evaluate the function.
-Given that , find .
A)
B)
C)
D)
-Given that , find .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
35
Evaluate the function.
-given that , find .
A)
B)
C)
D)
-given that , find .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
36
Evaluate the function.
-Given that , find .
A)
B)
C)
D)
-Given that , find .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
37
Evaluate the function.
-Determine when .
A)
B)
C)
D)
-Determine when .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
38
Evaluate the function.
-Find when:
A)
B)
C)
D)
-Find when:
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
39
Evaluate the function.
-Given that , find .
A)
B)
C)
D)
-Given that , find .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
40
Evaluate the function.
-Given that , find .
A)
B)
C)
D)
-Given that , find .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
41
For the given function, find .
-
A) 1
B)
C)
D) 9
-
A) 1
B)
C)
D) 9

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
42
For the given function, find .
-
A)
B) 1
C) -9
D)
-
A)
B) 1
C) -9
D)

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
43
For the given function, find .
-
A)
B)
C)
D) 1
-
A)
B)
C)
D) 1

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
44
For the given function, find .
-
A)
B)
C)
D)
-
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
45
For the given function, find .
-
A)
B)
C)
D) 1
-
A)
B)
C)
D) 1

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
46
Solve the problem.
-Suppose the cost of producing computers is . Find the cost of producing 80 computers.
A)
B)
C)
D)
-Suppose the cost of producing computers is . Find the cost of producing 80 computers.
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
47
Solve the problem.
-If a rock is thrown vertically upward from the surface of the moon at a speed of , its height after seconds will be meters. Find its height after 6 seconds.
A) 90.0 meters
B) 125.2 meters
C) 102.96 meters
D) 97.2 meters
-If a rock is thrown vertically upward from the surface of the moon at a speed of , its height after seconds will be meters. Find its height after 6 seconds.
A) 90.0 meters
B) 125.2 meters
C) 102.96 meters
D) 97.2 meters

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
48
Solve the problem.
-The water is drained from a tank by opening a valve at the bottom of the tank. The depth of the water in the tank thours after the valve is opened is meters. If the valve is opened at , what is the depth of water at ?
A) 2.62 meters
B) 1.50 meters
C) 0.38 meters
D) 0.17 meters
-The water is drained from a tank by opening a valve at the bottom of the tank. The depth of the water in the tank thours after the valve is opened is meters. If the valve is opened at , what is the depth of water at ?
A) 2.62 meters
B) 1.50 meters
C) 0.38 meters
D) 0.17 meters

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
49
Solve the problem.
-the size (in thousands) of a certain population in year is approximated by the function (where corresponds to the year 1990). predict the size of the population in the year 1997 .
A) About 73
B) About 61,140
C) About 72,900
D) About 1,599,038
-the size (in thousands) of a certain population in year is approximated by the function (where corresponds to the year 1990). predict the size of the population in the year 1997 .
A) About 73
B) About 61,140
C) About 72,900
D) About 1,599,038

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
50
Solve the problem.
-A cereal factory has weekly fixed costs of . It costs to produce each box of cereal. A box of cereal sells for . Find the rule of the cost function that gives the total weekly cost of producing boxes of cereal.
A)
B)
C)
D)
-A cereal factory has weekly fixed costs of . It costs to produce each box of cereal. A box of cereal sells for . Find the rule of the cost function that gives the total weekly cost of producing boxes of cereal.
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
51
Solve the problem.
-A cereal factory has weekly fixed costs of . It costs to produce each box of cereal. A box of cereal sells for . Find the rule of the revenue function that gives the total weekly revenue from selling boxes of cereal.
A)
B)
C)
D)
-A cereal factory has weekly fixed costs of . It costs to produce each box of cereal. A box of cereal sells for . Find the rule of the revenue function that gives the total weekly revenue from selling boxes of cereal.
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
52
Solve the problem.
-A cereal factory has weekly fixed costs of . It costs to produce each box of cereal. A box of cereal sells for . Find the rule of the profit function that gives the total weekly profit from boxes of cereal.
A)
B)
C)
D)
-A cereal factory has weekly fixed costs of . It costs to produce each box of cereal. A box of cereal sells for . Find the rule of the profit function that gives the total weekly profit from boxes of cereal.
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
53
Solve the problem.
-On a business trip, Jon must drive from his office to a clients's office, a distance of 204 miles. He sets off a . Suppose that he drives at a constant speed of . Find the rule of the function that gives his distance from the client's office at time hours (with corresponding to 8 a.m.)
A)
B)
C)
D)
-On a business trip, Jon must drive from his office to a clients's office, a distance of 204 miles. He sets off a . Suppose that he drives at a constant speed of . Find the rule of the function that gives his distance from the client's office at time hours (with corresponding to 8 a.m.)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
54
Solve the problem.
-Janice is running a 8354 meter race. Assume that she runs at a constant speed of 309 meters per minute. Find the rule of the function that gives her distance from the finishing line minutes after the start of the race.
A)
B)
C)
D)
-Janice is running a 8354 meter race. Assume that she runs at a constant speed of 309 meters per minute. Find the rule of the function that gives her distance from the finishing line minutes after the start of the race.
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
55
Graph the linear function.
-
A)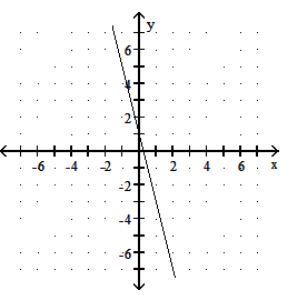
B)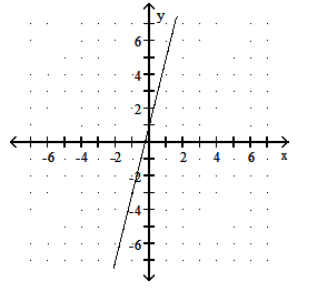
-

A)

B)


Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
56
Graph the linear function.
-
A)
B)
-

A)

B)


Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
57
Graph the linear function.
-
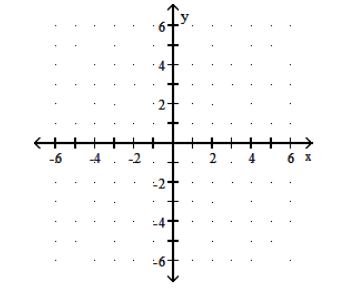
A)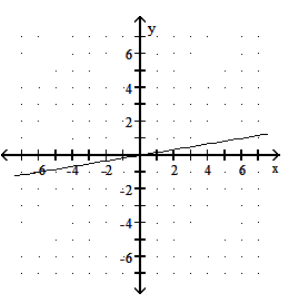
B)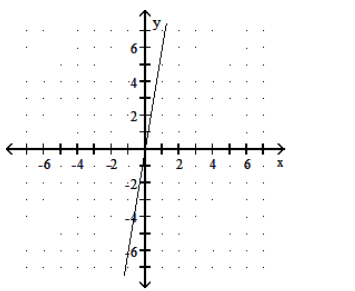
-

A)

B)


Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
58
Graph the linear function.
-
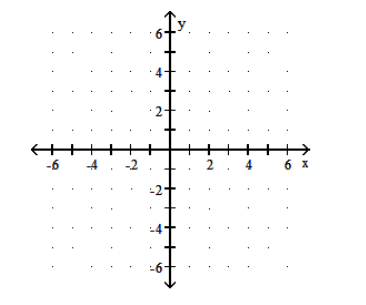
A)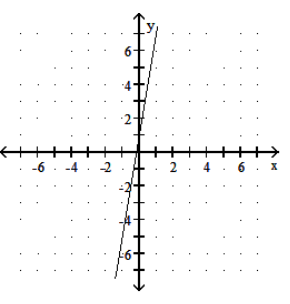
B)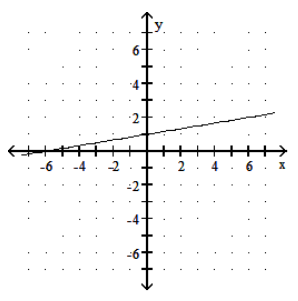
-

A)

B)


Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
59
Graph the linear function.
-
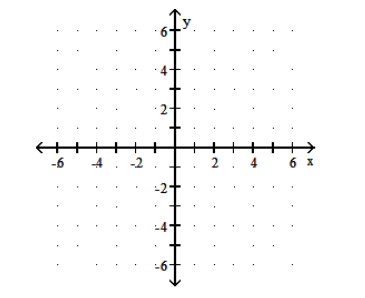
A)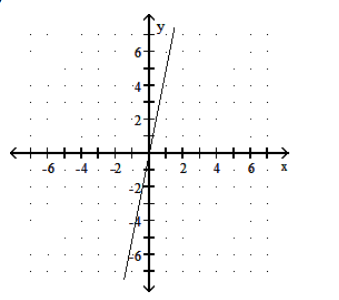
B)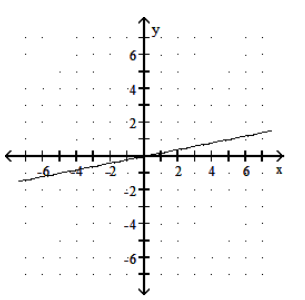
-

A)

B)


Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
60
Graph the piecewise linear function.
-
A)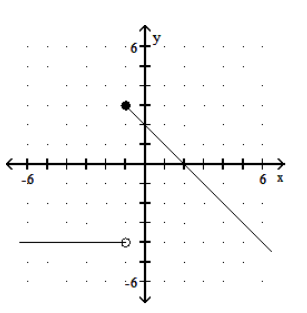
B)
C)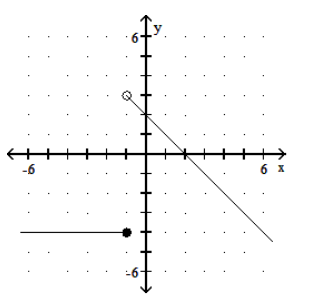
D)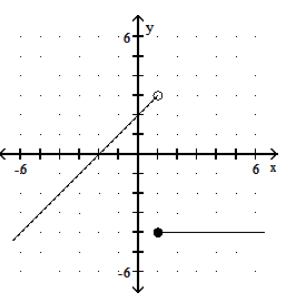
-

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
61
Graph the piecewise linear function.
-
A)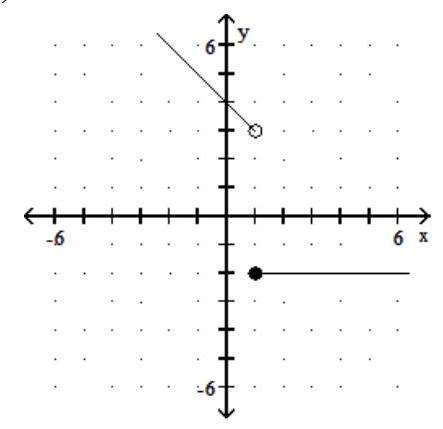
B)
C)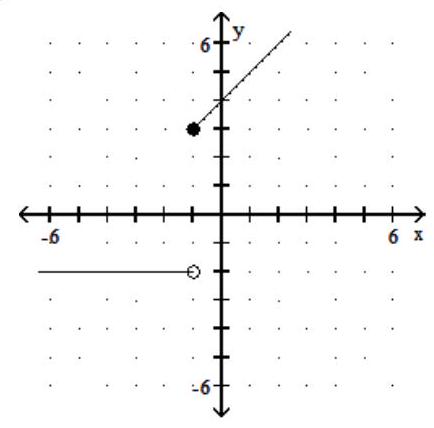
D)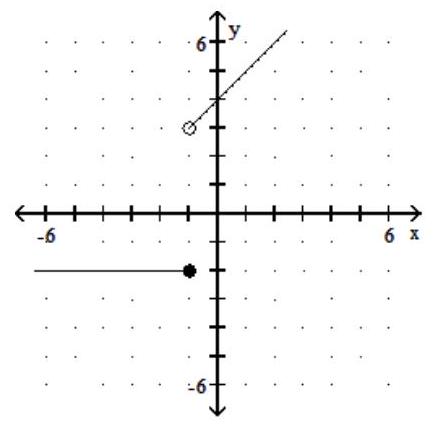
-

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
62
Graph the piecewise linear function.
-
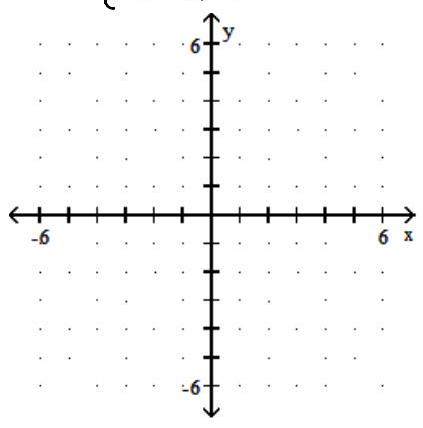
A)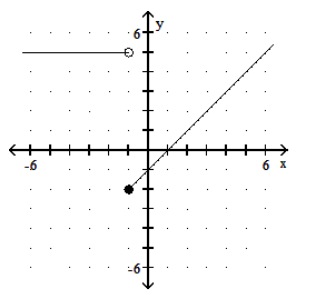
B)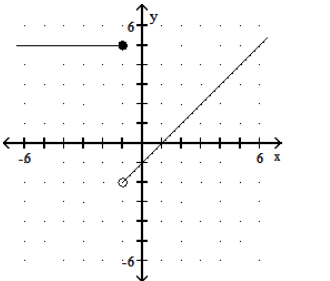
C)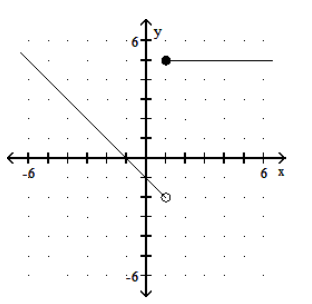
D)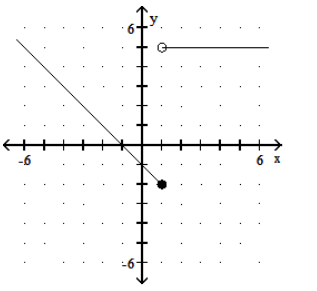
-

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
63
Graph the piecewise linear function.
-
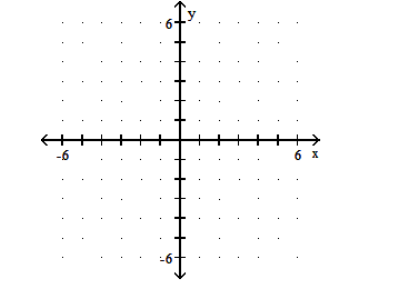
A)
B)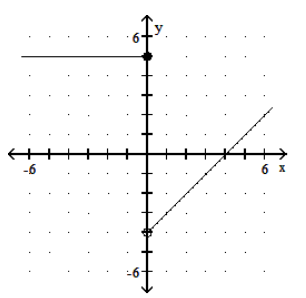
C)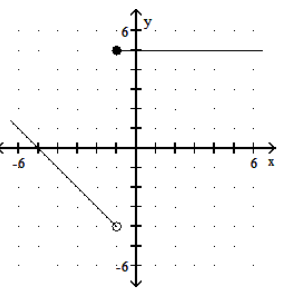
D)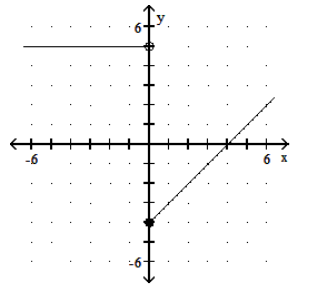
-

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
64
Graph the piecewise linear function.
-
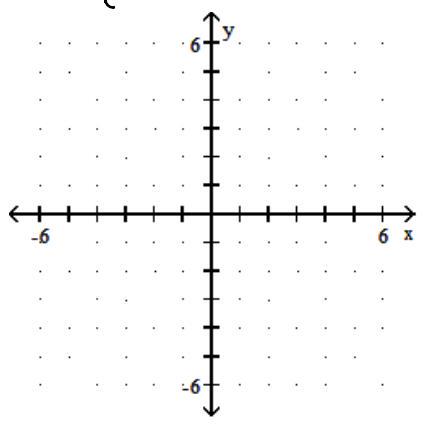
A)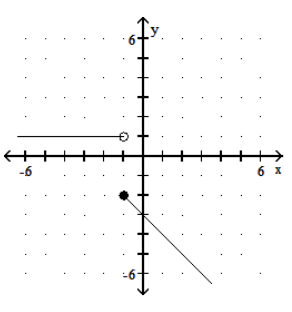
B)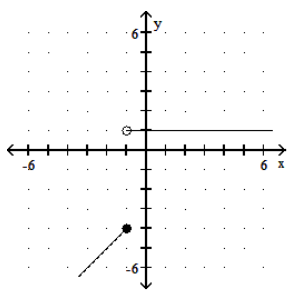
C)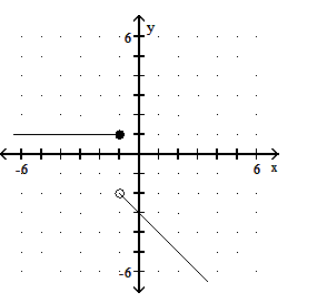
D)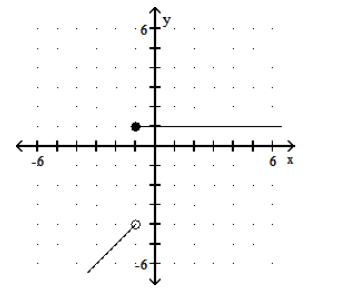
-

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
65
Graph the piecewise linear function.
-
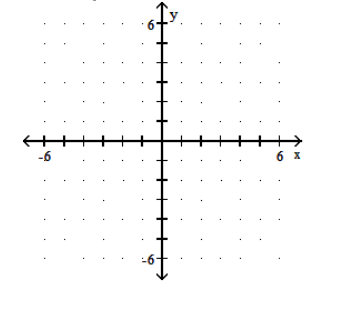
A)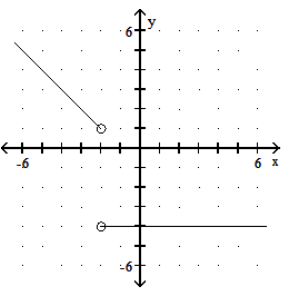
B)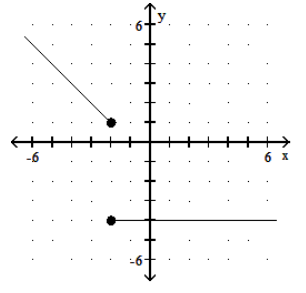
C)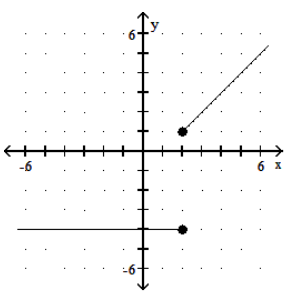
D)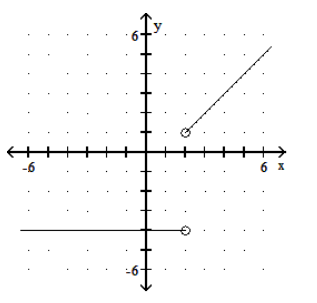
-

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
66
Graph the piecewise linear function.
-
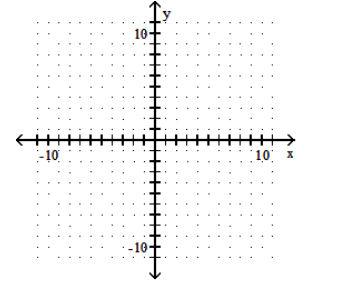
A)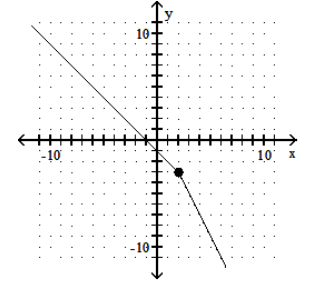
B)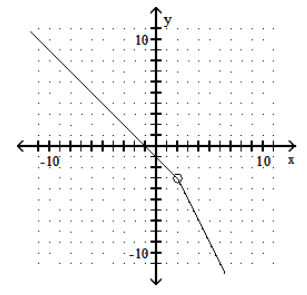
C)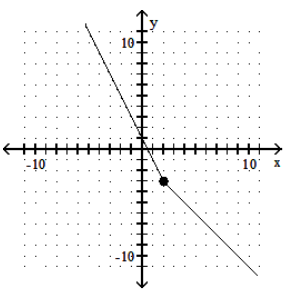
D)
-

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
67
Graph the function.
-
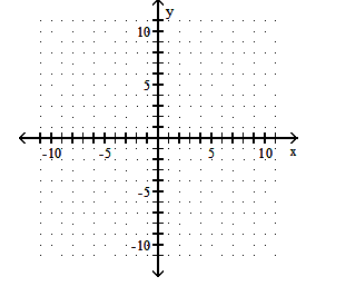
A)
B)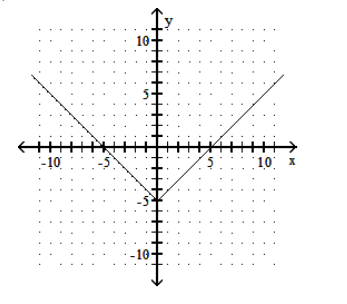
C)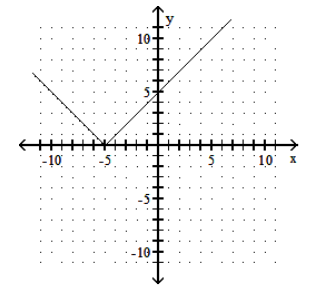
D)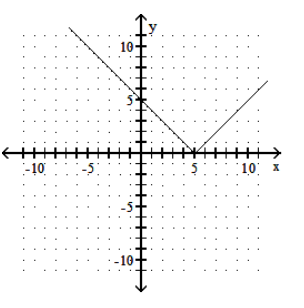
-

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
68
Graph the function.
-
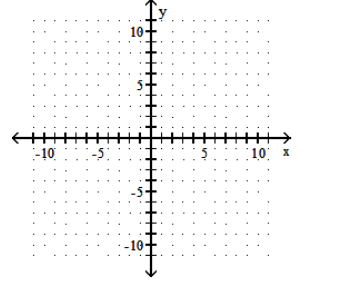
A)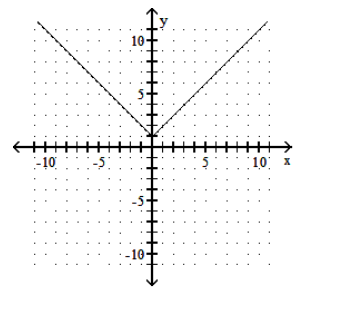
B)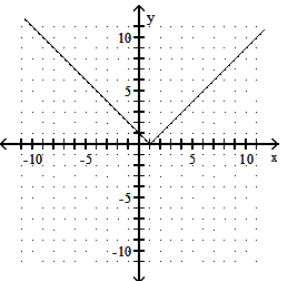
C)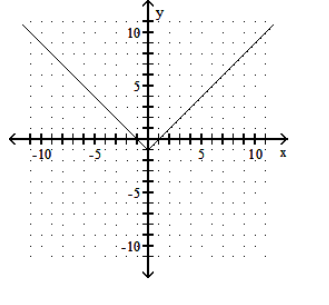
D)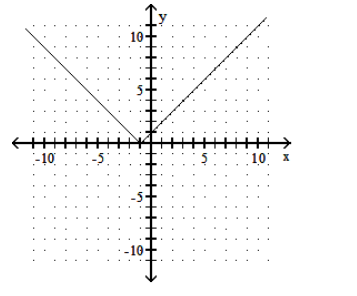
-

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
69
Graph the function.
-

A)
B)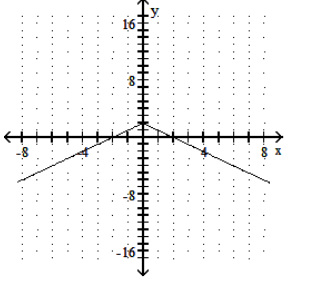
C)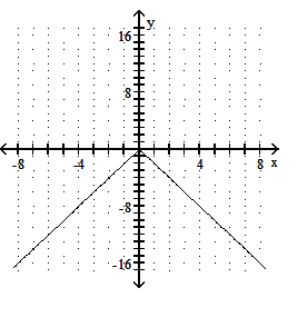
D)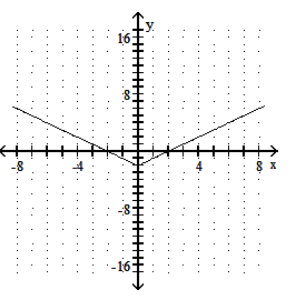
-

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
70
Graph the function.
-
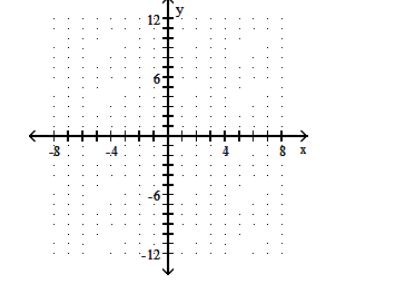
A)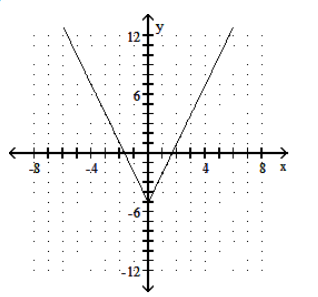
B)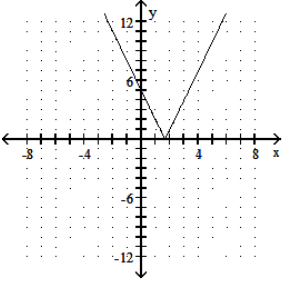
C)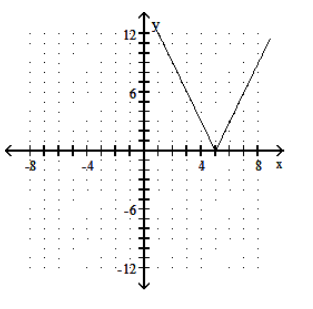
D)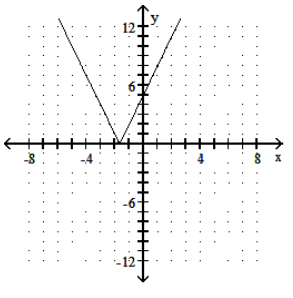
-

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
71
Graph the function.
-
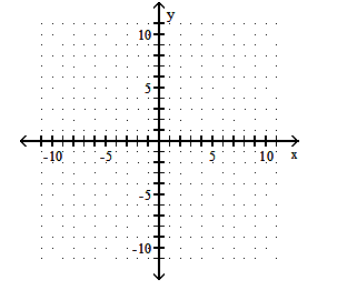
A)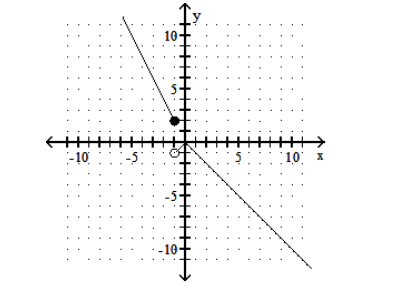
B)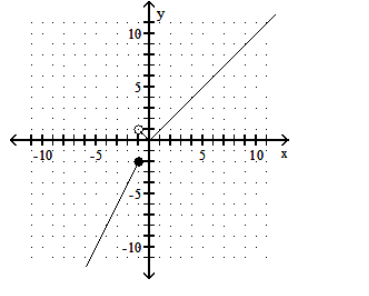
C)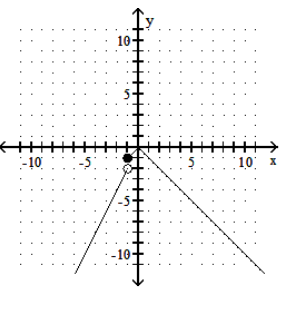
D)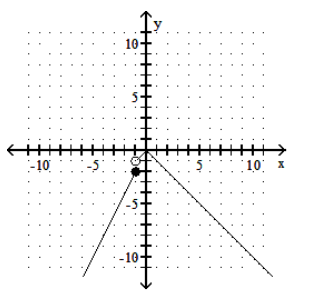
-

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
72
Graph the function.
-

A)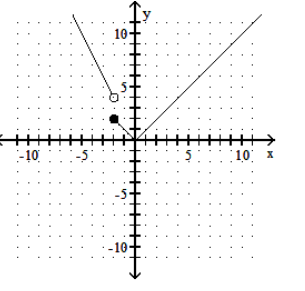
B)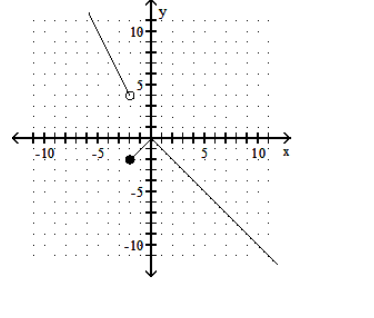
C)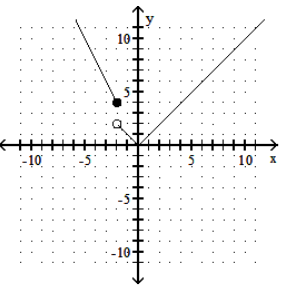
D)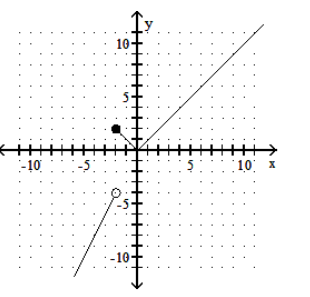
-

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
73
Graph the function.
-
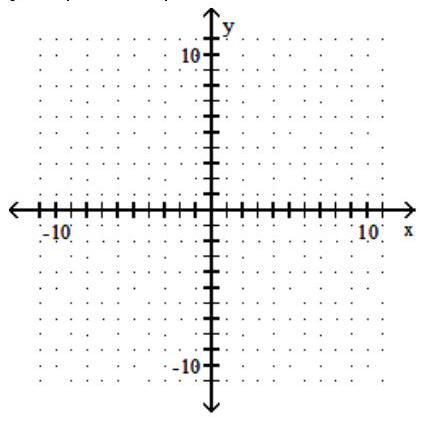
A)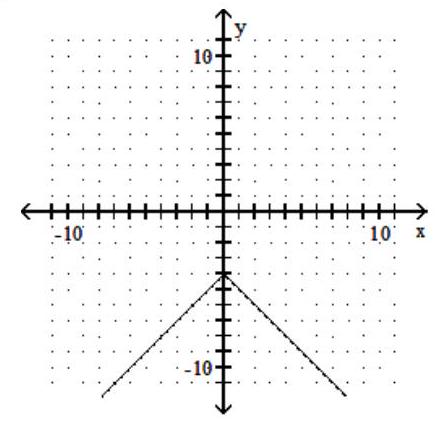
B)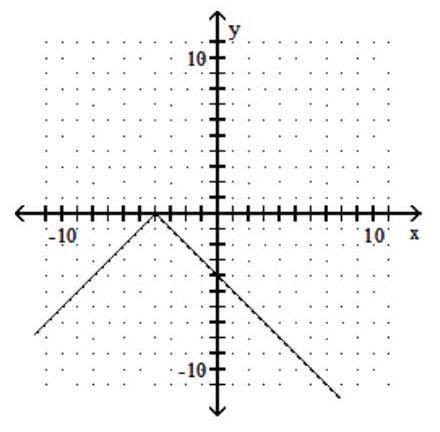
C)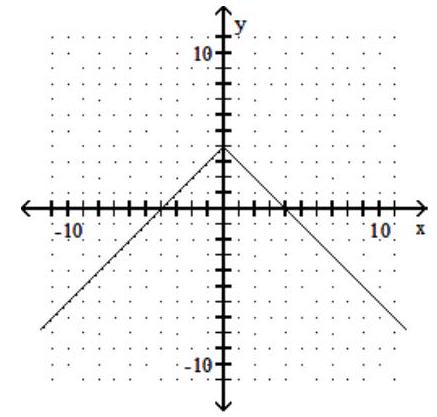
D)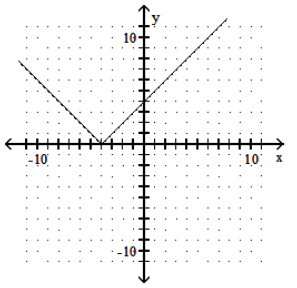
-

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
74
Graph the function.
-
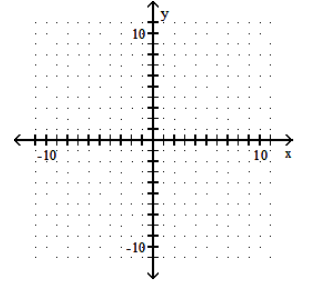
A)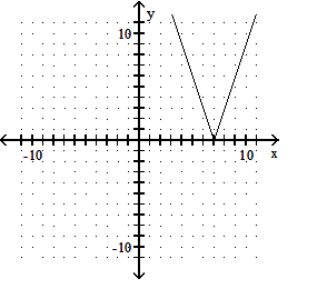
B)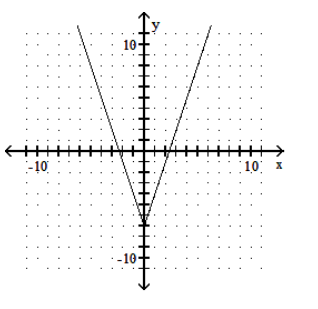
C)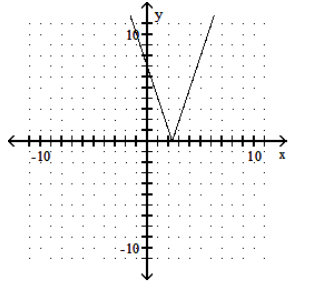
D)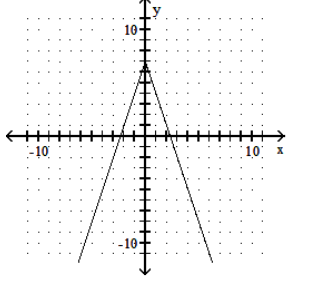
-

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
75
Graph the function.
-
A)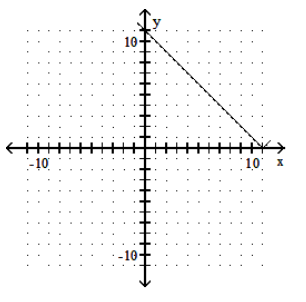
B)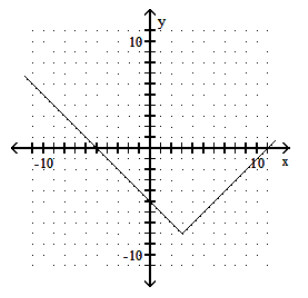
C)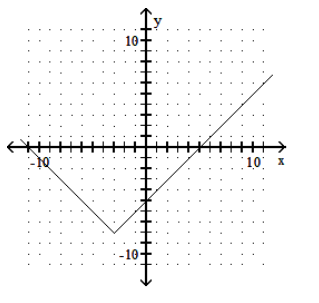
D)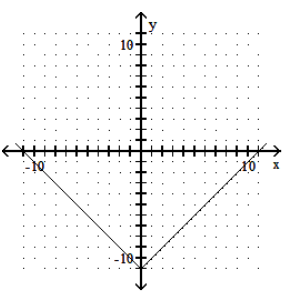
-

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
76
Graph the function.
-
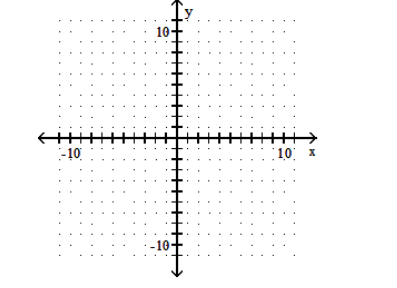
A)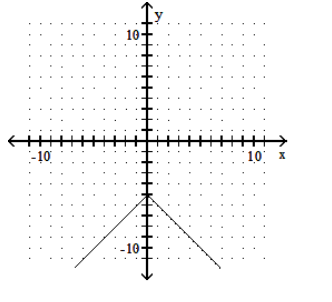
B)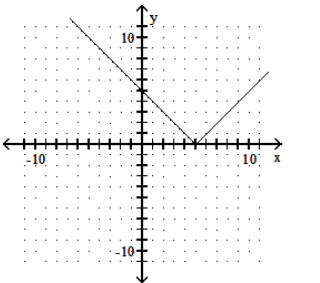
C)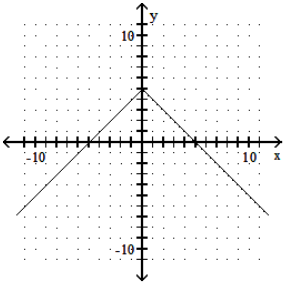
D)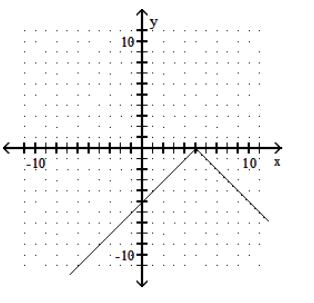
-

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
77
Graph the function.
-
![<strong>Graph the function. - y=[x]+1 </strong> A) B)](https://storage.examlex.com/TB10479/11eef996_cb98_4a92_b4b4_2b512e1e61f6_TB10479_00.jpg)
A)![<strong>Graph the function. - y=[x]+1 </strong> A) B)](https://storage.examlex.com/TB10479/11eef996_cb98_4a93_b4b4_3fa41bf9d10c_TB10479_00.jpg)
B)![<strong>Graph the function. - y=[x]+1 </strong> A) B)](https://storage.examlex.com/TB10479/11eef996_cb98_4a94_b4b4_e1c3a2248a3b_TB10479_00.jpg)
-
![<strong>Graph the function. - y=[x]+1 </strong> A) B)](https://storage.examlex.com/TB10479/11eef996_cb98_4a92_b4b4_2b512e1e61f6_TB10479_00.jpg)
A)
![<strong>Graph the function. - y=[x]+1 </strong> A) B)](https://storage.examlex.com/TB10479/11eef996_cb98_4a93_b4b4_3fa41bf9d10c_TB10479_00.jpg)
B)
![<strong>Graph the function. - y=[x]+1 </strong> A) B)](https://storage.examlex.com/TB10479/11eef996_cb98_4a94_b4b4_e1c3a2248a3b_TB10479_00.jpg)

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
78
Graph the function.
-
![<strong>Graph the function. - y=[x+1] </strong> A) B)](https://storage.examlex.com/TB10479/11eef996_cb98_71a5_b4b4_a9b17a09bdb1_TB10479_00.jpg)
A)![<strong>Graph the function. - y=[x+1] </strong> A) B)](https://storage.examlex.com/TB10479/11eef996_cb98_71a6_b4b4_7d1223827e9d_TB10479_00.jpg)
B)![<strong>Graph the function. - y=[x+1] </strong> A) B)](https://storage.examlex.com/TB10479/11eef996_cb98_71a7_b4b4_5ba109ef5370_TB10479_00.jpg)
-
![<strong>Graph the function. - y=[x+1] </strong> A) B)](https://storage.examlex.com/TB10479/11eef996_cb98_71a5_b4b4_a9b17a09bdb1_TB10479_00.jpg)
A)
![<strong>Graph the function. - y=[x+1] </strong> A) B)](https://storage.examlex.com/TB10479/11eef996_cb98_71a6_b4b4_7d1223827e9d_TB10479_00.jpg)
B)
![<strong>Graph the function. - y=[x+1] </strong> A) B)](https://storage.examlex.com/TB10479/11eef996_cb98_71a7_b4b4_5ba109ef5370_TB10479_00.jpg)

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
79
Graph the function.
-
![<strong>Graph the function. - y=[x]-1 </strong> A) B)](https://storage.examlex.com/TB10479/11eef996_cb98_71a8_b4b4_85132c445a03_TB10479_00.jpg)
A)![<strong>Graph the function. - y=[x]-1 </strong> A) B)](https://storage.examlex.com/TB10479/11eef996_cb98_71a9_b4b4_8d1fd638287a_TB10479_00.jpg)
B)![<strong>Graph the function. - y=[x]-1 </strong> A) B)](https://storage.examlex.com/TB10479/11eef996_cb98_71aa_b4b4_db46e366a301_TB10479_00.jpg)
-
![<strong>Graph the function. - y=[x]-1 </strong> A) B)](https://storage.examlex.com/TB10479/11eef996_cb98_71a8_b4b4_85132c445a03_TB10479_00.jpg)
A)
![<strong>Graph the function. - y=[x]-1 </strong> A) B)](https://storage.examlex.com/TB10479/11eef996_cb98_71a9_b4b4_8d1fd638287a_TB10479_00.jpg)
B)
![<strong>Graph the function. - y=[x]-1 </strong> A) B)](https://storage.examlex.com/TB10479/11eef996_cb98_71aa_b4b4_db46e366a301_TB10479_00.jpg)

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck
80
Graph the function.
-
![<strong>Graph the function. - y=[x-1] </strong> A) B)](https://storage.examlex.com/TB10479/11eef996_cb98_71ab_b4b4_efe8eaabb756_TB10479_00.jpg)
A)![<strong>Graph the function. - y=[x-1] </strong> A) B)](https://storage.examlex.com/TB10479/11eef996_cb98_71ac_b4b4_c31ce7251783_TB10479_00.jpg)
B)![<strong>Graph the function. - y=[x-1] </strong> A) B)](https://storage.examlex.com/TB10479/11eef996_cb98_98bd_b4b4_43394636e9a7_TB10479_00.jpg)
-
![<strong>Graph the function. - y=[x-1] </strong> A) B)](https://storage.examlex.com/TB10479/11eef996_cb98_71ab_b4b4_efe8eaabb756_TB10479_00.jpg)
A)
![<strong>Graph the function. - y=[x-1] </strong> A) B)](https://storage.examlex.com/TB10479/11eef996_cb98_71ac_b4b4_c31ce7251783_TB10479_00.jpg)
B)
![<strong>Graph the function. - y=[x-1] </strong> A) B)](https://storage.examlex.com/TB10479/11eef996_cb98_98bd_b4b4_43394636e9a7_TB10479_00.jpg)

Unlock Deck
Unlock for access to all 323 flashcards in this deck.
Unlock Deck
k this deck


