Deck 7: Extension G: Differential Equations
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/10
Play
Full screen (f)
Deck 7: Extension G: Differential Equations
1
Solve the initial-value problem.
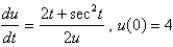
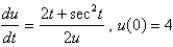

2
A tank contains 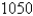 L of brine with
L of brine with 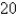 kg of dissolved salt.Pure water enters the tank at a rate of
kg of dissolved salt.Pure water enters the tank at a rate of 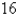 L/min.The solution is kept thoroughly mixed and drains from the tank at the same rate.How much salt is in the tank after
L/min.The solution is kept thoroughly mixed and drains from the tank at the same rate.How much salt is in the tank after 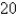 minutes?
minutes?
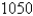 L of brine with
L of brine with 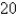 kg of dissolved salt.Pure water enters the tank at a rate of
kg of dissolved salt.Pure water enters the tank at a rate of 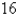 L/min.The solution is kept thoroughly mixed and drains from the tank at the same rate.How much salt is in the tank after
L/min.The solution is kept thoroughly mixed and drains from the tank at the same rate.How much salt is in the tank after 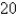 minutes?
minutes? kg
kg 3
A certain small country has $20 billion in paper currency in circulation,and each day $70 million comes into the country's banks.The government decides to introduce new currency by having the banks replace old bills with new ones whenever old currency comes into the banks.Let  denote the amount of new currency in circulation at time t with
denote the amount of new currency in circulation at time t with  Formulate and solve a mathematical model in the form of an initial-value problem that represents the "flow" of the new currency into circulation (in billions per day).
Formulate and solve a mathematical model in the form of an initial-value problem that represents the "flow" of the new currency into circulation (in billions per day).
 denote the amount of new currency in circulation at time t with
denote the amount of new currency in circulation at time t with  Formulate and solve a mathematical model in the form of an initial-value problem that represents the "flow" of the new currency into circulation (in billions per day).
Formulate and solve a mathematical model in the form of an initial-value problem that represents the "flow" of the new currency into circulation (in billions per day).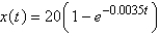
4
The Pacific halibut fishery has been modeled by the differential equation 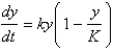 where
where 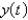 is the biomass (the total mass of the members of the population)in kilograms at time t (measured in years),the carrying capacity is estimated to be
is the biomass (the total mass of the members of the population)in kilograms at time t (measured in years),the carrying capacity is estimated to be 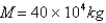 and
and 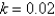 per year.If
per year.If 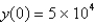 ,find the biomass a year later.
,find the biomass a year later.
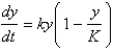 where
where 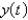 is the biomass (the total mass of the members of the population)in kilograms at time t (measured in years),the carrying capacity is estimated to be
is the biomass (the total mass of the members of the population)in kilograms at time t (measured in years),the carrying capacity is estimated to be 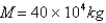 and
and 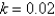 per year.If
per year.If 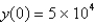 ,find the biomass a year later.
,find the biomass a year later.
Unlock Deck
Unlock for access to all 10 flashcards in this deck.
Unlock Deck
k this deck
5
One model for the spread of an epidemic is that the rate of spread is jointly proportional to the number of infected people and the number of uninfected people.In an isolated town of 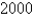 inhabitants,
inhabitants,  people have a disease at the beginning of the week and
people have a disease at the beginning of the week and  have it at the end of the week.How long does it take for
have it at the end of the week.How long does it take for 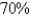 of the population to be infected?
of the population to be infected?
A)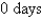
B)
C)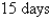
D)
E)
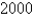 inhabitants,
inhabitants,  people have a disease at the beginning of the week and
people have a disease at the beginning of the week and  have it at the end of the week.How long does it take for
have it at the end of the week.How long does it take for 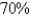 of the population to be infected?
of the population to be infected?A)
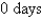
B)

C)
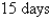
D)

E)


Unlock Deck
Unlock for access to all 10 flashcards in this deck.
Unlock Deck
k this deck
6
Biologists stocked a lake with 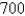 fish and estimated the carrying capacity (the maximal population for the fish of that species in that lake)to be
fish and estimated the carrying capacity (the maximal population for the fish of that species in that lake)to be  The number of fish tripled in the first year.Assuming that the size of the fish population satisfies the logistic equation,find an expression for the size of the population after t years.
The number of fish tripled in the first year.Assuming that the size of the fish population satisfies the logistic equation,find an expression for the size of the population after t years.
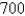 fish and estimated the carrying capacity (the maximal population for the fish of that species in that lake)to be
fish and estimated the carrying capacity (the maximal population for the fish of that species in that lake)to be  The number of fish tripled in the first year.Assuming that the size of the fish population satisfies the logistic equation,find an expression for the size of the population after t years.
The number of fish tripled in the first year.Assuming that the size of the fish population satisfies the logistic equation,find an expression for the size of the population after t years.
Unlock Deck
Unlock for access to all 10 flashcards in this deck.
Unlock Deck
k this deck
7
Solve the differential equation. 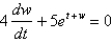
A)
B)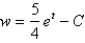
C)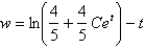
D)
E) none of these
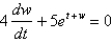
A)

B)
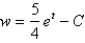
C)
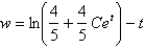
D)

E) none of these

Unlock Deck
Unlock for access to all 10 flashcards in this deck.
Unlock Deck
k this deck
8
Choose the differential equation corresponding to this direction field. 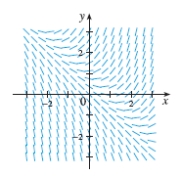
A)
B)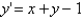
C)
D)
E)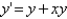

A)

B)
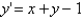
C)

D)

E)

Unlock Deck
Unlock for access to all 10 flashcards in this deck.
Unlock Deck
k this deck
9
Solve the differential equation. 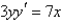
A)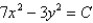
B)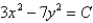
C)
D)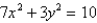
E)
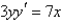
A)
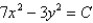
B)
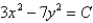
C)

D)
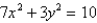
E)


Unlock Deck
Unlock for access to all 10 flashcards in this deck.
Unlock Deck
k this deck
10
Find the solution of the differential equation 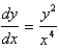 that satisfies the initial condition
that satisfies the initial condition 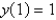
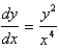 that satisfies the initial condition
that satisfies the initial condition 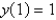

Unlock Deck
Unlock for access to all 10 flashcards in this deck.
Unlock Deck
k this deck


