Deck 3: Section 8: Differentiation
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/12
Play
Full screen (f)
Deck 3: Section 8: Differentiation
1
Complete two iterations of Newton's Method for the function 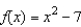 using the initial guess
using the initial guess 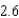 . Round your answers to four decimal places.
. Round your answers to four decimal places.
A)
B)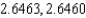
C)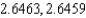
D)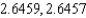
E)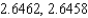
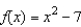 using the initial guess
using the initial guess 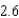 . Round your answers to four decimal places.
. Round your answers to four decimal places.A)

B)
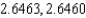
C)
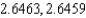
D)
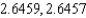
E)
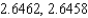
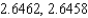
2
Suppose that the total number of arrests T (in thousands) for all males ages 14 to 27 in 2006 is approximated by the model 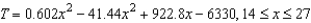 where x is the age in years (see figure). Approximate the two ages to one decimal place that had total arrests of
where x is the age in years (see figure). Approximate the two ages to one decimal place that had total arrests of  thousand.
thousand. 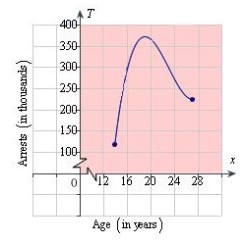
A)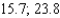
B)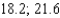
C)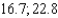
D)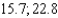
E)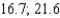
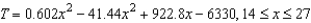 where x is the age in years (see figure). Approximate the two ages to one decimal place that had total arrests of
where x is the age in years (see figure). Approximate the two ages to one decimal place that had total arrests of  thousand.
thousand. 
A)
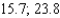
B)
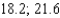
C)
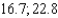
D)
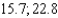
E)
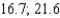
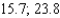
3
Apply Newton's Method to approximate the x-value of the indicated point of intersection of ![<strong>Apply Newton's Method to approximate the x-value of the indicated point of intersection of Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] Round your answer to three decimal places. </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6609_7f4d_a696_bfb1bef59e1c_TB4584_11_TB4584_11.jpg) Continue the process until two successive approximations differ by less than 0.001. [Hint: Let
Continue the process until two successive approximations differ by less than 0.001. [Hint: Let ![<strong>Apply Newton's Method to approximate the x-value of the indicated point of intersection of Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] Round your answer to three decimal places. </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6609_7f4e_a696_875a52cc469f_TB4584_11_TB4584_11.jpg) .] Round your answer to three decimal places.
.] Round your answer to three decimal places. ![<strong>Apply Newton's Method to approximate the x-value of the indicated point of intersection of Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] Round your answer to three decimal places. </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6609_a65f_a696_8df6267cc9c2_TB4584_00_TB4584_00.jpg)
A)![<strong>Apply Newton's Method to approximate the x-value of the indicated point of intersection of Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] Round your answer to three decimal places. </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6609_cd70_a696_c35766a656eb_TB4584_11_TB4584_11.jpg)
B)![<strong>Apply Newton's Method to approximate the x-value of the indicated point of intersection of Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] Round your answer to three decimal places. </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6609_f481_a696_9985b69bbd7f_TB4584_11_TB4584_11.jpg)
C)![<strong>Apply Newton's Method to approximate the x-value of the indicated point of intersection of Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] Round your answer to three decimal places. </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_660a_1b92_a696_27bf843fdfa8_TB4584_11_TB4584_11.jpg)
D)![<strong>Apply Newton's Method to approximate the x-value of the indicated point of intersection of Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] Round your answer to three decimal places. </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_660a_1b93_a696_cdef2cea802e_TB4584_11_TB4584_11.jpg)
E)![<strong>Apply Newton's Method to approximate the x-value of the indicated point of intersection of Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] Round your answer to three decimal places. </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_660a_42a4_a696_35e498a78b3f_TB4584_11_TB4584_11.jpg)
![<strong>Apply Newton's Method to approximate the x-value of the indicated point of intersection of Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] Round your answer to three decimal places. </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6609_7f4d_a696_bfb1bef59e1c_TB4584_11_TB4584_11.jpg) Continue the process until two successive approximations differ by less than 0.001. [Hint: Let
Continue the process until two successive approximations differ by less than 0.001. [Hint: Let ![<strong>Apply Newton's Method to approximate the x-value of the indicated point of intersection of Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] Round your answer to three decimal places. </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6609_7f4e_a696_875a52cc469f_TB4584_11_TB4584_11.jpg) .] Round your answer to three decimal places.
.] Round your answer to three decimal places. ![<strong>Apply Newton's Method to approximate the x-value of the indicated point of intersection of Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] Round your answer to three decimal places. </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6609_a65f_a696_8df6267cc9c2_TB4584_00_TB4584_00.jpg)
A)
![<strong>Apply Newton's Method to approximate the x-value of the indicated point of intersection of Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] Round your answer to three decimal places. </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6609_cd70_a696_c35766a656eb_TB4584_11_TB4584_11.jpg)
B)
![<strong>Apply Newton's Method to approximate the x-value of the indicated point of intersection of Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] Round your answer to three decimal places. </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6609_f481_a696_9985b69bbd7f_TB4584_11_TB4584_11.jpg)
C)
![<strong>Apply Newton's Method to approximate the x-value of the indicated point of intersection of Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] Round your answer to three decimal places. </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_660a_1b92_a696_27bf843fdfa8_TB4584_11_TB4584_11.jpg)
D)
![<strong>Apply Newton's Method to approximate the x-value of the indicated point of intersection of Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] Round your answer to three decimal places. </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_660a_1b93_a696_cdef2cea802e_TB4584_11_TB4584_11.jpg)
E)
![<strong>Apply Newton's Method to approximate the x-value of the indicated point of intersection of Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] Round your answer to three decimal places. </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_660a_42a4_a696_35e498a78b3f_TB4584_11_TB4584_11.jpg)
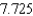
4
Use Newton´s Method to approximate the x-value of the indicated point of intersection of the two graphs accurate to three decimal places.Continue the process until two successive approximations differ by less than 0.001. [Hint: Let ![<strong>Use Newton´s Method to approximate the x-value of the indicated point of intersection of the two graphs accurate to three decimal places.Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6607_f89c_a696_cbb0176b9fa4_TB4584_11_TB4584_11.jpg) .]
.] ![<strong>Use Newton´s Method to approximate the x-value of the indicated point of intersection of the two graphs accurate to three decimal places.Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6607_f89d_a696_dd29157046ad_TB4584_00_TB4584_00.jpg)
![<strong>Use Newton´s Method to approximate the x-value of the indicated point of intersection of the two graphs accurate to three decimal places.Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6607_f89e_a696_55ae741fcc09_TB4584_00_TB4584_00.jpg)
![<strong>Use Newton´s Method to approximate the x-value of the indicated point of intersection of the two graphs accurate to three decimal places.Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6608_1faf_a696_57a52513685c_TB4584_00_TB4584_00.jpg)
A)![<strong>Use Newton´s Method to approximate the x-value of the indicated point of intersection of the two graphs accurate to three decimal places.Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6608_1fb0_a696_b70497fef4e9_TB4584_11_TB4584_11.jpg)
B)![<strong>Use Newton´s Method to approximate the x-value of the indicated point of intersection of the two graphs accurate to three decimal places.Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6608_46c1_a696_e5b5c6630e69_TB4584_11_TB4584_11.jpg)
C)![<strong>Use Newton´s Method to approximate the x-value of the indicated point of intersection of the two graphs accurate to three decimal places.Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6608_46c2_a696_3d4d0a2f7e23_TB4584_11_TB4584_11.jpg)
D)![<strong>Use Newton´s Method to approximate the x-value of the indicated point of intersection of the two graphs accurate to three decimal places.Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6608_6dd3_a696_5b615414bbd9_TB4584_11_TB4584_11.jpg)
E)![<strong>Use Newton´s Method to approximate the x-value of the indicated point of intersection of the two graphs accurate to three decimal places.Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6608_94e4_a696_95e47aa2db86_TB4584_11_TB4584_11.jpg)
![<strong>Use Newton´s Method to approximate the x-value of the indicated point of intersection of the two graphs accurate to three decimal places.Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6607_f89c_a696_cbb0176b9fa4_TB4584_11_TB4584_11.jpg) .]
.] ![<strong>Use Newton´s Method to approximate the x-value of the indicated point of intersection of the two graphs accurate to three decimal places.Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6607_f89d_a696_dd29157046ad_TB4584_00_TB4584_00.jpg)
![<strong>Use Newton´s Method to approximate the x-value of the indicated point of intersection of the two graphs accurate to three decimal places.Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6607_f89e_a696_55ae741fcc09_TB4584_00_TB4584_00.jpg)
![<strong>Use Newton´s Method to approximate the x-value of the indicated point of intersection of the two graphs accurate to three decimal places.Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6608_1faf_a696_57a52513685c_TB4584_00_TB4584_00.jpg)
A)
![<strong>Use Newton´s Method to approximate the x-value of the indicated point of intersection of the two graphs accurate to three decimal places.Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6608_1fb0_a696_b70497fef4e9_TB4584_11_TB4584_11.jpg)
B)
![<strong>Use Newton´s Method to approximate the x-value of the indicated point of intersection of the two graphs accurate to three decimal places.Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6608_46c1_a696_e5b5c6630e69_TB4584_11_TB4584_11.jpg)
C)
![<strong>Use Newton´s Method to approximate the x-value of the indicated point of intersection of the two graphs accurate to three decimal places.Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6608_46c2_a696_3d4d0a2f7e23_TB4584_11_TB4584_11.jpg)
D)
![<strong>Use Newton´s Method to approximate the x-value of the indicated point of intersection of the two graphs accurate to three decimal places.Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6608_6dd3_a696_5b615414bbd9_TB4584_11_TB4584_11.jpg)
E)
![<strong>Use Newton´s Method to approximate the x-value of the indicated point of intersection of the two graphs accurate to three decimal places.Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6608_94e4_a696_95e47aa2db86_TB4584_11_TB4584_11.jpg)

Unlock Deck
Unlock for access to all 12 flashcards in this deck.
Unlock Deck
k this deck
5
A manufacturer of digital audio players estimates that the profit for selling a particular model is 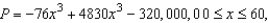 where P is the profit in dollars and x is the advertising expense in 10,000's of dollars (see figure). Find the smaller of two advertising amounts that yield a profit P of $
where P is the profit in dollars and x is the advertising expense in 10,000's of dollars (see figure). Find the smaller of two advertising amounts that yield a profit P of $ 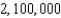 . Round your answer to the nearest dollar.
. Round your answer to the nearest dollar. 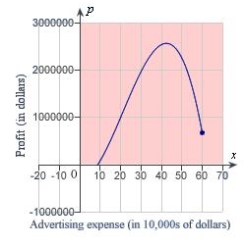
A)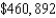
B)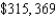
C)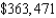
D)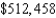
E)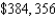
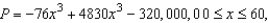 where P is the profit in dollars and x is the advertising expense in 10,000's of dollars (see figure). Find the smaller of two advertising amounts that yield a profit P of $
where P is the profit in dollars and x is the advertising expense in 10,000's of dollars (see figure). Find the smaller of two advertising amounts that yield a profit P of $ 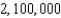 . Round your answer to the nearest dollar.
. Round your answer to the nearest dollar. 
A)
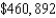
B)
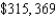
C)
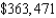
D)
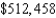
E)
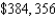

Unlock Deck
Unlock for access to all 12 flashcards in this deck.
Unlock Deck
k this deck
6
Use Newton's Method to approximate the zero(s) of the function 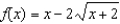 accurate to three decimal places.
accurate to three decimal places.
A)
B)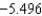
C)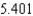
D)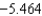
E)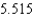
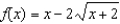 accurate to three decimal places.
accurate to three decimal places.A)

B)
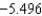
C)
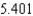
D)
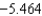
E)
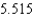

Unlock Deck
Unlock for access to all 12 flashcards in this deck.
Unlock Deck
k this deck
7
Use Newton´s Method to approximate the zero(s) of the function 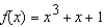 accurate to three decimal places.
accurate to three decimal places.
A)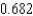
B)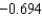
C)
D)
E)
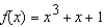 accurate to three decimal places.
accurate to three decimal places.A)
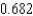
B)
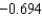
C)

D)

E)


Unlock Deck
Unlock for access to all 12 flashcards in this deck.
Unlock Deck
k this deck
8
Use Newton's Method to approximate the zero(s) of the function 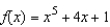 accurate to three decimal places.
accurate to three decimal places.
A)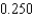
B)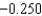
C)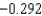
D)
E)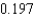
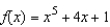 accurate to three decimal places.
accurate to three decimal places.A)
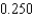
B)
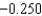
C)
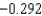
D)

E)
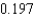

Unlock Deck
Unlock for access to all 12 flashcards in this deck.
Unlock Deck
k this deck
9
Approximate the positive zero(s) of the function 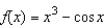 to three decimal places. Use Newton's Method and continue the process until two successive approximations differ by less than 0.001.
to three decimal places. Use Newton's Method and continue the process until two successive approximations differ by less than 0.001.
A)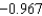
B)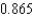
C)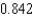
D)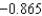
E)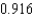
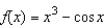 to three decimal places. Use Newton's Method and continue the process until two successive approximations differ by less than 0.001.
to three decimal places. Use Newton's Method and continue the process until two successive approximations differ by less than 0.001.A)
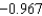
B)
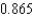
C)
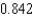
D)
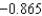
E)
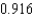

Unlock Deck
Unlock for access to all 12 flashcards in this deck.
Unlock Deck
k this deck
10
Approximate the fixed point of the function ![<strong>Approximate the fixed point of the function between to two decimal places. [A of a function f is a value of x such that .]</strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_660a_69b5_a696_ad16604d32c7_TB4584_11_TB4584_11.jpg) between
between ![<strong>Approximate the fixed point of the function between to two decimal places. [A of a function f is a value of x such that .]</strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_660a_69b6_a696_5385491cb3f7_TB4584_11_TB4584_11.jpg) to two decimal places. [A
to two decimal places. [A ![<strong>Approximate the fixed point of the function between to two decimal places. [A of a function f is a value of x such that .]</strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_660a_90c7_a696_2f2139759300_TB4584_11_TB4584_11.jpg) of a function f is a value of x such that
of a function f is a value of x such that ![<strong>Approximate the fixed point of the function between to two decimal places. [A of a function f is a value of x such that .]</strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_660a_90c8_a696_f112da3761d3_TB4584_11_TB4584_11.jpg) .]
.]
A)![<strong>Approximate the fixed point of the function between to two decimal places. [A of a function f is a value of x such that .]</strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_660a_b7d9_a696_af3e54aae378_TB4584_11_TB4584_11.jpg)
B)![<strong>Approximate the fixed point of the function between to two decimal places. [A of a function f is a value of x such that .]</strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_660a_b7da_a696_474c3279d1f8_TB4584_11_TB4584_11.jpg)
C)![<strong>Approximate the fixed point of the function between to two decimal places. [A of a function f is a value of x such that .]</strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_660a_deeb_a696_3169d7b8819d_TB4584_11_TB4584_11.jpg)
D)![<strong>Approximate the fixed point of the function between to two decimal places. [A of a function f is a value of x such that .]</strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_660b_05fc_a696_1b3cf133d8f8_TB4584_11_TB4584_11.jpg)
E)![<strong>Approximate the fixed point of the function between to two decimal places. [A of a function f is a value of x such that .]</strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_660b_05fd_a696_39f8901c4cfe_TB4584_11_TB4584_11.jpg)
![<strong>Approximate the fixed point of the function between to two decimal places. [A of a function f is a value of x such that .]</strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_660a_69b5_a696_ad16604d32c7_TB4584_11_TB4584_11.jpg) between
between ![<strong>Approximate the fixed point of the function between to two decimal places. [A of a function f is a value of x such that .]</strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_660a_69b6_a696_5385491cb3f7_TB4584_11_TB4584_11.jpg) to two decimal places. [A
to two decimal places. [A ![<strong>Approximate the fixed point of the function between to two decimal places. [A of a function f is a value of x such that .]</strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_660a_90c7_a696_2f2139759300_TB4584_11_TB4584_11.jpg) of a function f is a value of x such that
of a function f is a value of x such that ![<strong>Approximate the fixed point of the function between to two decimal places. [A of a function f is a value of x such that .]</strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_660a_90c8_a696_f112da3761d3_TB4584_11_TB4584_11.jpg) .]
.]A)
![<strong>Approximate the fixed point of the function between to two decimal places. [A of a function f is a value of x such that .]</strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_660a_b7d9_a696_af3e54aae378_TB4584_11_TB4584_11.jpg)
B)
![<strong>Approximate the fixed point of the function between to two decimal places. [A of a function f is a value of x such that .]</strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_660a_b7da_a696_474c3279d1f8_TB4584_11_TB4584_11.jpg)
C)
![<strong>Approximate the fixed point of the function between to two decimal places. [A of a function f is a value of x such that .]</strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_660a_deeb_a696_3169d7b8819d_TB4584_11_TB4584_11.jpg)
D)
![<strong>Approximate the fixed point of the function between to two decimal places. [A of a function f is a value of x such that .]</strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_660b_05fc_a696_1b3cf133d8f8_TB4584_11_TB4584_11.jpg)
E)
![<strong>Approximate the fixed point of the function between to two decimal places. [A of a function f is a value of x such that .]</strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_660b_05fd_a696_39f8901c4cfe_TB4584_11_TB4584_11.jpg)

Unlock Deck
Unlock for access to all 12 flashcards in this deck.
Unlock Deck
k this deck
11
Use Newton's Method to approximate the x-value of the indicated point of intersection of the two graphs accurate to three decimal places.Continue the process until two successive approximations differ by less than 0.001. [Hint: Let ![<strong>Use Newton's Method to approximate the x-value of the indicated point of intersection of the two graphs accurate to three decimal places.Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6608_bbf5_a696_958c99954c2d_TB4584_11_TB4584_11.jpg) .]
.] ![<strong>Use Newton's Method to approximate the x-value of the indicated point of intersection of the two graphs accurate to three decimal places.Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6608_bbf6_a696_010ba5867d35_TB4584_11_TB4584_11.jpg)
![<strong>Use Newton's Method to approximate the x-value of the indicated point of intersection of the two graphs accurate to three decimal places.Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6608_bbf7_a696_4bee90c14861_TB4584_00_TB4584_00.jpg)
A)![<strong>Use Newton's Method to approximate the x-value of the indicated point of intersection of the two graphs accurate to three decimal places.Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6608_e308_a696_3f71ff183f07_TB4584_11_TB4584_11.jpg)
B)![<strong>Use Newton's Method to approximate the x-value of the indicated point of intersection of the two graphs accurate to three decimal places.Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6608_e309_a696_2be2facaf94f_TB4584_11_TB4584_11.jpg)
C)![<strong>Use Newton's Method to approximate the x-value of the indicated point of intersection of the two graphs accurate to three decimal places.Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6609_0a1a_a696_5585d85c7db6_TB4584_11_TB4584_11.jpg)
D)![<strong>Use Newton's Method to approximate the x-value of the indicated point of intersection of the two graphs accurate to three decimal places.Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6609_312b_a696_d71e4e063563_TB4584_11_TB4584_11.jpg)
E)![<strong>Use Newton's Method to approximate the x-value of the indicated point of intersection of the two graphs accurate to three decimal places.Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6609_312c_a696_d1c4d4bc6158_TB4584_11_TB4584_11.jpg)
![<strong>Use Newton's Method to approximate the x-value of the indicated point of intersection of the two graphs accurate to three decimal places.Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6608_bbf5_a696_958c99954c2d_TB4584_11_TB4584_11.jpg) .]
.] ![<strong>Use Newton's Method to approximate the x-value of the indicated point of intersection of the two graphs accurate to three decimal places.Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6608_bbf6_a696_010ba5867d35_TB4584_11_TB4584_11.jpg)
![<strong>Use Newton's Method to approximate the x-value of the indicated point of intersection of the two graphs accurate to three decimal places.Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6608_bbf7_a696_4bee90c14861_TB4584_00_TB4584_00.jpg)
A)
![<strong>Use Newton's Method to approximate the x-value of the indicated point of intersection of the two graphs accurate to three decimal places.Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6608_e308_a696_3f71ff183f07_TB4584_11_TB4584_11.jpg)
B)
![<strong>Use Newton's Method to approximate the x-value of the indicated point of intersection of the two graphs accurate to three decimal places.Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6608_e309_a696_2be2facaf94f_TB4584_11_TB4584_11.jpg)
C)
![<strong>Use Newton's Method to approximate the x-value of the indicated point of intersection of the two graphs accurate to three decimal places.Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6609_0a1a_a696_5585d85c7db6_TB4584_11_TB4584_11.jpg)
D)
![<strong>Use Newton's Method to approximate the x-value of the indicated point of intersection of the two graphs accurate to three decimal places.Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6609_312b_a696_d71e4e063563_TB4584_11_TB4584_11.jpg)
E)
![<strong>Use Newton's Method to approximate the x-value of the indicated point of intersection of the two graphs accurate to three decimal places.Continue the process until two successive approximations differ by less than 0.001. [Hint: Let .] </strong> A) B) C) D) E)](https://storage.examlex.com/TB4584/11eaa595_6609_312c_a696_d1c4d4bc6158_TB4584_11_TB4584_11.jpg)

Unlock Deck
Unlock for access to all 12 flashcards in this deck.
Unlock Deck
k this deck
12
Complete two iterations of Newton's Method for the function 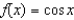 using the initial guess
using the initial guess 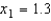 . Round all numerical values in your answer to four decimal places.
. Round all numerical values in your answer to four decimal places.
A)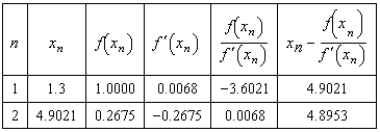
B)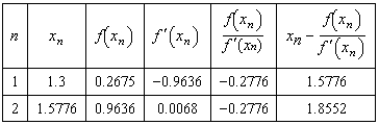
C)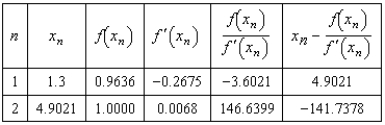
D)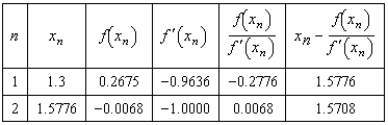
E)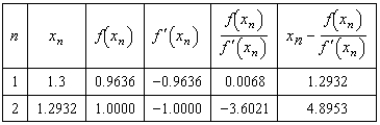
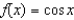 using the initial guess
using the initial guess 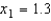 . Round all numerical values in your answer to four decimal places.
. Round all numerical values in your answer to four decimal places.A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 12 flashcards in this deck.
Unlock Deck
k this deck


