Deck 4: Applications of the Derivative
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/77
Play
Full screen (f)
Deck 4: Applications of the Derivative
1
Find the linearization of the given function centered at 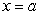 , and use it to estimate
, and use it to estimate 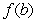 .
.
A)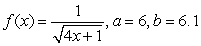
B)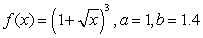
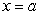 , and use it to estimate
, and use it to estimate 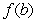 .
. A)
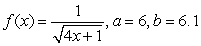
B)
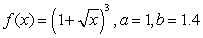
A) 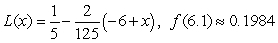 B)
B) 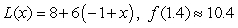
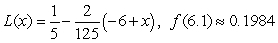 B)
B) 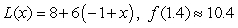
2
A ball produced on an assembly line is supposed to have a volume of 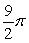
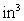 . Use linearization to estimate the maximum allowable error in the radius
. Use linearization to estimate the maximum allowable error in the radius 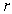 if the volume of the sphere must have an error of less than
if the volume of the sphere must have an error of less than 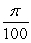
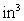 .
.
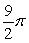
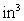 . Use linearization to estimate the maximum allowable error in the radius
. Use linearization to estimate the maximum allowable error in the radius 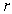 if the volume of the sphere must have an error of less than
if the volume of the sphere must have an error of less than 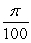
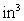 .
.Radius error must be less than 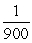 inch.
inch.
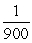 inch.
inch. 3
By Rolle's Theorem the equation 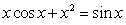 has at most two roots.
has at most two roots.
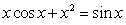 has at most two roots.
has at most two roots.True
4
The radius  of a sphere is measured at 4 m. Use linear approximation to estimate the maximum error in the surface area of the sphere if
of a sphere is measured at 4 m. Use linear approximation to estimate the maximum error in the surface area of the sphere if 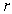 is accurate to within
is accurate to within 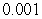 m.
m.
 of a sphere is measured at 4 m. Use linear approximation to estimate the maximum error in the surface area of the sphere if
of a sphere is measured at 4 m. Use linear approximation to estimate the maximum error in the surface area of the sphere if 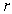 is accurate to within
is accurate to within 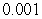 m.
m.
Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
5
Find the maximum value 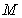 and the minimum value
and the minimum value 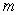 of the function in the given interval
of the function in the given interval
A)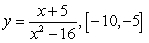
B)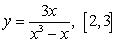
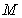 and the minimum value
and the minimum value 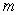 of the function in the given interval
of the function in the given interval A)
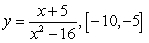
B)
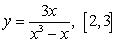

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
6
Find the maximum value 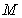 and the minimum value
and the minimum value  of the function in the given interval
of the function in the given interval
A)
B)
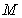 and the minimum value
and the minimum value  of the function in the given interval
of the function in the given interval A)
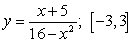
B)


Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
7
Find the maximum value 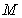 and the minimum value
and the minimum value 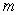 of the function in the given interval or in its natural domain
of the function in the given interval or in its natural domain
A)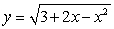
B)
C)
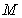 and the minimum value
and the minimum value 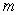 of the function in the given interval or in its natural domain
of the function in the given interval or in its natural domain A)
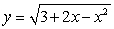
B)

C)


Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
8
Estimate 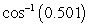 using linear approximation.
using linear approximation.
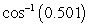 using linear approximation.
using linear approximation.
Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
9
The minimum and maximum of which of the following functions does not occur at a critical point in the open interval:
A)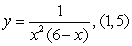
B)
C)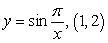
D)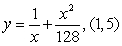
E)
A)
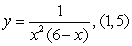
B)

C)
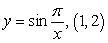
D)
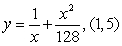
E)


Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
10
Let 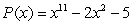 . By Rolle's Theorem we can prove that
. By Rolle's Theorem we can prove that
A)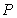 has 11 real roots.
has 11 real roots.
B)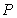 has at most 3 real roots.
has at most 3 real roots.
C)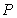 has no real roots.
has no real roots.
D)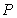 has at most 2 real roots.
has at most 2 real roots.
E)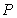 has 10 real roots.
has 10 real roots.
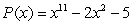 . By Rolle's Theorem we can prove that
. By Rolle's Theorem we can prove thatA)
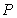 has 11 real roots.
has 11 real roots.B)
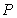 has at most 3 real roots.
has at most 3 real roots.C)
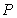 has no real roots.
has no real roots.D)
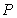 has at most 2 real roots.
has at most 2 real roots.E)
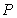 has 10 real roots.
has 10 real roots.
Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
11
Find the maximum value 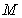 and the minimum value
and the minimum value 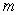 of the function in the given interval.
of the function in the given interval.
A)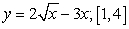
B)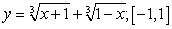
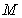 and the minimum value
and the minimum value 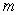 of the function in the given interval.
of the function in the given interval. A)
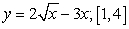
B)
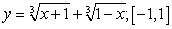

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
12
Each of the following functions restricted to the given interval attains a minimum and maximum value. Which attain their maximum or minimum value at a point in the interior of the interval?
A)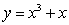 on
on 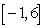
B)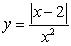 on
on 
C)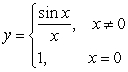 on
on 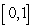
D)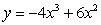 on
on 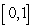
A)
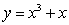 on
on 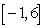
B)
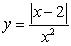 on
on 
C)
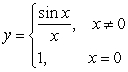 on
on 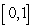
D)
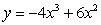 on
on 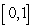

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
13
Estimate the length of the hypotenuse in a right triangle with sides 3 and 4.01, using the linear approximation for  .
.
 .
.
Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
14
Find the linearization of the given function centered at 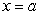 and use it to estimate
and use it to estimate 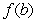 .
.
A)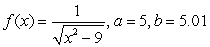
B)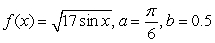
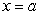 and use it to estimate
and use it to estimate 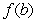 .
. A)
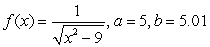
B)
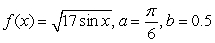

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
15
Estimate the roots of the equation 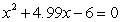 to three decimal places using the linear approximation for
to three decimal places using the linear approximation for 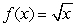 .
.
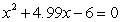 to three decimal places using the linear approximation for
to three decimal places using the linear approximation for 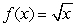 .
.
Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
16
Estimate the roots of the equation 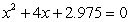 using the linear approximation for
using the linear approximation for  at
at 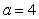 .
.
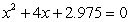 using the linear approximation for
using the linear approximation for  at
at 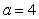 .
.
Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
17
Estimate the roots of the equation 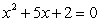 using the linear approximation for
using the linear approximation for 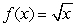 .
.
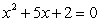 using the linear approximation for
using the linear approximation for 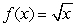 .
.
Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
18
Estimate the value of 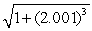 using the linear approximation and find the error using a calculator.
using the linear approximation and find the error using a calculator.
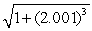 using the linear approximation and find the error using a calculator.
using the linear approximation and find the error using a calculator.
Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
19
Suppose that a function 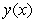 satisfies the following equation for small values of
satisfies the following equation for small values of  :
: 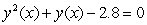 .
.
Also,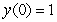 and
and 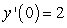 .
.
A) Find the linearization of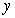 at
at 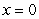 .
.
B) Replace by its linearization and find a quadratic equation for
by its linearization and find a quadratic equation for 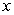 .
.
C) Estimate the roots of the quadratic equation in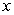 to 4 decimal digits using linearization for
to 4 decimal digits using linearization for 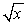 .
.
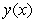 satisfies the following equation for small values of
satisfies the following equation for small values of  :
: 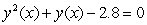 .
.Also,
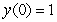 and
and 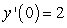 .
. A) Find the linearization of
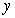 at
at 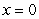 .
. B) Replace
 by its linearization and find a quadratic equation for
by its linearization and find a quadratic equation for 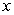 .
. C) Estimate the roots of the quadratic equation in
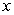 to 4 decimal digits using linearization for
to 4 decimal digits using linearization for 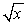 .
.
Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
20
Find all the critical points of the function
A)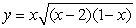
B)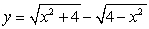
A)
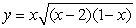
B)
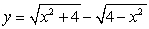

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
21
The following function has a local extremum at a point in the interval 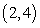 :
:
A)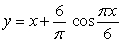
B)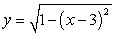
C)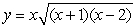
D)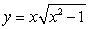
E) None of the above.
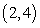 :
:A)
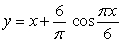
B)
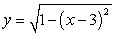
C)
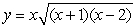
D)
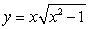
E) None of the above.

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
22
Determine the greater between 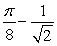 and
and 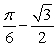 without using a calculator, by considering the function
without using a calculator, by considering the function 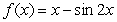 for
for 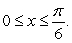
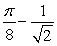 and
and 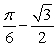 without using a calculator, by considering the function
without using a calculator, by considering the function 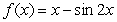 for
for 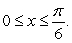

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
23
The function 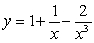 has
has
A) local minimum at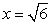 , inflection at
, inflection at  .
.
B) local minimum at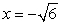 , local maximum at
, local maximum at 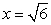 , inflection at
, inflection at 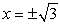 .
.
C) local minimum at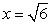 , local maximum at
, local maximum at 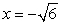 , inflection at
, inflection at 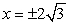 .
.
D) local minimum at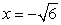 , local maximum at
, local maximum at 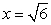 , inflection at
, inflection at 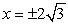 .
.
E) None of the above.
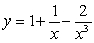 has
hasA) local minimum at
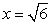 , inflection at
, inflection at  .
.B) local minimum at
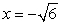 , local maximum at
, local maximum at 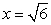 , inflection at
, inflection at 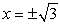 .
.C) local minimum at
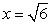 , local maximum at
, local maximum at 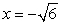 , inflection at
, inflection at 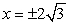 .
.D) local minimum at
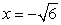 , local maximum at
, local maximum at 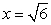 , inflection at
, inflection at 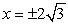 .
.E) None of the above.

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
24
The increase/decrease intervals of the function 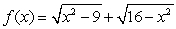 are
are
A) increasing on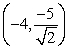 ,
, 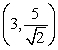 and decreasing on
and decreasing on 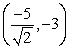
 .
.
B) increasing on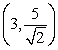 and decreasing on
and decreasing on 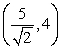 .
.
C) increasing on and decreasing on
and decreasing on 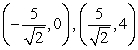 .
.
D) increasing on and decreasing on
and decreasing on 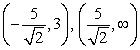 .
.
E) None of the above.
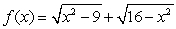 are
areA) increasing on
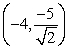 ,
, 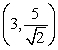 and decreasing on
and decreasing on 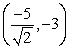
 .
.B) increasing on
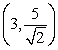 and decreasing on
and decreasing on 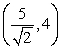 .
.C) increasing on
 and decreasing on
and decreasing on 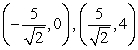 .
.D) increasing on
 and decreasing on
and decreasing on 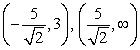 .
.E) None of the above.

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
25
Given 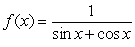 ,
,
Which of the following statements is correct?
A)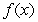 is increasing on
is increasing on 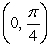 and decreasing on
and decreasing on 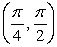 .
.
B) is increasing on
is increasing on 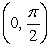 .
.
C) is decreasing on
is decreasing on 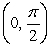 .
.
D)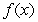 is decreasing on
is decreasing on  and increasing on
and increasing on  .
.
E)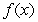 is decreasing on
is decreasing on 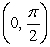 and increasing on
and increasing on 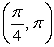 .
.
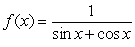 ,
,Which of the following statements is correct?
A)
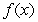 is increasing on
is increasing on 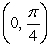 and decreasing on
and decreasing on 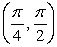 .
.B)
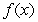 is increasing on
is increasing on 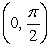 .
.C)
 is decreasing on
is decreasing on 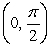 .
.D)
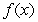 is decreasing on
is decreasing on  and increasing on
and increasing on  .
.E)
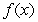 is decreasing on
is decreasing on 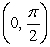 and increasing on
and increasing on 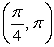 .
.
Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
26
Given the function 
 is
is
A) a local maximum point.
B) a local minimum point.
C) is not a point of local extremum nor an inflection point.
D) an inflection point.
E) not in the domain of the function.

 is
isA) a local maximum point.
B) a local minimum point.
C) is not a point of local extremum nor an inflection point.
D) an inflection point.
E) not in the domain of the function.

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
27
Find the maximum value 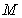 and the minimum value
and the minimum value  of the function in the given interval.
of the function in the given interval.
A)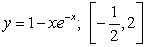
B)
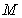 and the minimum value
and the minimum value  of the function in the given interval.
of the function in the given interval. A)
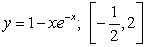
B)


Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
28
Given 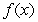 such that
such that 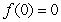 and
and 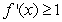 for all
for all 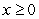 , is
, is  for all
for all 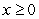 ?
?
(Hint: Use the MVT.)
A) Yes
B) No
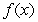 such that
such that 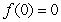 and
and 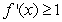 for all
for all 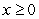 , is
, is  for all
for all 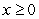 ?
?(Hint: Use the MVT.)
A) Yes
B) No

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
29
Find a point 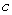 satisfying the conclusion of the Mean Value Theorem for the function
satisfying the conclusion of the Mean Value Theorem for the function  in the interval
in the interval  .
.
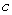 satisfying the conclusion of the Mean Value Theorem for the function
satisfying the conclusion of the Mean Value Theorem for the function  in the interval
in the interval  .
.
Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
30
Find all the critical points of the following functions (if they exist)
A)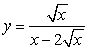
B)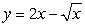
C)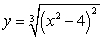
A)
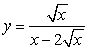
B)
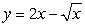
C)
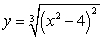

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
31
Let 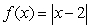 .
.
A) Show that there is no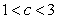 such that
such that  .
.
B) Explain why the above does not contradict the MVT.
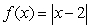 .
. A) Show that there is no
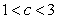 such that
such that  .
. B) Explain why the above does not contradict the MVT.

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
32
The following function has a local minimum at a point on the interval 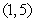 :
:
A)
B)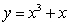
C)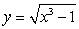
D)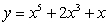
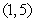 :
: A)

B)
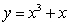
C)
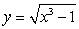
D)
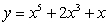

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
33
Which of the following functions has a local maximum at a point in the interval 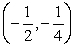 :
:
A)
B)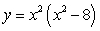
C)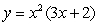
D)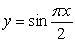
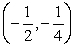 :
: A)

B)
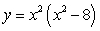
C)
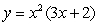
D)
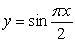

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
34
Given 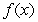 such that
such that 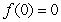 and
and 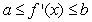 for all
for all 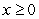 .
.
Use the Mean Value Theorem to find which of the following statements is correct
A)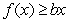
B)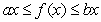
C)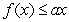
D)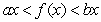
E) None of the above.
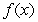 such that
such that 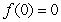 and
and 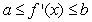 for all
for all 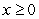 .
.Use the Mean Value Theorem to find which of the following statements is correct
A)
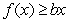
B)
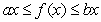
C)
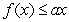
D)
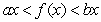
E) None of the above.

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
35
Let  for
for  Based on the MVT, which of the following statements is correct?
Based on the MVT, which of the following statements is correct?
A)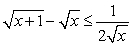
B)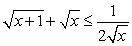
C)
D)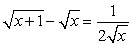
E)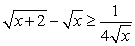
 for
for  Based on the MVT, which of the following statements is correct?
Based on the MVT, which of the following statements is correct?A)
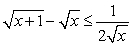
B)
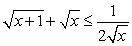
C)

D)
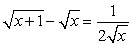
E)
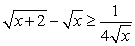

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
36
True or False: Let 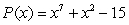 . By Rolle's Theorem,
. By Rolle's Theorem, 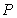 has at most 3 real roots.
has at most 3 real roots.
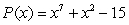 . By Rolle's Theorem,
. By Rolle's Theorem, 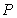 has at most 3 real roots.
has at most 3 real roots.
Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
37
Suppose that 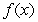 is differentiable for
is differentiable for 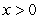 ,
, 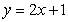 is the tangent line to the graph of
is the tangent line to the graph of 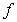 at
at 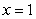 and
and 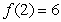 . The following can be concluded:
. The following can be concluded:
A)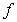 is concave up on
is concave up on 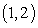 .
.
B)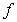 is not concave down on
is not concave down on 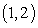 .
.
C)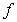 is concave down on
is concave down on 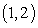 .
.
D) is not concave up on
is not concave up on  .
.
E) None of the above.
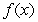 is differentiable for
is differentiable for 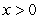 ,
, 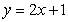 is the tangent line to the graph of
is the tangent line to the graph of 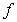 at
at 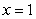 and
and 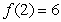 . The following can be concluded:
. The following can be concluded:A)
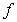 is concave up on
is concave up on 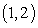 .
.B)
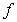 is not concave down on
is not concave down on 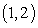 .
.C)
 is concave down on
is concave down on 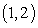 .
.D)
 is not concave up on
is not concave up on  .
.E) None of the above.

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
38
Find the intervals on which the function is concave up and concave down and indicate the points of inflection
A)
B)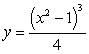
A)

B)
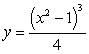

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
39
Let 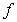 be the function on
be the function on 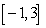 given by
given by 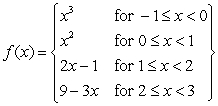 and the graph:
and the graph: 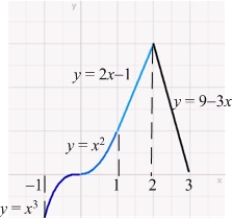 The MVT can be applied on the following interval:
The MVT can be applied on the following interval:
A)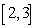
B)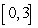
C)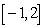
D)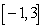
E) A and C are correct.
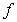 be the function on
be the function on 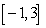 given by
given by 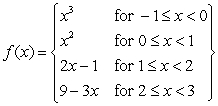 and the graph:
and the graph:  The MVT can be applied on the following interval:
The MVT can be applied on the following interval:A)
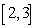
B)
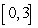
C)
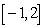
D)
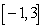
E) A and C are correct.

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
40
The function 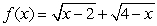 is
is
A) increasing on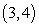 and decreasing on
and decreasing on 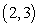 .
.
B) increasing on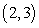 and decreasing on
and decreasing on 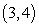 .
.
C) increasing on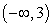 and decreasing on
and decreasing on 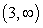 .
.
D) increasing in all its domain.
E) decreasing in all its domain.
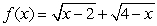 is
isA) increasing on
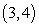 and decreasing on
and decreasing on 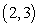 .
.B) increasing on
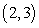 and decreasing on
and decreasing on 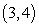 .
.C) increasing on
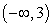 and decreasing on
and decreasing on 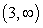 .
.D) increasing in all its domain.
E) decreasing in all its domain.

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
41
The following table describes the signs of the first and second derivatives of a function 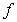 :
: 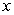 0
0
1
2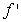 +
+
+
+
+
+
0
-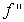 -
-
0
+
-
-
-
-
Which of the following is a possible graph of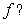
A)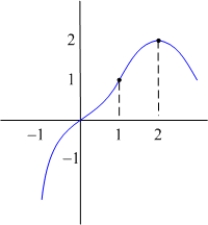
B)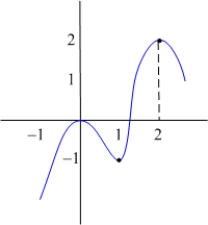
C)
D)
E) None of the above.
 :
:  0
01
2
 +
++
+
+
+
0
-
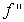 -
-0
+
-
-
-
-
Which of the following is a possible graph of
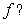
A)

B)

C)

D)

E) None of the above.

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
42
Sketch the graph of the following function. Indicate the asymptotes, local extrema, and points of inflection. 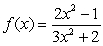 .
.
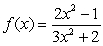 .
.
Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
43
Sketch the graph of the function 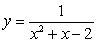 and indicate the transition points and asymptotes.
and indicate the transition points and asymptotes.
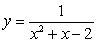 and indicate the transition points and asymptotes.
and indicate the transition points and asymptotes.
Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
44
The following is the graph of 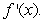 Where do the points of inflection of
Where do the points of inflection of 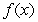 occur, and on which intervals is
occur, and on which intervals is 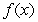 concave up?
concave up? 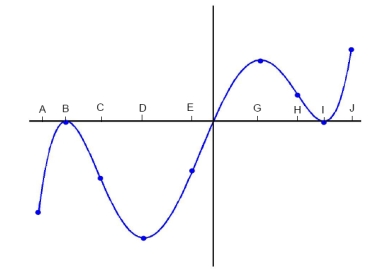
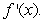 Where do the points of inflection of
Where do the points of inflection of 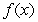 occur, and on which intervals is
occur, and on which intervals is 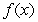 concave up?
concave up? 

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
45
Sketch the graph of the function. Indicate the asymptotes, local extrema, and points of inflection. 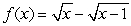
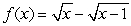

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
46
Sketch the graph of a function such that 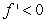 for
for 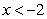
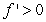 for
for 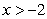
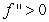 for
for 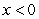
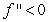 for
for 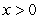
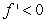 for
for 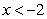
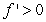 for
for 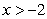
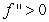 for
for 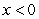
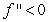 for
for 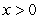

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
47
Find the sides of the isosceles triangle with maximum area, inscribed in a circle of radius 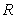 .
.
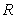 .
.
Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
48
Find the dimensions and the perimeter of the rectangle with maximum perimeter, inscribed in the ellipse 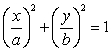 .
. 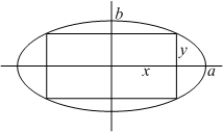
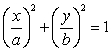 .
. 

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
49
Find the intervals on which the function is concave up and concave down and indicate the points of inflection
A)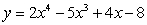
B)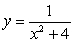
A)
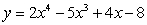
B)
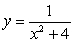

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
50
Match the functions with their graphs
(a)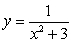 (b)
(b) 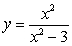 (c)
(c) 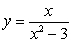 (d)
(d)  (A)
(A) 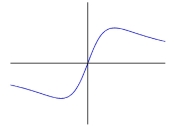 (B)
(B) 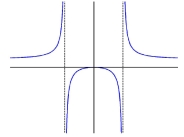 (C)
(C) 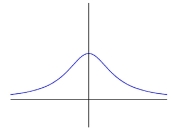 (D)
(D) 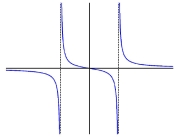
(a)
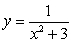 (b)
(b) 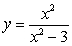 (c)
(c) 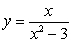 (d)
(d)  (A)
(A)  (B)
(B)  (C)
(C)  (D)
(D) 

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
51
A manufacturer sells instruments for 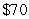 per unit.
per unit.
The cost of producing instruments is
instruments is 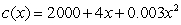 , and no more than
, and no more than 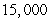 instruments can be produced in a week.
instruments can be produced in a week.
Find the amount of instruments that should be produced in a week to obtain maximum profit.
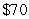 per unit.
per unit.The cost of producing
 instruments is
instruments is 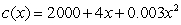 , and no more than
, and no more than 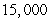 instruments can be produced in a week.
instruments can be produced in a week.Find the amount of instruments that should be produced in a week to obtain maximum profit.

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
52
The following table describes the signs of the first and second derivatives of a function 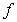 :
: 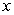 0
0
1
2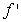
 0
0 

 0
0 
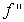
 0
0  0
0 
 If
If 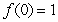 , sketch a possible graph of
, sketch a possible graph of 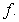
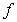 :
: 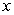 0
01
2
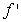
 0
0 

 0
0 
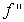
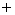 0
0  0
0 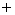
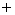
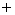 If
If 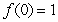 , sketch a possible graph of
, sketch a possible graph of 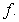

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
53
Find the angles of the isosceles triangle of minimum area in which a circle of radius 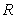 is inscribed.
is inscribed.
What are the sides of this triangle?
(You don't have to prove that the area is a minimum.)
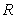 is inscribed.
is inscribed.What are the sides of this triangle?
(You don't have to prove that the area is a minimum.)


Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
54
For the function 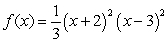 , find the transition points, intervals of increase/decrease, concavity, and asymptotic behavior. Then sketch the graph using this information.
, find the transition points, intervals of increase/decrease, concavity, and asymptotic behavior. Then sketch the graph using this information.
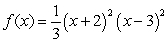 , find the transition points, intervals of increase/decrease, concavity, and asymptotic behavior. Then sketch the graph using this information.
, find the transition points, intervals of increase/decrease, concavity, and asymptotic behavior. Then sketch the graph using this information.
Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
55
The function 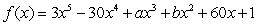 has inflection points at
has inflection points at  and
and 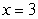 . Find
. Find 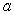 and
and 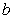 .
.
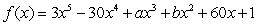 has inflection points at
has inflection points at  and
and 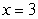 . Find
. Find 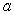 and
and 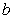 .
.
Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
56
The tangent line to 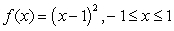 at a point
at a point 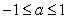 intersects the
intersects the  and
and 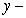 axes at points
axes at points  and
and 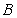 , respectively. Find
, respectively. Find  such that the area of the triangle
such that the area of the triangle 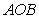 is maximum.
is maximum. 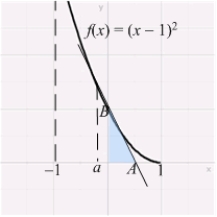
 at a point
at a point  intersects the
intersects the  and
and 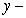 axes at points
axes at points  and
and 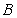 , respectively. Find
, respectively. Find  such that the area of the triangle
such that the area of the triangle 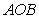 is maximum.
is maximum. 

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
57
What can you conclude about the graph of 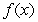 from the following table?
from the following table?
-1
-6 +
+
0
-
-
-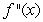 +
+
0
-
0
-
A)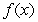 is concave down on
is concave down on 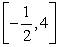 and
and 
B) is an inflection point and
is an inflection point and 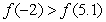
C)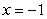 is an inflection point and
is an inflection point and 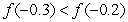
D)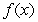 is concave up on
is concave up on 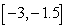 and
and 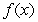 is increasing on
is increasing on 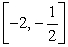
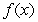 from the following table?
from the following table?-1
-6
 +
+0
-
-
-
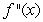 +
+0
-
0
-
A)
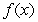 is concave down on
is concave down on 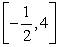 and
and 
B)
 is an inflection point and
is an inflection point and 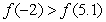
C)
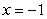 is an inflection point and
is an inflection point and 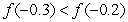
D)
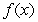 is concave up on
is concave up on 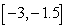 and
and 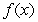 is increasing on
is increasing on 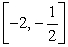

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
58
Sketch the graph of the function  .
.
Indicate the asymptotes, local extrema, and points of inflection if they exist.
 .
.Indicate the asymptotes, local extrema, and points of inflection if they exist.

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
59
Which of the following functions does not have horizontal asymptotes?
A)
B)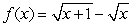
C)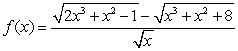
D)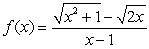
E) None of the above.
A)

B)
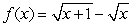
C)
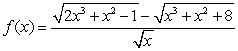
D)
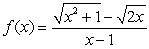
E) None of the above.

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
60
The following is the graph of  Where do the points of inflection of
Where do the points of inflection of  occur, and on which intervals is
occur, and on which intervals is 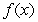 concave up?
concave up? 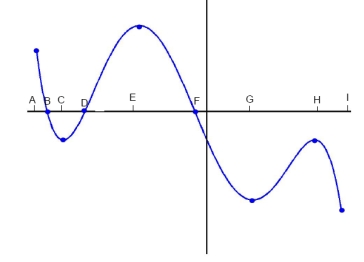
 Where do the points of inflection of
Where do the points of inflection of 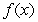 occur, and on which intervals is
occur, and on which intervals is 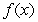 concave up?
concave up? 

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
61
Use Newton's Method with 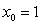 to find an approximate root for
to find an approximate root for 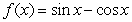 and use it to approximate
and use it to approximate 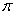 to within an error of at most
to within an error of at most 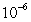 .
.
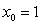 to find an approximate root for
to find an approximate root for 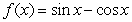 and use it to approximate
and use it to approximate 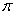 to within an error of at most
to within an error of at most 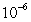 .
.
Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
62
A factory produces 2000 products each month. The expenses on each product are 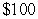 and the income from each product is
and the income from each product is 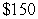 . For each additional product beyond the first 2000 products, the income for each product reduces by
. For each additional product beyond the first 2000 products, the income for each product reduces by 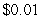 (for example, for 2001 products the income from each product is
(for example, for 2001 products the income from each product is 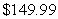 ).
).
A) Find the profit in a month for which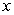 additional product are produced.
additional product are produced.
B) How many additional products should be produced to obtain maximum profit in a month?
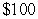 and the income from each product is
and the income from each product is 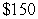 . For each additional product beyond the first 2000 products, the income for each product reduces by
. For each additional product beyond the first 2000 products, the income for each product reduces by 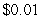 (for example, for 2001 products the income from each product is
(for example, for 2001 products the income from each product is 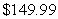 ).
). A) Find the profit in a month for which
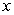 additional product are produced.
additional product are produced. B) How many additional products should be produced to obtain maximum profit in a month?

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
63
Use the given graph of 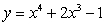 and Newton's Method to approximate the positive root to within an error of at most
and Newton's Method to approximate the positive root to within an error of at most 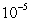 .
. 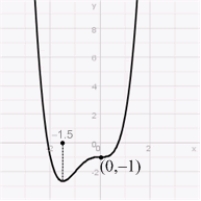
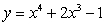 and Newton's Method to approximate the positive root to within an error of at most
and Newton's Method to approximate the positive root to within an error of at most 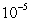 .
. 

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
64
Apply Newton's Method to 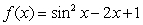 with an initial guess of
with an initial guess of 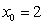 to calculate
to calculate 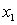 ,
, 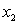 , and
, and 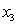 .
.
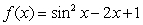 with an initial guess of
with an initial guess of 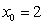 to calculate
to calculate 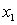 ,
, 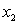 , and
, and 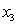 .
.
Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
65
Show that the equation 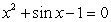 has a root
has a root 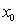 in the interval
in the interval 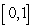 and use Newton's Method to approximate it to four decimal places.
and use Newton's Method to approximate it to four decimal places.
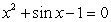 has a root
has a root 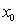 in the interval
in the interval 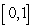 and use Newton's Method to approximate it to four decimal places.
and use Newton's Method to approximate it to four decimal places.
Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
66
Find the point on the graph of 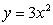 that is closest to the point
that is closest to the point  .
.
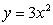 that is closest to the point
that is closest to the point  .
.
Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
67
Show that the equation 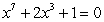 has a root in the interval
has a root in the interval 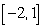 and use Newton's Method to approximate it with an error of at most
and use Newton's Method to approximate it with an error of at most 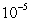 .
.
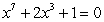 has a root in the interval
has a root in the interval 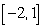 and use Newton's Method to approximate it with an error of at most
and use Newton's Method to approximate it with an error of at most 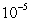 .
.
Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
68
A producer can sell 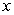 instruments per week for
instruments per week for 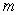 dollars each, where
dollars each, where 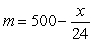 . His cost for producing
. His cost for producing 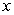 instruments is
instruments is  dollars.
dollars.
Find the amount of instruments that should be produced in a week to obtain maximum profit.
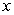 instruments per week for
instruments per week for 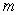 dollars each, where
dollars each, where 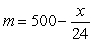 . His cost for producing
. His cost for producing 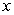 instruments is
instruments is  dollars.
dollars.Find the amount of instruments that should be produced in a week to obtain maximum profit.

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
69
Show that the equation 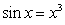 has a solution in the interval
has a solution in the interval 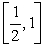 and use Newton's Method to approximate it within an error of at most
and use Newton's Method to approximate it within an error of at most 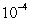 .
.
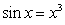 has a solution in the interval
has a solution in the interval 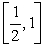 and use Newton's Method to approximate it within an error of at most
and use Newton's Method to approximate it within an error of at most 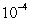 .
.
Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
70
Use Newton's Method to approximate the only positive root for 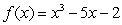 to within an error of at most
to within an error of at most 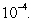
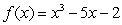 to within an error of at most
to within an error of at most 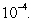

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
71
Show that the equation 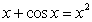 has a solution in the interval
has a solution in the interval 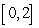 and use Newton's Method to approximate it to within an error of at most
and use Newton's Method to approximate it to within an error of at most 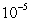 .
.
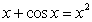 has a solution in the interval
has a solution in the interval 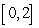 and use Newton's Method to approximate it to within an error of at most
and use Newton's Method to approximate it to within an error of at most 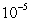 .
.
Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
72
Show that the equation 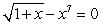 has a solution
has a solution  in the interval
in the interval  and approximate it by Newton's Method to seven decimal places.
and approximate it by Newton's Method to seven decimal places.
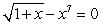 has a solution
has a solution  in the interval
in the interval  and approximate it by Newton's Method to seven decimal places.
and approximate it by Newton's Method to seven decimal places.
Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
73
A farmer wants to fence in a rectangular field for livestock. The boundary for one
side of the field is a long, straight river. No fencing is needed on this side. For the
remaining three sides, he has 400 meters of fencing available. What are the dimensions of the largest rectangular area that can be formed with this amount of fencing?
side of the field is a long, straight river. No fencing is needed on this side. For the
remaining three sides, he has 400 meters of fencing available. What are the dimensions of the largest rectangular area that can be formed with this amount of fencing?

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
74
A cylinder of radius  and height
and height 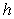 has surface area
has surface area 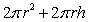 and volume
and volume 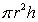 . Find the dimensions of a cylinder with volume
. Find the dimensions of a cylinder with volume 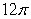 and minimal surface area.
and minimal surface area.
 and height
and height 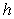 has surface area
has surface area 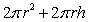 and volume
and volume 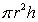 . Find the dimensions of a cylinder with volume
. Find the dimensions of a cylinder with volume 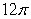 and minimal surface area.
and minimal surface area.
Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
75
Find the dimensions and the area of the rectangle with maximum area inscribed in the ellipse 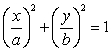 .
. 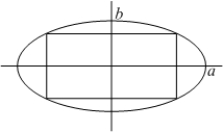
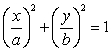 .
. 

Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
76
A right circular cylinder is to be constructed with a volume of 400 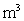 . The material used to build the top and base of the cylinder costs
. The material used to build the top and base of the cylinder costs  . The material for the remainder of the cylinder costs
. The material for the remainder of the cylinder costs  . Find the radius value which will minimize the material cost of constructing the cylinder.
. Find the radius value which will minimize the material cost of constructing the cylinder.
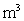 . The material used to build the top and base of the cylinder costs
. The material used to build the top and base of the cylinder costs  . The material for the remainder of the cylinder costs
. The material for the remainder of the cylinder costs  . Find the radius value which will minimize the material cost of constructing the cylinder.
. Find the radius value which will minimize the material cost of constructing the cylinder.
Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck
77
Apply Newton's Method to  with an initial guess of
with an initial guess of 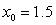 to calculate
to calculate 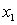 ,
, 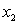 , and
, and 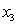 .
.
 with an initial guess of
with an initial guess of 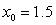 to calculate
to calculate 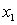 ,
, 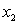 , and
, and 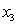 .
.
Unlock Deck
Unlock for access to all 77 flashcards in this deck.
Unlock Deck
k this deck


