Deck 7: Probability
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/273
Play
Full screen (f)
Deck 7: Probability
1
An experiment consists of tossing a coin, rolling a die, and observing the outcomes. Describe an appropriate sample space for this experiment.
A) {(H, H, H, H, H, H, T, T, T, T, T, T, 1, 2, 3, 4, 5, 6)}
B) {(1, 2, 3, 4, 5, 6), (H, T)}
C) {(H, 1), (T, 2), (H, 3), (T, 4), (H, 5), (T, 6), (T, 1), (T, 4), (T, 5), (H, 6)}
D) {(1, H, T), (2, H, T), (3, H, T), (4, H, T), (5, H, T), (6, H, T)}
E) {(H, 1), (H, 2), (H, 3), (H, 4), (H, 5), (H, 6), (T, 1), (T, 2), (T, 3), (T, 4), (T, 5), (T, 6)}
A) {(H, H, H, H, H, H, T, T, T, T, T, T, 1, 2, 3, 4, 5, 6)}
B) {(1, 2, 3, 4, 5, 6), (H, T)}
C) {(H, 1), (T, 2), (H, 3), (T, 4), (H, 5), (T, 6), (T, 1), (T, 4), (T, 5), (H, 6)}
D) {(1, H, T), (2, H, T), (3, H, T), (4, H, T), (5, H, T), (6, H, T)}
E) {(H, 1), (H, 2), (H, 3), (H, 4), (H, 5), (H, 6), (T, 1), (T, 2), (T, 3), (T, 4), (T, 5), (T, 6)}
{(H, 1), (H, 2), (H, 3), (H, 4), (H, 5), (H, 6), (T, 1), (T, 2), (T, 3), (T, 4), (T, 5), (T, 6)}
2
In a television game show, the winner is asked to select three prizes from five different prizes, A, B, C, D, and E. Describing a sample space of possible outcomes (order is not important) determine the number of points there are in the sample space corresponding to a selection that includes A.
A) 6
B) 9
C) 4
D) 2
A) 6
B) 9
C) 4
D) 2
6
3
A sample of two transistors taken from a local electronics store was examined to determine whether the transistors were defective (d) or nondefective (n). What is an appropriate sample space for this experiment?
A) {dd, dn, nd}
B) {dd, dn, nd, nn}
C) {dd, nn, nd}
D) {(d,d), (n,n)}
A) {dd, dn, nd}
B) {dd, dn, nd, nn}
C) {dd, nn, nd}
D) {(d,d), (n,n)}
{dd, dn, nd, nn}
4
Let 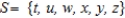 be a sample space of an experiment and let
be a sample space of an experiment and let 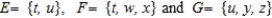 be events of this experiment.
be events of this experiment.
Find the events Fc and E ∩ Gc.
A)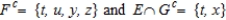
B)
C)
D)
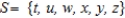 be a sample space of an experiment and let
be a sample space of an experiment and let 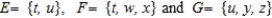 be events of this experiment.
be events of this experiment. Find the events Fc and E ∩ Gc.
A)
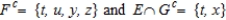
B)

C)

D)


Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
5
An experiment consists of selecting a card from a standard deck of playing cards and noting whether the card is black (B) or red (R). Describe an appropriate sample space for this experiment.
A) {BR, RB}
B) {R, R}
C) {R, B, B, R}
D) {B, R}
E) {B, B}
A) {BR, RB}
B) {R, R}
C) {R, B, B, R}
D) {B, R}
E) {B, B}

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
6
Let 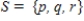 be a sample space of an experiment with outcomes p, q and r. List all the events of this experiment.
be a sample space of an experiment with outcomes p, q and r. List all the events of this experiment.
A) Ø, {p}, {q}, {r}, {p, q}, {p, r}, {q, r}, S
B) {p}, {q}, {r}
C) Ø, {p}, {q}, {r}, {p, q}, {p, r}, {q, r}
D) {p}, {q}, {r}, {p, q}, {p, r}, {q, r}
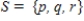 be a sample space of an experiment with outcomes p, q and r. List all the events of this experiment.
be a sample space of an experiment with outcomes p, q and r. List all the events of this experiment. A) Ø, {p}, {q}, {r}, {p, q}, {p, r}, {q, r}, S
B) {p}, {q}, {r}
C) Ø, {p}, {q}, {r}, {p, q}, {p, r}, {q, r}
D) {p}, {q}, {r}, {p, q}, {p, r}, {q, r}

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
7
An experiment consists of casting a pair of dice and observing the number that falls uppermost on each die. We may represent each outcome of the experiment by an ordered pair of numbers, the first representing the number that appears uppermost on the first die and the second representing the number that appears uppermost on the second die. Consider the sample space S = {(1, 1), (1, 2), (1, 3), (1, 4), (1, 5), (1, 6),
(2, 1), (2, 2), (2, 3), (2, 4), (2, 5), (2, 6),
(3, 1), (3, 2), (3, 3), (3, 4), (3, 5), (3, 6),
(4, 1), (4, 2), (4, 3), (4, 4), (4, 5), (4, 6),
(5, 1), (5, 2), (5, 3), (5, 4), (5, 5), (5, 6),
(6, 1), (6, 2), (6, 3), (6, 4), (6, 5), (6, 6)}
Determine the event that the number that falls uppermost on the second die is double that of the number that falls on the first die.
A) {(1, 2), (2, 4), (3, 6)}
B) {(3, 1), (2, 6), (4, 4)}
C) {(1, 1), (2, 1), (1, 6)}
D) {(1, 2), (5, 3), (6, 6)}
(2, 1), (2, 2), (2, 3), (2, 4), (2, 5), (2, 6),
(3, 1), (3, 2), (3, 3), (3, 4), (3, 5), (3, 6),
(4, 1), (4, 2), (4, 3), (4, 4), (4, 5), (4, 6),
(5, 1), (5, 2), (5, 3), (5, 4), (5, 5), (5, 6),
(6, 1), (6, 2), (6, 3), (6, 4), (6, 5), (6, 6)}
Determine the event that the number that falls uppermost on the second die is double that of the number that falls on the first die.
A) {(1, 2), (2, 4), (3, 6)}
B) {(3, 1), (2, 6), (4, 4)}
C) {(1, 1), (2, 1), (1, 6)}
D) {(1, 2), (5, 3), (6, 6)}

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
8
An experiment consists of casting a pair of dice and observing the number that falls uppermost on each die. We may represent each outcome of the experiment by an ordered pair of numbers, the first representing the number that appears uppermost on the first die and the second representing the number that appears uppermost on the second die. Consider the sample space S = {(1, 1), (1, 2), (1, 3), (1, 4), (1, 5), (1, 6),
(2, 1), (2, 2), (2, 3), (2, 4), (2, 5), (2, 6),
(3, 1), (3, 2), (3, 3), (3, 4), (3, 5), (3, 6),
(4, 1), (4, 2), (4, 3), (4, 4), (4, 5), (4, 6),
(5, 1), (5, 2), (5, 3), (5, 4), (5, 5), (5, 6),
(6, 1), (6, 2), (6, 3), (6, 4), (6, 5), (6, 6)}
Determine the event that the number that falls uppermost on the first die is greater than the number that falls uppermost on the second die.
A) {(2, 1), (3, 1), (4, 1), (5, 1), (6, 1), (3, 2), (4, 2), (5, 2), (6, 2), (4, 3), (5, 3), (6, 3), (5, 4), (6, 4), (6, 5)}
B) {(1, 2), (1, 3), (1, 4), (1, 5), (1, 6), (3, 2), (4, 2), (5, 2), (6, 2), (4, 3), (5, 3), (6, 3), (5, 4), (6, 4), (5, 6)}
C) {(2, 1), (3, 1), (4, 1), (5, 1), (6, 1), (2, 3), (2, 4), (2, 5), (2, 6), (3, 4), (3, 5), (3, 6), (4, 5), (6, 4), (6, 5)}
D) Ø
(2, 1), (2, 2), (2, 3), (2, 4), (2, 5), (2, 6),
(3, 1), (3, 2), (3, 3), (3, 4), (3, 5), (3, 6),
(4, 1), (4, 2), (4, 3), (4, 4), (4, 5), (4, 6),
(5, 1), (5, 2), (5, 3), (5, 4), (5, 5), (5, 6),
(6, 1), (6, 2), (6, 3), (6, 4), (6, 5), (6, 6)}
Determine the event that the number that falls uppermost on the first die is greater than the number that falls uppermost on the second die.
A) {(2, 1), (3, 1), (4, 1), (5, 1), (6, 1), (3, 2), (4, 2), (5, 2), (6, 2), (4, 3), (5, 3), (6, 3), (5, 4), (6, 4), (6, 5)}
B) {(1, 2), (1, 3), (1, 4), (1, 5), (1, 6), (3, 2), (4, 2), (5, 2), (6, 2), (4, 3), (5, 3), (6, 3), (5, 4), (6, 4), (5, 6)}
C) {(2, 1), (3, 1), (4, 1), (5, 1), (6, 1), (2, 3), (2, 4), (2, 5), (2, 6), (3, 4), (3, 5), (3, 6), (4, 5), (6, 4), (6, 5)}
D) Ø

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
9
Let S = {1, 2, 3, 4, 5, 6}, E = {1, 3, 5}, F = {2, 4, 6} and G = {2, 3}. Are the events F and G mutually exclusive?
A) yes
B) no
A) yes
B) no

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
10
Let 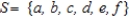 be a sample space of an experiment and let
be a sample space of an experiment and let  , and
, and 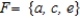 be events of this experiment.
be events of this experiment.
Find the events E ∪ F and E ∩ F.
A)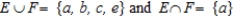
B)
C)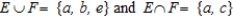
D)
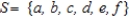 be a sample space of an experiment and let
be a sample space of an experiment and let  , and
, and 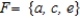 be events of this experiment.
be events of this experiment. Find the events E ∪ F and E ∩ F.
A)
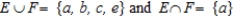
B)

C)
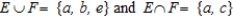
D)


Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
11
An experiment consists of casting a pair of dice and observing the number that falls uppermost on each die. We may represent each outcome of the experiment by an ordered pair of numbers, the first representing the number that appears uppermost on the first die and the second representing the number that appears uppermost on the second die. Consider the sample space S = {(1, 1), (1, 2), (1, 3), (1, 4), (1, 5), (1, 6),
(2, 1), (2, 2), (2, 3), (2, 4), (2, 5), (2, 6),
(3, 1), (3, 2), (3, 3), (3, 4), (3, 5), (3, 6),
(4, 1), (4, 2), (4, 3), (4, 4), (4, 5), (4, 6),
(5, 1), (5, 2), (5, 3), (5, 4), (5, 5), (5, 6),
(6, 1), (6, 2), (6, 3), (6, 4), (6, 5), (6, 6)}
Determine the event that the number falling uppermost on one die is a 4 and the number falling uppermost on the other die is less than 4.
A) {(1, 4), (2, 4), (3, 4), (4, 3), (4, 2), (4, 1)}
B) {(4, 5), (4, 6), (5, 2), (6, 4)}
C) {(2, 3), (2, 4), (6, 5), (2, 6), (3, 2), (4, 2), (5, 1), (6, 2)}
D) Ø
(2, 1), (2, 2), (2, 3), (2, 4), (2, 5), (2, 6),
(3, 1), (3, 2), (3, 3), (3, 4), (3, 5), (3, 6),
(4, 1), (4, 2), (4, 3), (4, 4), (4, 5), (4, 6),
(5, 1), (5, 2), (5, 3), (5, 4), (5, 5), (5, 6),
(6, 1), (6, 2), (6, 3), (6, 4), (6, 5), (6, 6)}
Determine the event that the number falling uppermost on one die is a 4 and the number falling uppermost on the other die is less than 4.
A) {(1, 4), (2, 4), (3, 4), (4, 3), (4, 2), (4, 1)}
B) {(4, 5), (4, 6), (5, 2), (6, 4)}
C) {(2, 3), (2, 4), (6, 5), (2, 6), (3, 2), (4, 2), (5, 1), (6, 2)}
D) Ø

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
12
An experiment consists of tossing a coin, rolling a die, and observing the outcomes. Describe the event "A head is tossed and an even number is rolled."
A)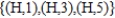
B)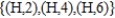
C)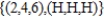
D)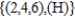
A)
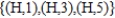
B)
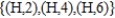
C)
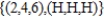
D)
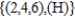

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
13
An experiment consists of casting a pair of dice and observing the number that falls uppermost on each die. We may represent each outcome of the experiment by an ordered pair of numbers, the first representing the number that appears uppermost on the first die and the second representing the number that appears uppermost on the second die. Consider the sample space S = {(1, 1), (1, 2), (1, 3), (1, 4), (1, 5), (1, 6),
(2, 1), (2, 2), (2, 3), (2, 4), (2, 5), (2, 6),
(3, 1), (3, 2), (3, 3), (3, 4), (3, 5), (3, 6),
(4, 1), (4, 2), (4, 3), (4, 4), (4, 5), (4, 6),
(5, 1), (5, 2), (5, 3), (5, 4), (5, 5), (5, 6),
(6, 1), (6, 2), (6, 3), (6, 4), (6, 5), (6, 6)}
Determine the event that the sum of the numbers falling uppermost is greater than or equal to 7.
A) {(1, 1), (2, 2), (3, 3), (4, 4), (5, 5), (6, 6)}
B) {(1, 2), (1, 3), (1, 4), (1, 5), (1, 6), (2, 3), (2, 4), (2, 5), (2, 6), (3, 4), (3, 5), (3, 6), (4, 5), (4, 6), (5, 6)}
C) {(1, 6), (2, 5), (2, 6), (3, 4), (3, 5), (3, 6), (4, 3), (4, 5), (4, 6), (5, 2), (5, 3), (5, 4), (5, 5), (5, 6), (6, 1), (6, 2), (6, 3), (6, 4), (6, 5), (6, 6)}
D) Ø
(2, 1), (2, 2), (2, 3), (2, 4), (2, 5), (2, 6),
(3, 1), (3, 2), (3, 3), (3, 4), (3, 5), (3, 6),
(4, 1), (4, 2), (4, 3), (4, 4), (4, 5), (4, 6),
(5, 1), (5, 2), (5, 3), (5, 4), (5, 5), (5, 6),
(6, 1), (6, 2), (6, 3), (6, 4), (6, 5), (6, 6)}
Determine the event that the sum of the numbers falling uppermost is greater than or equal to 7.
A) {(1, 1), (2, 2), (3, 3), (4, 4), (5, 5), (6, 6)}
B) {(1, 2), (1, 3), (1, 4), (1, 5), (1, 6), (2, 3), (2, 4), (2, 5), (2, 6), (3, 4), (3, 5), (3, 6), (4, 5), (4, 6), (5, 6)}
C) {(1, 6), (2, 5), (2, 6), (3, 4), (3, 5), (3, 6), (4, 3), (4, 5), (4, 6), (5, 2), (5, 3), (5, 4), (5, 5), (5, 6), (6, 1), (6, 2), (6, 3), (6, 4), (6, 5), (6, 6)}
D) Ø

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
14
An experiment consists of selecting a card from a standard deck of playing cards and noting whether the card is black (B) or red (R). What are the events of this experiment?
A) {B, R}, {R, B}
B) {Ø}, {B}, {R}
C) {B}, {R}, {B, B}, {R, R}
D) {Ø}, {B}, {R}, {B, R}
E) {Ø}, {B, R}, {R, B}, {R, R}, {B, B}
A) {B, R}, {R, B}
B) {Ø}, {B}, {R}
C) {B}, {R}, {B, B}, {R, R}
D) {Ø}, {B}, {R}, {B, R}
E) {Ø}, {B, R}, {R, B}, {R, R}, {B, B}

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
15
Let S = {1, 2, 3, 4, 5, 6}, E = {1, 3, 5}, F = {2, 4, 6} and G = {3, 4}. Are the events F and G complementary?
A) no
B) yes
A) no
B) yes

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
16
A die is cast and the number that falls uppermost is observed. Let E denote the event that the number shown is a 6 and let F denote the event that the number shown is an even number.
Are the events E and F mutually exclusive?
A) no
B) yes
Are the events E and F mutually exclusive?
A) no
B) yes

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
17
Let S = {1, 2, 3, 4, 5, 6}, E = {1, 3, 5}, F = {2, 4, 6} and G = {2, 3} . Find the event E ∪ F ∪ G.
A) E ∪ F ∪ G = {2, 3, 4, 5, 7}
B) E ∪ F ∪ G = {1, 3, 4, 5, 6}
C) E ∪ F ∪ G = {1, 2, 3, 4, 5, 6}
D) E ∪ F ∪ G = {1, 2, 3, 5, 6}
A) E ∪ F ∪ G = {2, 3, 4, 5, 7}
B) E ∪ F ∪ G = {1, 3, 4, 5, 6}
C) E ∪ F ∪ G = {1, 2, 3, 4, 5, 6}
D) E ∪ F ∪ G = {1, 2, 3, 5, 6}

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
18
An experiment consists of selecting a letter at random from the letters in the word PENNSYLVANIA and observing the outcomes. What is an appropriate sample space for this experiment?
A)
B)
C)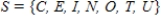
D)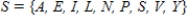
A)

B)

C)
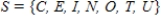
D)
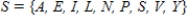

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
19
Let 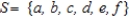 be a sample space of an experiment and let
be a sample space of an experiment and let 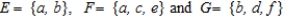 be events of this experiment.
be events of this experiment.
Are the events E and F mutually exclusive?
A) yes
B) no
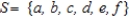 be a sample space of an experiment and let
be a sample space of an experiment and let 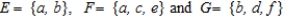 be events of this experiment.
be events of this experiment. Are the events E and F mutually exclusive?
A) yes
B) no

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
20
Let S = {1, 2, 3, 4, 5, 6}, E = {2, 4, 6, }, F = {1, 3, 5} and G = {4, 5} .
Find the event (E ∩ F ∩ G)c.
A) (E ∩ F ∩ G)c = {1, 2, 3, 4, 5, 6}
B) (E ∩ F ∩ G)c = {1, 2, 3, 4, 5}
C) (E ∩ F ∩ G)c = {2, 3, 4, 5, 6}
D) (E ∩ F ∩ G)c = {1, 2, 5, 6, 9}
Find the event (E ∩ F ∩ G)c.
A) (E ∩ F ∩ G)c = {1, 2, 3, 4, 5, 6}
B) (E ∩ F ∩ G)c = {1, 2, 3, 4, 5}
C) (E ∩ F ∩ G)c = {2, 3, 4, 5, 6}
D) (E ∩ F ∩ G)c = {1, 2, 5, 6, 9}

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
21
Let S be a sample space for an experiment and let G and A be events of this experiment. Show that the events  and
and 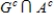 are mutually exclusive.
are mutually exclusive.
Hint: Use De Morgan's law.
A) By De Morgan's law,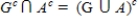 , so the events are mutually exclusive.
, so the events are mutually exclusive.
B) By De Morgan's law,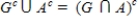 , so the events are mutually exclusive.
, so the events are mutually exclusive.
C) By De Morgan's law,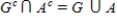 , so the events are mutually exclusive.
, so the events are mutually exclusive.
D) By De Morgan's law,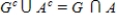 , so the events are mutually exclusive.
, so the events are mutually exclusive.
E) By De Morgan's law,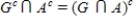 , so the events are not mutually exclusive.
, so the events are not mutually exclusive.
 and
and 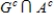 are mutually exclusive.
are mutually exclusive. Hint: Use De Morgan's law.
A) By De Morgan's law,
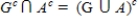 , so the events are mutually exclusive.
, so the events are mutually exclusive.B) By De Morgan's law,
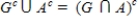 , so the events are mutually exclusive.
, so the events are mutually exclusive.C) By De Morgan's law,
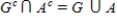 , so the events are mutually exclusive.
, so the events are mutually exclusive.D) By De Morgan's law,
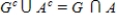 , so the events are mutually exclusive.
, so the events are mutually exclusive.E) By De Morgan's law,
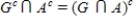 , so the events are not mutually exclusive.
, so the events are not mutually exclusive.
Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
22
Let S be any sample space and N, F, and E be any three events associated with the experiment. Describe the following event, using the symbols ∪, ∩, and c.
The event that E does not occur.
A) Ec
B)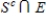
C)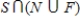
D)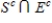
E)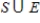
The event that E does not occur.
A) Ec
B)
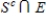
C)
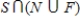
D)
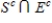
E)
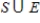

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
23
A certain airport hotel operates a shuttle bus service between the hotel and the airport. The maximum capacity of a bus is 20 passengers. On alternate trips of the shuttle bus over a period of 1 wk, the hotel manager kept a record of the number of passengers arriving at the hotel in each bus. Describe the event E that a shuttle bus carried fewer than twelve passengers.
A) {0, 1, 2, 3, 4, 5, 6, 7, 8}
B) {0, 1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12, 13, 14, 15}
C) {0, 1, 2, 3, 4, 5, 6, 7}
D) {0, 1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11}
A) {0, 1, 2, 3, 4, 5, 6, 7, 8}
B) {0, 1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12, 13, 14, 15}
C) {0, 1, 2, 3, 4, 5, 6, 7}
D) {0, 1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11}

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
24
An experiment consists of casting a pair of dice and observing the number that falls uppermost on each die. We may represent each outcome of the experiment by an ordered pair of numbers, the first representing the number that appears uppermost on the first die and the second representing the number that appears uppermost on the second die. Consider the sample space
S = {(1, 1), (1, 2), (1, 3), (1, 4), (1, 5), (1, 6),
(2, 1), (2, 2), (2, 3), (2, 4), (2, 5), (2, 6),
(3, 1), (3, 2), (3, 3), (3, 4), (3, 5), (3, 6),
(4, 1), (4, 2), (4, 3), (4, 4), (4, 5), (4, 6),
(5, 1), (5, 2), (5, 3), (5, 4), (5, 5), (5, 6),
(6, 1), (6, 2), (6, 3), (6, 4), (6, 5), (6, 6)}
Determine the event that the sum of the numbers falling uppermost is less than or equal to 6.
Express your answer using set notation.
S = {(1, 1), (1, 2), (1, 3), (1, 4), (1, 5), (1, 6),
(2, 1), (2, 2), (2, 3), (2, 4), (2, 5), (2, 6),
(3, 1), (3, 2), (3, 3), (3, 4), (3, 5), (3, 6),
(4, 1), (4, 2), (4, 3), (4, 4), (4, 5), (4, 6),
(5, 1), (5, 2), (5, 3), (5, 4), (5, 5), (5, 6),
(6, 1), (6, 2), (6, 3), (6, 4), (6, 5), (6, 6)}
Determine the event that the sum of the numbers falling uppermost is less than or equal to 6.
Express your answer using set notation.

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
25
As part of a quality-control procedure, an inspector at Bristol Farms randomly selects ten eggs from each consignment of eggs he receives and records the number of broken eggs. Describe the event F that at least nine eggs are broken.
A) {9, 10}
B) {7, 8, 9, 10}
C) {6, 7, 8, 9, 10}
D) {0, 1, 2, 3, 4, 5}
A) {9, 10}
B) {7, 8, 9, 10}
C) {6, 7, 8, 9, 10}
D) {0, 1, 2, 3, 4, 5}

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
26
Eight players, A, B, C, D, E, F, G, and H, are competing in a series of elimination matches of a tennis tournament in which the winner of each preliminary match will advance to the semifinals and the winner of the semifinals will advance to the finals. An outline of the scheduled matches follows. Describe a sample space listing the possible participants in the finals. 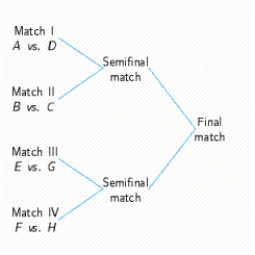
A) S = {(A,D), (A,F), (A,H), (C,B), (C,F), (C,H), (E,B), (E,D), (E, H), (G,B),
(G,D), (G,F)}
B) S = {(A,B), (A,D), (A,F), (A,H), (C,B), (C,D), (C,F), (C,H), (E,B), (E,D),
(E,F), (E, H), (G,B), (G,D), (G,F), (G,H)}
C) S = {(A,E), (A,F), (A,G), (A,H), (B,E), (B,F), (B,G), (B,H), (C, E), (C,F),
(C,G), (C,H), (D,E), (D,F), (D,G), (D,H)}
D) S = {(A,B), (A,C), (A,D), (B,С), (B,D), (C,D), (E,F), (E,G), (E, H), (F,G),
(F,H), (G,H)}
E) None of the above.

A) S = {(A,D), (A,F), (A,H), (C,B), (C,F), (C,H), (E,B), (E,D), (E, H), (G,B),
(G,D), (G,F)}
B) S = {(A,B), (A,D), (A,F), (A,H), (C,B), (C,D), (C,F), (C,H), (E,B), (E,D),
(E,F), (E, H), (G,B), (G,D), (G,F), (G,H)}
C) S = {(A,E), (A,F), (A,G), (A,H), (B,E), (B,F), (B,G), (B,H), (C, E), (C,F),
(C,G), (C,H), (D,E), (D,F), (D,G), (D,H)}
D) S = {(A,B), (A,C), (A,D), (B,С), (B,D), (C,D), (E,F), (E,G), (E, H), (F,G),
(F,H), (G,H)}
E) None of the above.

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
27
The customer service department of Universal Instruments, manufacturer of the Galaxy home computer, conducted a survey among customers who had returned their purchase registration cards. Purchasers of its deluxe model home computer were asked to report the length of time (t) in days before service was required. Describe a sample space corresponding to this survey.
A) {t | t > 0}
B) {t | 0 < t ≤ 370}
C) {t | 100 ≤ t ≤ 370}
D) {t | 0 < t ≤ 100}
A) {t | t > 0}
B) {t | 0 < t ≤ 370}
C) {t | 100 ≤ t ≤ 370}
D) {t | 0 < t ≤ 100}

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
28
An experiment consists of casting a pair of dice and observing the number that falls uppermost on each die. We may represent each outcome of the experiment by an ordered pair of numbers, the first representing the number that appears uppermost on the first die and the second representing the number that appears uppermost on the second die. Consider the sample space
S = {(1, 1), (1, 2), (1, 3), (1, 4), (1, 5), (1, 6),
(2, 1), (2, 2), (2, 3), (2, 4), (2, 5), (2, 6),
(3, 1), (3, 2), (3, 3), (3, 4), (3, 5), (3, 6),
(4, 1), (4, 2), (4, 3), (4, 4), (4, 5), (4, 6),
(5, 1), (5, 2), (5, 3), (5, 4), (5, 5), (5, 6),
(6, 1), (6, 2), (6, 3), (6, 4), (6, 5), (6, 6)}
Determine the event that the number that falls uppermost on the first die is less than the number that falls uppermost on the second die.
Express your answer using set notation.
S = {(1, 1), (1, 2), (1, 3), (1, 4), (1, 5), (1, 6),
(2, 1), (2, 2), (2, 3), (2, 4), (2, 5), (2, 6),
(3, 1), (3, 2), (3, 3), (3, 4), (3, 5), (3, 6),
(4, 1), (4, 2), (4, 3), (4, 4), (4, 5), (4, 6),
(5, 1), (5, 2), (5, 3), (5, 4), (5, 5), (5, 6),
(6, 1), (6, 2), (6, 3), (6, 4), (6, 5), (6, 6)}
Determine the event that the number that falls uppermost on the first die is less than the number that falls uppermost on the second die.
Express your answer using set notation.

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
29
An experiment consists of casting a pair of dice and observing the number that falls uppermost on each die. We may represent each outcome of the experiment by an ordered pair of numbers, the first representing the number that appears uppermost on the first die and the second representing the number that appears uppermost on the second die. Consider the sample space
S = {(1, 1), (1, 2), (1, 3), (1, 4), (1, 5), (1, 6),
(2, 1), (2, 2), (2, 3), (2, 4), (2, 5), (2, 6),
(3, 1), (3, 2), (3, 3), (3, 4), (3, 5), (3, 6),
(4, 1), (4, 2), (4, 3), (4, 4), (4, 5), (4, 6),
(5, 1), (5, 2), (5, 3), (5, 4), (5, 5), (5, 6),
(6, 1), (6, 2), (6, 3), (6, 4), (6, 5), (6, 6)}
Determine the event that the number that falls uppermost on the second die is double that of the number that falls on the first die.
Express your answer using set notation.
S = {(1, 1), (1, 2), (1, 3), (1, 4), (1, 5), (1, 6),
(2, 1), (2, 2), (2, 3), (2, 4), (2, 5), (2, 6),
(3, 1), (3, 2), (3, 3), (3, 4), (3, 5), (3, 6),
(4, 1), (4, 2), (4, 3), (4, 4), (4, 5), (4, 6),
(5, 1), (5, 2), (5, 3), (5, 4), (5, 5), (5, 6),
(6, 1), (6, 2), (6, 3), (6, 4), (6, 5), (6, 6)}
Determine the event that the number that falls uppermost on the second die is double that of the number that falls on the first die.
Express your answer using set notation.

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
30
Let 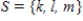 be a sample space of an experiment with outcomes k, l and m. List all the events of this experiment.
be a sample space of an experiment with outcomes k, l and m. List all the events of this experiment.
Express your answer using set notation.
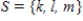 be a sample space of an experiment with outcomes k, l and m. List all the events of this experiment.
be a sample space of an experiment with outcomes k, l and m. List all the events of this experiment.
Express your answer using set notation.

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
31
Let 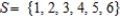 ,
, 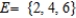 ,
, 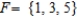 and
and 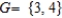 .
.
Find the event E ∪ F ∪ G.
Express your answer using set notation.
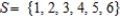 ,
, 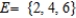 ,
, 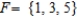 and
and 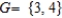 .
.Find the event E ∪ F ∪ G.
Express your answer using set notation.

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
32
Robin purchased shares of a machine tool company and shares of an airline company. Let E be the event that the shares of the machine tool company increase in value over the next 5 months, and let F be the event that the shares of the airline company increase in value over the next 5 months. Using the symbols ∪, ∩ and c describe the event that the shares of only one of the two companies increase in value.
A) (E ∩ Fc) ∪ (Ec ∩ F)
B) E ∪ F
C) Ec ∩ Fc
D) Ø
A) (E ∩ Fc) ∪ (Ec ∩ F)
B) E ∪ F
C) Ec ∩ Fc
D) Ø

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
33
Human blood is classified by the presence or absence of three main antigens (A, B, and Rh). When a blood specimen is typed, the presence of the A and/or B antigen is indicated by listing the letter A and/or the letter B. If neither the A nor B antigen is present, the letter O is used. The presence or absence of the Rh antigen is indicated by the symbols + or -, respectively. Thus, if a blood specimen is classified as AB+, it contains the A and the B antigens as well as the Rh antigen. Similarly, O- blood contains none of the three antigens. Using this information, determine the sample space corresponding to the different blood groups.
A) {AB+, AB-, AO+, BO+, AO-, BO-, O+, O-}
B) {AB+, AB-, O+, O-}
C) {A+, B+, A-, B-, O+, O-}
D) {AB+, AB-, A+, B+, A-, B-, O+, O-, ABO-, AO+, AO-, BO+, BO-}
E) {AB+, AB-, A+, B+, A-, B-, O+, O-}
A) {AB+, AB-, AO+, BO+, AO-, BO-, O+, O-}
B) {AB+, AB-, O+, O-}
C) {A+, B+, A-, B-, O+, O-}
D) {AB+, AB-, A+, B+, A-, B-, O+, O-, ABO-, AO+, AO-, BO+, BO-}
E) {AB+, AB-, A+, B+, A-, B-, O+, O-}

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
34
Let S be any sample space and K, E, and M be any three events associated with the experiment. Describe the following event, using the symbols ∪, ∩, and c.
The event that M does not occur.
The event that M does not occur.

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
35
An experiment consists of casting a pair of dice and observing the number that falls uppermost on each die. We may represent each outcome of the experiment by an ordered pair of numbers, the first representing the number that appears uppermost on the first die and the second representing the number that appears uppermost on the second die. Consider the sample space
S = {(1, 1), (1, 2), (1, 3), (1, 4), (1, 5), (1, 6),
(2, 1), (2, 2), (2, 3), (2, 4), (2, 5), (2, 6),
(3, 1), (3, 2), (3, 3), (3, 4), (3, 5), (3, 6),
(4, 1), (4, 2), (4, 3), (4, 4), (4, 5), (4, 6),
(5, 1), (5, 2), (5, 3), (5, 4), (5, 5), (5, 6),
(6, 1), (6, 2), (6, 3), (6, 4), (6, 5), (6, 6)}
Determine the event that the number falling uppermost on one die is a 2 and the number falling uppermost on the other die is greater than 2.
Express your answer using set notation.
S = {(1, 1), (1, 2), (1, 3), (1, 4), (1, 5), (1, 6),
(2, 1), (2, 2), (2, 3), (2, 4), (2, 5), (2, 6),
(3, 1), (3, 2), (3, 3), (3, 4), (3, 5), (3, 6),
(4, 1), (4, 2), (4, 3), (4, 4), (4, 5), (4, 6),
(5, 1), (5, 2), (5, 3), (5, 4), (5, 5), (5, 6),
(6, 1), (6, 2), (6, 3), (6, 4), (6, 5), (6, 6)}
Determine the event that the number falling uppermost on one die is a 2 and the number falling uppermost on the other die is greater than 2.
Express your answer using set notation.

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
36
Let 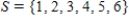 ,
, 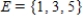 ,
, 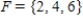 and
and 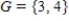 .
.
Find the event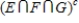 .
.
Express your answer using set notation.
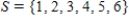 ,
, 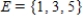 ,
, 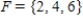 and
and 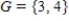 .
.
Find the event
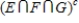 .
.
Express your answer using set notation.

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
37
An experiment consists of selecting a letter at random from the letters in the word LOUISIANA and observing the outcomes. What is an appropriate sample space for this experiment?
Express your answer using set notation.
Express your answer using set notation.

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
38
An opinion poll is conducted among a state's electorate to determine the relationship between their income levels and their stands on a proposition aimed at reducing state income taxes. Voters are classified as belonging to either the low-, middle-, or upper-income group. They are asked whether they favor, oppose, or are undecided about the proposition. Let the letters L, M, and U represent the low-, middle-, and upper-income groups, respectively, and let the letters f, o, and u represent the responses - favor, oppose, and undecided, respectively.
Describe the event E that a respondent does not oppose the proposition and does not belong to the upper-income group.
A) E = {(L,o), (L,u), (L,f), (M,o), (M,u), (M,f), (U,o), (U,u)}
B) E = {(L,f), (L,u), (M,f), (M,u)}
C) E = {(L,f), (M,f), (U,o), (U,u)}
D) E = {(L,o), (L,u), (L,f), (M,o), (M,u), (M,f)}
E) E = {(L,o), (L,u), (M,o), (M,u), (U,f)}
Describe the event E that a respondent does not oppose the proposition and does not belong to the upper-income group.
A) E = {(L,o), (L,u), (L,f), (M,o), (M,u), (M,f), (U,o), (U,u)}
B) E = {(L,f), (L,u), (M,f), (M,u)}
C) E = {(L,f), (M,f), (U,o), (U,u)}
D) E = {(L,o), (L,u), (L,f), (M,o), (M,u), (M,f)}
E) E = {(L,o), (L,u), (M,o), (M,u), (U,f)}

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
39
Let 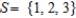 be a sample space associated with an experiment. How many subsets of S contain either the number 2 or the number 1?
be a sample space associated with an experiment. How many subsets of S contain either the number 2 or the number 1?
A) 5
B) 7
C) 4
D) 6
E) 3
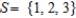 be a sample space associated with an experiment. How many subsets of S contain either the number 2 or the number 1?
be a sample space associated with an experiment. How many subsets of S contain either the number 2 or the number 1?A) 5
B) 7
C) 4
D) 6
E) 3

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
40
A time study was conducted by the production manager of Vista Vision to determine the length of time (t) in minutes required by an assembly worker to complete a certain task during the assembly of its Pulsar color television sets. Describe a sample space corresponding to this time study.
A) {t | 0 < t < 8}
B) {t | 8 ≤ t ≤ 10}
C) {t | 0< t ≤ 10}
D) {t | t > 0}
A) {t | 0 < t < 8}
B) {t | 8 ≤ t ≤ 10}
C) {t | 0< t ≤ 10}
D) {t | t > 0}

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
41
Eight players, A, B, C, D, E, F, G, and H, are competing in a series of elimination matches of a tennis tournament in which the winner of each preliminary match will advance to the semifinals and the winner of the semifinals will advance to the finals. An outline of the scheduled matches follows. Describe a sample space listing the possible participants in the finals.
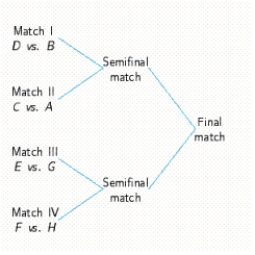


Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
42
The grade distribution for a certain class is shown in the table. Find the probability distribution associated with these data. 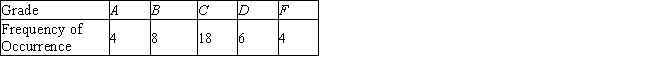
A) 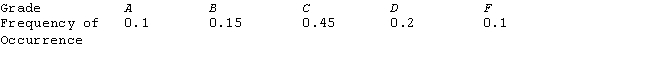
B)
C)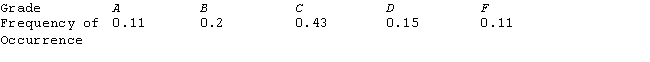
D) 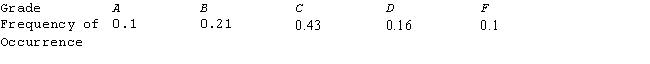

A)
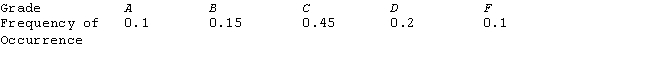
B)

C)
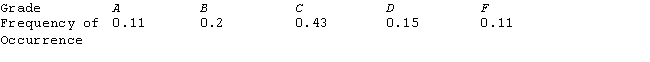
D)
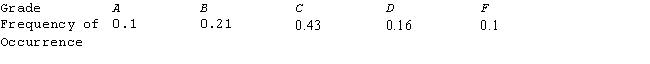

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
43
Let 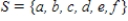 be a sample space of an experiment and let
be a sample space of an experiment and let 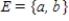 ,
,  and
and 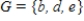 be events of this experiment.
be events of this experiment.
Are the events E and F mutually exclusive? Answer yes or no.
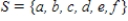 be a sample space of an experiment and let
be a sample space of an experiment and let 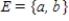 ,
,  and
and 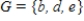 be events of this experiment.
be events of this experiment.
Are the events E and F mutually exclusive? Answer yes or no.

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
44
If a ball is selected at random from an urn containing two red balls, three white balls, and five blue balls, what is the probability that it will be a white ball?
A) The probability is 0.31
B) The probability is 0.33
C) The probability is 0.30
D) The probability is 0.32
A) The probability is 0.31
B) The probability is 0.33
C) The probability is 0.30
D) The probability is 0.32

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
45
In a survey of 3000 adults 18 year old and older conducted in 2000, the following question was asked : Is your family income keeping pace with the cost of living ? The results of the survey follow :  Determine the empirical probability distribution associated with these data.
Determine the empirical probability distribution associated with these data.
A) 
B) 
C) 
D) 
 Determine the empirical probability distribution associated with these data.
Determine the empirical probability distribution associated with these data.A)

B)

C)

D)


Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
46
The number of subscribers to five leading e-mail services is shown in the accompanying table: 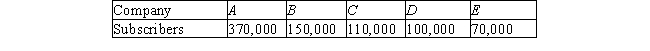 Find the empirical probability distribution associated with these data.
Find the empirical probability distribution associated with these data.
A) 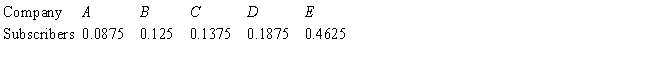
B) 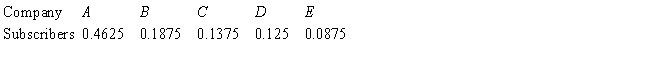
C) 
D) 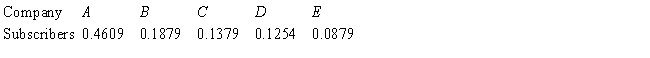
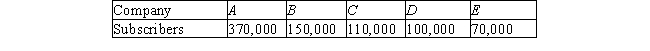 Find the empirical probability distribution associated with these data.
Find the empirical probability distribution associated with these data.A)
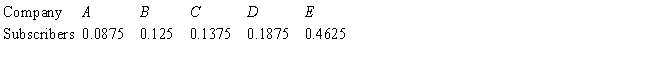
B)
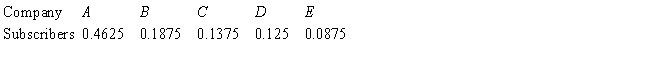
C)

D)
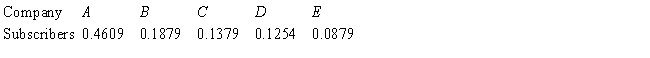

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
47
The following breakdown of a total of 18,613 transportation fatalities that occured in 2007 was obtained from records compiled by the U.S. Department of Transportation (DOT).  What is the probability that a victim randomly selected from this list of transportation fatalities for 2007 died in a car crash or bicycle accident? Round answer to two decimal places.
What is the probability that a victim randomly selected from this list of transportation fatalities for 2007 died in a car crash or bicycle accident? Round answer to two decimal places.
A) 0.98
B) 0.92
C) 0.89
D) 0.93
 What is the probability that a victim randomly selected from this list of transportation fatalities for 2007 died in a car crash or bicycle accident? Round answer to two decimal places.
What is the probability that a victim randomly selected from this list of transportation fatalities for 2007 died in a car crash or bicycle accident? Round answer to two decimal places.A) 0.98
B) 0.92
C) 0.89
D) 0.93

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
48
One light bulb is selected at random from a lot of 140 light bulbs, of which 2% are defective. What is the probability that the light bulb selected is defective?
A) The probability is 0.04
B) The probability is 0.01
C) The probability is 0.02
D) The probability is 0.03
A) The probability is 0.04
B) The probability is 0.01
C) The probability is 0.02
D) The probability is 0.03

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
49
In a poll conducted among 2,000 college freshmen to ascertain the political views of college students, the accompanying data were obtained:  A: Far Left B: Liberal
A: Far Left B: Liberal
C: Middle of the road
D: Conservative
E: Far right
Determine the empirical probability distribution associated with these data.
A) 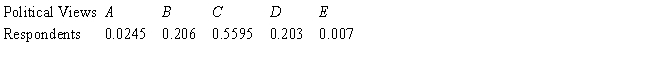
B) 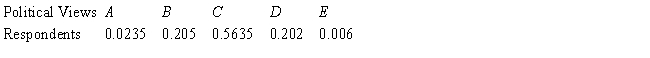
C) 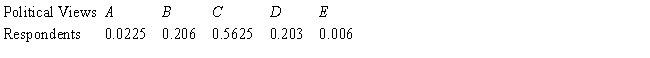
D) 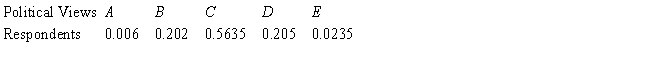
 A: Far Left B: Liberal
A: Far Left B: LiberalC: Middle of the road
D: Conservative
E: Far right
Determine the empirical probability distribution associated with these data.
A)
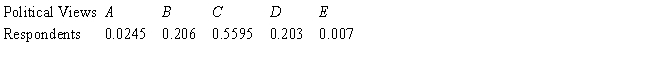
B)
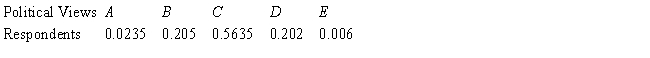
C)
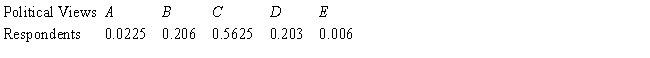
D)
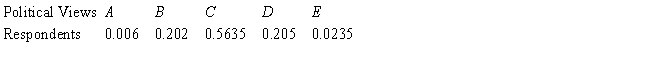

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
50
Human blood is classified by the presence or absence of three main antigens (A, B, and Rh). When a blood specimen is typed, the presence of the A and/or B antigen is indicated by listing the letter A and/or the letter B. If neither the A nor B antigen is present, the letter O is used. The presence or absence of the Rh antigen is indicated by the symbols + or -, respectively. Thus, if a blood specimen is classified as AB+, it contains the A and the B antigens as well as the Rh antigen. Similarly, O- blood contains none of the three antigens.
Using this information, determine the sample space corresponding to the different blood groups.
Using this information, determine the sample space corresponding to the different blood groups.

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
51
List the simple events associated with the given experiment.
As part of a quality-control procedure, eight circuit boards are checked, and the number of defectives is recorded.
A) {number 0 is recorded}, {number 1 is recorded}, {number 2 is recorded}, {number 3 is recorded}, {number 4 is recorded}, {number 5 is recorded}, {number 6 is recorded}, {number 7 is recorded}, {number 8 is recorded}, {number 9 is recorded}, {number 10 is recorded}
B) Ø
C) {number 8 is recorded}
D) {number 0 is recorded}, {number 1 is recorded}, {number 2 is recorded}, {number 3 is recorded}, {number 4 is recorded}, {number 5 is recorded}, {number 6 is recorded}, {number 7 is recorded}, {number 8 is recorded}
As part of a quality-control procedure, eight circuit boards are checked, and the number of defectives is recorded.
A) {number 0 is recorded}, {number 1 is recorded}, {number 2 is recorded}, {number 3 is recorded}, {number 4 is recorded}, {number 5 is recorded}, {number 6 is recorded}, {number 7 is recorded}, {number 8 is recorded}, {number 9 is recorded}, {number 10 is recorded}
B) Ø
C) {number 8 is recorded}
D) {number 0 is recorded}, {number 1 is recorded}, {number 2 is recorded}, {number 3 is recorded}, {number 4 is recorded}, {number 5 is recorded}, {number 6 is recorded}, {number 7 is recorded}, {number 8 is recorded}

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
52
The following breakdown of a total of 18,597 transportation fatalities that occured in 2007 was obtained from records compiled by the U.S. Department of Transportation (DOT).  What is the probability that a victim randomly selected from this list of transportation fatalities for 2007 died in a train or a plane accident? Round answer to two decimal places.
What is the probability that a victim randomly selected from this list of transportation fatalities for 2007 died in a train or a plane accident? Round answer to two decimal places.
A) 0.05
B) 0.11
C) 0.07
D) 0.08
 What is the probability that a victim randomly selected from this list of transportation fatalities for 2007 died in a train or a plane accident? Round answer to two decimal places.
What is the probability that a victim randomly selected from this list of transportation fatalities for 2007 died in a train or a plane accident? Round answer to two decimal places.A) 0.05
B) 0.11
C) 0.07
D) 0.08

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
53
According to a survey of 176 retailers, 41% of them use electronic tags as protection against shoplifting and employee theft. If one of these retailers is selected at random, what is the probability that he or she uses electronic tags as anti-theft devices?
A) The probability is 0.40
B) The probability is 0.42
C) The probability is 0.41
D) The probability is 0.43
A) The probability is 0.40
B) The probability is 0.42
C) The probability is 0.41
D) The probability is 0.43

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
54
Let 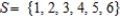 ,
, 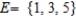 ,
, 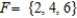 and
and  .
.
Are the events F and G complementary? Answer yes or no.
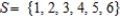 ,
, 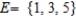 ,
, 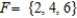 and
and  .
.
Are the events F and G complementary? Answer yes or no.

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
55
In a survey of 700 likely voters, the following question was asked: Do you support using cameras to identify red-light runners? The results of the survey follow: 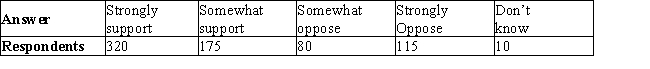 What is the probability that a person in the survey selected at random favors using cameras to identify red-light runners?
What is the probability that a person in the survey selected at random favors using cameras to identify red-light runners?
A) 0.66
B) 0.71
C) 0.29
D) 0.61
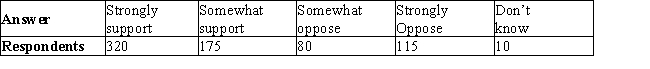 What is the probability that a person in the survey selected at random favors using cameras to identify red-light runners?
What is the probability that a person in the survey selected at random favors using cameras to identify red-light runners?A) 0.66
B) 0.71
C) 0.29
D) 0.61

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
56
According to data obtained from the National Weather Service, 390 of the 442 people killed by lightning in the United States between 1985 and 1992 were men. (Job and recreational habits of men make them more vulnerable to lightning.)
Assuming that this trend holds in the future, what is the probability that a person killed by lightning is a male? Round your answer to the nearest thousandth.
A) The probability is 0.879
B) The probability is 0.882
C) The probability is 0.003
D) The probability is 0.885
Assuming that this trend holds in the future, what is the probability that a person killed by lightning is a male? Round your answer to the nearest thousandth.
A) The probability is 0.879
B) The probability is 0.882
C) The probability is 0.003
D) The probability is 0.885

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
57
According to Mediamark Research, 86 million out of 179 million adults in the United States correct their vision by using prescription eyeglasses, bifocals, or contact lenses. (Some respondents use more than one type.) What is the probability that an adult selected at random from the adult population uses corrective lenses? Round your answer to the nearest thousandth.
A) The probability is 0.481
B) The probability is 0.479
C) The probability is 0.001
D) The probability is 0.480
A) The probability is 0.481
B) The probability is 0.479
C) The probability is 0.001
D) The probability is 0.480

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
58
Let S be a sample space for an experiment and let N and L be events of this experiment. Show that the events N ∪ L and Nc ∩ Lc are mutually exclusive.
Hint: Use De Morgan's law.
Hint: Use De Morgan's law.

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
59
Let 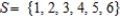 ,
,  ,
, 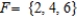 and
and 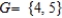 .
.
Are the events F and G mutually exclusive? Answer yes or no.
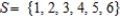 ,
,  ,
, 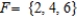 and
and 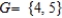 .
.
Are the events F and G mutually exclusive? Answer yes or no.

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
60
The percentage of the general population that has each blood type is shown in the table:  Determine the probability distribution associated with these data.
Determine the probability distribution associated with these data.
A) Blood Type A B AB O
Population, % 0.36 0.11 0.05 0.48
B) Blood Type A B AB O
Population, % 0.4 0.11 0.03 0.46
C) Blood Type A B AB O
Population, % 0.46 0.03 0.11 0.4
D) Blood Type A B AB O
Population, % 0.42 0.13 0.03 0.42
 Determine the probability distribution associated with these data.
Determine the probability distribution associated with these data.A) Blood Type A B AB O
Population, % 0.36 0.11 0.05 0.48
B) Blood Type A B AB O
Population, % 0.4 0.11 0.03 0.46
C) Blood Type A B AB O
Population, % 0.46 0.03 0.11 0.4
D) Blood Type A B AB O
Population, % 0.42 0.13 0.03 0.42

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
61
The grade distribution for a certain class is shown in the table. 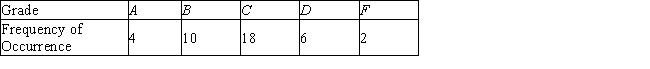 What is the probability that a student selected at random from this class received a passing grade (D or better)? Round to two decimal places, if necessary, and be sure to express answer in decimal form, not as a percentage.
What is the probability that a student selected at random from this class received a passing grade (D or better)? Round to two decimal places, if necessary, and be sure to express answer in decimal form, not as a percentage.
The probability is __________.
 What is the probability that a student selected at random from this class received a passing grade (D or better)? Round to two decimal places, if necessary, and be sure to express answer in decimal form, not as a percentage.
What is the probability that a student selected at random from this class received a passing grade (D or better)? Round to two decimal places, if necessary, and be sure to express answer in decimal form, not as a percentage.The probability is __________.

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
62
If a ball is selected at random from an urn containing three red balls, five white balls, and two blue balls, what is the probability that it will be a white ball? Round to two decimal places, if necessary, and be sure to express answer in decimal form, not as a percentage.
The probability is __________.
The probability is __________.

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
63
A pair of fair dice is cast. What is the probability that the sum of the numbers shown uppermost is less than 6?
A) The probability is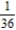
B) The probability is
C) The probability is
D) The probability is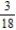
A) The probability is
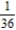
B) The probability is

C) The probability is

D) The probability is
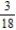

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
64
According to data obtained from the National Weather Service, 387 of the 432 people killed by lightning in the United States between 1985 and 1992 were men. (Job and recreational habits of men make them more vulnerable to lightning.)
Assuming that this trend holds in the future, what is the probability that a person killed by lightning is a male? Round to three decimal places, if necessary, and be sure to express answer in decimal form, not as a percentage.
The probability is __________.
Assuming that this trend holds in the future, what is the probability that a person killed by lightning is a male? Round to three decimal places, if necessary, and be sure to express answer in decimal form, not as a percentage.
The probability is __________.

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
65
According to Mediamark Research, 85 million out of 179 million adults in the United States correct their vision by using prescription eyeglasses, bifocals, or contact lenses. (Some respondents use more than one type.)
What is the probability that an adult selected at random from the adult population uses corrective lenses? Round to three decimal places, if necessary, and be sure to express answer in decimal form, not as a percentage.
The probability is __________ .
What is the probability that an adult selected at random from the adult population uses corrective lenses? Round to three decimal places, if necessary, and be sure to express answer in decimal form, not as a percentage.
The probability is __________ .

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
66
According to a survey of 176 retailers, 51% of them use electronic tags as protection against shoplifting and employee theft.
If one of these retailers is selected at random, what is the probability that he or she uses electronic tags as anti-theft devices? Round to two decimal places, if necessary, and be sure to express answer in decimal form, not as a percentage.
The probability is __________.
If one of these retailers is selected at random, what is the probability that he or she uses electronic tags as anti-theft devices? Round to two decimal places, if necessary, and be sure to express answer in decimal form, not as a percentage.
The probability is __________.

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
67
An opinion poll was conducted among a group of registered voters in a certain state concerning a proposition aimed at limiting state and local taxes. Results of the poll indicated that 31% of the voters favored the proposition, 37% were against it, and the remaining group were undecided. If the results of the poll are assumed to be representative of the opinions of the state's electorate, what is the probability that a registered voter selected at random from the electorate is undecided about the proposition?
A) 0.26
B) 0.28
C) 0.30
D) 0.32
E) 0.24
A) 0.26
B) 0.28
C) 0.30
D) 0.32
E) 0.24

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
68
Consider the composition of a three-child family in which the children were born at different times. Assume that a girl is as likely as a boy at each birth. What is the probability that there are two girls and a boy in the family?
A)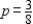
B)
C)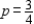
D)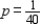
E)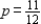
A)
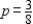
B)

C)
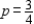
D)
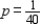
E)
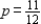

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
69
What is the probability of arriving at a traffic light when it is red if the red signal is flashed for 35 sec, the yellow signal for 5 sec, and the green signal for 60 sec?
A) The probability is 0.30
B) The probability is 0.35
C) The probability is 0.45
D) The probability is 0.40
A) The probability is 0.30
B) The probability is 0.35
C) The probability is 0.45
D) The probability is 0.40

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
70
The grade distribution for a certain class is shown in the table. 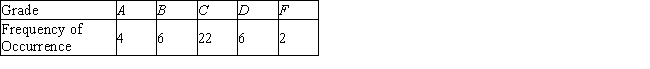 What is the probability that a student selected at random from this class received a passing grade (D or better)?
What is the probability that a student selected at random from this class received a passing grade (D or better)?
A) The probability is 0.95
B) The probability is 0.99
C) The probability is 0.97
D) The probability is 0.93
 What is the probability that a student selected at random from this class received a passing grade (D or better)?
What is the probability that a student selected at random from this class received a passing grade (D or better)?A) The probability is 0.95
B) The probability is 0.99
C) The probability is 0.97
D) The probability is 0.93

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
71
A study conducted by the Corrections Department of a certain state revealed that 162,883 people out of a total adult population of 1,713,709 were under correctional supervision (on probation, parole, or in jail). What is the probability that a person selected at random from the adult population in that state is under correctional supervision?
A)
B)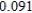
C)
D)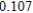
E)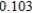
A)

B)
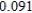
C)

D)
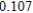
E)
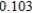

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
72
A study conducted by the Corrections Department of a certain state revealed that 166,756 people out of a total adult population of 1,714,697 were under correctional supervision (on probation, parole, or in jail).
What is the probability that a person selected at random from the adult population in that state is under correctional supervision? Round to three decimal places, if necessary, and be sure to express answer in decimal form, not as a percentage.
The probability is __________.
What is the probability that a person selected at random from the adult population in that state is under correctional supervision? Round to three decimal places, if necessary, and be sure to express answer in decimal form, not as a percentage.
The probability is __________.

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
73
The number of cars entering a tunnel leading to an airport in a major city over a period of 200 peak hours was observed and the following data were obtained.
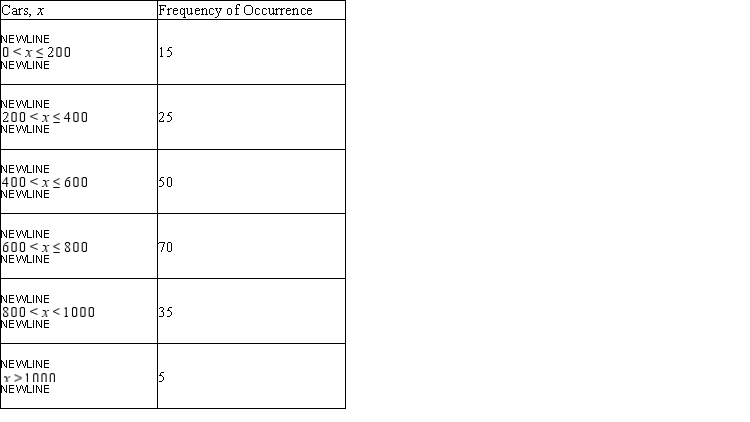
What is the probability that more than 600 cars will enter the airport tunnel during a peak hour?
A)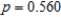
B)
C)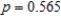
D)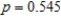
E)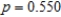

What is the probability that more than 600 cars will enter the airport tunnel during a peak hour?
A)
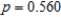
B)

C)
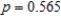
D)
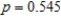
E)
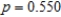

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
74
In a sweepstakes sponsored by Gemini Paper Products, 100,000 entries have been received. If 2 grand prize, 5 first prizes, 35 second prizes, and 600 third prizes are to be awarded, what is the probability that a person who has submitted one entry will win a prize? Round to five decimal places, if necessary, and be sure to express answer in decimal form, not as a percentage.
The probability is __________.
The probability is __________.

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
75
In a sweepstakes sponsored by Gemini Paper Products, 100,000 entries have been received. If 3 grand prize, 5 first prizes, 30 second prizes, and 400 third prizes are to be awarded, what is the probability that a person who has submitted one entry will win a prize?
A) The probability is 0.00508
B) The probability is 0.01138
C) The probability is 0.00438
D) The probability is 0.00445
A) The probability is 0.00508
B) The probability is 0.01138
C) The probability is 0.00438
D) The probability is 0.00445

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
76
What is the probability of arriving at a traffic light when it is red if the red signal is flashed for 15 sec, the yellow signal for 5 sec, and the green signal for 55 sec? Round to two decimal places, if necessary, and be sure to express answer in decimal form, not as a percentage.
The probability is __________.
The probability is __________.

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
77
One light bulb is selected at random from a lot of 100 light bulbs, of which 6% are defective.
What is the probability that the light bulb selected is defective? Round to two decimal places, if necessary, and be sure to express answer in decimal form, not as a percentage.
The probability is __________.
What is the probability that the light bulb selected is defective? Round to two decimal places, if necessary, and be sure to express answer in decimal form, not as a percentage.
The probability is __________.

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
78
In a survey conducted in 2007 of 970 adults 18 years old and older, the following question was asked: How are American companies doing on protecting the environment compared with companies in other countries? The results are summarized below:  If an adult in the survey selected at random, what is the probability that he or she said that American companies are equal or ahead on protecting the environment compared with companies in other countries? Round your answer to two decimal places.
If an adult in the survey selected at random, what is the probability that he or she said that American companies are equal or ahead on protecting the environment compared with companies in other countries? Round your answer to two decimal places.
A) 0.52
B) 0.45
C) 0.60
D) 0.65
E) 0.38
 If an adult in the survey selected at random, what is the probability that he or she said that American companies are equal or ahead on protecting the environment compared with companies in other countries? Round your answer to two decimal places.
If an adult in the survey selected at random, what is the probability that he or she said that American companies are equal or ahead on protecting the environment compared with companies in other countries? Round your answer to two decimal places.A) 0.52
B) 0.45
C) 0.60
D) 0.65
E) 0.38

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
79
Let 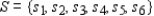 be the sample space associated with an experiment having the following probability distribution:
be the sample space associated with an experiment having the following probability distribution:  Find the probability of the event
Find the probability of the event  .
.
A) The probability is 0
B) The probability is
C) The probability is
D) The probability is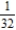
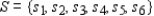 be the sample space associated with an experiment having the following probability distribution:
be the sample space associated with an experiment having the following probability distribution:  Find the probability of the event
Find the probability of the event  .
.A) The probability is 0
B) The probability is

C) The probability is

D) The probability is
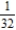

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck
80
Determine whether the given experiment has a sample space with equally likely outcomes. Two fair dice are cast, and the sum of the numbers appearing uppermost is recorded.
A) yes
B) no
A) yes
B) no

Unlock Deck
Unlock for access to all 273 flashcards in this deck.
Unlock Deck
k this deck


