TABLE 14-15 the Superintendent of a School District Wanted to Predict the Predict

True/False

TABLE 14-15
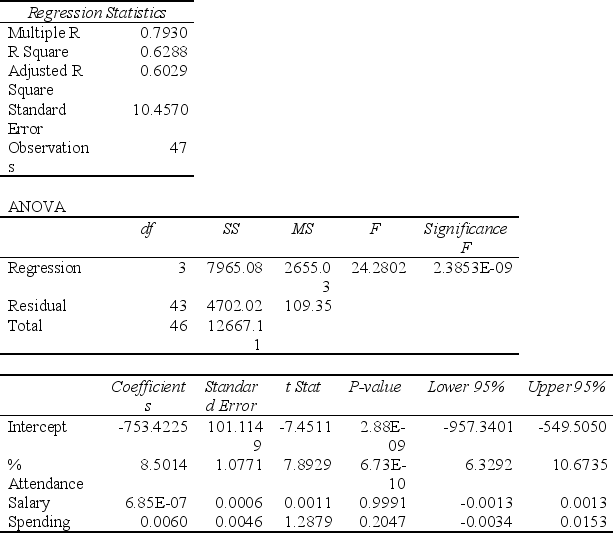
The superintendent of a school district wanted to predict the percentage of students passing a sixth-grade proficiency test. She obtained the data on percentage of students passing the proficiency test (% Passing), daily average of the percentage of students attending class (% Attendance), average teacher salary in dollars (Salaries), and instructional spending per pupil in dollars (Spending) of 47 schools in the state.
Following is the multiple regression output with Y = % Passing as the dependent variable, X₁= % Attendance, X₂= Salaries and X₃= Spending:
Note:

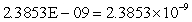
-Referring to Table 14-15, the null hypothesis H₀ : β₁ = β₂ = β₃ = 0 implies that percentage of students passing the proficiency test is not affected by any of the explanatory variables.