Abby Kratz, a Market Specialist at the Market Research Firm

Multiple Choice

Abby Kratz, a market specialist at the market research firm of Saez, Sikes, and Spitz, is analyzing household budget data collected by her firm.Abby's dependent variable is weekly household expenditures on groceries (in $'s) , and her independent variables are annual household income (in $1,000's) and household neighborhood (0 = suburban, 1 = rural) .Regression analysis of the data yielded the following table.
$\begin{array}{c}\begin{array}{|l|}\hline \text { } \\\hline \text {Intercept}\\\hline \text {\( X_{1} \) (income) }\\\hline \text {\( \mathrm{X}_{2} \) }\\ \text {(neighborhood) }\\\hline \end{array}\begin{array}{l}\hline \text { Coefficients }\\\hline 19.68247 \\\hline 1.735272 \\\hline 49.12456\\\\\hline \end{array}\begin{array}{|l|}\hline \text {Standard Error}\\\hline10.01176 \\\hline 0.174564 \\\hline 7.655776\\\\\hline \end{array}\begin{array}{l|}\hline t \text { Statistic } \\\hline 1.965934 \\\hline 9.940612 \\\hline 6.416667 \\\\\hline \end{array}\begin{array}{l|}\hline p \text {-value }\\\hline0.077667\\\hline1.68 \mathrm{E}-06\\\hline7.67 \mathrm{E}-05\\\\\hline\end{array}\end{array}$
Abby's model is ________________.

A) 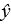$\mathrm{X}_{2}$ }\\ \text {(neighborhood) }\\ \hline \end{array} \begin{array}{l} \hline \text { Coefficients }\\ \hline 19.68247 \\ \hline 1.735272 \\ \hline 49.12456\\ \\ \hline \end{array} \begin{array}{|l|} \hline \text {Standard Error}\\ \hline10.01176 \\ \hline 0.174564 \\ \hline 7.655776\\ \\ \hline \end{array} \begin{array}{l|} \hline t \text { Statistic } \\ \hline 1.965934 \\ \hline 9.940612 \\ \hline 6.416667 \\ \\ \hline \end{array} \begin{array}{l|} \hline p \text {-value }\\ \hline0.077667\\ \hline1.68 \mathrm{E}-06\\ \hline7.67 \mathrm{E}-05\\ \\ \hline \end{array} \end{array} Abby's model is ________________. A) = 19.68247 + 10.01176 x₁ + 1.965934 x₂ B) = 1.965934 + 9.940612 x₁ + 6.416667 x₂ C) = 10.01176 + 0.174564 x₁ + 7.655776 x₂ D) = 19.68247 - 1.735272 x₁ + 49.12456 x₂ E) = 19.68247 + 1.735272 x₁ + 49.12456 x₂ " class="answers-bank-image d-block" rel="preload" > = 19.68247 + 10.01176 x₁ + 1.965934 x₂
B) 11efcd21_6411_aee5_b057_4518c9fddc20_TB7041_00= 1.965934 + 9.940612 x₁ + 6.416667 x₂
C) 11efcd21_6411_aee5_b057_4518c9fddc20_TB7041_00= 10.01176 + 0.174564 x₁ + 7.655776 x₂
D) 11efcd21_6411_aee5_b057_4518c9fddc20_TB7041_00= 19.68247 - 1.735272 x₁ + 49.12456 x₂
E) 11efcd21_6411_aee5_b057_4518c9fddc20_TB7041_00 = 19.68247 + 1.735272 x₁ + 49.12456 x₂