Essay
A cellular phone company wants to locate two new communications towers to cover 4 regions. The company wants to minimize the cost of installing the two towers. The regions that can be covered by each tower site are indicated by a 1 in the following table:
Based on this ILP formulation of the problem what formulas should go in cells F6:F14 of the following Excel spreadsheet?
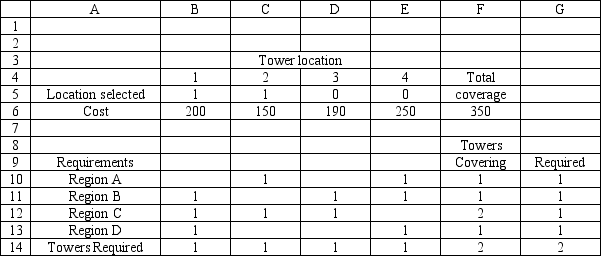
Correct Answer:

Verified
Correct Answer:
Verified
Q2: A company is planning next month's
Q5: Which of the following are potential pitfalls
Q28: How is an LP problem changed into
Q38: A company has four projects, numbered 1
Q45: A company wants to build a
Q49: A company produces three products which
Q51: A research director must pick a
Q54: A company is developing its weekly
Q55: A certain military deployment requires supplies
Q67: A company wants to select 1