Multiple Choice
Select a graph of the function and the tangent line at the point .Use the graph to approximate the slope of the tangent line.
A) 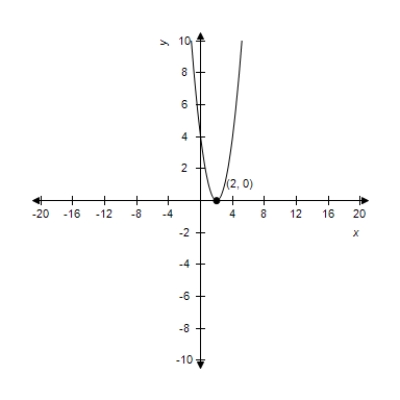 Slope at
Slope at
B) 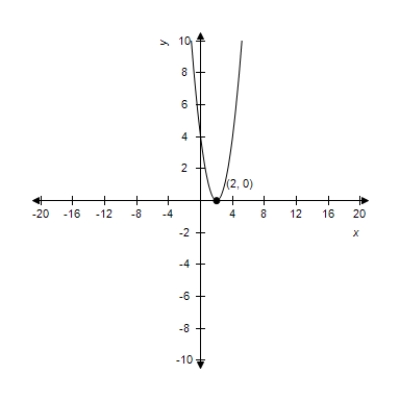 Slope at
Slope at
C)  Slope at
Slope at
D)  Slope at
Slope at
E) 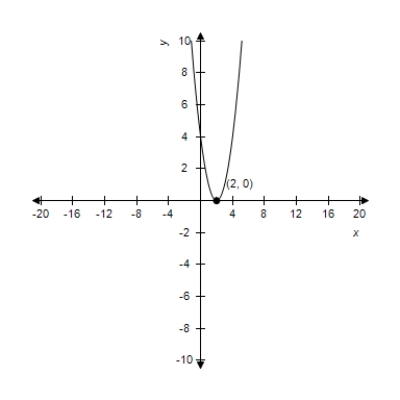 Slope at
Slope at
Correct Answer:

Verified
Correct Answer:
Verified
Related Questions
Q37: Rewrite the sum as a rational
Q38: Evaluate the sum using the summation
Q39: Find the derivative of the function.
Q40: Select the correct graph for the
Q41: Find the slope of the graph
Q43: Evaluate the sum using the summation
Q44: Use the graph to find
Q45: Select the correct graph for the
Q46: Given that <span class="ql-formula" data-value="\lim
Q47: Find the limit (if it exists).