Short Answer
A regression analysis between sales (in $1000) and advertising (in $) yielded the least squares line 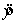 in sales. }\\ \hline B&\text { increase in advertising is expected to result in an increase of in sales.}\\ \hline C&\text { increase of in advertising is expected to result in an increase of in sales. }\\ \hline D&\text {increase of in advertising is expected to result in an increase of in sales. }\\ \hline \end{array} " class="answers-bank-image d-inline" rel="preload" > = 80 000 + 5x. This implies that an:
in sales. }\\ \hline B&\text { increase in advertising is expected to result in an increase of in sales.}\\ \hline C&\text { increase of in advertising is expected to result in an increase of in sales. }\\ \hline D&\text {increase of in advertising is expected to result in an increase of in sales. }\\ \hline \end{array} " class="answers-bank-image d-inline" rel="preload" > = 80 000 + 5x. This implies that an:
Correct Answer:

Verified
Correct Answer:
Verified
Q51: A professor of economics wants to
Q53: If the sum of squared residuals
Q54: In a regression problem the following
Q55: A professor of economics wants to
Q57: The variance of the error variable,
Q58: A professor of economics wants to
Q59: In regression analysis, if the coefficient
Q61: The residuals are observations of the
Q233: An outlier is an observation that is
Q248: Another name for the residual term in