A Rectangular Beam, Supported at Its Two Ends, Will Sag $S = C p x ^ { 4 } / w h ^ { 3 }$

Multiple Choice

A rectangular beam, supported at its two ends, will sag when subjected to a uniform load.The amount of sag is calculated from the formula: $S = C p x ^ { 4 } / w h ^ { 3 }$ , where p is the load (in Newtons per meter) , x is the length between supports (in meters) , w is the width of the beam (in meters) , h is the height of the beam (in meters) , and C is a constant (depending on material and units of measurement used) .Determine $\partial S / \partial h$ for a beam 3 m long, 0.1 m wide, 0.2 m high subjected to a load of 80 N/m.

A) $\Delta S / \partial h_{(803,0102) }=$ -121,500,000 C.
B) 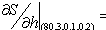-40,500,000 C.
C) 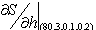121,500,000 C.
D) 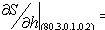40,500,000 C.