Multiple Choice
The report describes the results of a survey of 739 automobile owners age 18 to 29 and 564 automobile owners age 30 to 49 in a certain metropolitan area. Survey participants were asked if they own an automobile that is equipped with an electrical engine; 42% of the automobile owners age 18 to 29 and 36% of the automobile owners age 30 to 49 said "yes". Suppose you want to decide if there is evidence that the proportion of owners of automobiles equipped with electrical engine is different for age 18 to 29 and age 30 to 49.
What is the value of the test statistics and what is the associated P-value? If a significance level of 0.05 is selected for the test, will you reject or fail to reject the null hypothesis?
A) 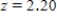 ;
; 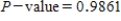 ; We reject H0; Assuming that the samples are representative of the populations of interest, we would conclude that the proportion of owners of automobiles equipped with electrical engine differs for age 18 to 29 and age 30 to 49.
; We reject H0; Assuming that the samples are representative of the populations of interest, we would conclude that the proportion of owners of automobiles equipped with electrical engine differs for age 18 to 29 and age 30 to 49.
B) 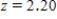 ;
; 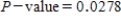 ; We reject H0; Assuming that the samples are representative of the populations of interest, we would conclude that the proportion of owners of automobiles equipped with electrical engine differs for age 18 to 29 and age 30 to 49.
; We reject H0; Assuming that the samples are representative of the populations of interest, we would conclude that the proportion of owners of automobiles equipped with electrical engine differs for age 18 to 29 and age 30 to 49.
C) 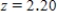 ;
; 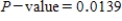 ; We fail to reject H0; Assuming that the samples are representative of the populations of interest, we would conclude that there is no evidence the proportion of owners of automobiles equipped with electrical engine differs for age 18 to 29 and age 30 to 49.
; We fail to reject H0; Assuming that the samples are representative of the populations of interest, we would conclude that there is no evidence the proportion of owners of automobiles equipped with electrical engine differs for age 18 to 29 and age 30 to 49.
D) 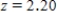 ;
; 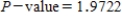 ; We fail to reject H0; Assuming that the samples are representative of the populations of interest, we would conclude that there is no evidence the proportion of owners of automobiles equipped with electrical engine differs for age 18 to 29 and age 30 to 49.
; We fail to reject H0; Assuming that the samples are representative of the populations of interest, we would conclude that there is no evidence the proportion of owners of automobiles equipped with electrical engine differs for age 18 to 29 and age 30 to 49.
E) 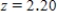 ;
; 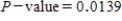 ; We reject H0; Assuming that the samples are representative of the populations of interest, we would conclude that the proportion of owners of automobiles equipped with electrical engine differs for age 18 to 29 and age 30 to 49.
; We reject H0; Assuming that the samples are representative of the populations of interest, we would conclude that the proportion of owners of automobiles equipped with electrical engine differs for age 18 to 29 and age 30 to 49.
Correct Answer:

Verified
Correct Answer:
Verified
Q7: An article describes the results of an
Q8: The hypothesis <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB7677/.jpg" alt="The hypothesis
Q9: Compare the results of the survey and
Q10: An article describes the results of an
Q11: One of the most striking human accomplishments
Q13: An article describes an experiment that investigated
Q14: A study determined that in 2009, 38%
Q15: A study seeks to determine whether the
Q16: A study compared flu vaccine administered by
Q17: One of the most striking human accomplishments