Multiple Choice
a. Use transformations to graph the function.
b. Write the domain and range in interval notation.
c. Determine the vertical asymptote.
-
A) a.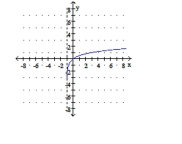
b. domain: , range
c. vertical asymptote:
B) a.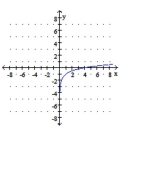
b. domain: , range
c. vertical asymptote:
C) a.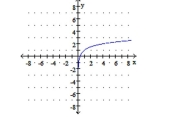
b. domain: , range
c. vertical asymptote:
D) a.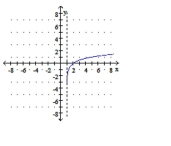
b. domain: , range
c. vertical asymptote:
Correct Answer:

Verified
Correct Answer:
Verified
Q14: Choose the one alternative that best
Q15: Write the word or phrase that
Q16: ng, in<br>-From <span class="ql-formula" data-value="1995
Q17: Write the word or phrase that
Q18: Write the logarithmic expression as a
Q20: Solve the problem.<br>-A <span class="ql-formula"
Q21: Choose the one alternative that best
Q22: Solve the logarithmic equation.<br>- <span class="ql-formula"
Q23: Choose the one alternative that best
Q24: A function defined by <span