SCENARIO 14-16
What Are the Factors That Determine the Acceleration $Y$

Short Answer

SCENARIO 14-16
What are the factors that determine the acceleration time (in sec.) from 0 to 60 miles per hour of a
car? Data on the following variables for 30 different vehicle models were collected: $Y$ (Accel Time): Acceleration time in sec.
$X _ { I }$ (Engine Size): c.c.
$X _ { 2 }$ (Sedan): 1 if the vehicle model is a sedan and 0 otherwise

The regression results using acceleration time as the dependent variable and the remaining variables as the independent variables are presented below.

$\begin{array}{lr}\hline{\text { Regression Statistics }} \\\hline \text { Multiple R } & 0.6096 \\\text { R Square } & 0.3716 \\\text { Adjusted R Square } & 0.3251 \\\text { Standard Error } & 1.4629 \\\text { Observations } & 30 \\\hline\end{array}$

ANOVA



$\begin{array}{lrrrrrr}\hline & \text { Coefficients } & \text { Standard Error } & \text { t Stat } & \text { P-value } & \text { Lower 95\% } & \text { Upper 95\% } \\\hline \text { Intercept } & 7.1052 & 0.6574 & 10.8086 & 0.0000 & 5.7564 & 8.4540 \\\text { Engine Size } & -0.0005 & 0.0001 & -3.6477 & 0.0011 & -0.0008 & -0.0002 \\\text { Sedan } & 0.7264 & 0.5564 & 1.3056 & 0.2027 & -0.4152 & 1.8681 \\\hline\end{array}$


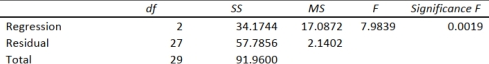
-Referring to Scenario 14-16, what is the correct interpretation for the estimated coefficient for $X _ { 1 }$ ?
A) As the 0 to 60 miles per hour acceleration time increases by one second, the mean engine size will decrease by an estimated 0.0005 c.c. without taking into consideration the other
Independent variable included in the model.
B) As the engine size increases by one c.c., the mean 0 to 60 miles per hour acceleration time will decrease by an estimated 0.0005 seconds without taking into consideration the
Other independent variable included in the model.
C) As the 0 to 60 miles per hour acceleration time increases by one second, the mean engine size will decrease by an estimated 0.0005 c.c. taking into consideration the other
Independent variable included in the model.
D) As the engine size increases by one c.c., the mean 0 to 60 miles per hour acceleration time will decrease by an estimated 0.0005 seconds taking into consideration the other
Independent variable included in the model.