Solve the Problem $$a _ { n } = a n + b$$

Multiple Choice

Solve the problem.
-The paired data below consist of the costs of advertising (in thousands of dollars) and the number of products sold (in thousands) . (i) Use a graphing calculator to fit a linear sequence regression function
$$a _ { n } = a n + b$$
to the data, where $n$ is the cost of advertising (in thousands of dollars) and $a _ { n }$ is number of products sold (in thousands) . (ii) Use this function to estimate the number of products sold if the cost of advertising is 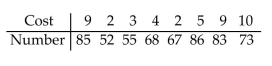$\$ 6000$ .

A) (i) $a _ { n } = 2.79 n + 55.8$ ;
B) (i) $a _ { n } = 5.79 n + 94.3$ ;
(ii) $72.54$ thousand products
(ii) $16,795.8$ products
C) (i) $a _ { n } = 3.02 n + 55.8$ ;
D) (i) $a _ { n } = 2.73 n + 34.8$ ;
(ii) $79.24$ thousand products
(ii) $69.54$ thousand products