Short Answer
Conduct Analysis of Variance on the Randomized Complete Block Design
-Assume that the data below come from populations that are normally distributed with the same variance. Let be the mean for treatment the mean for treatment 2 , and the mean for treatment 3 . In a test of : versus : at least one of the means is different, the null hypothesis is rejected at the level of significance. Use Tukey's test to determine which pairwise means differ using a familywise error rate of . Give the -value for each of the pairwise tests and state your conclusion.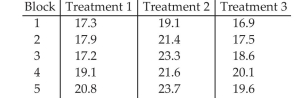
Correct Answer:

Verified
\[\begin{array} { l }
\mathrm { H } _ {...View Answer
Unlock this answer now
Get Access to more Verified Answers free of charge
Correct Answer:
Verified
\mathrm { H } _ {...
View Answer
Unlock this answer now
Get Access to more Verified Answers free of charge
Q7: How, in general, is the requirement of
Q16: Perform the Tukey Test<br>-The times (in
Q17: Test a Hypothesis Regarding Three or
Q18: Perform the Tukey Test<br>-A researcher is
Q19: Test a Hypothesis Regarding Three or
Q22: Test a Hypothesis Regarding Three or
Q23: Conduct Analysis of Variance on the
Q24: Conduct Analysis of Variance on the
Q25: Perform the Tukey Test<br>-Four different types
Q37: The analysis of variance is an inferential