Solved

Let S Be the Set of All Strings of 0's

Question 17

Essay

Let S be the set of all strings of 0's and 1's of length 3. Define a relation R on S as follows: for all strings s and t in S,
Let S be the set of all strings of 0's and 1's of length 3. Define a relation R on S as follows: for all strings s and t in S,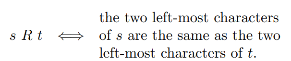 (a) Prove that R is an equivalence relation on S. (b) Find the distinct equivalence classes of R.
(a) Prove that R is an equivalence relation on S.
(b) Find the distinct equivalence classes of R.

Correct Answer:

verifed

Verified

a. Proof:
R is reflexive because for each...

View Answer

Unlock this answer now
Get Access to more Verified Answers free of charge

Related Questions

Q9: Let <span class="ql-formula" data-value="A =

Q10: Let R be the relation defined

Q11: Define a relation <span class="ql-formula"

Q12: Let <span class="ql-formula" data-value="B =

Q13: Find a positive inverse for 7 modulo

Q14: Define a relation <span class="ql-formula"

Q15: Let A = {1, 2, 3, 4}.

Q16: Prove directly from the definition of

Q18: Define a relation <span class="ql-formula"

Q19: Define a relation <span class="ql-formula"