Multiple Choice
Graph the function in the ts-plane (t-axis horizontal, s-axis vertical) . State the period and symmetry of the function.
- 
A) Period 4, symmetric about the s-axis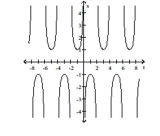
B) Period 4, symmetric about the origin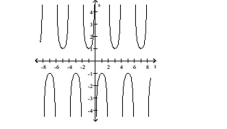
C) Period 4, symmetric about the origin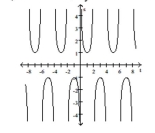
D) Period 4, symmetric about the s-axis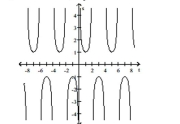
Correct Answer:

Verified
Correct Answer:
Verified
Q12: Rewrite the ratio as a ratio
Q13: Find the domain and range of
Q14: Find a formula for the function
Q15: Graph the function.<br>- <span class="ql-formula" data-value="\text
Q16: Solve the problem.<br>-The population of a
Q18: Express the given quantity in terms
Q19: Find the domain and range for
Q20: The problem tells by what factor
Q21: Determine if the function is even,
Q22: Is the function graphed below one-to-one?<br>-<img src="https://d2lvgg3v3hfg70.cloudfront.net/TB6591/.jpg"