Solved

-To Construct the Altitude from Vertex a to Side

Question 324

True/False

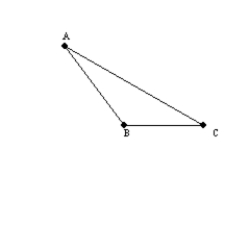
-To construct the altitude from vertex A to side of the obtuse triangle , one begins by constructing the midpoint of .
-To construct the altitude from vertex A to side -To construct the altitude from vertex A to side of the obtuse triangle , one begins by constructing the midpoint of . of the obtuse triangle -To construct the altitude from vertex A to side of the obtuse triangle , one begins by constructing the midpoint of . , one begins by constructing the midpoint of -To construct the altitude from vertex A to side 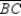of the obtuse triangle 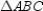, one begins by constructing the midpoint of . .

Correct Answer:

verifed

Verified

Unlock this answer now
Get Access to more Verified Answers free of charge

Related Questions

Q319: For an acute triangle, suppose that you

Q320: For <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB7237/.jpg" alt="For ,

Q321: <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB7237/.jpg" alt=" -To determine the

Q322: <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB7237/.jpg" alt=" -In the figure,

Q323: For a rectangle with base length of

Q325: If the measures of the angles of

Q326: <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB7237/.jpg" alt=" -For the right

Q327: <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB7237/.jpg" alt=" -In

Q328: <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB7237/.jpg" alt=" -In

Q329: <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB7237/.jpg" alt=" -The area of