Multiple Choice
TABLE 14-11
A weight-loss clinic wants to use regression analysis to build a model for weight-loss of a client (measured in pounds) . Two variables thought to affect weight-loss are client's length of time on the weight-loss program and time of session. These variables are described below:
Y = Weight-loss (in pounds)
X₁ = Length of time in weight-loss program (in months)
X₂ = 1 if morning session, 0 if not
X₃ = 1 if afternoon session, 0 if not (Base level = evening session)
Data for 12 clients on a weight-loss program at the clinic were collected and used to fit the interaction model:
Y = β₀ + β₁X₁ + β₂X₂ + β₃X₃ + β₄X₁X₂ + β₅X₁X₂ + ε
Partial output from Microsoft Excel follows:
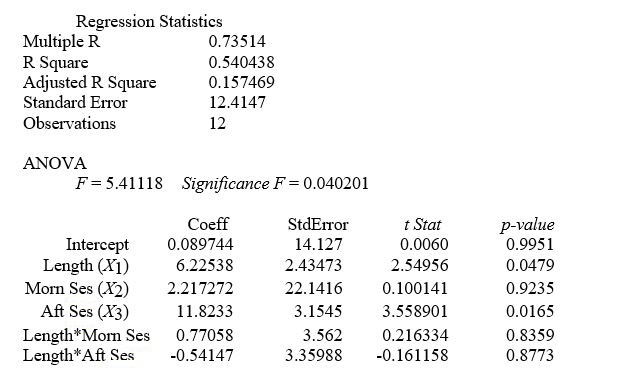
-Referring to Table 14-11, in terms of the βs in the model, give the mean change in weight-loss (Y) for every 1 month increase in time in the program (X₁) when attending the afternoon session.
A) β₁ + β₄
B) β₁ + β₅
C) β₁
D) β₄ + β₅
Correct Answer:

Verified
Correct Answer:
Verified
Q118: TABLE 14-8<br>A financial analyst wanted to examine
Q119: TABLE 14-18<br>A logistic regression model was estimated
Q120: TABLE 14-4<br>A real estate builder wishes to
Q122: TABLE 14-15<br>The superintendent of a school district
Q124: TABLE 14-17<br> <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB1602/.jpg" alt="TABLE 14-17
Q125: TABLE 14-16<br>What are the factors that determine
Q126: TABLE 14-19<br>The marketing manager for a nationally
Q127: TABLE 14-14<br>An automotive engineer would like to
Q128: TABLE 14-16<br>What are the factors that determine
Q220: A regression had the following results: SST