If _j, an Unbiased Estimator of _j, Is

Multiple Choice

If _j, an unbiased estimator of 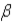_j, is consistent, then the:

A) distribution of _j becomes more and more loosely distributed around _j as the sample size grows.
B) distribution of _j becomes more and more tightly distributed around 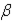_j as the sample size grows.
C) distribution of _j tends toward a standard normal distribution as the sample size grows.
D) distribution of _j remains unaffected as the sample size grows.