A Movie Critic Wanted to Determine Whether or Not Moviegoers

Short Answer

A movie critic wanted to determine whether or not moviegoers of different age groups evaluated a movie differently. With this objective, he commissioned a survey that asked people their ratings of the most recently watched movies. The rating categories were: 1 = terrible, 2 = fair, 3 = good, and 4 = excellent. Each respondent was also asked to categorize his or her age as either: 1 = teenager, 2 = young adult (20-34), 3 = middle age (35-50), and 4 = senior (over 50). The results are shown below. 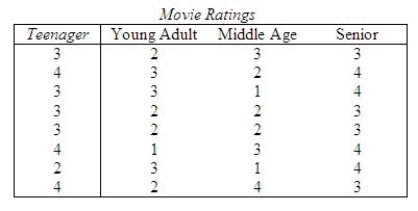Do these data provide sufficient evidence to infer at the 5% significance level that there were differences in ratings among the different age categories?
Test statistic:
H = ______________
What is the critical value for the test statistic?
Reject if the test statistic > ______________.
Conclude: ______________
There ______________ sufficient evidence to infer at the 5% significance level that there were differences in ratings among the different age categories.
What statement can be made about the p-value for this test?
______________