Short Answer
A survey of 500 respondents produced these cell counts in a 2  3 contingency table:
3 contingency table: 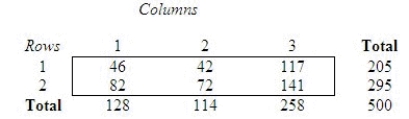 If you wish to test the null hypothesis of "independence" - that the probability that a response falls in any one row is independent of the column it falls in - and you plan to use a chi-square test, how many degrees of freedom will be associated with the
If you wish to test the null hypothesis of "independence" - that the probability that a response falls in any one row is independent of the column it falls in - and you plan to use a chi-square test, how many degrees of freedom will be associated with the 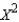 statistic?
statistic?
df = ______________
Compute 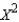 : = ______________
: = ______________
Reject 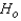 when
when 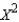 > ______________.
> ______________.
Conclude that there ______________ reason to expect a dependence between rows and columns.
Find the approximate p-value for the test.
The p-value ______________ .10
Correct Answer:

Verified
2; 4.1879;...View Answer
Unlock this answer now
Get Access to more Verified Answers free of charge
Correct Answer:
Verified
View Answer
Unlock this answer now
Get Access to more Verified Answers free of charge
Q28: A national survey stated that 30% of
Q29: A contingency table test with 4 rows
Q30: A multinomial experiment, where the outcome of
Q31: When the expected cell frequencies are smaller
Q32: Which of the following statements about a
Q34: If we wish to determine whether there
Q35: A large carpet store wishes to determine
Q36: A chi-square test for independence with 6
Q37: Consider a multinomial experiment with 200 trials,
Q38: All chi-square distributions are skewed to the