Related Questions
Q242: Decide if the function is an
Q243: State whether the function is an
Q244: Find the logistic function that satisfies
Q245: Find the logistic function that satisfies
Q246: Solve the problem.<br>-Suppose you contribute to a
Q248: Find the following using a calculator.
Q249: Assuming all variables are positive, use
Q250: Solve the problem.<br>-The formula R = log
Q251: Solve the problem.<br>-The Richter scale magnitude
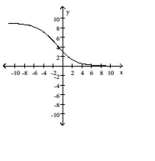
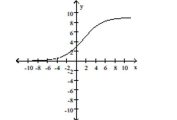
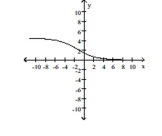
Q252: Graph the function. Describe its position