Multiple Choice
In a suspension bridge, the shape of the suspension cables is parabolic. The bridge shown in the figure has towers that are 1000 m apart, and the lowest point of the suspension cables is 200 m below the top of the towers. Find the equation of the parabolic part of the cables, placing the origin of the coordinate system at the lowest point of the cable. NOTE: This equation is used to find the length of the cable needed in the construction of the bridge. 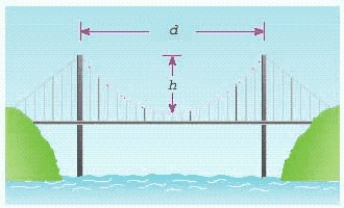
A) x 2 = -1,250y
B) x 2 = 1,250y
C) x 2 = 5,000y
D) x 2 = 2,500y
E) y 2 = 5,000x
Correct Answer:

Verified
Correct Answer:
Verified
Q11: Identify the conic by writing the equation
Q12: Find the vertex and focus of the
Q13: Write the equation of the ellipse with
Q14: Find the standard form of the equation
Q15: Write the equation of the ellipse that
Q17: Find the standard form of the equation
Q18: Find the equation of the parabola with
Q19: Find the center and vertices of the
Q20: Find the standard form of the equation
Q21: Graph the hyperbola. <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB8633/.jpg" alt="Graph the