TABLE 13-11 A Weight-Loss Clinic Wants to Use Regression Analysis to Build

Multiple Choice

TABLE 13-11
A weight-loss clinic wants to use regression analysis to build a model for weight loss of a client (measured in pounds) . Two variables thought to affect weight loss are client's length of time on the weight loss program and time of session. These variables are described below:
Y = Weight loss (in pounds)
X1 = Length of time in weight-loss program (in months)
X2 = 1 if morning session, 0 if not
X3 = 1 if afternoon session, 0 if not (Base level = evening session)
Data for 12 clients on a weight-loss program at the clinic were collected and used to fit the interaction model:
Y = β0 + β1X1 + β2X2 + β3X3 + β4X1X2 + β5X1X3 + ε
Partial output from Microsoft Excel follows:

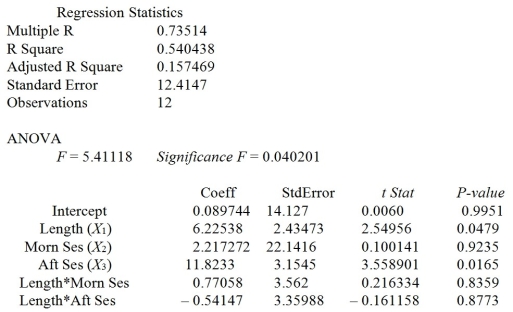
-Referring to Table 13-11, what null hypothesis would you test to determine whether the slope of the linear relationship between weight loss (Y) and time in the program (X₁) varies according to time of session?

A) H₀: β₁ = β₂ = β₃ = β₄ = β₅ = 0
B) H₀: β₂ = β₃ = β₄ = β₅ = 0
C) H₀: β₄ = β₅ = 0
D) H₀: β₂ = β₃ = 0