TABLE 13-15 the Superintendent of a School District Wanted to Predict the Predict

Multiple Choice

TABLE 13-15
The superintendent of a school district wanted to predict the percentage of students passing a sixth-grade proficiency test. She obtained the data on percentage of students passing the proficiency test (% Passing) , daily mean of the percentage of students attending class (% Attendance) , mean teacher salary in dollars (Salaries) , and instructional spending per pupil in dollars (Spending) of 47 schools in the state.
Following is the multiple regression output with Y = % Passing as the dependent variable, 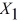= : % Attendance, 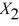= Salaries and 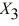= Spending:

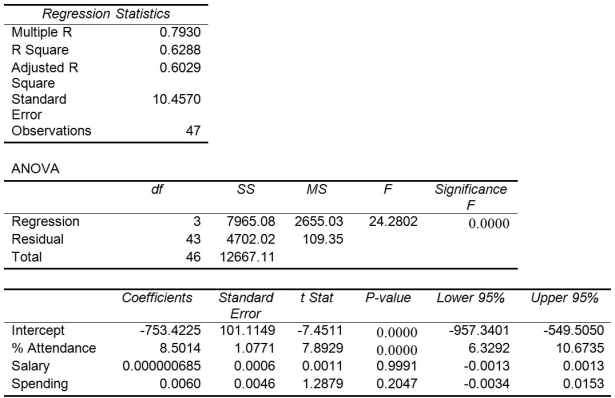
-Referring to Table 13-15, which of the following is the correct null hypothesis to determine whether there is a significant relationship between percentage of students passing the proficiency test and the entire set of explanatory variables?

A) H₀: β₀ = β₁ = β₂ = β₃ = 0
B) H₀: β₁ = β₂ = β₃ = 0
C) H₀: β₀ = β₁ = β₂ = β₃ ≠ 0
D) H₀: β₁ = β₂ = β₃ ≠ 0