Essay
for the circuit shown below, let
(a) Find impedances , and .
(b) Find the equivalent impedance of parallel combination of and .
(c) Find the total impedance seen from the voltage source.
(d) Find the phasor for the current I.
(e) Find the phasor for across the inductor .
(f) Find the phasors for and .
(g) Find .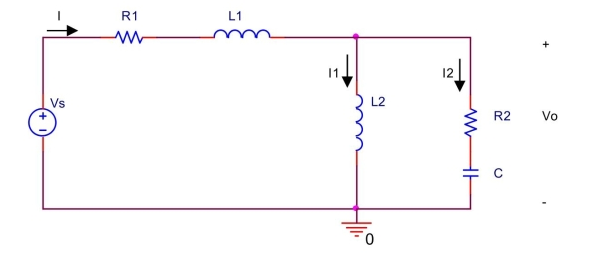
Correct Answer:

Verified
\[\begin{array} { l }
Z _ { \mathrm { L...View Answer
Unlock this answer now
Get Access to more Verified Answers free of charge
Correct Answer:
Verified
\[\begin{array} { l }
Z _ { \mathrm { L...
Z _ { \mathrm { L...
View Answer
Unlock this answer now
Get Access to more Verified Answers free of charge
Related Questions