Essay
Life Expectancy An actuary wanted to develop a model to predict how long individuals will live. After consulting a number of physicians, she collected the age at death ( y ), the average number of hours of exercise per week ( x 1), the cholesterol level ( x 2), and the number of points that the individual's blood pressure exceeded the recommended value ( x 3). A random sample of 40 individuals was selected. The computer output of the multiple regression model is shown below. THE REGRESSION EQUATION IS y = 55.8 + 1.79 x 1 - 0.021 x 2 - 0.061 x 3 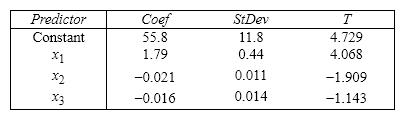
S = 9.47 R - Sq = 22.5%
ANALYSIS OF VARIANCE 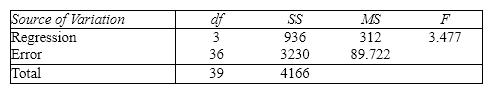 {Life Expectancy Narrative} Is there enough evidence at the 5% significance level to infer that the model is useful in predicting length of life?
{Life Expectancy Narrative} Is there enough evidence at the 5% significance level to infer that the model is useful in predicting length of life?
Correct Answer:

Verified

 At least one b i is not equal to zero. ...
At least one b i is not equal to zero. ...View Answer
Unlock this answer now
Get Access to more Verified Answers free of charge
Correct Answer:
Verified
View Answer
Unlock this answer now
Get Access to more Verified Answers free of charge
Q13: Life Expectancy An actuary wanted to develop
Q14: Real Estate Builder A real estate builder
Q15: Marc Anthony Concert At a recent Marc
Q22: Student's Final Grade A statistics professor investigated
Q33: We test an individual coefficient in a
Q43: One of the consequences of multicollinearity in
Q96: How do you go about checking for
Q129: Multicollinearity is present if the dependent variable
Q140: Some of the requirements for the error
Q170: When the error variable does not have