Figure 1:

Figure 1 Demonstrates an Excel

Multiple Choice

Figure 1:

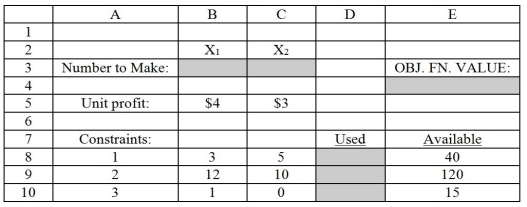
Figure 1 demonstrates an Excel spreadsheet that is used to model the following linear programming problem:
$$\begin{array} { l l } \operatorname { Max } : & 4 \mathrm { X } _ { 1 } + 3 \mathrm { X } _ { 2 } \\\text { Subject to: } & \\& 3 \mathrm { X } _ { 1 } + 5 \mathrm { X } _ { 2 } \leq 40 \\& 12 \mathrm { X } _ { 1 } + 10 \mathrm { X } _ { 2 } \leq 120 \\& \mathrm { X } _ { 1 } \geq 15 \\& \mathrm { X } _ { 1 } , \mathrm { X } _ { 2 } \geq 0\end{array}$$
Note: Cells B3 and C3 are the designated cells for the optimal values of X₁ and X₂,respectively,while cell E4 is the designated cell for the objective function value.Cells D8:D10 designate the left-hand side of the constraints.
-Refer to Figure 1.Which cell(s) are the Changing Cells as designated by "Solver"?

A) E4
B) B2:C2
C) B3:C3
D) D8:D10
E) B5:C5