Solved

Let V Be the Set of All Functions F

Question 15

Short Answer

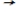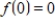Let V be the set of all functions f : R Let V be the set of all functions f : R R such that . Using the usual rules for vector addition and scalar multiplication of functions, determine if V is a vector space, and if not explain why. R such that Let V be the set of all functions f : R R such that . Using the usual rules for vector addition and scalar multiplication of functions, determine if V is a vector space, and if not explain why. . Using the usual rules for vector addition and scalar multiplication of functions, determine if V is a vector space, and if not explain why.

Correct Answer:

verifed

Verified

V is a vec...

View Answer

Unlock this answer now
Get Access to more Verified Answers free of charge

Related Questions

Q10: Determine if the given set is a

Q11: In the vector space <img src="https://d2lvgg3v3hfg70.cloudfront.net/TBMC1039/.jpg" alt="In

Q12: Determine if <img src="https://d2lvgg3v3hfg70.cloudfront.net/TBMC1039/.jpg" alt="Determine if

Q13: In the vector space <img src="https://d2lvgg3v3hfg70.cloudfront.net/TBMC1039/.jpg" alt="In

Q14: If V is a vector space and

Q16: Let V be a vector space with

Q17: If every finite set S in a

Q18: Determine if <img src="https://d2lvgg3v3hfg70.cloudfront.net/TBMC1039/.jpg" alt="Determine if

Q19: In the vector space <img src="https://d2lvgg3v3hfg70.cloudfront.net/TBMC1039/.jpg" alt="In

Q20: If <img src="https://d2lvgg3v3hfg70.cloudfront.net/TBMC1039/.jpg" alt="If and