Solved

Suppose That a Is an Matrix and Is

Question 6

True/False

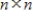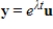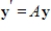Suppose that A is an Suppose that A is an matrix and is a solution to the system of linear differential equations where is an eigenvector of A with associated eigenvalue . If A is invertible, then is a solution to the system . matrix and Suppose that A is an matrix and is a solution to the system of linear differential equations where is an eigenvector of A with associated eigenvalue . If A is invertible, then is a solution to the system . is a solution to the system of linear differential equations Suppose that A is an matrix and is a solution to the system of linear differential equations where is an eigenvector of A with associated eigenvalue . If A is invertible, then is a solution to the system . where Suppose that A is an matrix and is a solution to the system of linear differential equations where is an eigenvector of A with associated eigenvalue . If A is invertible, then is a solution to the system . is an eigenvector of A with associated eigenvalue Suppose that A is an matrix and is a solution to the system of linear differential equations where is an eigenvector of A with associated eigenvalue . If A is invertible, then is a solution to the system .
. If A is invertible, then Suppose that A is an matrix and is a solution to the system of linear differential equations where is an eigenvector of A with associated eigenvalue . If A is invertible, then is a solution to the system . is a solution to the system Suppose that A is an matrix and is a solution to the system of linear differential equations where is an eigenvector of A with associated eigenvalue 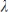. If A is invertible, then 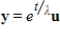is a solution to the system .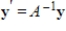
.

Correct Answer:

verifed

Verified

Unlock this answer now
Get Access to more Verified Answers free of charge

Related Questions

Q1: Diagonalize the matrix A, if possible.<br> <img

Q2: Find a basis for the eigenspace associated

Q3: Diagonalize the given matrix A, and use

Q4: The coefficient matrix for a system of

Q5: Compute the first two iterations of the

Q7: Suppose <img src="https://d2lvgg3v3hfg70.cloudfront.net/TBMC1039/.jpg" alt="Suppose is

Q8: If <img src="https://d2lvgg3v3hfg70.cloudfront.net/TBMC1039/.jpg" alt="If ,

Q9: If the <img src="https://d2lvgg3v3hfg70.cloudfront.net/TBMC1039/.jpg" alt="If the

Q10: The Shifted Inverse Power Method for an

Q11: Find the rotation-dilation matrix B within the