Tail-Feather Length Is a Sexually Dimorphic Trait in Long-Tailed Finches;

Multiple Choice

Tail-feather length is a sexually dimorphic trait in long-tailed finches; that is, the trait differs substantially for males and for females of the same species. A bird's size and environment might also influence tail-feather length. Researchers studied the relationship between tail-feather length (measuring the R1 central tail feather) and weight in a sample of 20 male long-tailed finches raised in an aviary and 5 male long-tailed finches caught in the wild. The data are displayed in the scatterplot below.
​
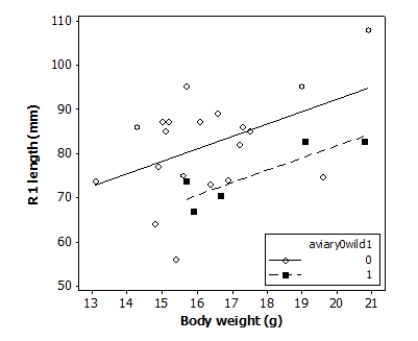The following model is proposed for predicting the length of the R1 feather from the bird's weight and origin:
R1Length_i = β₀ + β₁ (BodyWeight_i) + β₂ (Aviary0Wild1_i) + ε_i
Where the deviations ε_i were assumed to be independent and Normally distributed with mean 0 and standard deviation σ . This model was fit to the sample of 25 male finches. The following results summarize the least-squares regression fit of this model:
$\begin{array}{l}\begin{array}{lrr}\text { Predictor } & \text { Coef } & \text { SE Coef } \\\text { Constant } & 35.79 & 16.95 \\\text { BodyWeight } & 2.826 & 1.030 \\\text { Aviary0Wild1 } & -10.453 & 4.996\end{array}\\\mathrm{S}=9.62172 \quad \mathrm{R}-\mathrm{Sq}=30.2 \%\end{array}$

$\begin{array}{l}\text { Analysis of Variance }\\\begin{array}{lrrr}\text { Source } & \text { DF } & \text { SS } & \text { MS } \\\text { Regression } & 2 & 879.79 & 439.90 \\\text { Residual Error } & 22 & 2036.71 & 92.58 \\\text { Total } & 24 & 2916.50 &\end{array}\end{array}$ To determine whether the bird's origin affects tail-feather length in this model, we test the hypotheses
H₀: β₂ = 0, H_α: β₂ ≠ 0 in this particular model. Using the estimates provided, what is the P-value for this test?

A) Greater than 0.10
B) Between 0.05 and 0.10
C) Between 0.01 and 0.05
D) Less than 0.01