Solved

The Directional Derivative of a Function F(x,y) at a Point

Question 17

Multiple Choice

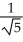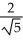The directional derivative of a function F(x,y) at a point P in the direction of the unit vector- The directional derivative of a function F(x,y) at a point P in the direction of the unit vector- i + j is equal to -4, while the directional derivative at P in the direction of the unit vector i+ j is equal to0. The value of F(P) is equal to: A) 20 B) ± 2 C) i - j D) i - j E) 4 i - 2 j i + The directional derivative of a function F(x,y) at a point P in the direction of the unit vector- i + j is equal to -4, while the directional derivative at P in the direction of the unit vector i+ j is equal to0. 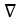The value of F(P) is equal to: A) 20 B) ± 2 C) i - j D) i - j E) 4 i - 2 j j is equal to -4, while the directional derivative at P in the direction of the unit vector The directional derivative of a function F(x,y) at a point P in the direction of the unit vector- i + j is equal to -4, while the directional derivative at P in the direction of the unit vector i+ j is equal to0. 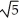The value of F(P) is equal to: A) 20 B) ± 2 C) i - j D) i - j E) 4 i - 2 j i+ The directional derivative of a function F(x,y) at a point P in the direction of the unit vector- i + j is equal to -4, while the directional derivative at P in the direction of the unit vector i+ j is equal to0. The value of F(P) is equal to: A) 20 B) ± 2 C) i - j D) i - j E) 4 i - 2 j j is equal to0. 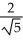The value ofThe directional derivative of a function F(x,y) at a point P in the direction of the unit vector- i + j is equal to -4, while the directional derivative at P in the direction of the unit vector i+ j is equal to0. The value of F(P) is equal to: A) 20 B) ± 2 C) i - j D) i - j E) 4 i - 2 jF(P) is equal to:


A) 20
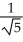B) ± 2 The directional derivative of a function F(x,y) at a point P in the direction of the unit vector- i + j is equal to -4, while the directional derivative at P in the direction of the unit vector i+ j is equal to0. The value of F(P) is equal to: A) 20 B) ± 2 C) i - j D) i - j E) 4 i - 2 j
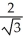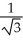C) The directional derivative of a function F(x,y) at a point P in the direction of the unit vector- i + j is equal to -4, while the directional derivative at P in the direction of the unit vector i+ j is equal to0. The value of F(P) is equal to: A) 20 B) ± 2 C) i - j D) i - j E) 4 i - 2 j i - The directional derivative of a function F(x,y) at a point P in the direction of the unit vector- i + j is equal to -4, while the directional derivative at P in the direction of the unit vector i+ j is equal to0. The value of F(P) is equal to: A) 20 B) ± 2 C) i - j D) i - j E) 4 i - 2 j j
D) The directional derivative of a function F(x,y) at a point P in the direction of the unit vector- i + j is equal to -4, while the directional derivative at P in the direction of the unit vector i+ j is equal to0. The value of F(P) is equal to: A) 20 B) ± 2 C) i - j D) i - j E) 4 i - 2 j i - The directional derivative of a function F(x,y) at a point P in the direction of the unit vector- i + j is equal to -4, while the directional derivative at P in the direction of the unit vector i+ j is equal to0. The value of F(P) is equal to: A) 20 B) ± 2 C) i - j D) i - j E) 4 i - 2 j j
E) 4 i - 2 j

Correct Answer:

verifed

Verified

Unlock this answer now
Get Access to more Verified Answers free of charge

Related Questions

Q12: Find f<sub>11</sub>(x, y) and f<sub>22</sub>(x, y) if

Q13: Use the linearization of f(x,y) = x<sup>3</sup>

Q14: Let h(t) = f(x, y), where f(x,

Q15: Find the Maclaurin polynomial of degree 5

Q16: Find the domain of the function f(x,

Q18: Find the equation of the plane tangent

Q19: z = <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB9661/.jpg" alt=" z

Q20: <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB9661/.jpg" alt=" A)

Q21: Find the equation of the tangent plane

Q22: Find the differential of the function f(x,