Multiple Choice
To construct a confidence interval for the difference of two population proportions the samples must be independently obtained random samples, both must consist of less than 5% of the population, and
A) only one of 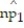 (1 -
(1 - 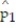 ) ? 10 or
) ? 10 or 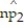 (1 -
(1 -  ) ? 10 must be true.
) ? 10 must be true.
B) 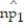 (1-
(1- 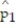 )
) 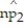 (1-
(1-  ) ?100.
) ?100.
C) both 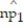 (1 -
(1 - 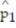 ) ? 10 and
) ? 10 and 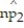 (1 -
(1 -  ) ? 10 must be true.
) ? 10 must be true.
D) 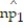 (1-
(1- 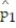 ) +
) + 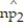 (1-
(1-  ) ?20.
) ?20.
Correct Answer:

Verified
Correct Answer:
Verified
Q54: What is the <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB10223/.jpg" alt="What is
Q55: Perform the appropriate hypothesis test.<br>-The table below
Q56: A football coach claims that players can
Q57: Find the test statistic, z, to test
Q58: A new weight-reducing technique, consisting of a
Q60: Data sets A and B are dependent.
Q61: Test the hypothesis that <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB10223/.jpg" alt="Test
Q62: Data sets A and B are dependent.
Q63: Test the hypothesis that <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB10223/.jpg" alt="Test
Q64: Perform the appropriate hypothesis test.<br>-A random sample