Multiple Choice
Area of a Triangle. A formula for finding the area of a triangle when given the measures of the angles and one side is area =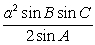
where a is the length of the side opposite angle A. If the measures of angles B and C are26° and 61° , respectively, and if a = 19 feet, use the appropriate product-to-sum identity to change the formula so that you can solve for the area of a triangle. Round your answer to two decimal places.
A) 69.30 ft2
B) 3.65 ft2
C) 138.60 ft2
D) 69.11 ft2
Correct Answer:

Verified
Correct Answer:
Verified
Q7: Solve the given trigonometric equation exactly over
Q8: Write cos 1.2x + cos 0.6x as
Q9: Use a calculator to evaluate the expression.
Q10: Solve the given trigonometric equation exactly over
Q11: Simplify the trigonometric expression<br><img src="https://d2lvgg3v3hfg70.cloudfront.net/TB10663/.jpg" alt="Simplify the
Q13: Use a half-angle identity to find tan<br>
Q14: Use a half-angle identity to find the
Q15: Verify the trigonometric identity <br> <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB10663/.jpg"
Q16: Write <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB10663/.jpg" alt="Write as
Q17: Write sin 3x cos 8x as a