Multiple Choice
Introduce slack variables as necessary and write the initial simplex tableau for the problem.
-Maximize
Subject to:

A) 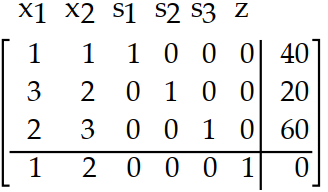
B) 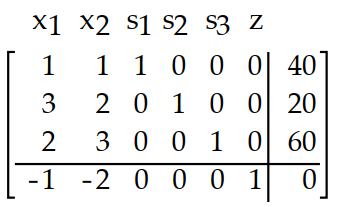
C) 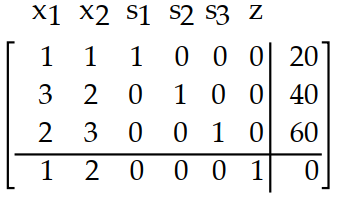
D) 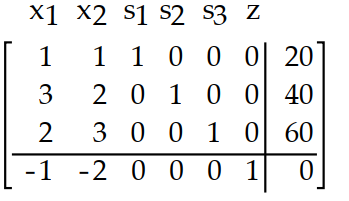
Correct Answer:

Verified
Correct Answer:
Verified
Related Questions
Q74: Use duality to solve the problem.<br>-Minimize
Q75: A manufacturer of wooden chairs and
Q76: Find the value(s) of the function,
Q77: Use graphical methods to solve the
Q78: Rewrite the system of inequalities, adding
Q80: Graph the linear inequality.<br>- <span class="ql-formula"
Q81: Use the two-stage method to solve.<br>-Minimize
Q82: Solve the problem.<br>-The manager of a concert
Q83: Use the two-stage method to solve.<br>-Maximize
Q84: A toy making company has at